PSD analysis and control strategy of optical component polishing
-
摘要:
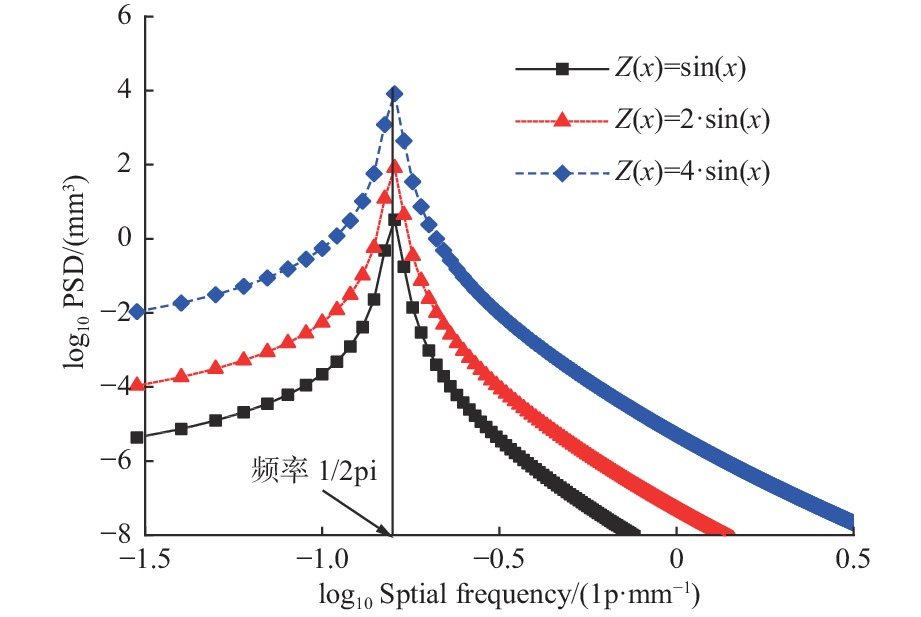
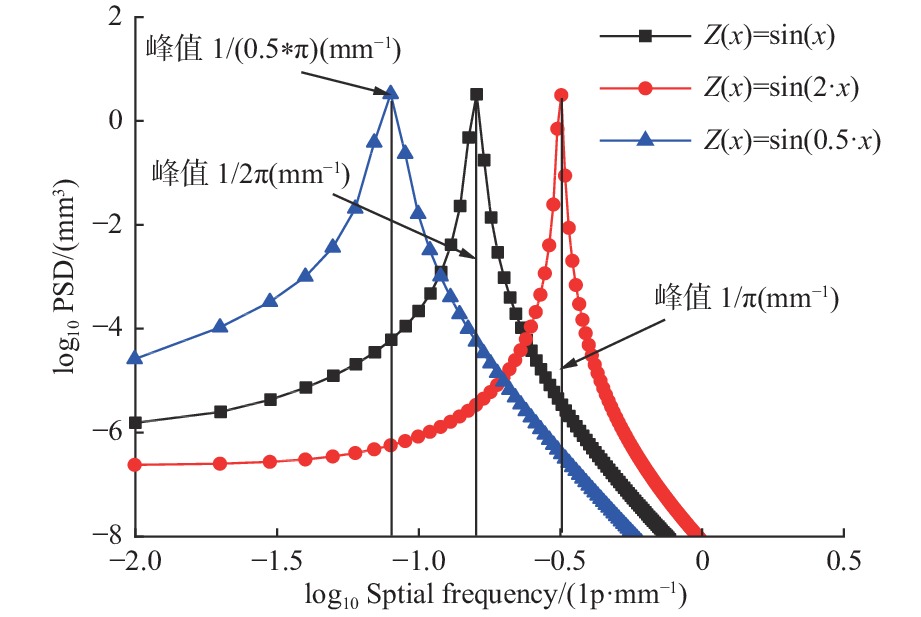
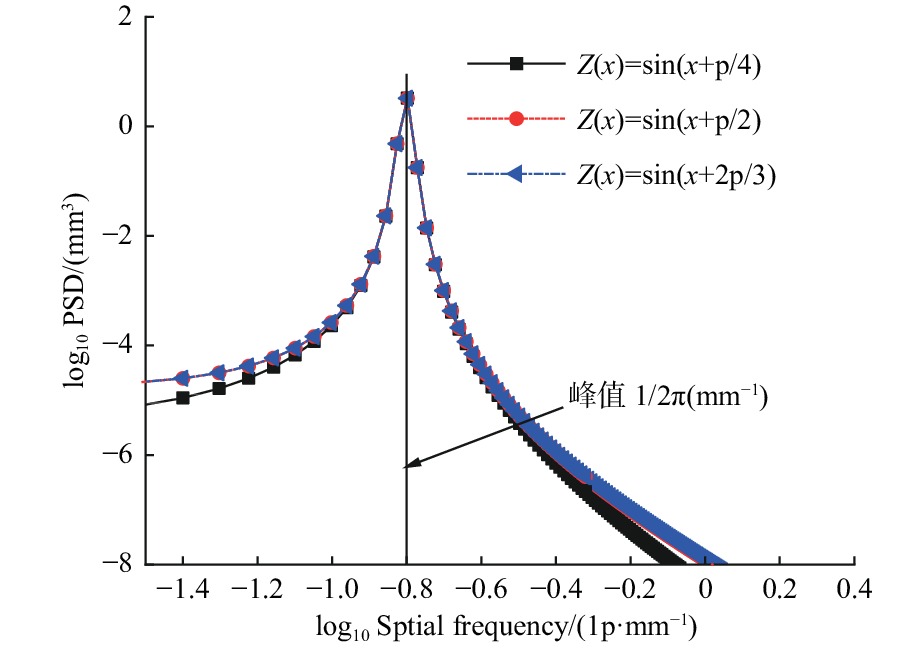
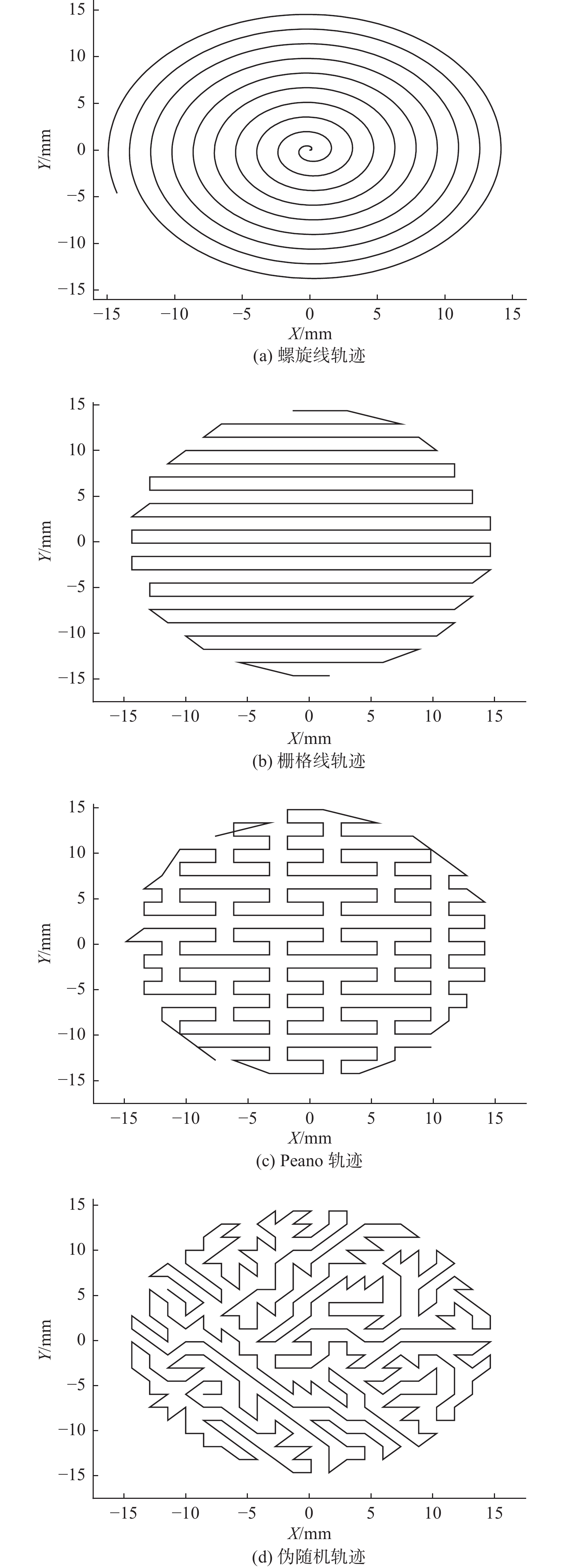
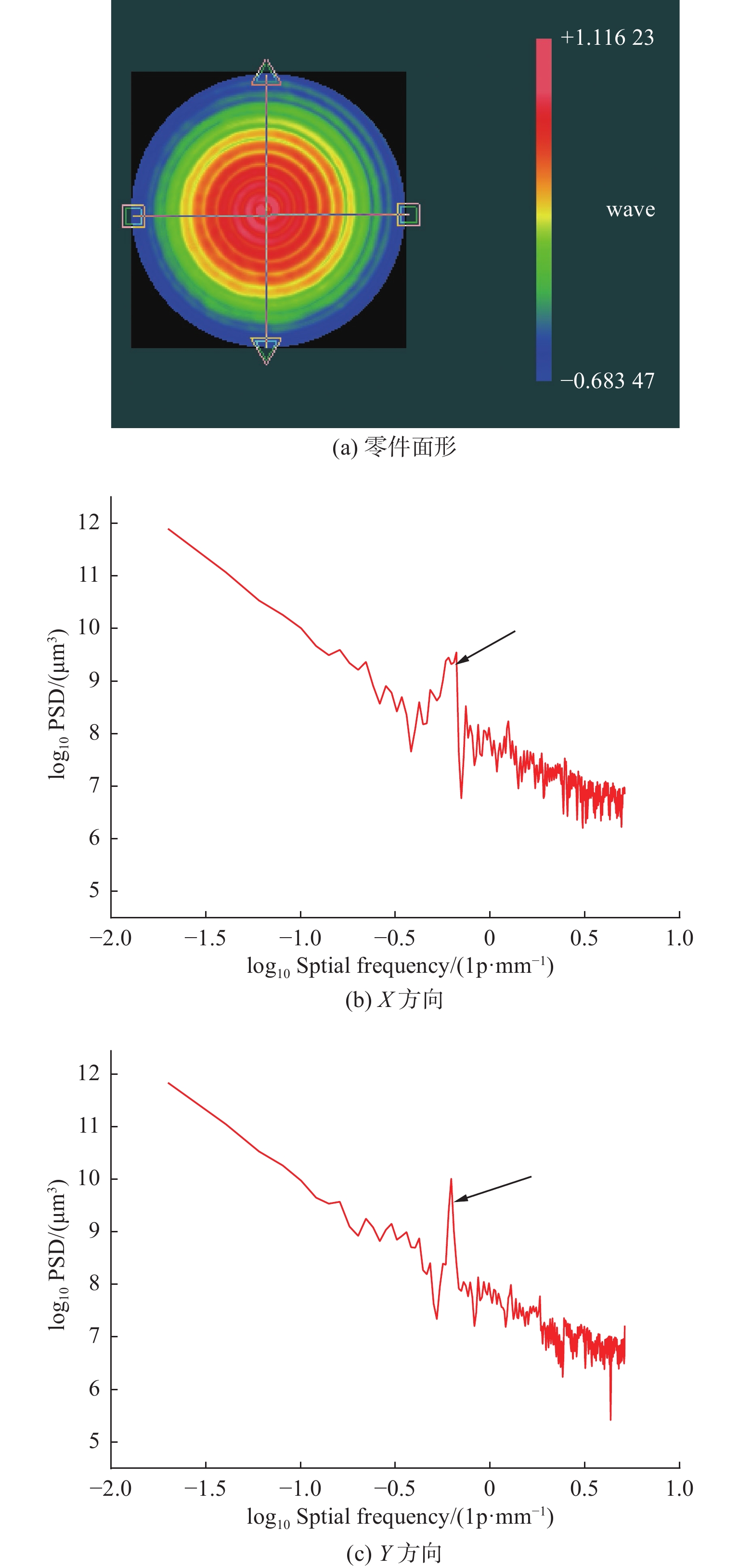
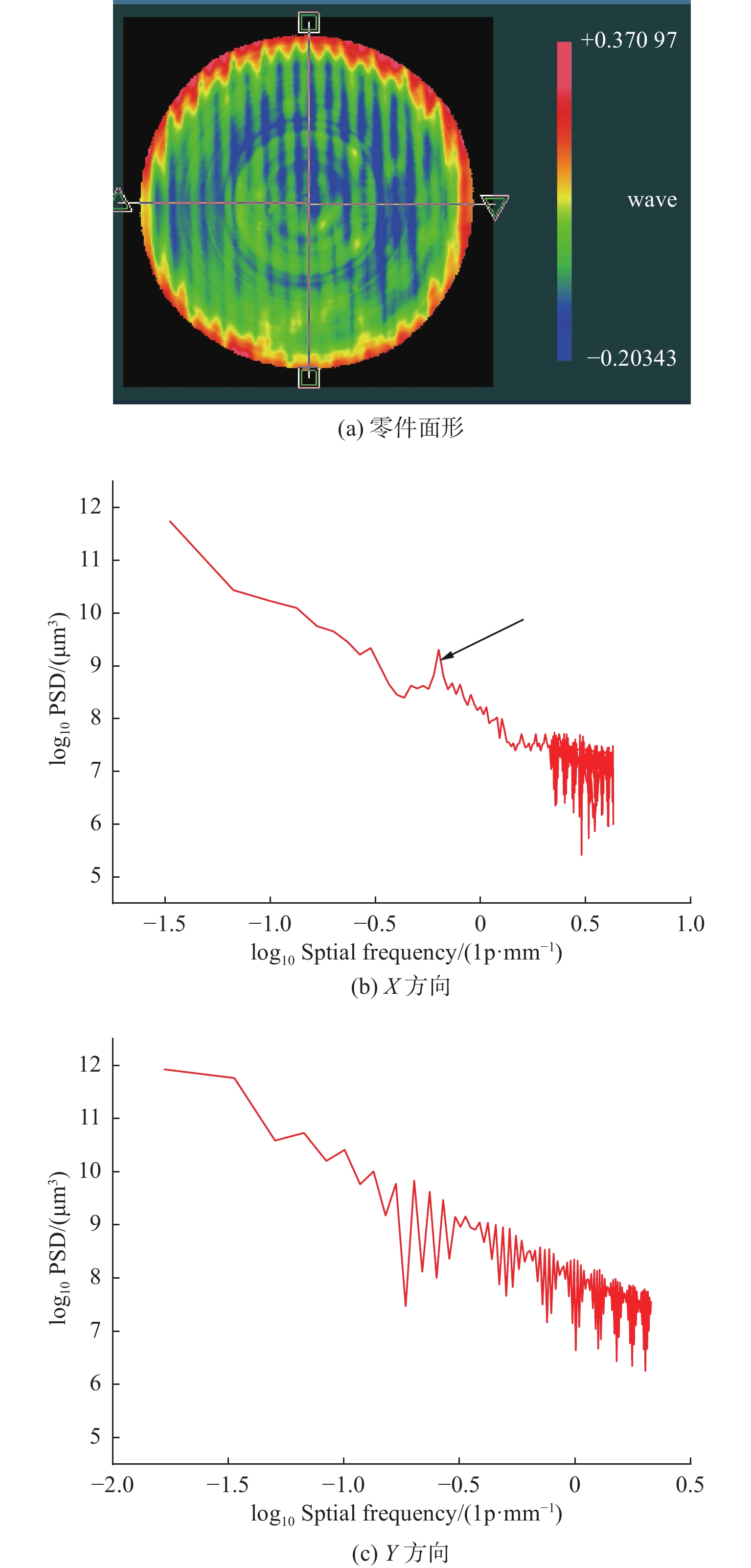
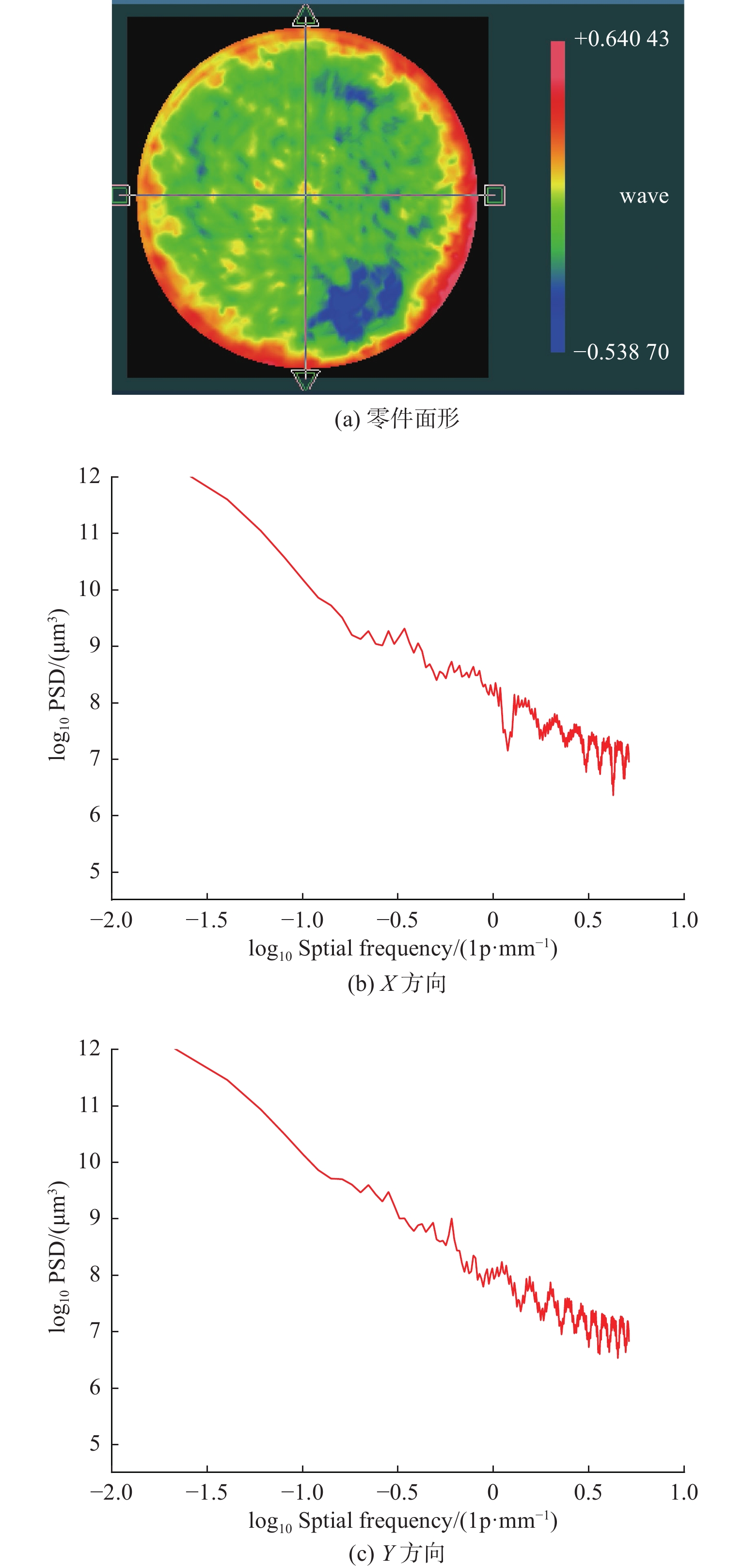
在光学加工领域，采用功率谱密度（power spectral density, PSD）对误差频谱方面信息进行表征，但是功率谱密度是表面误差统计信息，不如峰谷值 (peak-valley，PV)和均方根值(root mean square，RMS)直观。为了分析功率谱密度与工艺参数之间的关系，该文从PSD定义出发，分析了随机面形轮廓不同参数对光学PSD的影响规律，总结了PSD控制的要点，在平面玻璃上对数控抛光典型路径下加工的PSD曲线进行分析。分析结果表明：PSD与随机轮廓幅值、频率分布有关，相位对它几乎无影响；在RMS接近情况下，PSD线性拟合斜率和RMS Slope随随机轮廓的自相关长度增加而下降；短程加工路径相较于长程有序路径能够有效抑制PSD曲线峰值，使得光学元件符合频谱抑制要求。
Abstract:In the field of optical fabrication, the power spectral density (PSD) is used to characterize the error spectrum. However, the PSD is the statistical information of surface error, which is not as intuitive as peak-valley (PV) and root mean square (RMS). In order to analyze the relationship between PSD and process parameters, based on the definition of PSD, the influence of different parameters of random surface contour on optical PSD was analyzed, and summarized the key control points of PSD. Then, the PSD curves processed under the typical paths of numerical control polishing were analyzed on flat glass. The results show that the PSD is correlated with the amplitude and frequency distribution of random contour, and the phase has almost no influence on it. When the RMS is close, the slope of PSD linear fitting and RMS Slope decreases with the increase of the auto-correlation length of the random profile. The short-range machining path can effectively suppress the peak value of PSD curve compared with the long-range ordered path, which makes the optical element meet the requirements of spectrum suppression.
-
引言
高光谱遥感器是指利用分光技术获取连续窄带光谱的遥感器，其通道光谱带宽Δλ与中心波长λ的关系为Δλ/λ≈0.01[1]。在高光谱遥感器使用前，需要进行光谱定标和辐射定标。光谱定标的目的是建立高光谱遥感器光谱通道与不同入瞳波长的对应关系，辐射定标时建立高光谱遥感器的输出值与相应已知的、用国际单位(SI)表示的辐射量基准之间定量关系[2-3]。光谱定标和辐射定标是保证高光谱遥感器获取数据的科学可信度和最大限度的定量化应用基础。
高光谱遥感器的光谱定标和辐射定标通常在实验室内进行，其中光谱定标通常利用基于元素特征谱的光谱定标参考光源[4](低压汞灯和高压钠灯等特征谱线灯安装在积分球里面)或经过波长校准的单色仪或可调谐激光器[5]等精细扫描的方法实现，辐射定标通常采用光谱平坦性较好的标准灯板系统或传统卤素灯积分球光源完成。由于高光谱遥感器工作环境复杂，通常需要在野外进行观测，尤其是长期观测，受本身老化和外界干扰等因素的影响，其光谱中心波长漂移会发生漂移，其辐射响应特性会不断衰减[6]。前期高光谱遥感器应用研究表明，中心波长漂移与通道光谱带宽比值在超出1%情况下，在光谱形状变化剧烈的谱段，辐射测量的偏差有可能超过5%，甚至达到20%[7]。因此有必要对高光谱遥感器光谱辐射定标精度在现场环境下进行精度验证，实现光谱定标精度优于±0.5 nm，确保辐射定标不确定小于5%。
目前在现场环境下，可利用大气廓线中的太阳夫琅禾费线以及氧气、二氧化碳和水汽等气体吸收光谱特征对高光谱遥感器进行光谱定标精度验证，例如Gao等人采用光谱匹配技术实现了机载高光谱成像光谱仪PHILLS的光谱定标[8-9]。通过选择大气干洁、地表开阔的场地，利用太阳光经过不同反射率漫反射参考板或靶标的反射信号对高光谱遥感器进行辐射定标精度验证[10-11]。这种定标方法较精确，但是在实际操作中，因为很难能找到稳定、晴好、无云的天气条件，进而导致定标数据质量下降。
现阶段尚未有高精度的光谱定标和辐射定标实现现场环境下光谱辐射定标验证方法。本文设计了一种高光谱遥感器现场光谱辐射定标精度的验证方法，以SVC光谱辐亮度计为例，利用新型具有高稳定性的光谱可调积分球参考光源STIS(a spectrum-tunable reference light source) [12]，在待验证波段内输出光谱辐亮度形状单调上升或单调下降状态，通过比对多波段辐亮度标准传递探测器MRSTD(a muti-band radiance standard transfer detector)[11]和SVC光谱辐亮度计辐亮度测量值的相对偏差，实现SVC光谱辐亮度计光谱辐射定标的精度验证和校正，其中光谱定标验证精度小于±0.2 nm, 辐射定标验证精度优于5%。
1 精度验证的方法
本文使用STIS和MRSTD验证高光谱光学遥感器实验室光谱定标精度。STIS采用多种波长的LED密集阵列发光单元和卤钨灯发光单元，在400 nm~800 nm具有分立波段输出功能，可输出半高带宽约20 nm~40 nm近似高斯形状的光谱辐亮度状态。STIS采用循环水冷温控和高精度可编程直流电源驱动技术，保障了光源的稳定性。STIS角度均匀性在±60°以内优于99.4%，面均匀性优于99.5%，2 h内稳定性优于99.8%。STIS积分球内径250 mm，出光口直径为60 mm，如图 1所示。
MRSTD是基于低温辐射计传递的多波段辐亮度标准传递探测器[13]，用于绝对辐射定标以及监测参考目标的辐射特性变化。MRSTD的工作波段有412 nm、443 nm、490 nm、520 nm、565 nm、670 nm、750 nm和865 nm，其通道带宽约为(10±2)nm。MRSTD采用激光导入积分球的单色面光源进行光谱辐射定标，其中心波长定标准确度优于±0.1 nm，其绝对不确定度 < 1.26%。
设计的高光谱遥感器光谱定标的精度验证方法如下：在MRSTD各通道的带内区域，利用STIS输出光谱形状单调上升和单调下降的光谱辐亮度状态。使用MRSTD和SVC光谱辐亮度计交替测量STIS输出的光谱辐亮度，如图 2所示。通过比较MRSTD和SVC光谱辐亮度计辐亮度测量结果的相对偏差，分析SVC光谱辐亮度计的光谱辐射定标精度。
利用标准传递探测器第i通道响应DNi和其带内响应度为Ri，测量光谱可调积分球参考光源输出辐亮度Lc(λi)作为参考值，如(1)式和(2)式所示：
$$ {{L}_{c}}\left( {{\lambda }_{i}} \right)={D{{N}_{i}}}/{{{R}_{i}}}\; $$ (1) $$ {{R}_{i}}=\int_{{{\lambda }_{l}}}^{{{\lambda }_{u}}}{R\left( {{\lambda }_{i}} \right)}\text{d}\lambda $$ (2) SVC光谱辐亮度计在350 nm~1 000 nm范围内的光谱分辨率，即半高带宽优于3.5 nm，小于MRSTD通道带宽。根据前期研究[14]，SVC光谱辐亮度计通道光谱响应度一般接近于sinc函数，与MRSTD通道的类似高斯光谱响应度曲线形状不同，因此两种仪器测量的辐亮度无法直接进行比对。
解决方法：首先对SVC光谱辐亮度计测量的光谱辐亮度曲线进行线性插值，插值波长间隔为0.2 nm；其次卷积SVC光谱辐亮度计测量的光谱辐亮度Lsvc(λi)和MRSTD第i通道的相对光谱响应度R(λi)，得到光谱辐亮度计对应MRSTD第i通道辐亮度测量值LBSR(λi)：
$$ {{L}_{BSR}}\left( {{\lambda }_{i}} \right)=\int_{{{\lambda }_{l}}}^{{{\lambda }_{u}}}{{{L}_{SVC}}\left( {{\lambda }_{i}} \right)R\left( {{\lambda }_{i}} \right)}{\text{d}\lambda }/{{{R}_{i}}}\; $$ (3) 计算MRSTD的参考辐亮度Lc(λi)和SVC光谱辐亮度计辐亮度LBSR(λi)测量值之间的相对偏差Δi：
$$ {{\Delta }_{i}}=\frac{{{L}_{BSR}}\left( {{\lambda }_{i}} \right)-{{L}_{c}}\left( {{\lambda }_{i}} \right)}{{{L}_{c}}\left( {{\lambda }_{i}} \right)}\times 100% $$ (4) 当SVC光谱辐亮度计存在波长偏移时，参考辐亮度Lc(λi)和辐亮度LBSR (λi)测量值之间的相对偏差Δi差异明显。在MRSTD通道对应(10±2)nm光谱范围，SVC光谱辐亮度计的光谱带宽可默认为不发生变化。此时参考前期研究方法[8-9]，利用光谱匹配技术，即对SVC光谱辐亮度计测量的光谱辐亮度LSVC(λi)进行波长平移，判断Δi的变化情况。
根据测量不确定度合格评定通用比对标准[15]，当SVC光谱辐亮度计不存在波长偏移时，参考辐亮度Lc(λi)和辐亮度LBSR (λi)测量值的相对偏差Δi应满足下式：
$$ {\Delta _i} \le \sqrt {{u^2}\left( {{\rm{SVC}}} \right) + {u^2}\left( {{\rm{MRSTD}}} \right)}<5\% $$ (5) 式中：u(SVC)为SVC光谱辐亮度计的辐射定标不确定度；u(MRSTD)为MRSTD辐射定标不确定度。设计最大相对偏差Δi＜5%为判据，认为此时平移后的波长为SVC光谱辐亮度计真实的波长，进而验证SVC光谱辐亮度计的光谱辐射定标精度。
2 实验及数据处理
利用STIS在MRSTD第2通道的带内区域输出4种光谱辐亮度度状态，对应的峰值中心波长分别为474.5 nm、475.9 nm、496.8 nm和499.7 nm。MRSTD测量的辐射亮度值分别为4.409μW·cm-2·sr-1、6.0377μW·cm-2·sr-1、13.950 7 μW·cm-2·sr-1和13.967 7 μW·cm-2·sr-1。图 3(a)为SVC光谱辐亮度计测量的光谱辐亮度曲线。图 3(b)为MRSTD第2通道490 nm波段的相对光谱响应度曲线，其辐亮度测量值为参考值，如表 1的第一行所示。
![]()
图 3 SVC光谱辐亮度计测量的光谱辐亮度曲线和MRSTD 490 nm波段的相对光谱响应度Figure 3. Measured spectral radiance curve by SVC and relative spectral responsivity of MRSTD 490 nm band表 1 490 nm波段各波长相对偏移量(%) Table 1. Relative deviation of each wavelength in 490 nm band波长平移量/nm 相对偏差Δi 474.5 nm 475.9 nm 496.8 nm 499.7 nm 1.0 2.68 3.13 -4.65 -5.80 0.8 1.10 1.62 -3.66 -4.43 0.6 -0.47 0.14 -2.69 -3.08 0.4 -2.01 -1.32 -1.76 -1.74 0.2 -3.53 -2.76 -0.86 -0.43 0 -5.04 -4.18 0 0.85 -0.2 -6.53 -5.58 0.83 2.12 SVC光谱辐亮度计的波长不作平移时，计算MRSTD的参考辐亮度Lc(λi)和SVC光谱辐亮度计辐亮度LBSR (λi)测量值之间的相对偏差Δi最大为5.04%。将SVC波长向长波方向平移0.4 nm后，针对4种光谱辐亮度状态，计算的相对偏差Δi均小于2.01%。SVC光谱辐亮度计波长平移(长波方向为正)与相对偏差Δi的变化情况如图 4所示，相对偏差Δi变化的具体数值见表 1。
![]()
图 4 490nm波段MRSTD与SVC光谱辐亮度计波长平移的相对偏差Δi变化情况Figure 4. Changes of relative deviation of MRSTD and SVC wavelength translation in 490 nm band从表 1可以看出，针对STIS输出的474.5 nm和475.9 nm两种光谱辐亮度状态，SVC光谱辐亮度计波长平移与相对偏差Δi的变化规律趋向单调上升，对于STIS输出的496.8 nm和499.7 nm2种光谱辐亮度状态，SVC光谱辐亮度计波长平移与相对偏差Δi的变化规律呈单调下降趋势。通过两种STIS分别输出光谱形状单调上升和单调下降的两种光谱辐亮度状态，结合SVC光谱辐亮度计波长平移与MRSTD的参考辐亮度和SVC辐亮度测量值相对偏差Δi的变化规律和相对偏差Δi优于5%的判据，得到SVC光谱辐亮度计的波长偏移了0.4 nm。
SVC光谱辐亮度计760 nm波段的光谱和辐射定标精度验证情况如图 5所示，SVC的波长偏移了1.8 nm。SVC光谱辐亮度计波长平移与MRSTD的参考辐亮度和SVC辐亮度测量值相对偏差Δi的变化规律明显。760 nm波段测量与490 nm波段测量结果相比，SVC光谱辐亮度计波长平移与相对偏差Δi的变化规律呈单调上升和下降趋势较为平坦，主要是由于与峰值中心波长474.5 nm、475.9 nm、496.8 nm和499.7 nm的光谱辐亮度状态相比，STIS输出峰值中心波长748.3 nm和759.9 nm状态的光谱形状单调上升和单调下降的变化较为平缓。
![]()
图 5 760 nm波段MRSTD与SVC光谱辐亮度计波长平移的相对偏差Δi变化情况Figure 5. Changes of relative deviation of MRSTD and SVC wavelength translation in 760 nm band同样我们验证了SVC光谱辐亮度计在350 nm~800 nm光谱范围内MRSTD其余波段的光谱辐射定标精度。受制于STIS内部LED的种类，在800 nm以后无法输出半高带宽约20 nm~40 nm近似高斯形状的光谱辐亮度状态。
3 结论
本文以SVC野外光谱辐亮度计模拟高光谱遥感器，通过STIS和MRSTD设计了一种高光谱光学遥感器光谱辐射定标的精度验证方法。借助2种STIS在待验证的光谱通道内分别输出光谱形状单调上升和单调下降的两种光谱辐亮度状态，利用SVC光谱辐亮度计波长平移与MRSTD的参考辐亮度和SVC辐亮度测量值相对偏差Δi的变化规律，可有效验证SVC光谱辐亮度计光谱和辐射定标精度，其中光谱定标验证精度优于0.2 nm，辐射定标精度优于5%。这种光谱辐射定标精度验证方法可用于高光谱遥感器350 nm~800 nm光谱范围内实验室定标和现场定标，同时也为光谱连续型光学遥感器与窄带滤光片型光学遥感器之间的辐亮度测量提供一种参考方法。
-
![]()
图 4 随机频率以及幅值下降对PSD曲线影响
Figure 4. Effect of random frequency and amplitude decrease on PSD curves
![]()
图 6 PSD曲线与RMS，RMS Slope之间关系
Figure 6. Relationship between PSD curves with RMS and RMS Slope
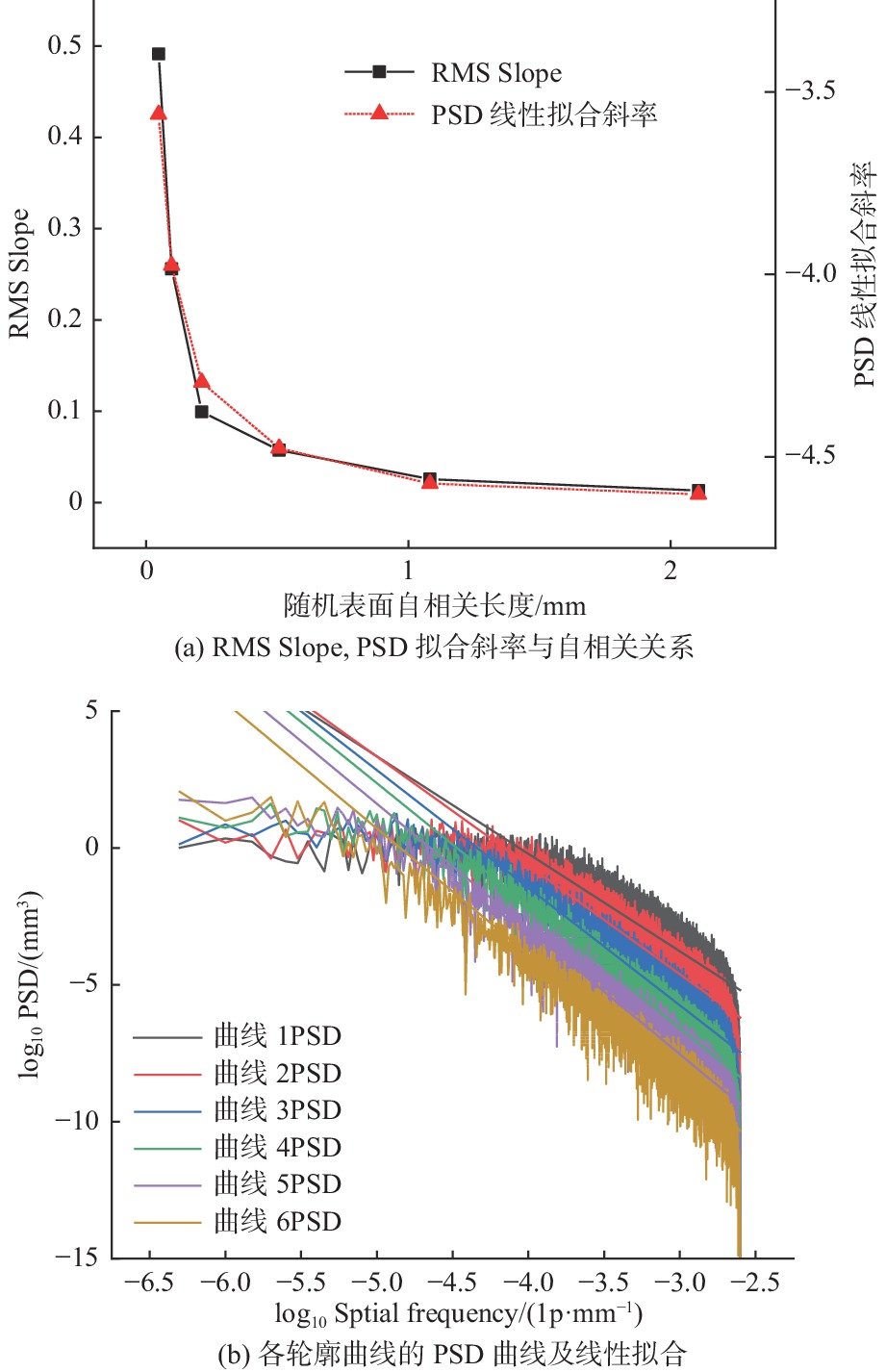
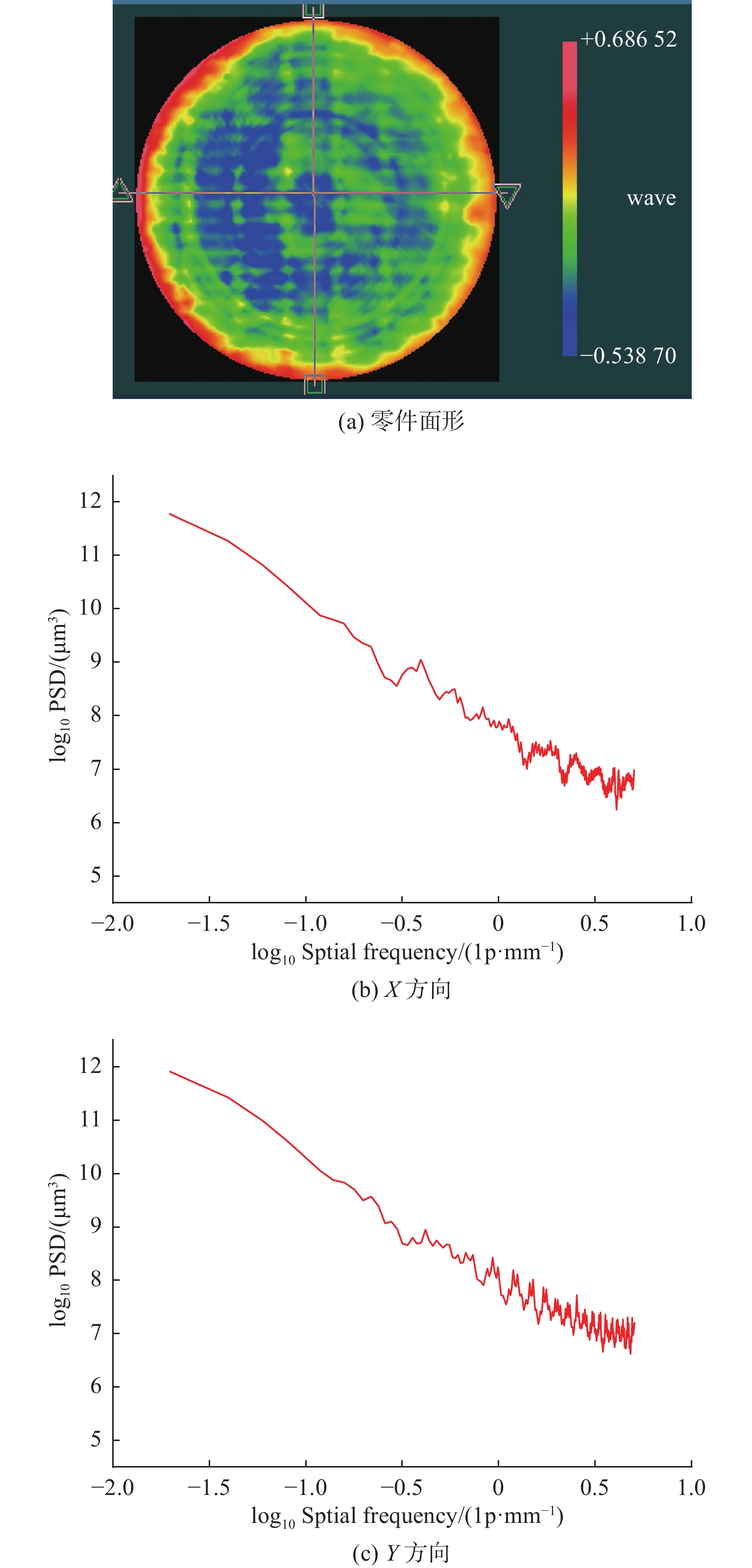
表 1 6条RMS接近的随机轮廓评价参数
Table 1 Evaluation parameters of six curves of random profile with RMS approximation
参数
名称曲线编号 1 2 3 4 5 6 RMS/mm 0.0137 0.0137 0.0136 0.0139 0.0138 0.0137 RMS
Slope0.4915 0.2561 0.0992 0.0574 0.0256 0.013 自相关
长度/mm0.0476 0.0971 0.21252 0.50755 1.0826 2.1067 PSD曲
线拟合
斜率−3.56145 −3.97554 −4.29558 −4.47599 −4.57322 −4.6028 
下载: 导出CSV
-
[1] KIMMEL R K , PARKS R E. ISO 10110 Optics and optical instruments preparation of drawings for optical elements and systems[S]. Washington DC: OSA Standards Committee, 1995.
[2] 李智钢, 鲍振军, 朱衡. 多磨头数控抛光对大口径离轴抛物面镜中频误差的抑制[J]. 强激光与粒子束,2018,30(6):41-46. doi: 10.11884/HPLPB201830.170457 LI ZhiGang, BAO Zhenjun, ZHU Heng, et al. Restraining mid-spatial-frequency error of large-size off-axis parabolic mirrors by multi-tool NC polishing[J]. High Power Laser and Particle Beams,2018,30(6):41-46. doi: 10.11884/HPLPB201830.170457
[3] 李富仁, 王贵林, 陈善勇, 等. 强光光学零件加工误差频谱分析与控制方法研究[J]. 航空精密制造技术,2013,49(4):1-4. doi: 10.3969/j.issn.1003-5451.2013.04.001 LI Furen, WANG GuiLin, CHEN Shanyong, et al. Study on spectrum characteristics and controlling method of machining errors of optical elements used in high power laser system[J]. Aviation Precision Manufacturing Technology,2013,49(4):1-4. doi: 10.3969/j.issn.1003-5451.2013.04.001
[4] 王贵林, 李完小, 向纪邦. 强光光学零件磁流变抛光误差的频谱特征与演变研究[J]. 航空精密制造技术,2019,55(1):6-9. WANG Guilin, LI Wanxiao, XIANG Jibang. Spectral feature and evolvement of machining error of high-power optical elements in magnetorheological finishing[J]. Aviation Precision Manufacturing Technology,2019,55(1):6-9.
[5] SHIRAISHI M, OSHINO T, MURAKAMI K, et al. Flare modeling and calculation on EUV optics[J]. SPIE,2010,7636:763629.
[6] KATSUHIKO M, TETSUYA O, HIROYUKI K, et al. Development progress of optics for extreme ultraviolet lithography at Nikon[J]. Journal of Micro/Nanolithography, MEMS, and MOEMS,2009,8(4):041507. doi: 10.1117/1.3238522
[7] AIKENS D M. WOLFE C R, LAWSON J K Use of power spectral density (PSD) functions in specifying optics for the national ignition facility[J]. SPIE,1995,2576:281-292.
[8] 钟波, 陈贤华, 王健, 等. 高精度离轴非球面透镜的制造与检测[J]. 红外与激光工程, 2018, 47(7): 0718003-1-8. ZHONG Bo, CHEN Xianhua, WANG Jian. Fabrication and test of high-precision off-axis aspheric lens[J]. Infrared and Laser Engineering. 2018, 47(7): 0718003-1-8.
[9] 陈建超. 超精密加工表面粗糙度测量方法对比及功率谱密度评价[D]. 哈尔滨: 哈尔滨工业大学, 2009. CHEN Jianchao. Comparison of ultra precision machined surface roughness measurement methods and power spectral density characterization[D]. Harbin: Harbin Institute of Technology, 2009.
[10] 张昊宇, 钟波, 赵世杰, 等. 光学表面非均匀中频误差的评价与修正[J]. 应用光学,2015,36(2):295-299. ZHANG Haoyu, ZHONG Bo, ZHAO Shijie, et al. Evaluation and modification of nonuniform mid-spatial frequency error in optic surface[J]. Journal of Applied Optics,2015,36(2):295-299.
[11] LIAO D, YUAN Z, TANG C, et al. Mid-spatial frequency error (PSD-2) of optics induced during CCOS and full-aperture polishing[J]. Journal of the European Optical Society,2013,8:13031-13035. doi: 10.2971/jeos.2013.13031
[12] ALCOCK S G, LUDBROOK G D, OWEN T, et al. Using the power spectral density method to characterize the surface topography of optical surfaces[J]. SPIE,2010,7801:780108.
[13] SEMI. MF1811-97:Guide for estimating the power spectral density function and related finish parameters from surface profile data[S]. Washington DC: ASTM , 2016.
[14] MA Zhanlong, PENG Lirong, WANG Junlin. Ultra-smooth polishing of high-precision optical surface[J]. Optik,2013,124(24):6586-6589. doi: 10.1016/j.ijleo.2013.05.093
[15] DUNN C, WALKER D D, BEAUCAMP A. et al, Improving surface PSD using a random tool path[J]. Frontiers in Optics,2008:OThB5.
[16] TIAN Huang, ZHAO Dong, CAO Zhongchen. Trajectory planning of optical polishing based on optimized implementation of dwell time[J]. Precision Engineering,2020,62:223-231. doi: 10.1016/j.precisioneng.2019.12.006
[17] BERGSTRÖM D. Rough surface generation & analysis [EB/OL]. ( 2012-2-26) [2022-6-5]. http://www.mysimlabs.com/surface_generation.html
-
期刊类型引用(8)
1. 张嘉显，王军，曹召良. 基于粒子群算法的高均匀性接触角测量光源研究. 激光与光电子学进展. 2025(03): 186-192 . 
百度学术
2. 吴铭扬，张巧芬，王桂棠，吴黎明，邓耀华. 基于LED反光杯系统的优化研究. 应用光学. 2024(02): 329-336 . 本站查看
3. 何慧萌，杨婷，施会丽，王平，邴喆，王星，白勃. 考虑HPSA的IRS辅助室内VLC系统光源优化. 西安电子科技大学学报. 2024(02): 46-55 . 百度学术
4. 徐承军，李嘉群，张鹏. 港口高压开关柜局部放电信号自适应小波去噪方法. 起重运输机械. 2024(11): 67-75 . 百度学术
5. 李谱林，吴玲玲，陈靖，占春连，余欢. 大面积高均匀照明的LED阵列设计. 激光与光电子学进展. 2024(13): 368-377 . 百度学术
6. 汤雄，王鹏，吕玲玲. LED植物照明灯具技术发展及应用现状. 应用技术学报. 2023(02): 120-124 . 百度学术
7. 曹震，巢渊，徐魏，杜帅帅，张敏. 基于改进梯度加权的零件图像高精度聚焦方法. 电子测量与仪器学报. 2023(11): 132-142 . 百度学术
8. 樊华羽，李典，郝海兵，梁益华. 基于Alpha-stable的粒子群算法变异策略研究及气动优化应用. 西北工业大学学报. 2022(06): 1385-1393 . 百度学术
其他类型引用(5)
计量
- 文章访问数: 539
- HTML全文浏览量: 283
- PDF下载量: 126
- 被引次数: 13
陕公网安备 61011302001501号 