Laser triangulation measurement model based on least square polynomial fitting method
-
摘要: 激光三角法具有非接触测量、测量范围大、相对测量精度高、结构简单、环境适应性强等多种优点, 得到了广泛应用。但是三角测量的理论公式具有非线性特征, 而且光学结构参数(a、b、θ)等在现实工程中具有不可测性。研究了三角测量中数学模型的建立方法, 选用多项式展开方法建立数学模型。通过应用最小二乘法拟合多项式的方法求解模型系数, 提出了根据最大相对拟合残差要求、结合相关系数用于控制拟合多项式阶数的评价方法, 并通过实际光学系统验证了该方法的可行性, 达到了0.01%的相对误差。最小二乘法拟合多项式的方法对于激光三角位移传感器的标定和系统误差消除具有实际的指导意义。Abstract: The laser triangulation method has been widely applied due to its advantages in non-contact measurement, large measurement range, high accuracy in measurement, simple structure, high adaptability, etc. However, the theoretical formula of laser triangulation is nonlinear and the parameters (a, b, θ) of optic structure in practical engineering is immeasurable. Therefore, we investigated the construction approach of the mathematical model in triangulation, and adopted the polynomial expansion approach to build the model. Under the application of least square fitting polynomial method of the solution to the model coefficient, we proposed a evaluation method according to the requirements of the maximum relative fitting residual combined with the relative coefficients for controlling the fitting polynomial order. The feasibility of the method was verified by the practical optical system, with the relative error of 0.01%. The method of least square fitting polynomial has practical guiding significance for the calibration of laser triangular displacement sensor and the elimination of systematic error.
-
-
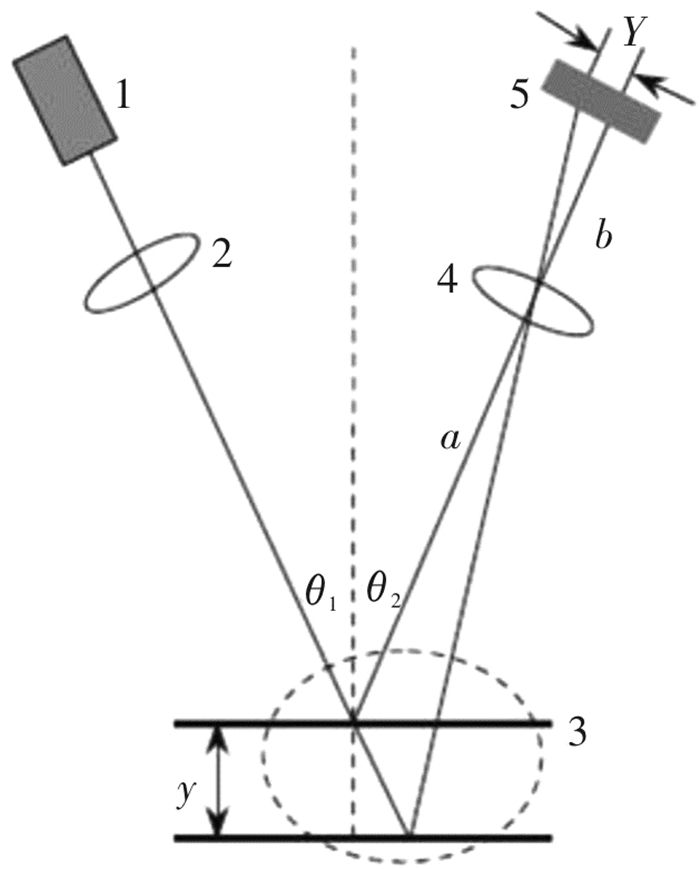
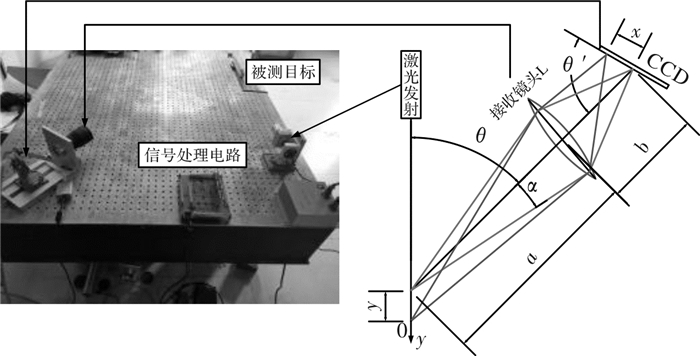
表 1 光学结构数据
Table 1 Optical structure data
序号 工作距离L/mm 测量范围W/mm 物镜焦距f/mm 夹角/(°) CCD类型 测量误差/mm 1 720 80 172 45 TCD1501 0.03 2 980 80 213 45 TCD1501 0.03 表 2 实验原始数据
Table 2 Experimental raw data
序号 1 2 3 4 5 6 7 8 9 1#像点位置xi 27 376 23 965 20 669 17 495 14 423 11 456 8 585 5 796 3 096 2#像点位置xi 24 387 22 303 20 162 17 976 15 742 13 443 11 093 8 682 6 208 物点位置yi/mm 0 10 20 30 40 50 60 70 80 表 3 最终计算结果
Table 3 Final calculation results
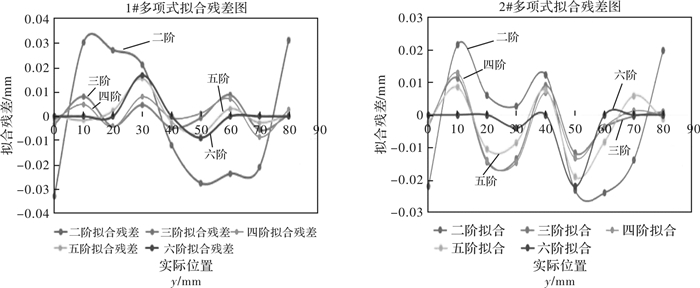
1# |P1(x)-yi|2 |P2(x)-yi|2 |P3(x)-yi|2 |P4(x)-yi|2 |P5(x)-yi|2 |P6(x)-yi|2 决定系数r2/% 99.890 727 77 99.999 915 86 99.999 996 10 99.999 996 92 99.999 999 49 100.000 000 00 最大相对残差ε/% 1.735 535 09 0.041 035 72 0.011 040 64 0.010 637 10 0.003 930 16 0.000 000 51 2# |P1(x)-yi|2 |P2(x)-yi|2 |P3(x)-yi|2 |P4(x)-yi|2 |P5(x)-yi|2 |P6(x)-yi2 决定系数r2/% 99.942 352 08 99.999 960 38 99.999 992 35 99.999 992 56 99.999 993 91 100.000 000 00 最大相对残差ε/% 1.270 742 99 0.029 958 82 0.018 145 74 0.017 229 01 0.013 162 22 0.000 007 80 -
[1] 黄战华, 蔡怀宇, 李贺桥, 等.三角法激光测量系统的误差分析及消除方法[J].光电工程, 2002, 29(3):58-61. http://d.old.wanfangdata.com.cn/Periodical/gdgc200203016 HUANG Zhanhua, CAI Huaiyu, LI Heqiao, et al. Analysis for error in triangular laser measurement system and an elimination method[J].Opto-Electronic Engineering, 2002, 29(3):58-61. http://d.old.wanfangdata.com.cn/Periodical/gdgc200203016
[2] 任伟明, 孙培懋, 王亚雷.一种标定三角测量法激光位移计的方法[J].光学技术, 1997(3):10-15. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199700150183 REN Weiming, SUN Peimao, WANG Yalei, et al. A calibration method for laser displacement system based on triangulation[J].Optical Tachnology, 1997(3):10-15. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199700150183
[3] 何凯, 陈星, 王建新.高精度激光三角位移测量系统误差分析[J].光学与光电技术, 2013, 11(3):62-66. http://d.old.wanfangdata.com.cn/Periodical/gxygdjs201303014 HE Kai, CHEN Xing, WANG Jian, et al.Analysis of error in high precision laser triangulation displacement measurement sysytem[J].Optics & Optoelectronics Technologe, 2013, 11(3):62-66. http://d.old.wanfangdata.com.cn/Periodical/gxygdjs201303014
[4] 白福忠, 张小艳, 刘珍, 等.激光三角法中工作角对系统灵敏度的影响[J].应用光学, 2017, 38(5):826-830. http://www.yygx.net/CN/abstract/abstract11021.shtml BAI Fuzhong, ZHANG Xiaoyan, LIU Zhen, et al.Effect of working angle on system sensitivity in laser triangulation[J].Journal of Applied Optics, 2017, 38(5):826-830. http://www.yygx.net/CN/abstract/abstract11021.shtml
[5] 冯俊艳, 冯其波, 匡萃方.高精度激光三角位移传感器的技术现状[J].应用光学, 2004, 25(3):33-36. http://www.yygx.net/CN/abstract/abstract8967.shtml FENG Junyan, FENG Qibo, KUANG Cuifang.Present status of high precision laser displacement sensor based on triangulation[J].Journal of Applied Optics, 2004, 25(3):33-36. http://www.yygx.net/CN/abstract/abstract8967.shtml
[6] 李田泽, 王生德, 卢恒炜, 等.光电位置敏感器件的非线性分析及应用[J].应用光学, 2006, 27(5):400-403. http://www.yygx.net/CN/abstract/abstract8942.shtml LI Tianze, WANG Shengde, LU Hengwei, et al. Nonlinearity analysis and application of optoelectronic position sensitive detector[J].Journal of Applied Optics, 2006, 27(5):400-403. http://www.yygx.net/CN/abstract/abstract8942.shtml
[7] 金攀, 屠大维, 张旭.水下同步扫描三角测距成像理论建模及仿真分析[J].应用光学, 2018, 39(6):849-855. http://www.yygx.net/CN/abstract/abstract11195.shtml JIN Pan, TU Dawei, ZHANG Xu.Theoretical modeling and simulation analysis of underwater synchronous scanning triangulation imaging system[J].Journal of Applied Optics, 2018, 39(6):849-855. http://www.yygx.net/CN/abstract/abstract11195.shtml
[8] 陈桂秀.用程序求解最小二乘拟合多项式的系数[J].青海师范大学学报(自然科学版), 2010(3):14-17. http://d.old.wanfangdata.com.cn/Periodical/qhsfdxxb-zrkx201003004 CHEN Guixiu. Solve the least square curve fitting polynomial coefficient with program[J].Journal of Qinghai Norm al University(Natural Science), 2010(3):14-17. http://d.old.wanfangdata.com.cn/Periodical/qhsfdxxb-zrkx201003004
[9] 王伟斌, 邱长泉.基于最小二乘曲线拟合的信号调理电路误差补偿方法[J].计算机测量与控制, 2009, 17(11):2286-2291. http://d.old.wanfangdata.com.cn/Periodical/jsjzdclykz200911057 WANG Weibin, QIU Changquan. Compensating error of signal conditioning circuit based on least square curve fitting[J].Computer Measurement & Control, 2009, 17(11):2286-2291. http://d.old.wanfangdata.com.cn/Periodical/jsjzdclykz200911057
[10] 卢治功, 刘先礼, 职连杰.一种激光自动测长仪的测量方法: 中国, CN1307225[P].2001-08-08. LU Zhigong, LIU Xianli, ZHI Lianjie, et al. The invention relates to a measuring method of laser automatic length measuring instrument: China, CN 1307225[P].2001-08-08.
[11] 阿克塞尔多涅斯, 莱因霍德诺尔.激光测量技术: 原理及应用[M].张书练, 译.武汉: 华中科技大学出版社, 1997. AXELl Dongs, REINHARD Noll.Laser measurement technology: Fundmamentals AND applications[M].ZHANB Shulian, translation.Wuhan: China University of Science and Technology Press, 1997.
[12] 孙长库, 叶声华.激光测量技术[M].天津:天津大学出版社, 2000. SUN Changku, YE Shenghua.Laser measurement trchnology[M].Tianjin: Tianjin University Press, 2000.
[13] 迟泽英, 陈文建.应用光学与光学设计基础[M].北京:高等教育出版社, 2017. CHI Zeying, CHEN Wenjian.Applied optics and elements of optical design[M].Beijing: Higher Education Press, 2017.
[14] 焦明星, 冯其波, 王鸣等[M].北京: 科学出版社, 2014. JIAO Mingbo, FENG Qingbo, WANG Ming, et al.Sensing and measuring with lasers[M].Beijing: Science Press, 2014.
[15] STEVEN C, CHAPRA, RAYMOND P Canale.Numerical methods for engineers[M].Fifth Edition.Beijing:Tsinghua University Press, 2007.
-
期刊类型引用(22)
1. 何坤,杜庆庆,刘纪阳,范吉鹏,郑金星,袁昊,奚维斌. 基于PPMS的低温温度计标定及应用研究. 低温与超导. 2025(01): 64-70 .  百度学术
百度学术
2. 秦峰,张雷,尚玉龙. 基于单目视觉的变焦测距方法. 江苏理工学院学报. 2024(02): 83-92 .  百度学术
百度学术
3. 白辉全,高德东,宋艳青. 基于图像处理的柔性穿刺针多种运动轨迹模型建立与比较. 中国医学物理学杂志. 2024(06): 710-715 .  百度学术
百度学术
4. 李志鹏,王慧,周德亮,王岩,苏润丰,李绍和,涂学凑,陈健,吴培亨. 双焦点太赫兹主动成像系统的扫描轨迹. 激光与光电子学进展. 2023(12): 283-287 .  百度学术
百度学术
5. 陈昊,张达. 基于混合卷积窗的激光多普勒信号处理研究. 激光与光电子学进展. 2023(17): 288-300 .  百度学术
百度学术
6. 芦颖,王泽民,杨博瑞. 基于畸变偏移矢量的结构光区域标定. 激光与光电子学进展. 2022(02): 332-339 .  百度学术
百度学术
7. 乔健,吴阳,陈能达,陈为林,杨景卫. 高反射带材传输空间位置检测系统. 光学精密工程. 2022(11): 1301-1309 .  百度学术
百度学术
8. 樊鹏辉,杨光永,晏婷,戈一航,徐天奇. 激光三角法测距传感器结构设计与参数优化的研究. 计算机与数字工程. 2022(09): 2074-2078 .  百度学术
百度学术
9. 何高清,肖健. 轴承尺寸检测数据的异常值检测与数据处理研究. 机电工程. 2021(02): 198-203 .  百度学术
百度学术
10. 樊鹏辉,杨光永,程满,刘叶,徐天奇. 基于线性补偿的测距传感器结构设计与参数优化. 单片机与嵌入式系统应用. 2021(02): 82-85 .  百度学术
百度学术
11. 沈春和,文贤馗,曾癸森,苏立,毛成,李林峰. 基于大数据技术的小水电机组负荷分配优化平台研究及应用. 电力大数据. 2021(01): 9-16 .  百度学术
百度学术
12. 郑锴,郑献民,殷少锋,林宏旭. 基于最小二乘拟合的无人机运动目标测速方法. 兵器装备工程学报. 2021(03): 61-64 .  百度学术
百度学术
13. 储政勇,王俊. 激光雷达测量误差的分析与修正——以电科38所出品的激光雷达为例. 科技与创新. 2021(07): 18-20 .  百度学术
百度学术
14. 尹君,于健. 基于BIM技术的任意油箱剩余油量计算方法. 土木建筑工程信息技术. 2021(01): 77-82 .  百度学术
百度学术
15. 宫海晓,贺杰,杨秋慧. 机载激光雷达扫描三维地形的渲染研究. 激光杂志. 2021(04): 179-182 .  百度学术
百度学术
16. 王闯,武君胜,周光辉,张凯. AdaBoost算法使能的刀具磨损状态分阶段评估集成方法. 机械科学与技术. 2021(05): 727-733 .  百度学术
百度学术
17. 汪洋浪,王科未,邹斌. 基于粒子群优化算法的激光雷达实时检测隧道中心线. 激光与光电子学进展. 2021(03): 296-304 .  百度学术
百度学术
18. 肖欣招,刘建旭,伍国靖,付东翔. 三维流场测量中的微风量多传感器数据融合. 电子科技. 2021(12): 81-86 .  百度学术
百度学术
19. 杨梅梅,赵佩瑶,冯斌,赵峰. 偏振滤光结合多项式拟合的水面太阳耀光抑制方法. 激光与光电子学进展. 2021(24): 125-136 .  百度学术
百度学术
20. 唐佳林,张冲,郭岩锋,苏秉华,苏清朗. 融合多颜色空间信息的彩色图像色差校正算法. 计算机科学. 2020(S1): 157-160+165 .  百度学术
百度学术
21. 郝良耀,王冬青,许崇立. 基于最小二乘多项式的焊缝图像拟合研究. 电子设计工程. 2020(23): 173-176+182 .  百度学术
百度学术
22. 李东旭,胡鑫,王宇,李壮,陈珂. 基于点激光检测的筒管焊缝磨削方法. 制造技术与机床. 2020(12): 87-91 .  百度学术
百度学术
其他类型引用(36)



 下载:
下载:

 陕公网安备 61011302001501号
陕公网安备 61011302001501号 