Research on multi-aperture receiving characteristics of optical transmission based on composite phase plate
-
摘要:
多孔径接收技术可以有效抑制无线激光通信中的光强闪烁，然而接收孔径的直径、间距以及个数对于改善无线激光通信性能的作用规律目前还尚不清晰完备。因此，提出一种基于复合相位屏的光传输方法，并对多孔径接收特性进行了研究。首先，将功率谱反演法和zernike倾斜方法相结合以生成复合相位屏，并构建了光传输多孔径接收的数学模型；然后，改变接收孔径的直径、间距以及个数，统计接收端光强闪烁的变化情况；最后，在不同的大气折射率结构常数、光波波长、传输距离的实际光传输场景下，分析了多孔径接收光强闪烁的变化规律。结果表明，当大气折射率结构常数从
2.2313 ×10−16 m−2/3减小至1.5812 ×10−17 m−2/3，5个孔径直径为0.2 m，孔径间距为0.4 m的接收孔径的光强闪烁从0.2032 减小到0.0524 ；当光波波长由532 nm变为1550 nm，5个孔径直径为0.14 m，孔径间距为0.4 m的接收孔径的光强闪烁从0.2696 减小到0.1982 ；当传播距离从7×103 m增大到7×105 m，5个孔径直径为0.2 m，孔径间距为0.4 m的接收孔径的光强闪烁从0.0043 增大到0.3239 ，该项研究总结了无线激光通信的多孔径接收特性的规律。Abstract:Multi-aperture receiving technology can effectively suppress intensity scintillation in wireless laser communication. However, the effect of the diameter, spacing and number of receiving aperture on improving the performance of wireless laser communication is not clear and complete. Therefore, an optical transmission method based on composite phase screen was proposed, and the characteristics of multi-aperture reception were studied. Firstly, the power spectrum inversion method was combined with Zernike tilt method to generate a composite phase screen, and the mathematical model of optical transmission multi-aperture reception was constructed. Then, the diameter, spacing and number of receiving aperture were changed to calculate the change of intensity scintillation at the receiving end. Finally, the variation of multi-aperture received intensity scintillation was analyzed under the actual optical transmission scenario with different atmospheric refractive index structure constants, wavelengths and transmission distances. The results show that the intensity scintillation of five receiving apertures with diameters of 0.2 m and aperture spacing of 0.4 m decreases from
0.2032 to0.0524 when the structure constant of atmospheric refractive index decreases from2.2313 ×10−16 m−2/3 to1.5812 ×10−17 m−2/3. When the wavelength of light wave changes from 532 nm to1550 nm, the intensity scintillation of five receiving apertures with diameters of 0.2 m and apertures spacing of 0.4 m decreases from0.2165 to0.1523 . When the propagation distance increases from 7×103 m to 7×105 m, the intensity scintillation of five receiving apertures with diameters of 0.2 m and aperture spacing of 0.4 m increases from0.0043 to0.3239 . This study summarizes the law of multi-aperture receiving characteristics of wireless laser communication. -
引言
无线激光通信受大气湍流的影响，会引起光波波前相位畸变，产生光强闪烁现象。因此，大气湍流是限制激光通信性能的主要原因之一[1-2]，如何减少大气湍流对无线激光通信的影响已成为当今的研究重点。为了减少光强闪烁，提高通信的可靠性，研究者已经提出很多方法，如空间分集技术、自适应技术、信道编码、信道调制和大孔径接收等[3-8]，其中多孔径接收技术作为空间分集的一种，可以有效缓解大气湍流造成的影响，提高传输性能。
1996年，IBRAHIM M M等人[9]第一次提出了多孔径接收系统，能够有效地改善空间光通信系统的通信性能，并分析了对数正态分布大气信道条件下的多孔径接收系统的通信性能。TSIFTSIS T A等人[10]基于K分布的大气湍流模型，分析了多发多收、单发多收、单发单收和多发单收的无线通信系统的误码率。PRABU K等人[11]基于副载波调制，研究了当存在对准误差时，多孔径接收系统的通信性能。ANDROUTSOS N A[12]等人在Gamma-Gamma模型下基于瑞利分布指向误差，分析了多孔径接收在不同湍流强度和指向误差强度条件下的通信性能。劳陈哲[13]等人基于相干合成光束的多孔径接收技术，研究了星地激光通信的误码率随多孔径接收对湍流效应的补偿效果的变化情况。柯熙政等人[14]基于Gamma-Gamma信道模型，分析了不同湍流强度下多孔径接收系统的误码率特性。窦冰冰等人[15]对Mixure-Gamma分布下星地下行相位补偿多孔径接收系统性能进行了研究。目前，对于多孔径接收技术的理论研究已经蓬勃发展，但现有研究未对接收孔径的直径、间距以及个数与通信性能之间的作用规律进行清晰描述，因此，针对大气折射率结构常数、光波波长、传输距离对多孔径接收的影响研究亟需完备。
本文提出一种基于复合相位屏的光传输方法，并对多参数变化的多孔径接收特性进行了研究。提出了光传输多孔径接收数学物理模型，基于功率谱反演法加zernike倾斜共同作用产生成复合相位屏，改变接收孔径的直径、间距及个数，统计接收端光强闪烁的变化情况。分析了在不同大气折射率结构常数、不同光波波长、不同传输距离条件下的多孔径接收光强闪烁的变化规律。分析了基于多孔径接收的光传输过程中大气湍流等外界干扰因素对光传输通信性能的影响，为后续在不同湍流条件、传输距离及光波波长的条件下，如何选择多孔径接收的接收孔径直径、间距及个数最优的配比，从而抑制传输过程中大气湍流等带来的光束畸变，并有效提高通信性能提供了先验条件。
1 基本原理
1.1 相位屏生成
由于大气折射率受高度与风速的影响较大，故采用H-V模型[16]来计算不同高度下激光通信的不同大气折射率$ C_n^2(h) $的值。
$$ \begin{split} C_n^2(h) =& 5.94 \times {10^{{ { - }}53}}{\left( {\frac{{\bar v}}{{27}}} \right)^2}{h^{10}}\exp \left( {\frac{{ - h}}{{1\;000}}} \right) +\\ & 2.7 \times {10^{ - 16}}\exp \left( {\frac{{ - h}}{{1\;500}}} \right) + 1.9 \times {10^{ - 15}}\exp \left( {\frac{{ - h}}{{1\;000}}} \right) \end{split} $$ (1) 式中：$\bar v$为风速；h为高度。
相位屏采用模糊加倾斜两部分生成。从NOLL R J的研究结果[17]可知，zernike第2阶和第3阶泽尼克系数负责倾斜，其他高阶泽尼克系数负责模糊。为了使zernike相位屏有充分的高频分量，需要zernike有足够高的阶数，而随着zernike阶数的增加，运行速度会越来越慢，这给仿真模拟带来了巨大的不便，故模糊部分采用功率谱反演法。图1为功率谱反演法加zernike倾斜的方法生成的复合相位屏。
将倾斜和模糊分开进行相位屏仿真，则相位屏的结构函数等于负责生成倾斜部分zernike的2阶、3阶结构函数，加上负责模糊部分的功率谱反演法生成的结构函数。
基于Kolmogorov谱，由功率谱反演法生成相位屏的结构函数[18]和CHANAN G A[19]提出对于zernike的2阶、3阶倾斜部分的结构函数，可得复合相位屏的结构函数为
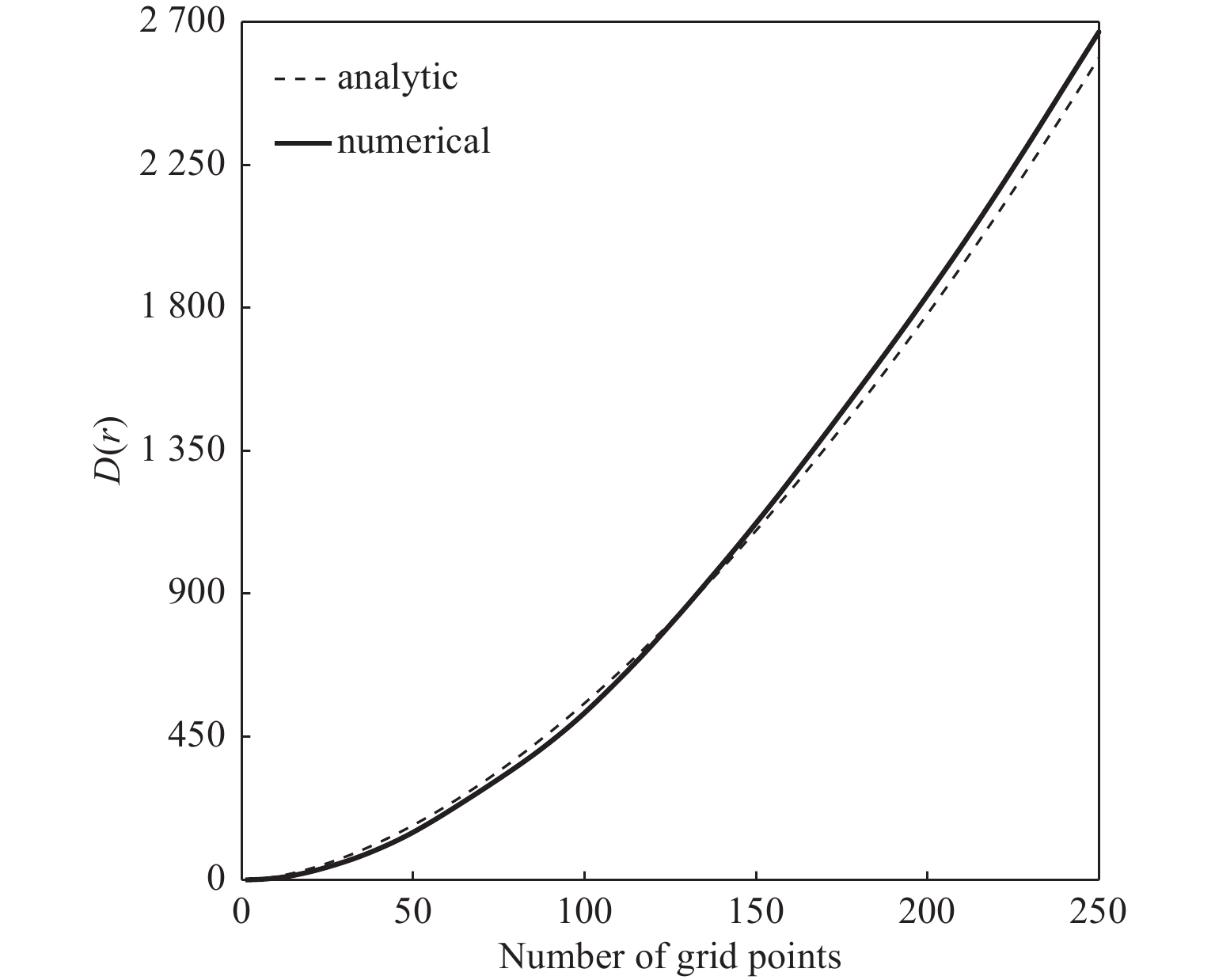
$$ \begin{split} D(r) = & 2{\left[ {\frac{{24}}{5}\Gamma \left(\frac{6}{5}\right)} \right]^{5/6}}{\left( {\frac{r}{{{r_0}}}} \right)^{5/3}} + 7.775\;4{\left( {\frac{D}{{{r_0}}}} \right)^{\tfrac{5}{3}}}\cdot\\ & \left[ {{I_{022(s)}} - \cos 2{\psi _0}{I_{222}}(s)} \right] + \\ & 7.775\;4{\left( {\frac{D}{{{r_0}}}} \right)^{\tfrac{5}{3}}}\left[ {{I_{022(s)}} + \cos 2{\psi _0}{I_{222}}(s)} \right] \end{split} $$ (2) 将推导出的相位屏结构函数与实际生成的相位屏结构函数进行对比，如图2所示。数值曲线与解析曲线的拟合优度为
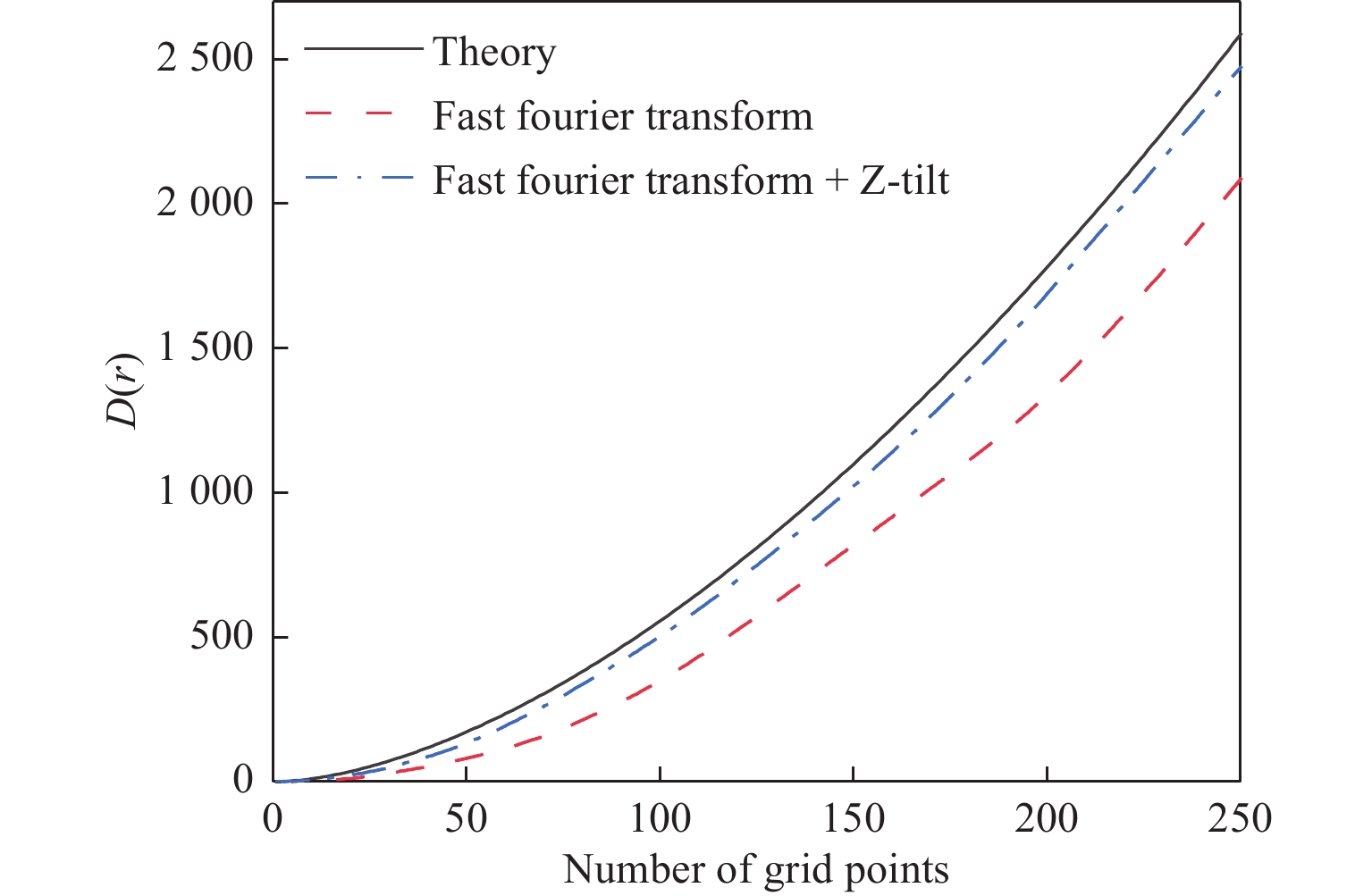
0.9975 ，拟合程度较好，由此证明了模拟相位屏的正确性。图3展示了基于快速傅里叶变换的功率谱反演法生成的相位屏结构函数，功率谱反演法加zernike倾斜相结合的复合相位屏结构函数以及大气湍流的理论结构函数的对比曲线。从图3中可以看出，复合相位屏与理论湍流更为接近。
![]()
图 2 复合相位屏结构函数数值与解析拟合曲线Figure 2. Numerical and analytic fitting curves of composite phase screen structure function![]()
图 3 大气湍流理论结构函数与功率谱反演法相位屏结构函数与复合相位屏结构函数对比Figure 3. Comparison between theoretical structure function of atmospheric turbulence, phase plate structure function of power spectrum inversion method and structure function of composite phase plate将高斯光源通过模拟生成的复合相位屏，并根据接收光斑的光强闪烁变换，研究接收孔径的间距、直径与个数对激光传输性能的影响。采用分步传播法，建立11个相位屏区间，相位屏间距为7×104 m，共传输7×105 m，得到的接收光斑如图4所示，其中高度h为800 m，风速为32 m/s。
1.2 光传输多孔径接收技术
光传输多孔径接收技术通过改变多孔径接收的接收孔径大小、孔径数目及孔径间距，得到不同平滑因子的值，从而降低光强闪烁，提高通信质量。
多孔径接收的光强闪烁$ \sigma _I^2(D) $在弱湍流下可根据对数起伏方差$ \sigma _{{\chi _A}}^2 $的关系计算得到[20]：
$$ \sigma _I^2(D) = \exp (4\sigma _{{\chi _A}}^2) - 1 $$ (3) 根据SASIELA R J等人的研究[21-23]，带有孔径平滑效应接收孔径直径D的球面波在弱湍流下的光强闪烁理论为
$$ \begin{split} \sigma _{{\chi _A}}^2 =& 0.132{\pi ^2}{\kappa ^2}L{D^{\tfrac{5}{3}}}\int_0^1 {{\mathrm{d}}uC_n^2(uL)\int_0^\infty {{k^{ - \tfrac{8}{3}}}} } \times\\ & {\sin ^2}\left( {\frac{{{k^2}\gamma u}}{{4\pi {F_N}}}} \right) {\left[ {\frac{{2{{\mathrm{J}}_1}(\gamma k/2)}}{{\gamma k/2}}} \right]^2}{\mathrm{d}}k \end{split} $$ (4) 式中：k为空间波数；L为总的传播距离；$u = {\textit{z}}/L$，z是作为积分的传输距离；${F_N} = {D^2}/\lambda L$，${F_N}$反映了孔径平滑效应对光强闪烁强弱的影响；$\gamma = 1 - {\textit{z}}/L$；$\kappa = 2\pi /\lambda $，$\lambda $为波长。
在中强湍流下，带有孔径平滑效应接收孔径直径D的球面波的光强闪烁理论公式为
$$ \begin{split} \sigma _I^2({F_N}) = & \exp \left[ {\frac{{0.2\sigma _R^2}}{{1 + 0.283{F_N} + 0.261\sigma _R^{12/5}}}} \right. + \\ & \left. {\frac{{0.2\sigma _R^2{{\left( {1 + 0.322\sigma _R^{12/5}} \right)}^{ - 5/6}}}}{{1 + 1.414{F_N} + 0.454{F_N}\sigma _R^{12/5}}}} \right] - 1 \end{split} $$ (5) 式中$ \sigma _R^2 = 0.132{k^{7/6}}C_n^2(h){L^{11/6}} $。
式(4)和式(5)中光强闪烁包含了能够反映孔径平滑效应的接收孔径直径D，将多孔径接收的等效孔径${D_{{\mathrm{th}}}}$带入式(4)或式(5)可得出多孔径接收的光强闪烁。等效孔径${D_{{\mathrm{th}}}}$通过多孔径接收的平滑因子与单孔径接收的平滑因子求出：
$$ {D_{{\mathrm{th}}}} = {\left( {\frac{{{{\left( {16{C_\Phi }(|{\boldsymbol{\rho}} + {\boldsymbol{x}}|)} \right)}^{1/2}}}}{{{A_N}\pi }}} \right)^{1/2}} $$ (6) 式中：$ {A_N} $是多孔径接收的孔径平滑因子[14]；${C_\Phi }(|.|)$函数为
$$ {C_\Phi }\left( {\left| {{\boldsymbol{\rho}} + {\boldsymbol{x}}} \right|} \right) = \int_0^D {{b_I}} ({\boldsymbol{x}} + {\boldsymbol{\rho}} )K({\boldsymbol{x}}){\mathrm{d}}{\boldsymbol{x}} $$ (7) 式中K(x)为接收孔径的调制传递函数。对于球面波传播，可由Kolmogorov谱得出表达式[24]：
$$ \begin{split} {b_I}(\rho ) = & 2.864\sum\limits_{n = 0}^\infty {\frac{{{{( - 1)}^n}{{(11/6)}_n}}}{{{{(1)}_n}n!}}} {\left( {\frac{{k{\rho ^2}}}{{4L}}} \right)^n}\cos \left[ {\left( {n + 5/6} \right)\frac{\pi }{2}} \right] -\\ &6.984{\left( {\frac{{k{\rho ^2}}}{{4L}}} \right)^{\tfrac{5}{6}}} - 5.541{\left( {\frac{{k{\rho ^2}}}{{4L}}} \right)^{\tfrac{{11}}{6}}}\sum\limits_{n = 1}^\infty {\frac{{{{( - 1)}^n}{{(11/3)}_n}}}{{{{(17/6)}_n}{{(17/6)}_n}}}} \times\\ & {\left( {\frac{{k{\rho ^2}}}{{4L}}} \right)^n}\sin (n\pi /2)\\[-103pt] \end{split} $$ (8) 2 仿真与实验结果分析
2.1 多孔径接收光强闪烁曲线
分别改变多孔径接收的接收孔径直径、接收孔径间距及接收孔径个数，得到在不同直径、间距、个数的接收孔径配比下的光强闪烁值，图5和图6是由表1所示的仿真参数得出。
![]()
图 5 不同接收孔径直径对应光强闪烁的关系Figure 5. Relationship between different receiving aperture diameters corresponding to intensity scintillation![]()
图 6 不同接收孔径间距对应光强闪烁的关系Figure 6. Relationship between different receiving aperture spacing corresponding to intensity scintillation表 1 数值仿真参数Table 1. Numerical simulation parametersParameter Value Wavelength $ \lambda $/ nm 1000 Propagation length L / m 8×104 Height h / km 0.8 Wind speed v / m.s−1 32 Inner scale l0 / m 0.001 Outer scale L0 / m inf Number of intervals in split-step
beam propagation method nscr11 Interval length in split-step beam
propagation method z / m8×103 图5为仿真接收孔径间距d为0.4 m，孔径直径D从0.02 m增大到0.5 m，不同接收孔径个数的光强闪烁变换曲线图。如图5所示，当接收孔径个数n=1时，接收孔径直径D=0.02 m的光强闪烁$ \sigma _I^2(D)= {0.795\;5} $，大于接收孔径直径D=0.08的光强闪烁$ \sigma _I^2(D)= {0.663\;8} $，…，大于接收孔径直径D=0.5的光强闪烁$ \sigma _I^2(D)= {0.225\;4} $，该结果说明随着接收孔径直径D的增大，光强闪烁越来越弱，增大接收孔径直径可以减小光强闪烁。当接收孔径直径D=0.02 m时，接收孔径个数n=1的光强闪烁$ \sigma _I^2(D)= {0.795\;5} $，大于接收孔径个数n=2的光强闪烁$ \sigma _I^2(D){ { = }} {0.611\;5} $，大于接收孔径个数n=3的光强闪烁$ \sigma _I^2(D)= {{\text{0}}{\text{.565 9}}} $，大于接收孔径个数n=4的光强闪烁$ \sigma _I^2(D)= {{\text{0}}{\text{.503 3}}} $，大于接收孔径个数n=5的光强闪烁$ \sigma _I^2(D){ { = 0}}{\text{.419 }}0 $，因此可以得出，随着接收孔径个数n的增加，光强闪烁越来越弱，增加接收孔径个数可以减小光强闪烁。
图6为仿真接收孔径直径D为0.16 m，孔径间距d从0.01 m增大到0.3 m，不同接收孔径个数的光强闪烁变换曲线图。如图6所示，当接收孔径个数n=2时，孔径间距d=0.01 m的光强闪烁$ \sigma _I^2(D)= {0.518\;4} $，孔径间距d=0.07 m的光强闪烁$ \sigma _I^2(D)= {0.444\;7} $，孔径间距d=0.1 m的光强闪烁$ \sigma _I^2(D)= {0.438\;4} $，孔径间距d=0.13 m的光强闪烁$ \sigma _I^2(D)= {0.441\;9} $，可以看出，随着接收孔径间距d的增大，接收孔径个数n=2的光强闪烁先是越来越弱，接收孔径间距d在拐点0.1 m附近后光强闪烁越来越强，接收孔径个数n=3时光强闪烁变化的孔径间距d的拐点在0.16 m附近，接收孔径个数n=4时光强闪烁变化的孔径间距d的拐点在0.21 m附近，接收孔径个数n=5时光强闪烁变化的孔径间距d的拐点在0.26 m附近，可以得出，不同个数n的接收孔径有不同的最佳接收孔径间距d使得光强闪烁的值最小。
2.2 真实场景中不同传输条件下的传输性能分析
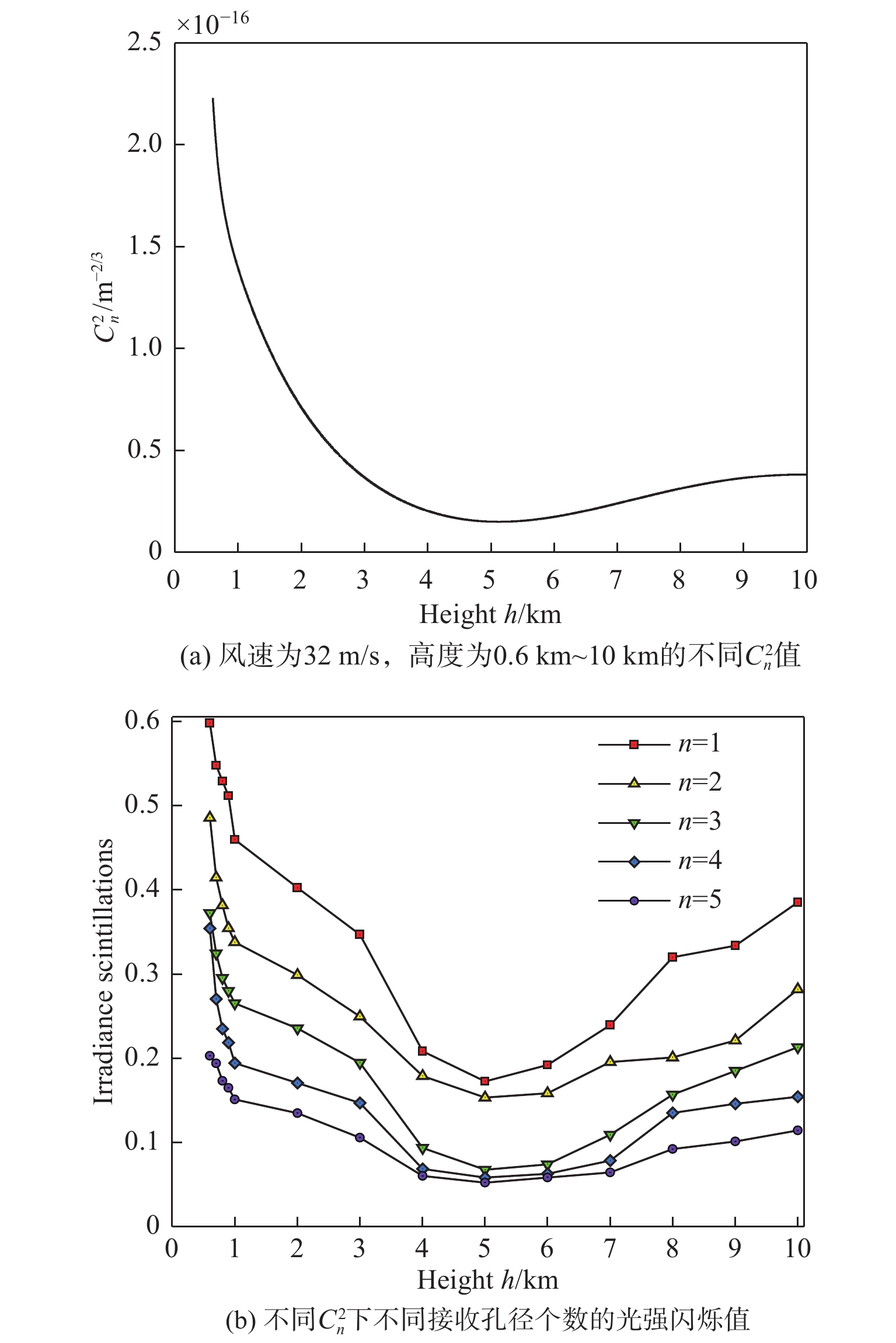
在真实场景中，其大气折射率结构常数、光波波长及传输距离都是重要的系统设计参数。因此，有必要分析在不同折射率结构常数、光波波长及传输距离的条件下，多孔径接收的变换规律。首先，分析不同大气折射率结构常数下的多孔径接收光强闪烁变化规律，在风速为32 m/s，高度h从0.6 km～10 km的不同$C_n^2$的条件下，且其他参数如表1所示的情况下，接收孔径间距d为0.4 m，接收孔径直径D为0.2 m，不同个数的接收孔径光强闪烁的变化曲线图，如图7所示。
![]()
图 7 不同高度的$C_n^2$下不同接收孔径个数的光强闪烁值Figure 7. Intensity scintillation values of different number of receiving apertures at different heights of $C_n^2$如图7所示，当高度h=1 km，$C_n^2{\text{ = 1}}{\text{.393 9}} \times {\text{1}}{{\text{0}}^{{ { - }}16}}\;{{\text{m}}^{ - 2/3}}$时，接收孔径个数n=1的光强闪烁$ \sigma _I^2(D) = 0{\text{.459 8}} $；当高度h=5 km，$C_n^2{\text{ = 1}}{\text{.512 2}} \times {\text{1}}{{\text{0}}^{{ { - }}17}}\;{{\text{m}}^{ - 2/3}}$时，接收孔径个数n=1的光强闪烁$ \sigma _I^2(D)= {0.172\;6} $；当高度h=6 km，$C_n^2{ { = }} 1{\text{.745 1}} \times {\text{1}}{{\text{0}}^{{ { - }}17}}\;{{\text{m}}^{ - 2/3}}$时，接收孔径个数n=1的光强闪烁$ \sigma _I^2(D)= {0.192\;1} $。可以得出，光强闪烁随着$C_n^2$的增大而增大，随着$C_n^2$的减小而减小。当高度h=2 km，$C_n^2{ { = 7}}{\text{.118}} \times {\text{1}}{{\text{0}}^{{ { - }}17}}\;{{\text{m}}^{ - 2/3}}$时，接收孔径个数n=1的光强闪烁$ \sigma _I^2(D)= {0.402\;5} $，大于接收孔径个数n=2的光强闪烁$ \sigma _I^2(D)= {0.299} $，大于接收孔径个数n=3的光强闪烁$ \sigma _I^2(D)= {0.235\;6} $，大于接收孔径个数n=4的光强闪烁$ \sigma _I^2(D)= {0.170\;9} $，大于接收孔径个数n=5的光强闪烁$ \sigma _I^2(D)= {0.134\;9} $，可以得出，在相同$C_n^2$下，随着接收孔径个数n的增加，光强闪烁越来越弱，因此增加接收孔径个数可以减小光强闪烁。
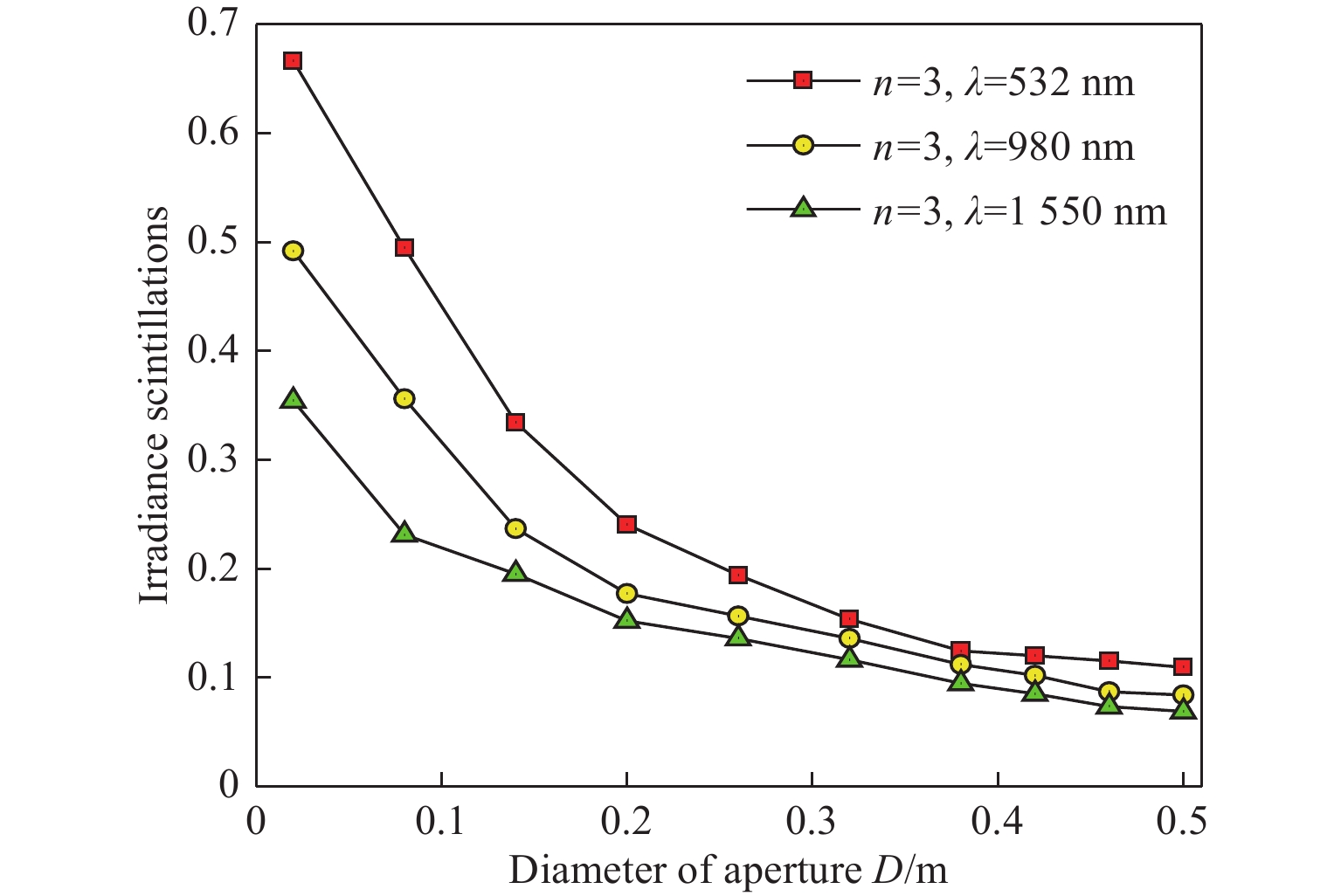
然后分析不同光波波长下的多孔径接收光强闪烁变化规律，在不同光波波长下，不同个数接收孔径的光强闪烁变换曲线图如图8、图9和图10所示。仿真接收孔径直径D从0.02 m增大到0.5 m，且孔径间距d为0.4 m，其他仿真参数如表2所示。
![]()
图 8 不同光波波长下接收孔径个数n=1对应光强闪烁的关系Figure 8. Relationship between number of receiving aperture n=1 and intensity scintillation under different light wavelengths如图8所示，当接收孔径个数n=1，接收孔径直径D=0.42 m时，光波波长$\lambda $ =532 nm的光强闪烁$\sigma _I^2(D){\rm{ = }}0.272\;9 $，大于光波波长$\lambda $=980 nm的光强闪烁$\sigma _I^2(D){\rm{ = 0}}{\rm{.244}} $，大于光波波长$\lambda $=1 550 nm的光强闪烁$\sigma _I^2(D){\rm{ = }}0.232\;9 $。如图9所示，当接收孔径个数n=3，接收孔径直径D=0.42 m时，光波波长$\lambda $=532 nm的光强闪烁$\sigma _I^2(D){\rm{ = 0}}{\rm{.120\;1}} $，大于光波波长$\lambda $=980 nm的光强闪烁$\sigma _I^2(D){\rm{ = }}0.102\;1 $，大于光波波长$\lambda $=1550 nm的光强闪烁$\sigma _I^2(D){\rm{ = }}0.085\;2 $。如图10所示，当接收孔径个数n=5，接收孔径直径D=0.42 m时，光波波长$\lambda $=532 nm的光强闪烁$\sigma _I^2(D){\rm{ = }}0.092\;8 $，大于光波波长$\lambda $=980 nm的光强闪烁$\sigma _I^2(D){\rm{ = }}0.081\;4 $，大于光波波长$\lambda $=1 550 nm的光强闪烁$\sigma _I^2(D){\rm{ = 0}}{\rm{.071\;4}} $。可以得出，随着光波波长的增大，多孔径接收的光强闪烁抑制能力越来越强。
![]()
图 9 不同光波波长下接收孔径个数n=3对应光强闪烁的关系Figure 9. Relationship between number of receiving aperture n=3 and intensity scintillation under different light wavelengths![]()
图 10 不同光波波长下接收孔径个数n=5对应光强闪烁的关系Figure 10. Relationship between number of receiving aperture n=5 and intensity scintillation under different light wavelengths表 2 改变波长数值仿真参数Table 2. Change wavelength numerical simulation parametersParameter Value Wavelength $ \lambda $/ nm 532/980/ 1550 Propagation length L/m 8×104 Height h/km 0.9 Wind speed v/(m·s−1) 32 Inner scale l0/ m 0.001 Outer scale L0/ m inf Number of intervals in split-step
beam propagation method nscr11 Interval length in split-step
beam propagation method z/m8×103 最后分析不同传输距离下的多孔径接收光强闪烁变化规律。在不同传输距离L下，不同个数接收孔径的光强闪烁变换曲线图如图11、图12和图13所示。仿真接收孔径直径D从0.02 m增大到0.5 m，孔径间距d为0.4 m，其他仿真参数如表3所示。
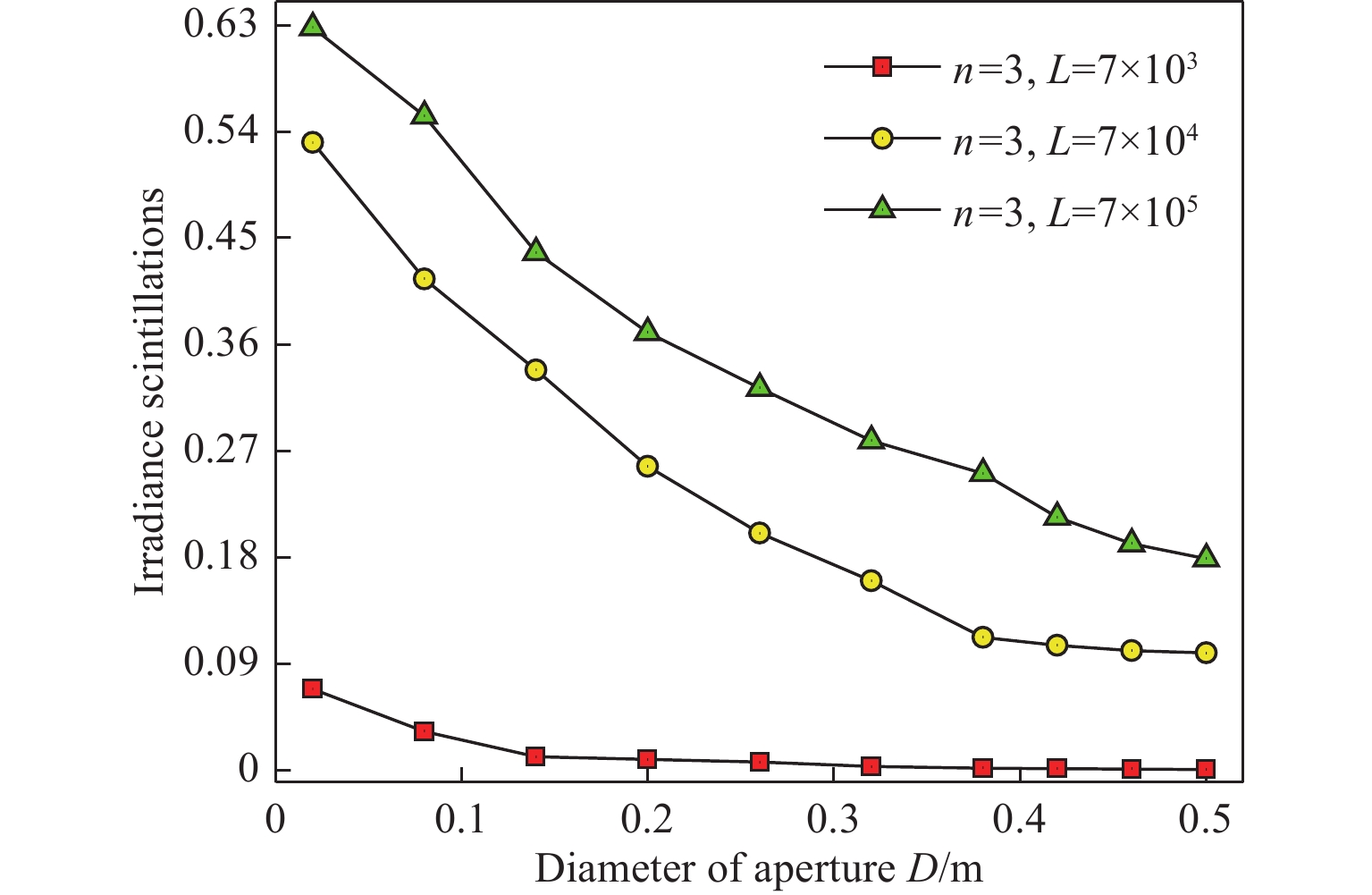
如图11所示，当接收孔径个数n=1，接收孔径直径D=0.2 m时，传输距离L=7×103 m的光强闪烁$ \sigma _I^2(D)= {0.012\;8} $，小于传输距离L=7×104 m的光强闪烁$ \sigma _I^2(D)= {0.326\;6} $，小于传输距离L=7×105 m的光强闪烁$ \sigma _I^2(D)= {0.453\;9} $。如图12所示，当接收孔径个数n=3，接收孔径直径D=0.2 m时，传输距离L=7×103 m的光强闪烁$ \sigma _I^2(D)= {0.009\;3} $，小于传输距离L=7×104 m的光强闪烁$ \sigma _I^2(D)= {0.257} $，小于传输距离L=7×105 m的光强闪烁$ \sigma _I^2(D)= {0.370\;4} $。如图13所示，当接收孔径个数n=5，接收孔径直径D=0.2 m时，传输距离L=7×103 m的光强闪烁$ \sigma _I^2(D)= {0.004\;3} $，小于传输距离L=7×104 m的光强闪烁$ \sigma _I^2(D)= {0.154\;2} $，小于传输距离L=7×105 m的光强闪烁$ \sigma _I^2(D)= {0.323\;9} $。可以得出，随着传输距离的增大，多孔径接收的光强闪烁越来越严重。
![]()
图 11 不同传输距离下接收孔径个数n=1对应光强闪烁的关系Figure 11. Relationship of receiving aperture number n=1 corresponding to intensity scintillation at different transmission distances因此，随着大气结构折射率常数的增加，多孔径接收光强闪烁越来越强；随着光波波长的增加，多孔径接收对光强闪烁的抑制作用越来越明显；且随着传播距离的增加，多孔径接收对光强闪烁的抑制作用越来越弱。
2.3 实验结果分析
实验验证采用液晶空间光调制器(LC-SLM)在室内模拟功率谱反演法加zernike倾斜共同作用产生的复合相位屏作为大气湍流，并用短波红外相机采集接收光斑，实验装置图如图14所示，主要实验参数如表4所示。
![]()
图 12 不同传输距离下接收孔径个数n=3对应光强闪烁的关系Figure 12. Relationship of receiving aperture number n=3 corresponding to intensity scintillation at different transmission distance![]()
图 13 不同传输距离下接收孔径个数n=5对应光强闪烁的关系Figure 13. Relationship of receiving aperture number n=5 corresponding to intensity scintillation at different transmission distances表 3 改变传输距离数值仿真参数Table 3. Change transmission distance numerical simulation parametersParameter Value Wavelength $ \lambda $/ nm 1000 Propagation length L / m 7×103/7×104/7×105 Height h / km 0.9 Wind speed v / (m·s−1) 32 Inner scale l0 / m 0.001 Outer scale L0 / m inf Number of intervals in split-step
beam propagation method nscr11 Interval length in split-step
beam propagation method z / m7×102/7×103/7×104 表 4 主要实验参数Table 4. Main experimental parametersEquipment name Parameters Laser Wavelength： 1550 nm
Power：10 dBm～15 dBmLiquid crystal spatial light modulation Operating wavelength： 1550 nm
Resolution：1920 ×1152
Velocity：845 HzShort-wave infrared camera Wave band：900 nm～ 1700 nm
Resolution：640×512
Frame rate：444 HZ在弱湍流、中强湍流和强湍流下的接收光斑如图15所示。
![]()
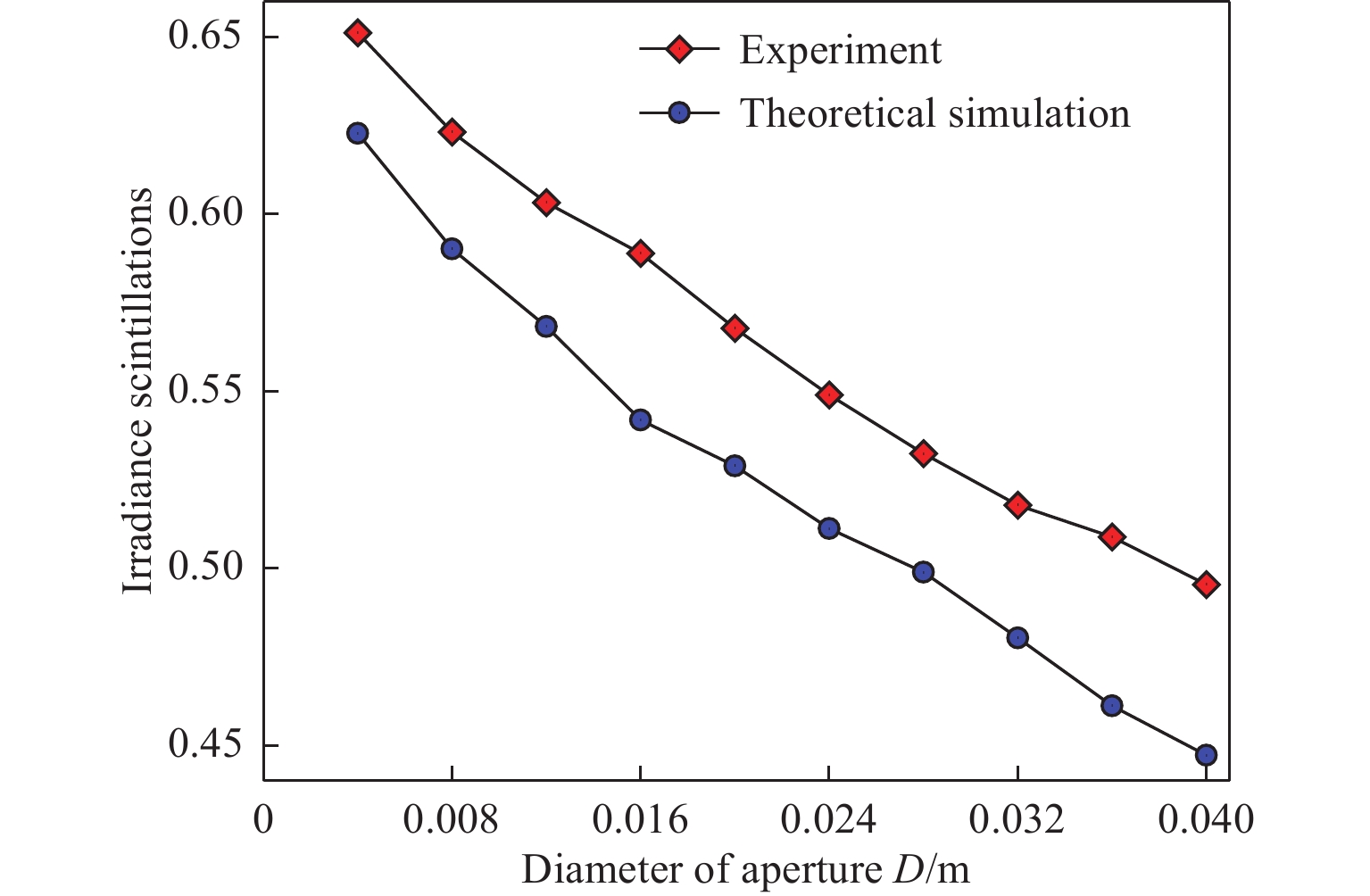
图 15 不同湍流强度下的接收光斑Figure 15. Receiving light spots under different turbulence intensities以4孔径接收为例，验证改变不同接收孔径直径与孔径间距对接收光斑光强闪烁的影响。图16所示为在$C_n^2{ { = 1}}{\text{.4}} \times {\text{1}}{{\text{0}}^{{ { - }}16}}\;{{\text{m}}^{ - 2/3}}$下，固定孔径间距，当接收孔径个数n=4时接收孔径在不同接收孔径直径下的光强闪烁变换曲线图。实验的接收孔径直径D从0.004 m增大到0.04 m，且孔径间距d为0.02 m的条件下，孔径个数n=4的光强闪烁变换曲线。
如图16所示，在实验中4个D=0.04 m的接收孔径的光强闪烁$ \sigma _I^2(D){\text{ = 0}}{\text{.495 4}} $，比1个D=0.04 m的接收孔径的光强闪烁$ \sigma _I^2(D){\text{ = 0}}{\text{.732 1}} $减少了32.332%。在理论仿真中4个D=0.04 m的接收孔径的光强闪烁$ \sigma _I^2(D){ { = 0}}{\text{.447 2}} $，比1个D=0.04 m的接收孔径的光强闪烁$ \sigma _I^2(D){ { = 0}}{\text{.667 1}} $减少了32.964%，实验结果与理论仿真结果近似。
![]()
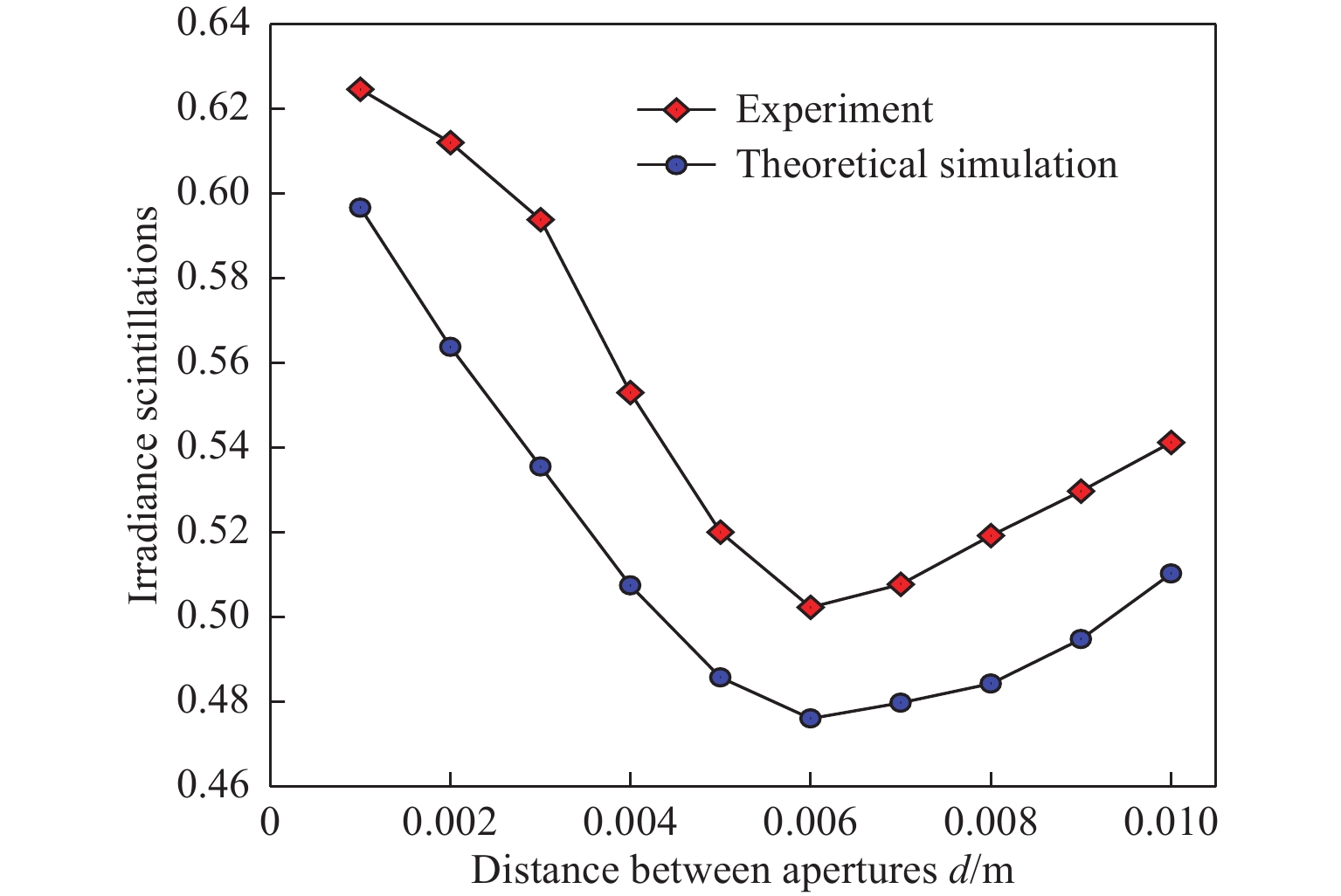
图 16 接收孔径个数n=4时不同接收孔径直径对应光强闪烁的关系Figure 16. Relationship of intensity scintillation corresponding to different receiving aperture diameters when number of receiving aperture n=4图17所示为在$C_n^2{\text{ = 1}}{\text{.4}} \times {\text{1}}{{\text{0}}^{{ { - }}16}}\;{{\text{m}}^{ - 2/3}}$下，固定孔径直径，当接收孔径个数n=4时接收孔径在不同接收孔径直间距下的光强闪烁变换曲线图。实验验证接收孔径间距d从0.001 m增大到0.01 m，且孔径直径D=0.02 m的条件下，孔径个数n=4的光强闪烁变换曲线。
如图17所示，在实验中孔径间距d=0.006 m处附近的光强闪烁$ \sigma _I^2(D){ { = 0}}{\text{.502 3}} $最小，且比1个D=0.02 m的接收孔径的光强闪烁$ \sigma _I^2(D){\text{ = 0}}{\text{.772 1}} $，减少了34.944%。在理论仿真中孔径间距d=0.006 m处附近的光强闪烁$ \sigma _I^2(D){\text{ = 0}}{\text{.476 1}} $最小，且比1个D=0.02 m的接收孔径的光强闪烁$ \sigma _I^2(D){\text{ = 0}}{\text{.715 6}} $，减少了35.579%，实验结果与理论结果近似。
![]()
图 17 接收孔径个数n=4时不同接收孔径间距对应光强闪烁的关系Figure 17. Relationship of intensity scintillation corresponding to different receiving aperture spacing when number of receiving aperture n=43 结论
提出了基于功率谱反演法与zernike倾斜共同作用产生的复合相位屏的方法，验证了该复合相位屏更加接近实际的大气湍流。分析了在该复合相位屏下的光传输多孔径接收特性，研究采用不同配比的接收孔径直径、孔径间距与孔径个数时光强闪烁强弱变化情况。结果表明，随着接收孔径个数的增加对光强闪烁的抑制作用越来越强，增加接收孔径直径可以减小光强闪烁，不同个数的接收孔径有自己的最佳接收孔径间距。多孔径接收光强闪烁与大气折射率结构常数和传播距离成正比，与光波波长成反比。该研究为基于多孔径接收的自由空间光接收系统的设计提供了理论基础和技术参考。
-
![]()
图 2 复合相位屏结构函数数值与解析拟合曲线
Figure 2. Numerical and analytic fitting curves of composite phase screen structure function
![]()
图 3 大气湍流理论结构函数与功率谱反演法相位屏结构函数与复合相位屏结构函数对比
Figure 3. Comparison between theoretical structure function of atmospheric turbulence, phase plate structure function of power spectrum inversion method and structure function of composite phase plate
![]()
图 5 不同接收孔径直径对应光强闪烁的关系
Figure 5. Relationship between different receiving aperture diameters corresponding to intensity scintillation
![]()
图 6 不同接收孔径间距对应光强闪烁的关系
Figure 6. Relationship between different receiving aperture spacing corresponding to intensity scintillation
![]()
图 7 不同高度的$C_n^2$下不同接收孔径个数的光强闪烁值
Figure 7. Intensity scintillation values of different number of receiving apertures at different heights of $C_n^2$
![]()
图 8 不同光波波长下接收孔径个数n=1对应光强闪烁的关系
Figure 8. Relationship between number of receiving aperture n=1 and intensity scintillation under different light wavelengths
![]()
图 9 不同光波波长下接收孔径个数n=3对应光强闪烁的关系
Figure 9. Relationship between number of receiving aperture n=3 and intensity scintillation under different light wavelengths
![]()
图 10 不同光波波长下接收孔径个数n=5对应光强闪烁的关系
Figure 10. Relationship between number of receiving aperture n=5 and intensity scintillation under different light wavelengths
![]()
图 11 不同传输距离下接收孔径个数n=1对应光强闪烁的关系
Figure 11. Relationship of receiving aperture number n=1 corresponding to intensity scintillation at different transmission distances
![]()
图 12 不同传输距离下接收孔径个数n=3对应光强闪烁的关系
Figure 12. Relationship of receiving aperture number n=3 corresponding to intensity scintillation at different transmission distance
![]()
图 13 不同传输距离下接收孔径个数n=5对应光强闪烁的关系
Figure 13. Relationship of receiving aperture number n=5 corresponding to intensity scintillation at different transmission distances
![]()
图 15 不同湍流强度下的接收光斑
Figure 15. Receiving light spots under different turbulence intensities
![]()
图 16 接收孔径个数n=4时不同接收孔径直径对应光强闪烁的关系
Figure 16. Relationship of intensity scintillation corresponding to different receiving aperture diameters when number of receiving aperture n=4
![]()
图 17 接收孔径个数n=4时不同接收孔径间距对应光强闪烁的关系
Figure 17. Relationship of intensity scintillation corresponding to different receiving aperture spacing when number of receiving aperture n=4
表 1 数值仿真参数
Table 1 Numerical simulation parameters
Parameter Value Wavelength $ \lambda $/ nm 1000 Propagation length L / m 8×104 Height h / km 0.8 Wind speed v / m.s−1 32 Inner scale l0 / m 0.001 Outer scale L0 / m inf Number of intervals in split-step
beam propagation method nscr11 Interval length in split-step beam
propagation method z / m8×103 
下载: 导出CSV
表 2 改变波长数值仿真参数
Table 2 Change wavelength numerical simulation parameters
Parameter Value Wavelength $ \lambda $/ nm 532/980/ 1550 Propagation length L/m 8×104 Height h/km 0.9 Wind speed v/(m·s−1) 32 Inner scale l0/ m 0.001 Outer scale L0/ m inf Number of intervals in split-step
beam propagation method nscr11 Interval length in split-step
beam propagation method z/m8×103
下载: 导出CSV
表 3 改变传输距离数值仿真参数
Table 3 Change transmission distance numerical simulation parameters
Parameter Value Wavelength $ \lambda $/ nm 1000 Propagation length L / m 7×103/7×104/7×105 Height h / km 0.9 Wind speed v / (m·s−1) 32 Inner scale l0 / m 0.001 Outer scale L0 / m inf Number of intervals in split-step
beam propagation method nscr11 Interval length in split-step
beam propagation method z / m7×102/7×103/7×104
下载: 导出CSV
表 4 主要实验参数
Table 4 Main experimental parameters
Equipment name Parameters Laser Wavelength： 1550 nm
Power：10 dBm～15 dBmLiquid crystal spatial light modulation Operating wavelength： 1550 nm
Resolution：1920 ×1152
Velocity：845 HzShort-wave infrared camera Wave band：900 nm～ 1700 nm
Resolution：640×512
Frame rate：444 HZ
下载: 导出CSV
-
[1] ANDREWS L C, PHILLIPS R L, YU P T. Optical scintillations and fade statistics for a satellite-communication system[J]. Applied Optics, 1995, 34(33): 7742-7751. doi: 10.1364/AO.34.007742
[2] FRIED D L, MEVERS G E, KEISTER M P. Measurements of laser-beam scintillation in the atmosphere[J]. Journal of the Optical Society of America, 1967, 57(6): 787. doi: 10.1364/JOSA.57.000787
[3] 尹涵, 宋英雄, 李迎春, 等. 基于MIMO模式分集相干接收的自由空间光通信大气湍流补偿技术研究[J]. 中国激光, 2022, 49(23): 2306002. doi: 10.3788/CJL202249.2306002 YIN Han, SONG Yingxiong, LI Yingchun, et al. Free-space optical communication atmospheric turbulence compensation based on multiple input multiple output mode diversity coherent reception[J]. Chinese Journal of Lasers, 2022, 49(23): 2306002. doi: 10.3788/CJL202249.2306002
[4] YAO H F, NI X L, CHEN C Y, et al. Performance of M-PAM FSO communication systems in atmospheric turbulence based on APD detector[J]. Optics Express, 2018, 26(18): 23819-23830. doi: 10.1364/OE.26.023819
[5] BELMONTE A, KAHN J M. Capacity of coherent free-space optical links using atmospheric compensation techniques[J]. Optics Express, 2009, 17(4): 2763-2773. doi: 10.1364/OE.17.002763
[6] YAO H F, NI X L, LIU Z, et al. Experimental demonstration of 4-PAM for high-speed indoor free-space OW communication based on cascade FIR-LMS adaptive equalizer[J]. Optics Communications, 2018, 426: 490-496. doi: 10.1016/j.optcom.2018.06.001
[7] YAO H F, NI X L, CHEN C Y, et al. Performance analysis of MPSK FSO communication based on the balanced detector in a fiber-coupling system[J]. IEEE Access, 2019, 7: 84197-84208. doi: 10.1109/ACCESS.2019.2924178
[8] GIRI R K, PATNAIK B. Error performance enhancement of satellite to earth FSO downlink employing hybrid subcarrier intensity modulation scheme with aperture averaging technique[J]. Optical and Quantum Electronics, 2019, 51(4): 111. doi: 10.1007/s11082-019-1817-9
[9] IBRAHIM M M, IBRAHIM A M. Performance analysis of optical receivers with space diversity reception[J]. IEE Proceedings - Communications, 1996, 143(6): 369. doi: 10.1049/ip-com:19960885
[10] TSIFTSIS T A, SANDALIDIS H G, KARAGIANNIDIS G K, et al. Optical wireless links with spatial diversity over strong atmospheric turbulence channels[J]. IEEE Transactions on Wireless Communications, 2009, 8(2): 951-957. doi: 10.1109/TWC.2009.071318
[11] PRABU K, KUMAR D S. BER analysis for BPSK based SIM–FSO communication system over strong atmospheric turbulence with spatial diversity and pointing errors[J]. Wireless Personal Communications, 2015, 81(3): 1143-1157. doi: 10.1007/s11277-014-2176-2
[12] ANDROUTSOS N A, NISTAZAKIS H E, CHATZIKONTIS E V, et al. Spatial jitter influence on the average BLER performance of SIMO FSO links over atmospheric turbulence channels[J]. Electronics, 2021, 10(16): 2033. doi: 10.3390/electronics10162033
[13] 劳陈哲, 孙建锋, 周煜, 等. 多孔径接收相干合束系统性能研究[J]. 中国激光, 2019, 46(7): 0705003. doi: 10.3788/CJL201946.0705003 LAO Chenzhe, SUN Jianfeng, ZHOU Yu, et al. Performance of coherent beam combining system with multiple aperture receiver[J]. Chinese Journal of Lasers, 2019, 46(7): 0705003. doi: 10.3788/CJL201946.0705003
[14] 柯熙政, 宋鹏, 裴国强. 无线激光通信中的多孔径接收技术研究[J]. 光学学报, 2011, 31(12): 1201003. doi: 10.3788/AOS201131.1201003 KE Xizheng, SONG Peng, PEI Guoqiang. Research on multi-aperture reception in wireless laser communication[J]. Acta Optica Sinica, 2011, 31(12): 1201003. doi: 10.3788/AOS201131.1201003
[15] 窦冰冰. Mixure-Gamma分布下星地下行相位补偿多孔径接收系统性能研究[D]. 哈尔滨: 哈尔滨工业大学, 2020. DOU Bingbing. Research on performance of satellite-to-ground downlink phase compensation multi-aperture receiving system under Mixure-Gamma distribution[D]. Harbin: Harbin Institute of Technology, 2020.
[16] 程知, 侯再红, 靖旭, 等. Hufnagel-Valley湍流强度廓线的高精度实时反演方法[J]. 红外与激光工程, 2013, 42(6): 1562-1567. doi: 10.3969/j.issn.1007-2276.2013.06.033 CHENG Zhi, HOU Zaihong, JING Xu, et al. High-precision and real-time inversion method of Hufnagel-Valley turbulence profile[J]. Infrared and Laser Engineering, 2013, 42(6): 1562-1567. doi: 10.3969/j.issn.1007-2276.2013.06.033
[17] NOLL R J. Zernike polynomials and atmospheric turbulence[J]. Journal of the Optical Society of America, 1976, 66(3): 207. doi: 10.1364/JOSA.66.000207
[18] 向劲松. 快速傅里叶变换湍流相位屏高频误差的补偿方法[J]. 光学学报, 2014, 34(10): 1001003. doi: 10.3788/AOS201434.1001003 XIANG Jinsong. High-frequency error compensation method for the fast Fourier transform-based turbulent phase screen[J]. Acta Optica Sinica, 2014, 34(10): 1001003. doi: 10.3788/AOS201434.1001003
[19] CHANAN G A. Calculation of wave-front tilt correlations associated with atmospheric turbulence[J]. Journal of the Optical Society of America A, 1992, 9(2): 298-301. doi: 10.1364/JOSAA.9.000298
[20] YU L K, SHEN H, JING X, et al. Study on the measurement of isoplanatic angle using stellar scintillation[J]. Acta Optica Sinica, 2014, 34(3): 0301001. doi: 10.3788/AOS201434.0301001
[21] SASIELA R J. Electromagnetic wave propagation in turbulence[M]. New York: SPIE, 2007: 193-203.
[22] YU L K, JING X, SHEN H, et al. Isoplanatic angle in finite distance: theory analysis on measurement feasibility[J]. Optics Letters, 2014, 39(4): 789-792. doi: 10.1364/OL.39.000789
[23] 沈红, 于龙昆, 周玉修, 等. 孔径平滑下平面波与球面波的光强闪烁[J]. 激光与光电子学进展, 2021, 58(23): 2301001. SHEN Hong, YU Longkun, ZHOU Yuxiu, et al. Light intensity scintillation of plane wave and spherical wave under aperture-averaging[J]. Laser & Optoelectronics Progress, 2021, 58(23): 2301001.
[24] ANDREWS L C. Aperture-averaging factor for optical scintillations of plane and spherical waves in the atmosphere[J]. Journal of the Optical Society of America A, 1992, 9(4): 597-600. doi: 10.1364/JOSAA.9.000597
-
期刊类型引用(4)
1. 戴羿隆，陈凯，吴光，贾梦辉，邓伦华，张三军. 基于时间数字转换器的高精度测速系统. 激光与光电子学进展. 2025(01): 185-192 . 
百度学术
2. 李敬，孙忠辉，倪晋平，田会. 采用双区截光幕测速装置标定靶距的方法. 光子学报. 2024(02): 146-155 . 百度学术
3. 赵悦，蔡荣立. 声光信号融合的外弹道参数测量方法研究. 光学与光电技术. 2024(02): 47-55 . 百度学术
4. 陈果，蔡荣立，李翰宗，许健男. 基于FPGA与STM32的光幕靶校验仪. 计算机测量与控制. 2024(05): 325-333 . 百度学术
其他类型引用(1)
计量
- 文章访问数: 89
- HTML全文浏览量: 15
- PDF下载量: 40
- 被引次数: 5
陕公网安备 61011302001501号 