FDM printing trajectory measurement and quality evaluation method based on infrared imaging
-
摘要:
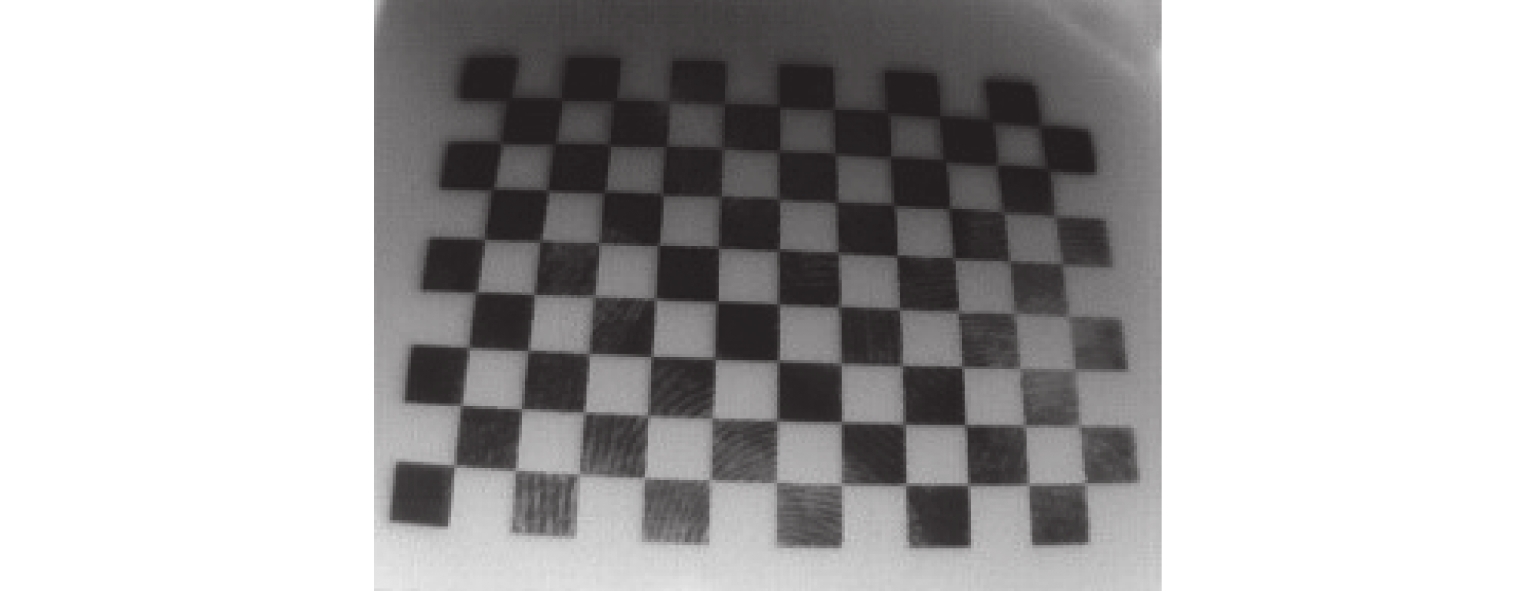
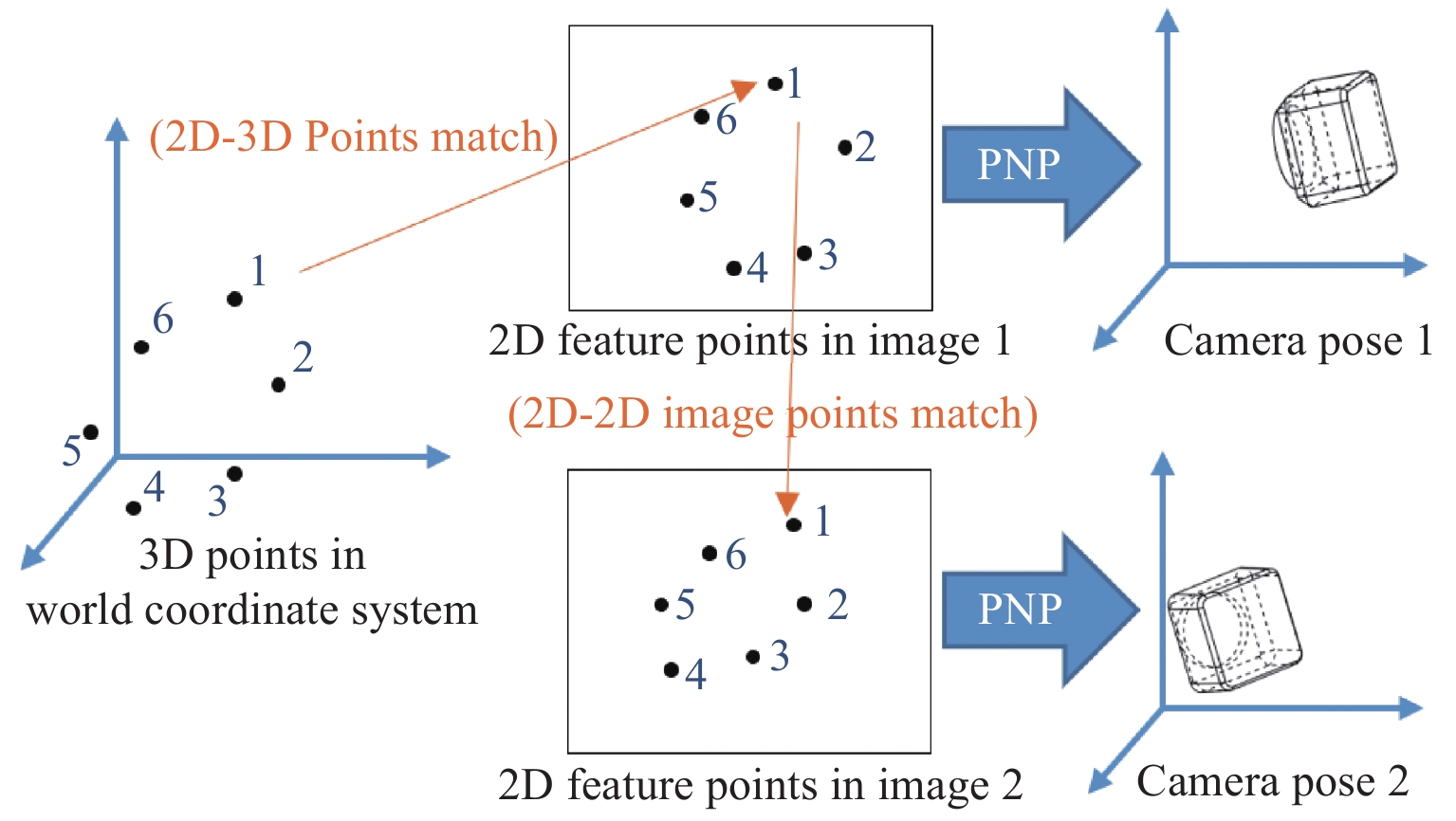
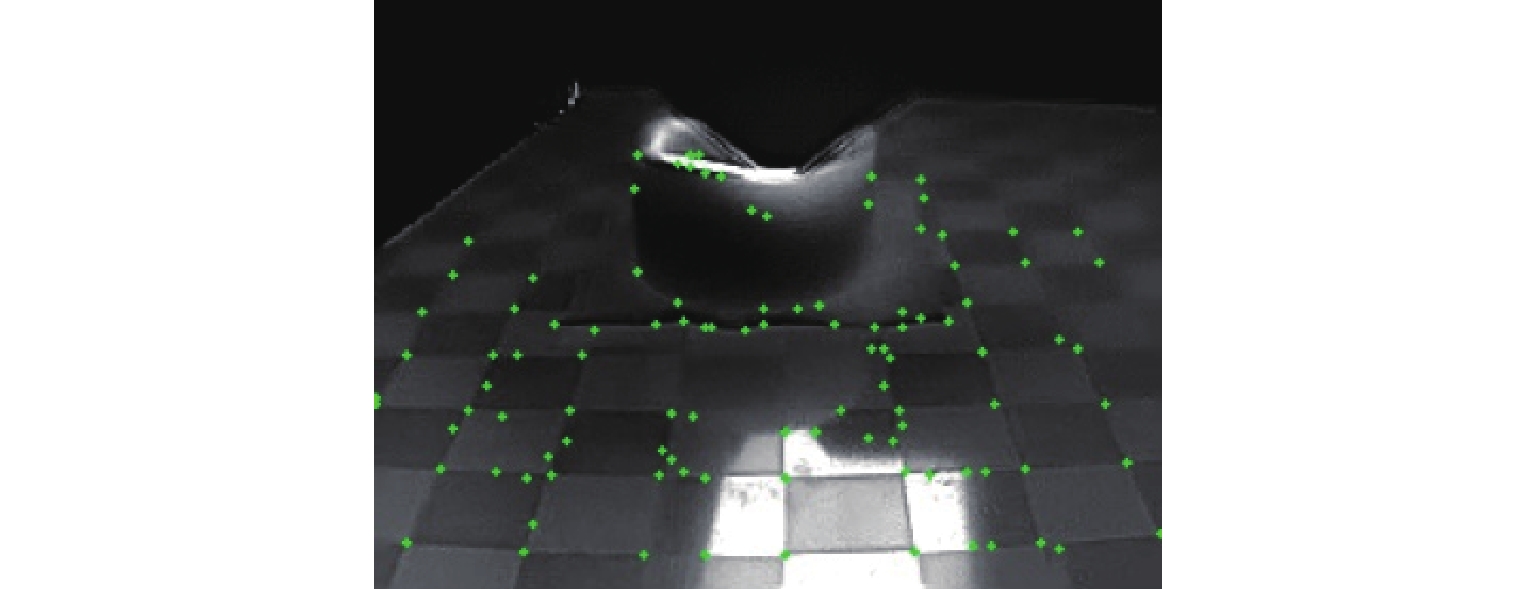
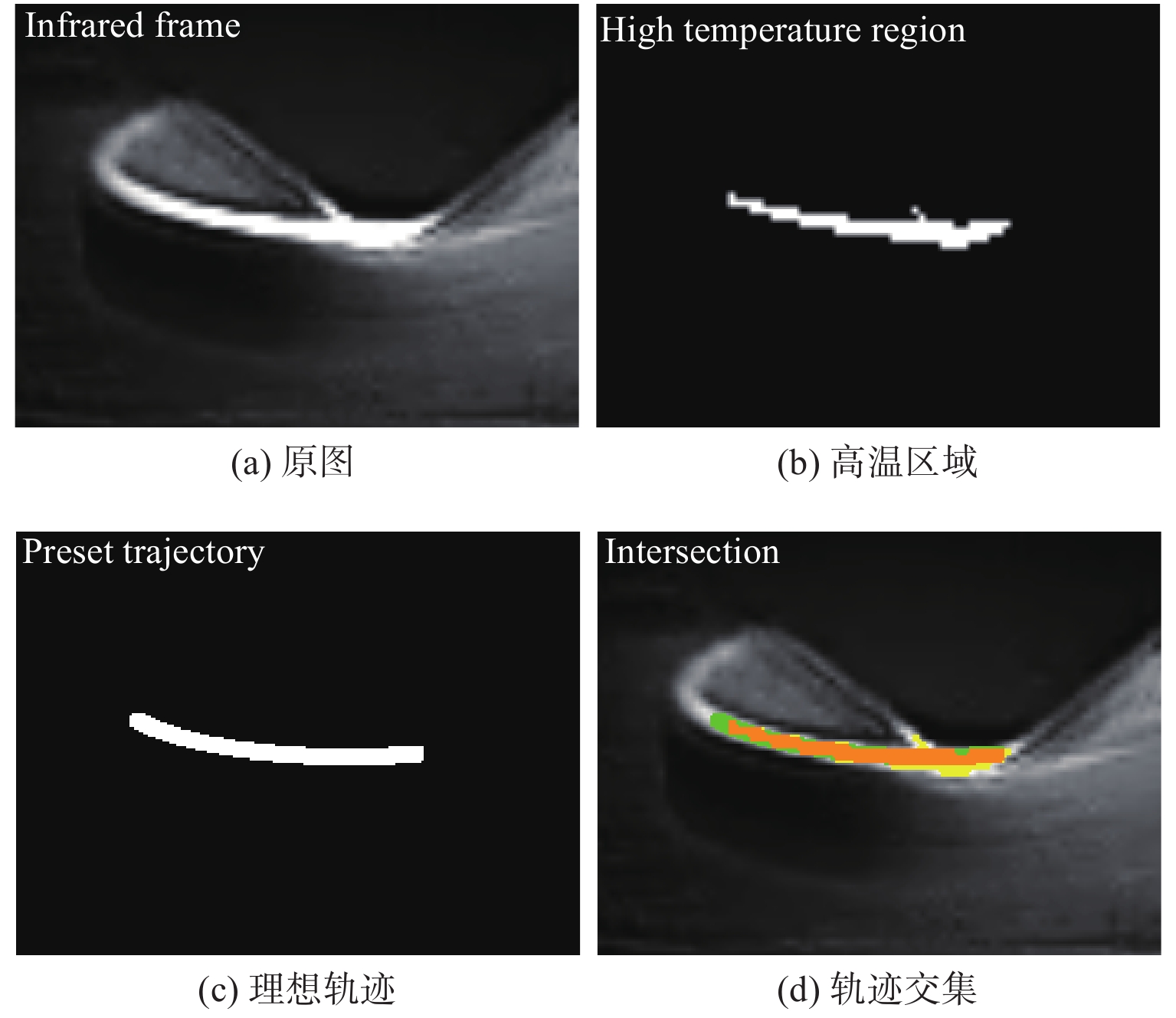
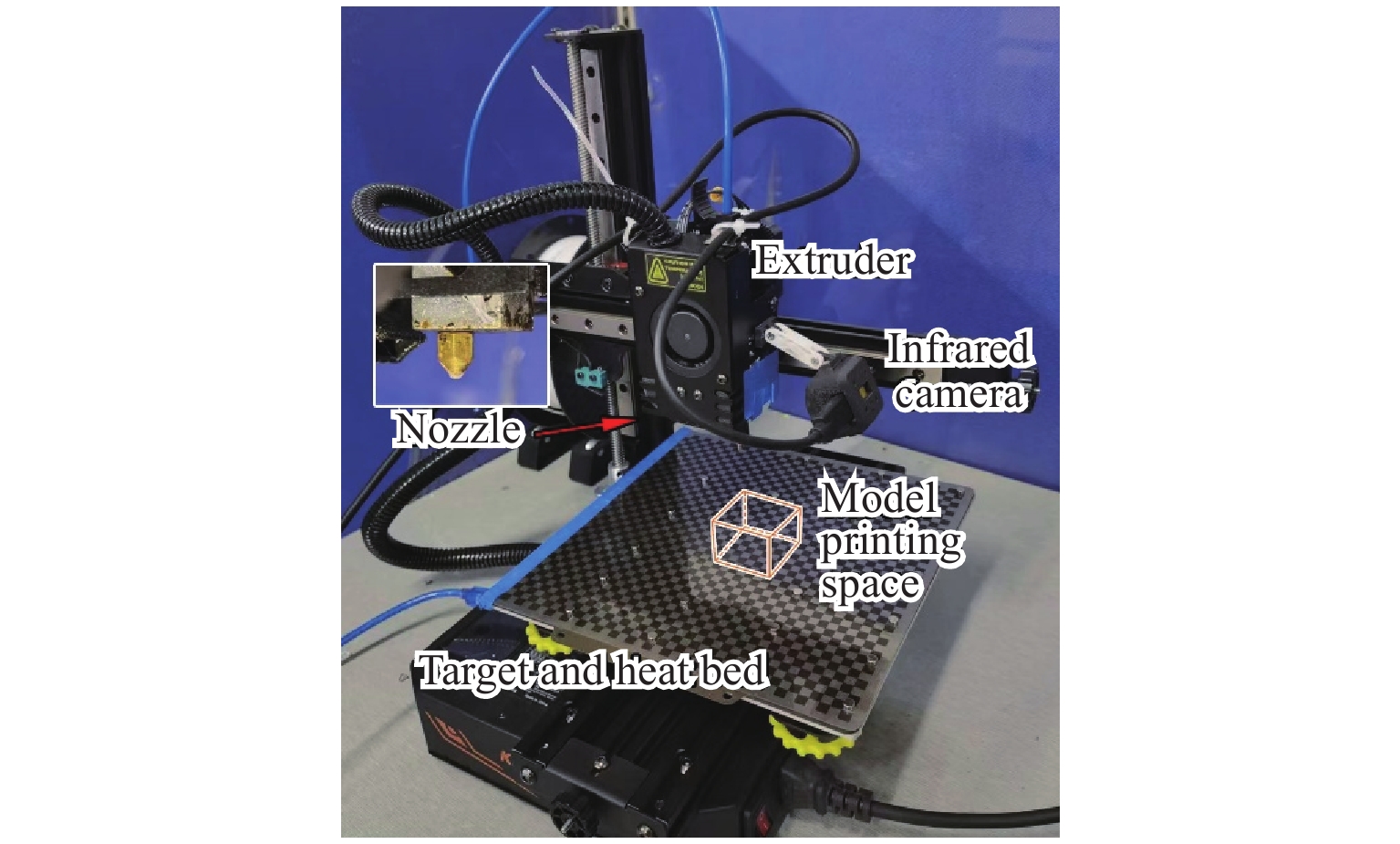
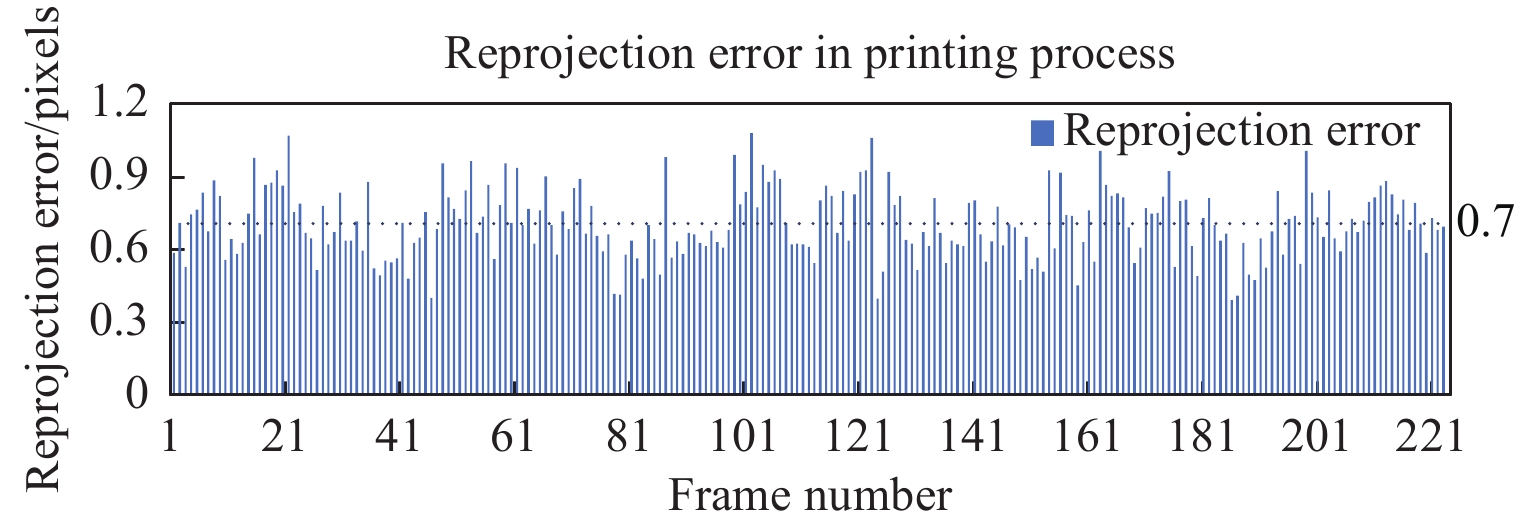
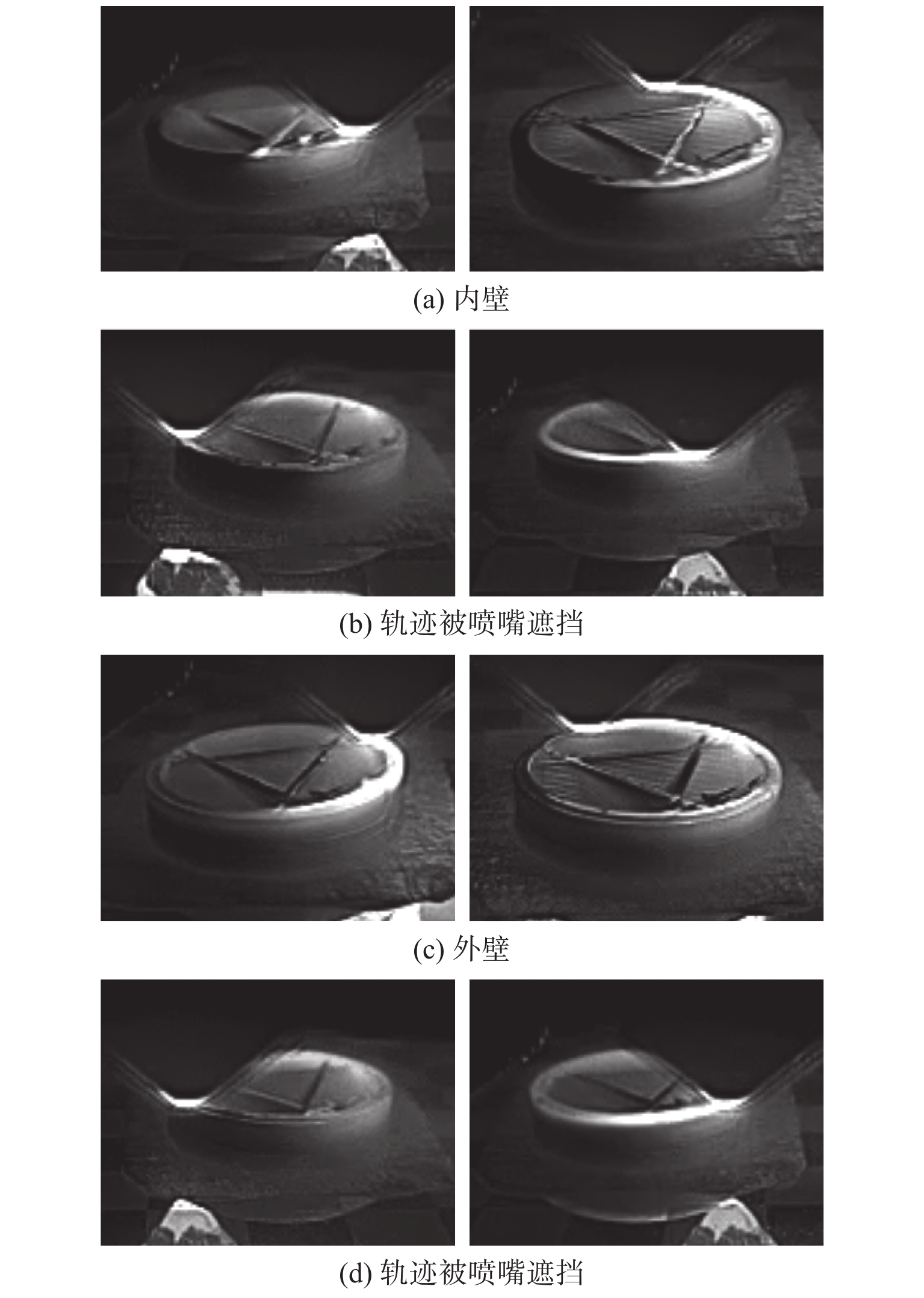
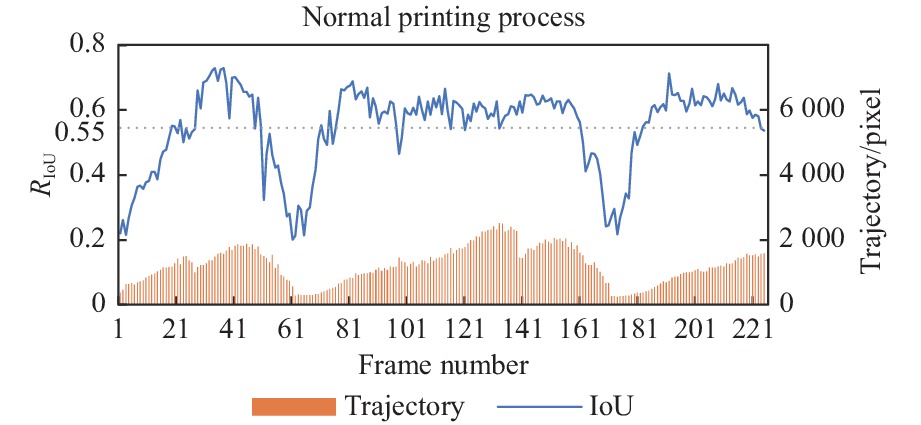
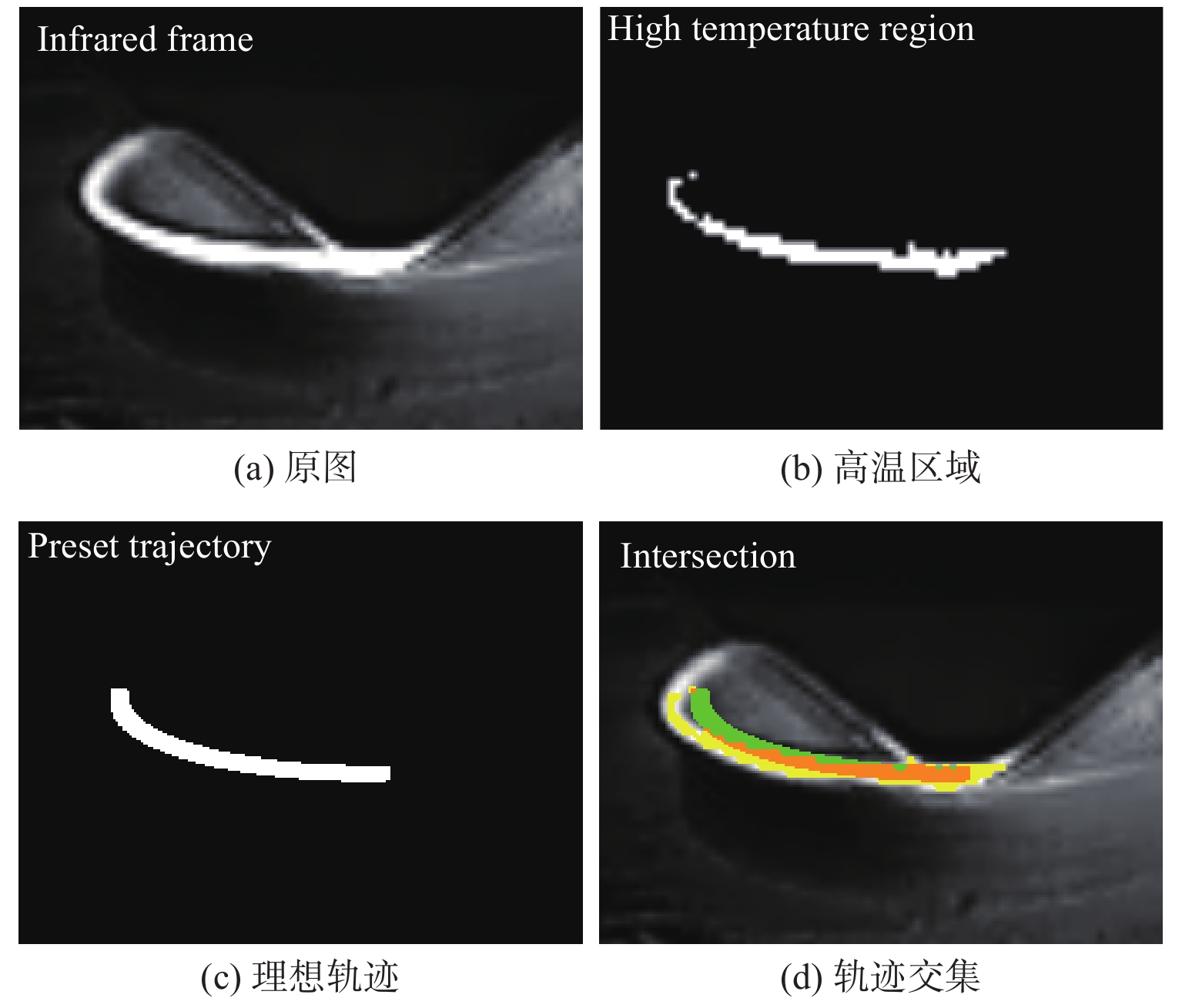
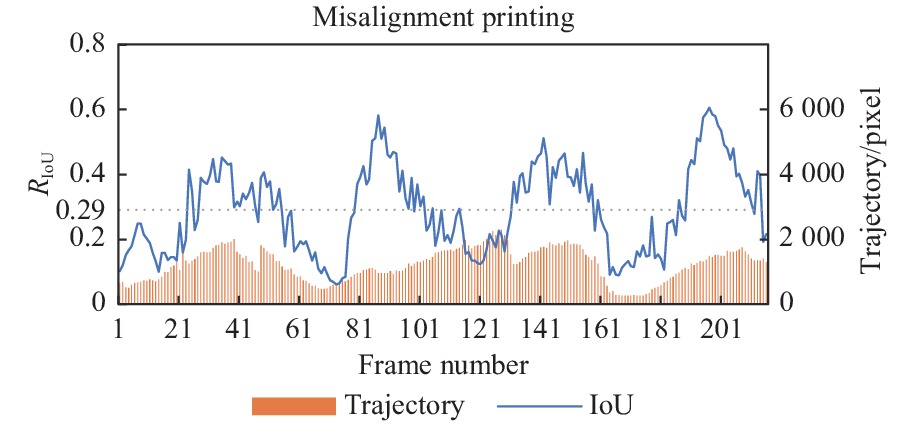
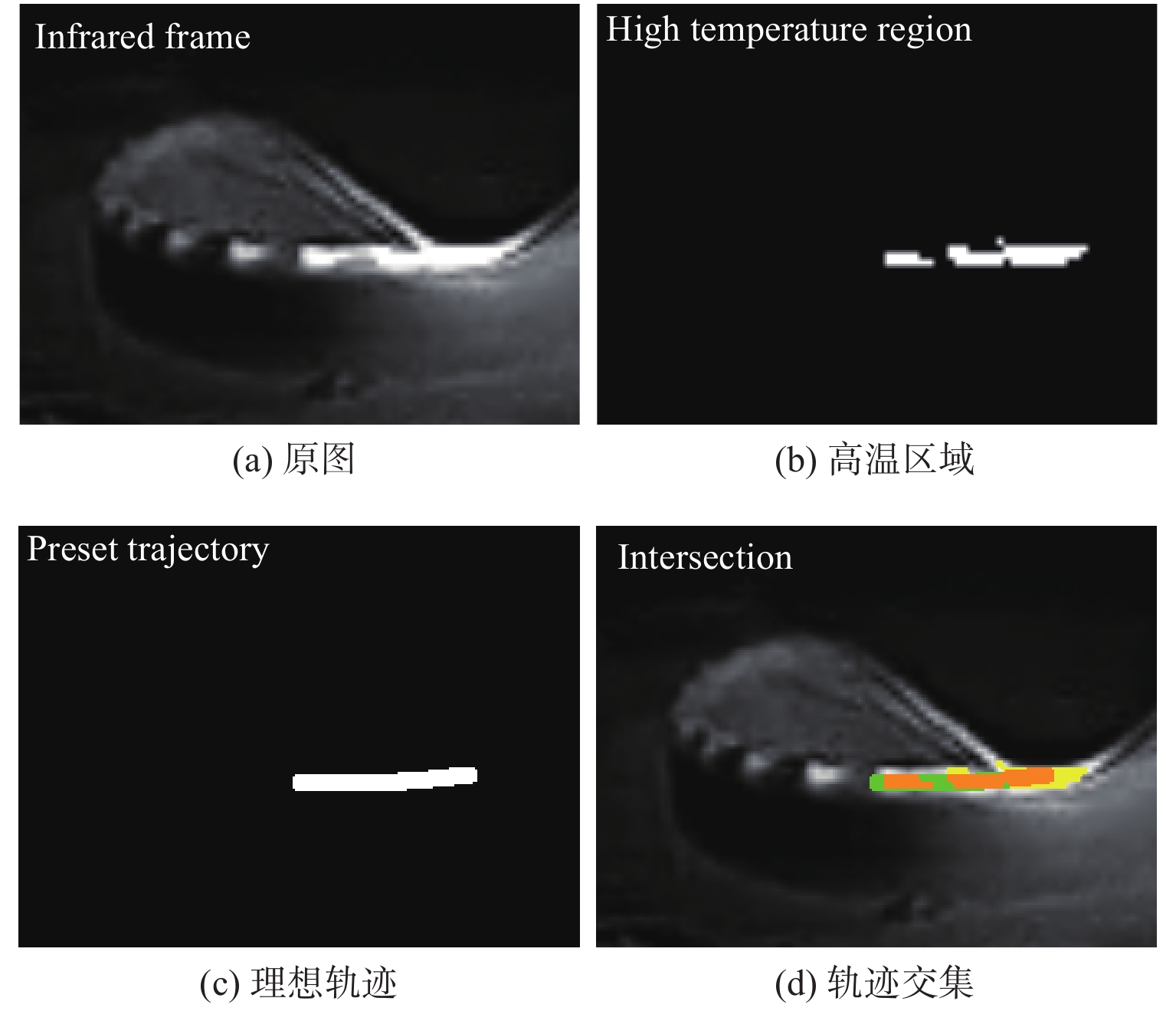
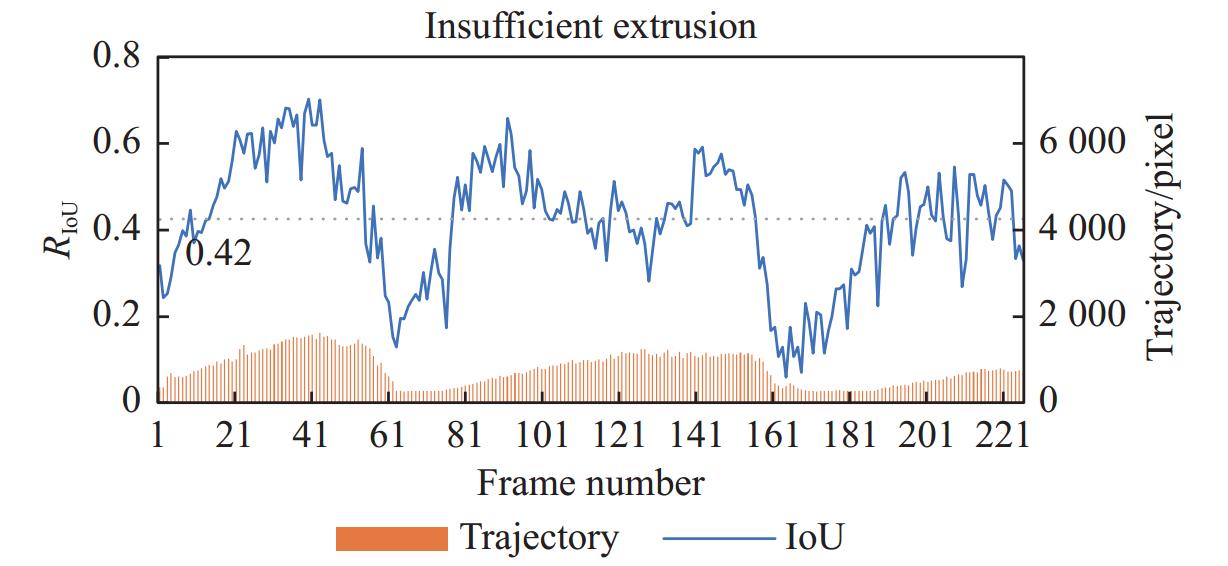
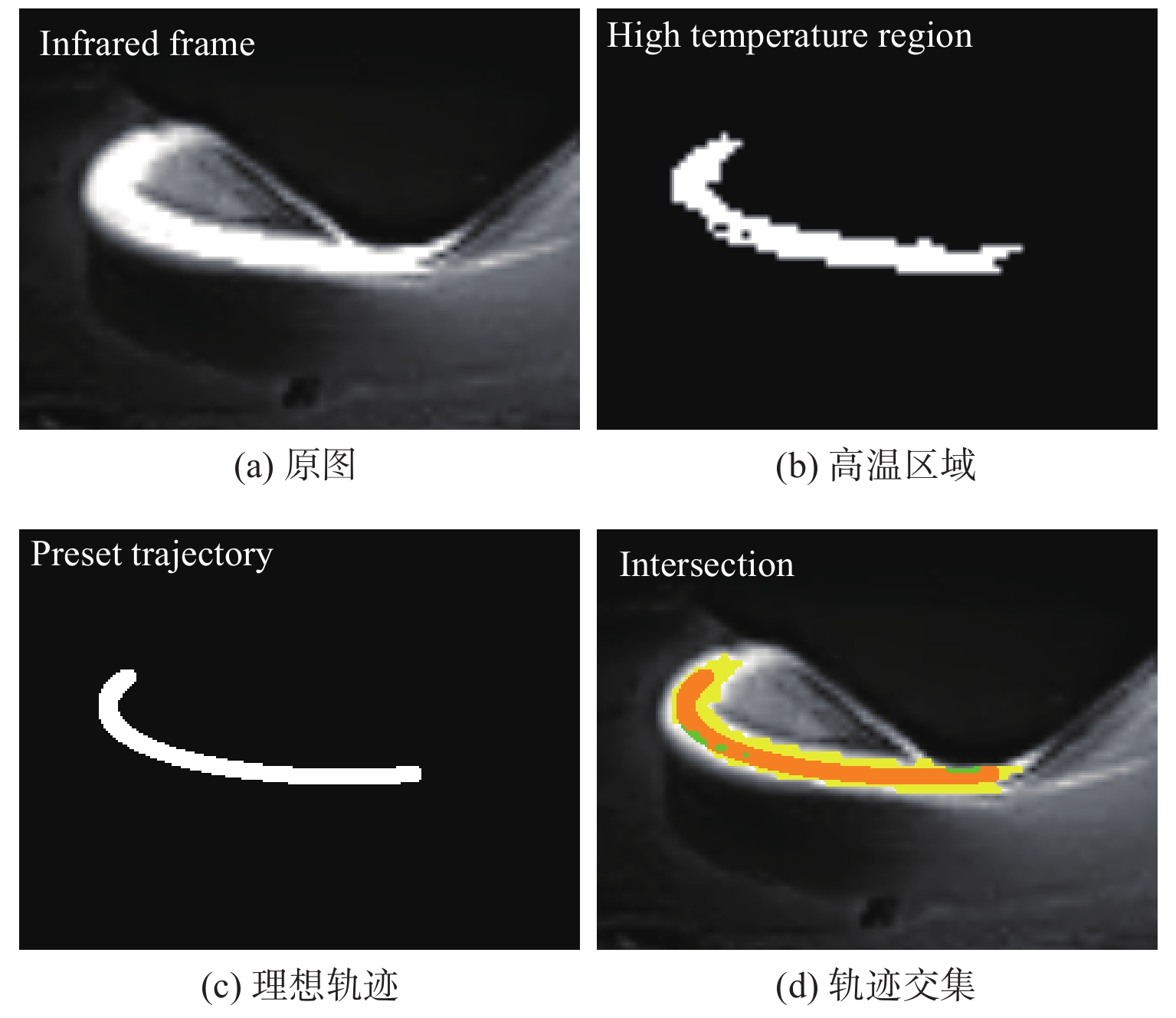
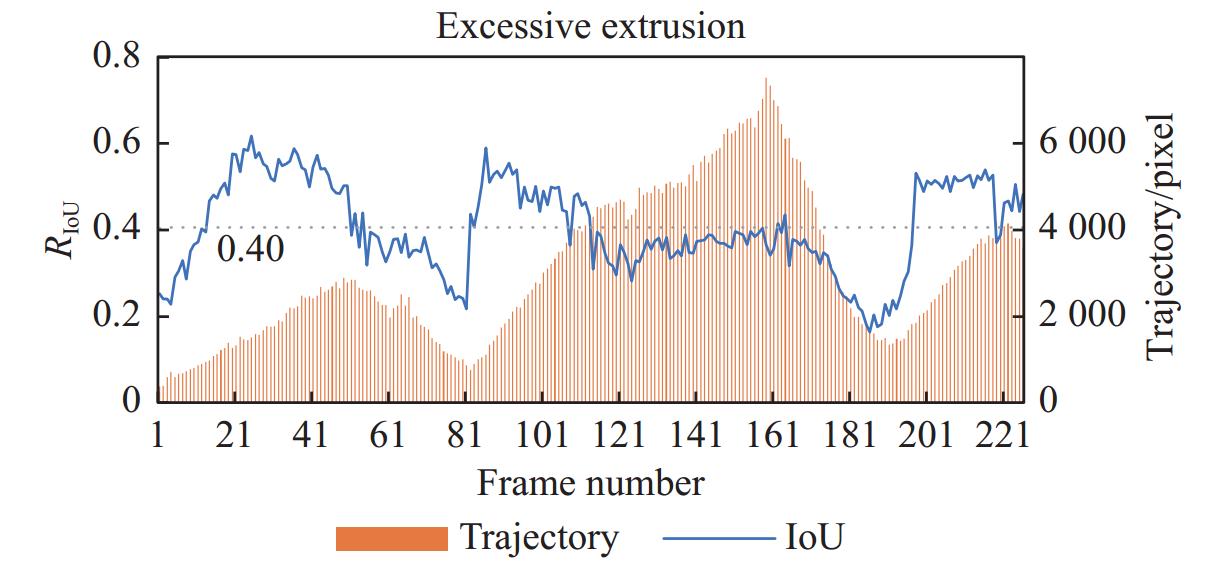
熔融沉积型（fused deposition modeling, FDM）增材制造是一个热加工过程，打印温度与运行精度的研究是2个相对独立的方向，红外成像中高温熔丝的形状信息通常得不到有效的利用，无法在温度监控的同时对打印设备的实时运行状态进行检测。提出了一种基于红外成像的FDM打印轨迹测量及质量评价方法，采用红外相机对FDM打印机的热加工过程进行连续测量，通过建立打印机系统坐标系与相机世界坐标系的位姿解算模型，以及动态帧间红外特征匹配的全局相机运动位姿解算模型，实现了对末端喷嘴运行位姿的实时测量，得到了精确的打印轨迹信息。在此基础上，建立了空间打印轨迹与红外图像中高温熔丝间的物像映射关系，并在图像域对打印过程中的典型缺陷进行了质量评价，其中图像处理平均耗时为25.9 ms，红外相机位姿测量的重投影误差为0.7像素，正常打印状态下，系统计算得到的理想打印轨迹与红外图像内高温区域的平均交并比为0.61。实验结果表明，该方法能够对打印过程中出现的模型错位、出料异常等典型问题进行准确识别，为打印质量在线评价的相关研究提供了一种新的解决途径。
Abstract:Fused deposition modeling (FDM) is a hot working process, the research on temperature and motion accuracy in the printing process are independent directions, and the shape of the high temperature filament in the infrared image is usually not concerned, making it impossible to detect the operating status and temperature of the printer at the same time. An FDM printing trajectory measurement and quality evaluation method based on infrared imaging was proposed. The method adopted infrared camera to continuously monitor the FDM printing process, and established the pose solution model of the printer coordinate system and the camera world coordinate system, as well as the global camera motion pose solution model matching the infrared features between dynamic frames. The real time measurement of the running position of the end nozzle was realized, and the accurate print trajectory information was obtained. On this basis, the object image mapping relationship between the spatial print trajectory and the high temperature filament in the infrared image was established, and the quality of the printing process and typical defects were evaluated in the image domain. The average time of image processing was 25.9 ms, and the reprojection error of infrared camera posture measurement was 0.7 pixel. Under normal printing conditions, the average IoU between the ideal print trajectory calculated by the system and the high temperature region in the infrared image is 0.61. The experimental results show that the proposed method can accurately identify the typical problems such as model dislocation and abnormal extruding in the printing process, which provides a new solution for the related research of online print quality evaluation.
-
引言
水下视觉成像在海底资源勘探、海洋开发、水下探测、水下反恐等方面有广泛的应用[1-2]。众所周知，水下声成像[3]的图像分辨率低，目标细节无法从图像中获取，难以满足上述应用需要。水下光成像，尤其是水下主动激光成像技术，相比于水下声成像有较高的成像分辨率，因而在海洋探索以及海洋军事方面具有重要的应用价值[4-6]。
激光逐点扫描三角测距成像[7-8]因其简单易行、成本低，受到人们的关注，但存在的问题是系统的横向扫描测量范围和纵向测距范围相互制约。M.Rioux等[9-10]提出的同步扫描三角测距成像方法，通过扫描光路与接收成像光路共用一扫描器，使得接收视场与扫描光束方向同步，扩大了系统横向扫描范围和纵向景深。文献[11]和[12]进一步研究了激光同步扫描三角测距成像系统的特点，从理论上推出了一般意义轨迹圆方程，并得出系统所能测量的最大和最小范围、距离测量分辨率等与系统参数的关系规律。对于激光同步扫描三角测距成像系统水下应用而言，由于光线在“空气-玻璃-水”发射过程，以及“水-玻璃-空气”接收过程中不同界面的多次折射，其测量方程、系统特性与系统参数的关系等，与文献[13]和[14]得到的空气中应用情况完全不同。因此，有必要对其作专门研究和讨论。文献[15]和[16]研究了使用同步扫描技术获取水下目标的二维图像，但无法对目标物体精确测量。
本文针对激光同步扫描三角测距成像系统水下应用，通过理论建模，得出水下同步扫描三角测距成像系统的空间三维坐标测量关系表达式，进一步分析了系统主要参数对系统测量范围、距离测量分辨率的影响，为水下同步扫描三角测距成像系统的设计提供了理论基础。
1 水下同步扫描三角测量系统建模
水下同步扫描三角测量系统工作原理如图 1(a)所示。激光器发出准直激光束经双面反射镜M3、反射镜M1反射后，通过密封窗玻璃M4进入水中，到达被测物体某一点$ \widetilde Q$，部分反射光通过密封窗玻璃M4进入空气中，经反射镜M2、双面反射镜M3另一反射面，由接收透镜接收，汇聚成像在成像探测器上。根据三角测距原理，汇聚成像的光点在成像探测器上的位置可以确定相对应的$ \widetilde Q$点的坐标。当M3绕轴往复摆动时，光点$ \widetilde Q$在被测物体表面上沿X轴方向行扫描；将该装置摆动或平移(本文采用平移方式)，光点$ \widetilde Q$在被测物体表面作光栅轨迹扫描，可得整个物面的三维坐标。
图 1(b)系统二维图中，双面反射镜M3位置坐标为系统坐标原点O=(0 0)，初始角度X轴方向为θ3。反射镜M1、M2位置坐标分别是：PM1=(d13 0)、PM2=(d23 0)，相对X轴的角度分别为θ1、θ2。焦距f0的接收透镜孔径为D，水平安放位置c=(0 -s)；成像探测器沿光轴左右对称，与透镜主平面的倾斜角度为0°；密封窗玻璃M4安放位置PM4=(0 h)，垂直于X-Y平面，厚度为e。系统基线距离为d=d13+d32。在图 1(b)中虚线为不发生界面折射时的光路，实线为发生界面折射后的光路。
图 2的光路展开中点P为初始位置点，在成像探测器上对应点为p0；P′点为M3转动一定角度，成像探测器上对应点未发生改变仍为p0的位置点；对于任意一点Q(x y)，M3转动一定角度且成像面光点位置发生改变，在成像探测器上对应点为p点，p点相对成像探测器主点p0的位置，记为q，是有方向的实数，与成像探测器转角同向为正，反向为负。
M3的转角为θ3时，发射光路激光在空气中方向向量为$ di{r_{\overline {E'{A_{p'}}} }} = 2{\theta _1} - 2{\theta _3} - \left( {{\rm{ \mathit{ π} }}/2} \right)$，接收光路激光在空气中方向向量为$di{r_{\overline {{W_{P'}}H'} }} = 2{\theta _2} - 2{\theta _3} - \left( {{\rm{ \mathit{ π} }}/2} \right) $，M3在展开光路中分别为M3′和M3″，O点位置分别对应展开光路的O′和O″点。O′和O″分别表示为
$$ \begin{array}{l} O' = {P_{{\rm{M1}}}} - \overline {O'{P_{{\rm{M1}}}}} = {\left[ {\begin{array}{*{20}{c}} { - {d_{13}} - {d_{13}}\cos \left( {2{\theta _1} - {\rm{ \mathit{ π} }}} \right)}\\ { - {d_{13}}\sin \left( {2{\theta _1} - {\rm{ \mathit{ π} }}} \right)} \end{array}} \right]^{\rm{T}}}\\ O'' = {P_{{\rm{M2}}}} + \overline {{P_{{\rm{M2}}}}O''} = {\left[ {\begin{array}{*{20}{c}} {{d_{32}} + {d_{32}}\cos \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right)}\\ {{d_{32}}\sin \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right)} \end{array}} \right]^{\rm{T}}} \end{array} $$ (1) 由(1)式可知，O′和O″点位置不随M3转动而变化。发射光线在展开光路中由于M3的转动由实线变化为点划线，转动中心为O′，如图 3所示。
![]()
图 3 成像点与成像光线角度之间的关系Figure 3. Relationship between imaging point and imaging angle of light如图 1(b)所示，设M3镜片厚度为ThM3，坐标原点O到M3上反射面的距离为ThM3_up，E、H两点的坐标分别为
$$ \begin{array}{l} E = {\left[ {\begin{array}{*{20}{c}} 0&{\frac{{T{h_{{\rm{M3\_up}}}}}}{{\cos \left( {{\theta _3}} \right)}}} \end{array}} \right]^{\rm{T}}}\\ H = {\left[ {\begin{array}{*{20}{c}} 0&{\frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}}} \end{array}} \right]^{\rm{T}}} \end{array} $$ (2) E、H在展开光路中对应点E′、H′的坐标为
$$ E' = O' + \overline {O'E'} = {\left[ {\begin{array}{*{20}{c}} { - {d_{13}} - {d_{13}}\cos \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}}}}{{\cos \left( {{\theta _3}} \right)}}\cos \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right)}\\ { - {d_{13}}\sin \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}}}}{{\cos \left( {{\theta _3}} \right)}}\sin \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right)} \end{array}} \right]^{\rm{T}}} $$ (3) $$ H' = O'' + \overline {O''H'} = {\left[ {\begin{array}{*{20}{c}} {{d_{32}} + {d_{32}}\cos \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}}\cos \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right)}\\ {{d_{32}}\sin \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}}\sin \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right)} \end{array}} \right]^{\rm{T}}} $$ (4) 由于M3具有一定厚度，因此光路行程会发生微小改变。透镜光心c到H点的行程为$s' = \left\| {\overline {cH} } \right\| = s + \frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}} $。透镜光心c在展开光路中的对应点c′坐标为
$$ c' = H' + \overline {H'c'} = \left[ {\begin{array}{*{20}{c}} {{d_{32}} + {d_{32}}\cos \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}}\cos \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right) + s'\cos \left( {2{\theta _2}{\rm{ - }}2{\theta _3}{\rm{ - }}\frac{{\rm{ \mathit{ π} }}}{2}} \right)}\\ {{d_{32}}\sin \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}}\sin \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right){\rm{ + }}s'{\rm{sin}}\left( {2{\theta _2}{\rm{ - }}2{\theta _3}{\rm{ - }}\frac{{\rm{ \mathit{ π} }}}{2}} \right)} \end{array}} \right] $$ (5) 图 3为成像光束主光线方向。在三角形Δc′p0p中，已知$ \angle c'{p_0}p = \frac{{\rm{ \mathit{ π} }}}{{\rm{2}}}$，$\left| {{p_0}c'} \right| = {f_0} $，$\left| {{p_0}p} \right| = q $，由正弦定理可得：$ \alpha = \arctan \left( {\frac{q}{{{f_0}}}} \right)$，因此成像光线方向为
$$ di{r_{\overline {WQP} }} = di{r_{\overline {c'p} }} = 2{\theta _2}{\rm{ - }}2{\theta _3}{\rm{ - }}\frac{{\rm{ \mathit{ π} }}}{2} + \alpha $$ (6) 如图 2所示，点$\widetilde Q $对应的Q点既在投射激光方向上又在入射成像光线上，由于光线经“空气-玻璃-水”界面的多次折射，Q点为这两条折线的交点。当M3的转角为θ3，成像探测器上成像点位置为p时，折线各段的方向向量如表 1所示。其中n1，n2，n3分别为光在空气、玻璃和水中的折射率。
表 1 各段光路方向向量Table 1. Direction vector for each section of light path线段 方向向量 线段 方向向量 E′AQ $di{r_{\overline {E'{\mathit{A}_\mathit{Q}}} }} = 2{\theta _1}{\rm{ - }}2{\theta _3}{\rm{ - }}\left( {{\rm{ \mathit{ π} }}/2} \right) $ WQH′ $di{r_{\overline {{W_Q}H'} }} = 2{\theta _2}{\rm{ - }}2{\theta _3}{\rm{ - }}\left( {{\rm{ \mathit{ π} }}/2} \right) + \alpha $ AQA′Q $di{r_{\overline {{A_Q}A{'_Q}} }} = \arccos \left( {\frac{{{n_1}}}{{{n_2}}}\cos \left( {di{r_{\overline {E'{\mathit{A}_{P'}}} }}} \right)} \right) $ WQW′Q $di{r_{\overline {{W_Q}W{'_Q}} }} = \arccos \left( {\frac{{{n_1}}}{{{n_2}}}\cos \left( {di{r_{\overline {{W_{P'}}H'} }}} \right)} \right) $ AQQ $di{r_{\overline {A{'_Q}Q} }} = \arccos \left( {\frac{{{n_2}}}{{{n_3}}}\cos \left( {di{r_{\overline {{A_{P'}}A{'_{P'}}} }}} \right)} \right) $ WQQ $di{r_{\overline {W{'_Q}Q} }} = \arccos \left( {\frac{{{n_2}}}{{{n_3}}}\cos \left( {di{r_{\overline {{W_{P'}}W{'_{P'}}} }}} \right)} \right) $ $$ \left\{ \begin{array}{l} \cot \left( {di{r_{\overline {E'{\mathit{A}_\mathit{Q}}} }}} \right) = \frac{{{A_{{Q_x}}} - O{'_x}}}{{{A_{{Q_y}}} - O{'_y}}}\\ \cot \left( {di{r_{\overline {c'{W_Q}} }}} \right) = \frac{{{W_{{Q_x}}} - c{'_x}}}{{{W_{{Q_y}}} - c{'_y}}} \end{array} \right. $$ (7) 解(7)式中2个方程，可得投射激光和入射成像光线与密封罩下表面的交点坐标：
$$ \left\{ \begin{array}{l} {A_Q} = {\left[ {\begin{array}{*{20}{c}} {\cot \left( {di{r_{\overline {E'{\mathit{A}_\mathit{Q}}} }}} \right)\left( {h - Q{'_y}} \right) - O{'_x}}&h \end{array}} \right]^{\rm{T}}}\\ {W_Q} = {\left[ {\begin{array}{*{20}{c}} {\cot \left( {di{r_{\overline {c'{W_Q}} }}} \right)\left( {h - c{'_y}} \right) - c{'_x}}&h \end{array}} \right]^{\rm{T}}} \end{array} \right. $$ (8) $$ \left\{ \begin{array}{l} \cot \left( {di{r_{\overline {{\mathit{A}_\mathit{Q}}A{'_Q}} }}} \right) = \frac{{{A_{{Q_x}}} - A{'_{{Q_x}}}}}{{{A_{{Q_y}}} - A{'_{{Q_y}}}}}\\ \cot \left( {di{r_{\overline {{W_\mathit{Q}}W{'_Q}} }}} \right) = \frac{{{W_{{Q_x}}} - W{'_{{Q_x}}}}}{{{W_{{Q_y}}} - W{'_{{Q_y}}}}} \end{array} \right. $$ (9) 解(9)式2个方程，可得投射激光和入射成像光线与密封罩上表面的交点坐标：
$$ \left\{ \begin{array}{l} A{'_Q} = {\left[ {\begin{array}{*{20}{c}} {\cot \left( {di{r_{\overline {{\mathit{A}_\mathit{Q}}A{'_Q}} }}} \right)\left( {h + e - {A_{{Q_y}}}} \right)}&{h + e} \end{array}} \right]^{\rm{T}}}\\ W{'_Q} = {\left[ {\begin{array}{*{20}{c}} {\cot \left( {di{r_{\overline {{W_\mathit{Q}}W{'_Q}} }}} \right)\left( {h + e - {W_{{Q_y}}}} \right)}&{h + e} \end{array}} \right]^{\rm{T}}} \end{array} \right. $$ (10) $$ \left\{ \begin{array}{l} \cot \left( {di{r_{\overline {A{'_Q}Q} }}} \right) = \frac{{{Q_x} - A{'_{{Q_x}}}}}{{{Q_y} - {A_{{Q_y}}}}}\\ \cot \left( {di{r_{\overline {W{'_Q}Q} }}} \right) = \frac{{{Q_x} - W{'_{{Q_x}}}}}{{{Q_y} - W{'_{{Q_y}}}}} \end{array} \right. $$ (11) 解方程组(11)，可得Q点在光路展开图中的坐标：
$$ Q = \left[ {\begin{array}{*{20}{c}} {\frac{{\left( {h + h' + e} \right)\left[ {\cot \left( {di{r_{\overline {W{'_Q}Q} }}} \right) - \cot \left( {di{r_{\overline {A{'_Q}Q} }}} \right)} \right] + A{'_{{Q_x}}} - W{'_{{Q_x}}}}}{{\cot \left( {di{r_{\overline {W{'_Q}Q} }}} \right) - \cot \left( {di{r_{\overline {A{'_Q}Q} }}} \right)}}}\\ {\left( {{Q_y} - h - h' - e} \right)\cot \left( {di{r_{\overline {A{'_Q}Q} }} + A{'_{{Q_x}}}} \right)} \end{array}} \right] $$ (12) 本文采用图 1整个装置平移方式实现对物面的光栅轨迹扫描，取该装置的在导轨上的位移为Qz，测量点三维坐标为
$$ \widetilde Q = \left[ {\begin{array}{*{20}{c}} {\frac{{\left( {h + h' + e} \right)\left[ {\cot \left( {di{r_{\overline {W{'_Q}Q} }}} \right) - \cot \left( {di{r_{\overline {A{'_Q}Q} }}} \right)} \right] + A{'_{{Q_x}}} - W{'_{{Q_x}}}}}{{\cot \left( {di{r_{\overline {W{'_Q}Q} }}} \right) - \cot \left( {di{r_{\overline {A{'_Q}Q} }}} \right)}}}\\ {\left( {{Q_y} - h - h' - e} \right)\cot \left( {di{r_{\overline {A{'_Q}Q} }} + A{'_{{Q_x}}}} \right)}\\ {{Q_z}} \end{array}} \right] $$ (13) 2 水下同步扫描三角测距成像系统分析
2.1 距离测量分辨率分析
系统采用的成像探测器为线阵CCD或PSD，根据三角测距原理，距离测量分辨率由成像探测器上最小可分辨单元决定，采用的成像探测器长度L为30 mm，最小分辨单元为0.005 mm。根据公式(12)，采用Matlab对系统进行仿真分析，考察基线距离d、物镜焦距f0、接收透镜到原点坐标的距离s、密封窗玻璃到原点坐标的距离h等系统参数改变对距离测量分辨率的影响情况。图 4(a)为基线距离d、物镜焦距f0改变，测量距离分辨率变化情况，此时取s为30 mm，h为60 mm；图 4(b)为接收透镜到原点坐标的距离s、密封窗玻璃到原点坐标的距离h改变，测量距离分辨率变化情况，此时取f0为75 mm，d为80 mm。从图 4可见，基线距离d和物镜的焦距f0对分辨率影响较大，而接收透镜到原点坐标的距离s和密封窗玻璃到原点坐标的距离h对分辨率影响较小。
![]()
图 4 测量距离分辨力随系统参数变化情况Figure 4. Resolution for measure distance versus system parameters图 5(a)为物镜焦距f0=75 mm，基线距离d为80 mm、90 mm、100 mm时，距离分辨率在不同测量距离上的变化情况，此时s=30 mm，h=60 mm。从图 5(a)中可见，距离测量分辨率随基线距离增大而提高，随测量距离增大而降低。图 5(b)为取基线距离d=80 mm，物镜焦距f0为70 mm、80 mm、90 mm时，距离测量分辨率在不同测量距离上的变化情况，此时s=30 mm，h=60 mm。从图 5中可见，距离分辨率随物镜焦距f0增大而提高，随测量距离增大而降低。
![]()
图 5 距离测量分辨率与不同距离的关系Figure 5. Relationship of resolution for measuring distance with different distances2.2 测量范围分析
与系统测量范围有关的参数主要有：基线距d、接收透镜到原点坐标的距离s、接收透镜焦距f0、密封窗玻璃到原点坐标的距离h、成像探测器长度L，取d、s、f0、h、L分别为75 mm、30 mm、85 mm、60 mm、25 mm。将其中一个参数分别改变5 mm、10 mm，其余参数保持不变，系统测量范围变化量如表 2所示。根据表 2可知，成像探测器长度L对系统测量范围的影响远大于其他参数。因此，成像探测器长度L是影响系统测量范围的主要参数。
表 2 系统测量范围变化Table 2. Change of system measurement range任一参数改变5 mm，其他参数不变 d s f0 h L 测量范围变化 横向/mm 25.45 4.37 -37.05 -0.08 176.83 纵向/mm 47.26 12.23 -98.63 -0.33 461.65 任一参数改变5 mm，其他参数不变 d s f0 h L 测量范围变化 横向/mm 51.62 8.23 -64.91 -0.17 575.09 纵向/mm 95.22 24.26 -174.62 -0.66 1473.76 根据同步扫描三角测距原理，成像探测器上任意点p所对应的物点为一曲线，左右极点决定系统最大、最小曲线轨迹，系统测量范围由最大、最小曲线轨迹决定。不同成像探测器长度下，系统最大、最小曲线轨迹如图 6所示，其中横坐标是横向扫描范围，纵坐标为纵向测距范围。图 6(a)表示L=20 mm时，系统最大、最小曲线轨迹；图 6(b)表示L=30 mm时，系统最大、最小曲线轨迹；图 6(c)表示L=40 mm时，系统最大、最小曲线轨迹。计算结果表明，当L从20 mm增加到40 mm时，纵向测量范围增加了1 787 mm，横向测量范围增加了689 mm。
2.3 设计实例
作为设计实例，取基线距离d为100 mm、物镜焦距f0为75 mm、接收透镜到原点坐标的距离s为25 mm、密封窗玻璃到原点坐标的距离h为50 mm、成像探测器长度L为37 mm，系统扫描测量范围和扫描测量范围内的距离测量分辨率如图 7所示。由图 7可知，在该参数条件下，最远处的距离测量分辨率为1.97 mm，最近处的距离测量分辨率为0.04 mm，系统横向扫描范围为864 mm，系统纵向测量范围为1 899 mm。
![]()
图 7 系统测量范围和距离测量分辨率Figure 7. System measurement range and distance measurement resolution3 结论
本文考虑水下同步扫描三角测量系统中, 光线在不同介质界面的折射效应, 通过理论建模，得出成像系统测量方程。分析了基线距离d、接收透镜焦距f0、成像探测器长度L等参数对距离测量分辨率及测量范围的影响。通过仿真分析可知，接收透镜焦距f0和基线距离d是影响距离测量分辨率的主要系统参数，成像探测面长度L决定系统测量范围。因此，在水下同步扫描三角测距成像系统设计时，可从系统性能要求出发，调整接收透镜焦距f0和基线距离d以满足距离测量分辨率要求。选取合适的成像探测面长度L，以满足系统测量范围要求，从而达到优化系统设计的目的，为水下同步扫描三角测距成像系统的设计提供参考。
-
![]()
图 17 圆柱内外壁打印过程红外图像
Figure 17. Infrared image in printing process of cylinder inner and outer wall
![]()
图 18 正常打印过程高温区域面积与交并比
Figure 18. High temperature area and IoU in normal printing process
![]()
图 20 错位打印过程高温区域面积与交并比
Figure 20. High temperature area and IoU in misalignment printing process
![]()
图 22 出料不足状态的打印高温区域面积与交并比
Figure 22. High temperature area and IoU in insufficient extrusion printing
![]()
图 24 出料过量状态的打印高温区域面积与交并比
Figure 24. High temperature area and IoU in excessive extrusion printing
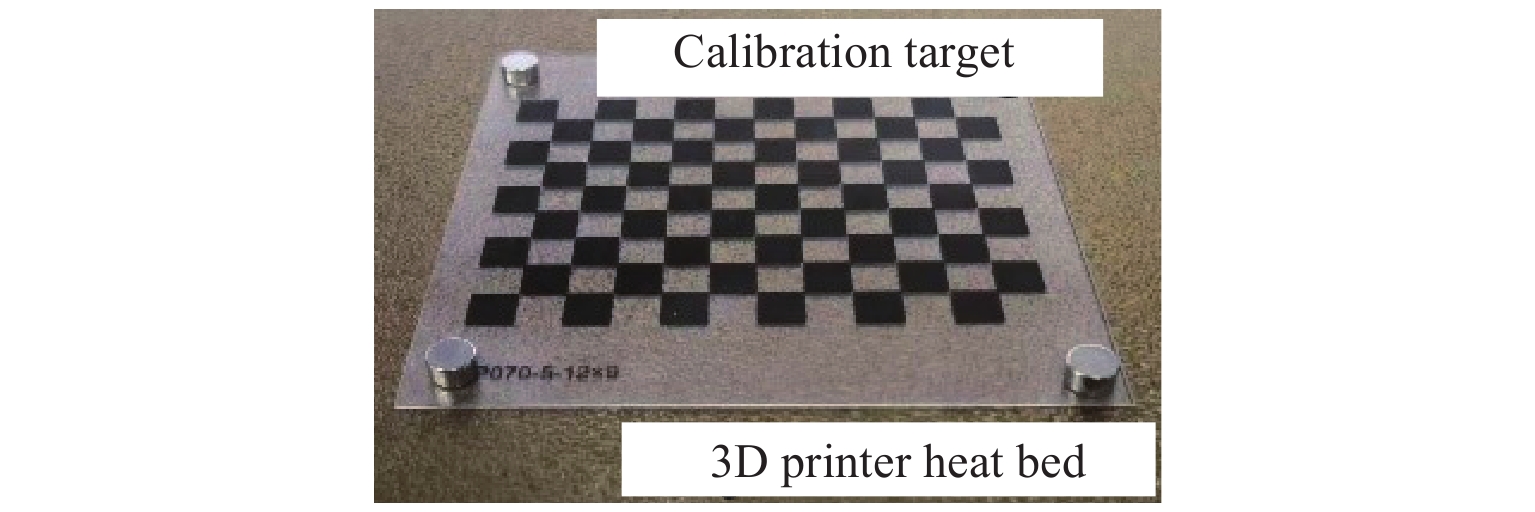
表 1 红外相机标定参数
Table 1 Infrared camera calibration parameters
参数 标定值 $ [{f_x},{f_y}]/{\text{pixel}} $ [334.767, 334.217] $[{u_0},{v_0}]/{\text{pixel}}$ [126.215, 100.097] ${k_1},{k_2},{k_3},{p_1},{p_2}$ [− 0.4112 ,0.1012 ,0.0008 , −0.1810 ,0.9387 ]
下载: 导出CSV
-
[1] 葛正浩, 岳奇, 吉涛. 3D打印控制系统研究综述[J]. 现代制造工程, 2021(10): 154-162. GE Zhenghao, YUE Qi, JI Tao. An overview of the research on 3D printing control systems[J]. Modern Manufacturing Engineering, 2021(10): 154-162.
[2] CHEN W J, HO J H, MUSTAPHA K B, et al. A vision based system for anomaly detection and classification in additive manufacturing[C]//2019 IEEE Conference on Sustainable Utilization and Development in Engineering and Technologies (CSUDET). Malaysia: IEEE, 2019: 87-92.
[3] BECKER P, SPIELBAUER N, ROENNAU A, et al. Real-time In-situ process error detection in additive manufacturing[C]//2020 Fourth IEEE International Conference on Robotic Computing (IRC). Taiwan, China: IEEE, 2020: 426-427.
[4] KOPSACHEILIS C, CHARALAMPOUS P, KOSTAVELIS I, et al. In situ visual quality control in 3D printing[C]//11th International Conference on Information Visualization Theory and Applications. [S.l.]: [S.n.], 2020: 317-324.
[5] RACHMAWATI S M, PARAMARTHA PUTRA M A, JUN T, et al. Fine-tuned CNN with data augmentation for 3D printer fault detection[C]//2022 13th International Conference on Information and Communication Technology Convergence (ICTC). Korea: IEEE, 2022: 902-905.
[6] 屈晨光, 张师军, 高达利, 等. 环境温度对3D打印成型精度的影响[J]. 塑料工业, 2015, 43(8): 53-55. doi: 10.3969/j.issn.1005-5770.2015.08.013 QU Chenguang, ZHANG Shijun, GAO Dali, et al. Effect of ambient temperature on the 3D printing molding precision[J]. China Plastics Industry, 2015, 43(8): 53-55. doi: 10.3969/j.issn.1005-5770.2015.08.013
[7] 徐子又, 胡镔, 邢泽华, 等. 层间预熔温度对熔融沉积成型打印件力学性能的影响[J]. 塑料科技, 2019, 47(5): 37-43. XU Ziyou, HU Bin, XING Zehua, et al. Effect of interlayer premelting temperature on mechanical properties of fused deposition molded printed parts[J]. Plastics Science and Technology, 2019, 47(5): 37-43.
[8] 张金立, 施一萍, 刘瑾, 等. 非封闭式FDM 3D打印机喷头温度控制器研究[J]. 传感器与微系统, 2021, 40(1): 53-55. ZHANG Jinli, SHI Yiping, LIU Jin, et al. Research on temperature controller of non-closed FDM 3D printer nozzle[J]. Transducer and Microsystem Technologies, 2021, 40(1): 53-55.
[9] WANG F F, JU F, ROWE K, et al. Real-time control for large scale additive manufacturing using thermal images[C]//2019 IEEE 15th International Conference on Automation Science and Engineering (CASE). Canada: IEEE, 2019: 36-41.
[10] POOLADVAND K, SALERNI A D, FURLONG C. In-situ thermal monitoring of printed components during rapid prototyping by fused deposition modeling[M]//Conference Proceedings of the Society for Experimental Mechanics Series. Cham: Springer International Publishing, 2020: 131-140.
[11] ZHANG Z Y. A flexible new technique for camera calibration[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 2000, 22(11): 1330-1334. doi: 10.1109/34.888718
[12] 王谭, 王磊磊, 张卫国, 等. 基于张正友标定法的红外靶标系统[J]. 光学 精密工程, 2019, 27(8): 1828-1835. doi: 10.3788/OPE.20192708.1828 WANG Tan, WANG Leilei, ZHANG Weiguo, et al. Design of infrared target system with Zhang Zhengyou calibration method[J]. Optics and Precision Engineering, 2019, 27(8): 1828-1835. doi: 10.3788/OPE.20192708.1828
[13] COLLINS T, BARTOLI A. Infinitesimal plane-based pose estimation[J]. International Journal of Computer Vision, 2014, 109(3): 252-286. doi: 10.1007/s11263-014-0725-5
[14] 宋栓军, 邱成鸿, 徐微, 等. 红外热像下激光熔丝成形过程冷却速率实时监测[J]. 红外与激光工程, 2022, 51(11): 3788/IRLA20220074. SONG Shuanjun, QIU Chenghong, XU Wei, et al. Real time monitoring of cooling rate in laser metal-wire forming process under infrared thermography[J]. Infrared and Laser Engineering, 2022, 51(11): 3788/IRLA20220074.
[15] 朱进前, 凌泽民, 杜发瑞, 等. 激光熔丝增材制造温度场的红外热像监测[J]. 红外与激光工程, 2018, 47(6): 0604002. doi: 10.3788/IRLA201847.0604002 ZHU Jinqian, LING Zemin, DU Farui, et al. Monitoring of laser metal-wire additive manufacturing temperature field using infrared thermography[J]. Infrared and Laser Engineering, 2018, 47(6): 0604002. doi: 10.3788/IRLA201847.0604002
-
期刊类型引用(6)
1. 王宇航，王新宇，张景辉，卜璐杰，张韬. 水下三维光学成像探测技术综述及展望. 激光与光电子学进展. 2024(22): 29-42 . 
百度学术
2. 张伟，李忠，袁四化，李梵若. 数据挖掘的单光束脉冲激光方位识别精度分析. 激光杂志. 2022(08): 180-184 . 百度学术
3. 吉勇，屠大维，张旭，李晨. 水下主动激光扫描系统中光场记录及散射背景分离研究. 电子测量与仪器学报. 2020(05): 58-64 . 百度学术
4. 卢治功，贺鹏，职连杰，陈文建. 基于最小二乘法多项式拟合三角测量模型研究. 应用光学. 2019(05): 853-858 . 本站查看
5. 田锋，黄秀祥，姜捷来，李仲阳. 反求工程关键问题研究综述. 装备制造技术. 2019(08): 18-20 . 百度学术
6. 赵丽花. 基于大数据分析的激光位移传感器测距自动校准方法研究. 激光杂志. 2019(11): 191-194 . 百度学术
其他类型引用(5)
计量
- 文章访问数: 64
- HTML全文浏览量: 6
- PDF下载量: 38
- 被引次数: 11
陕公网安备 61011302001501号 