Calibration method of topography error of white light interferometry on curved surface sample measurement
-
摘要:
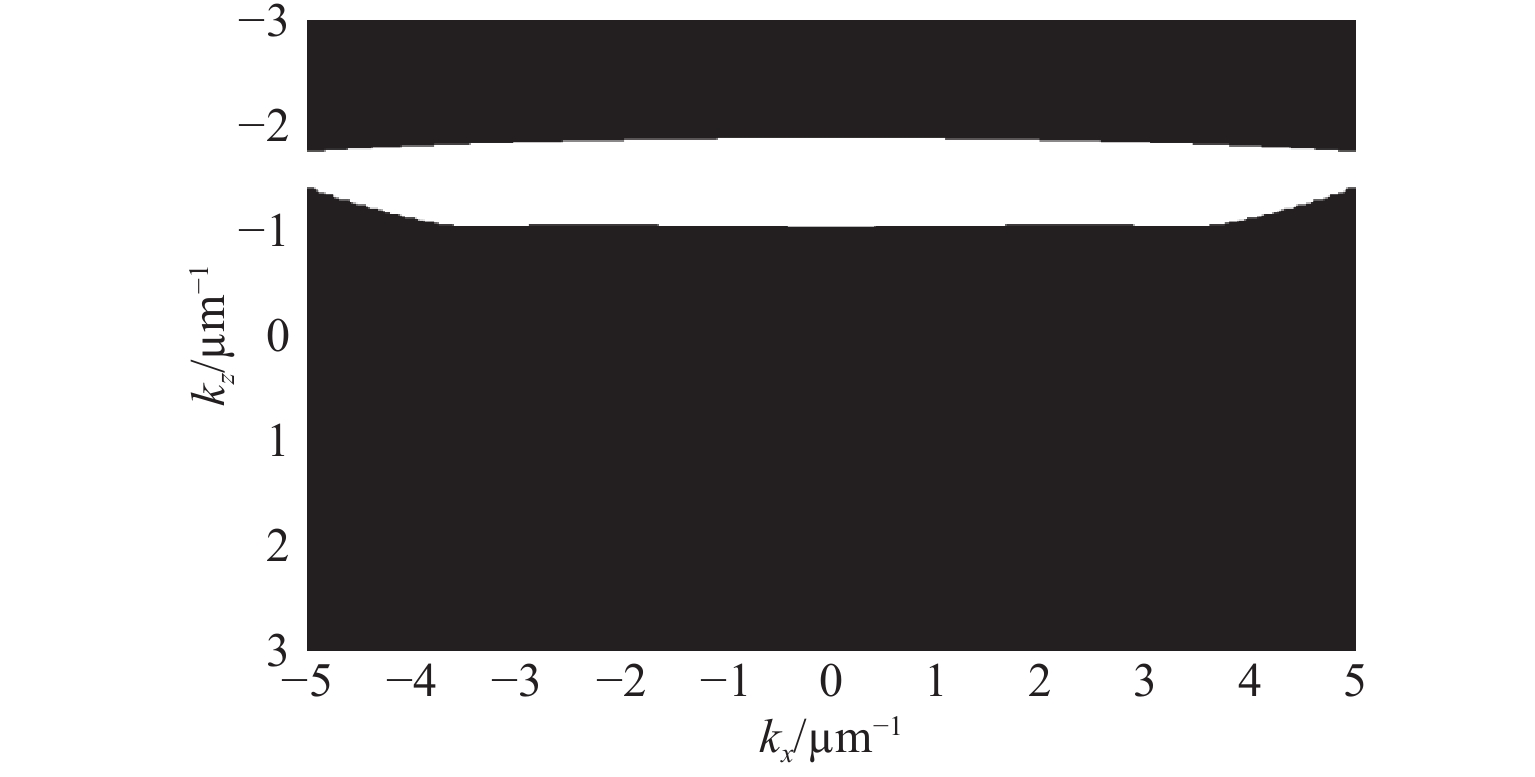
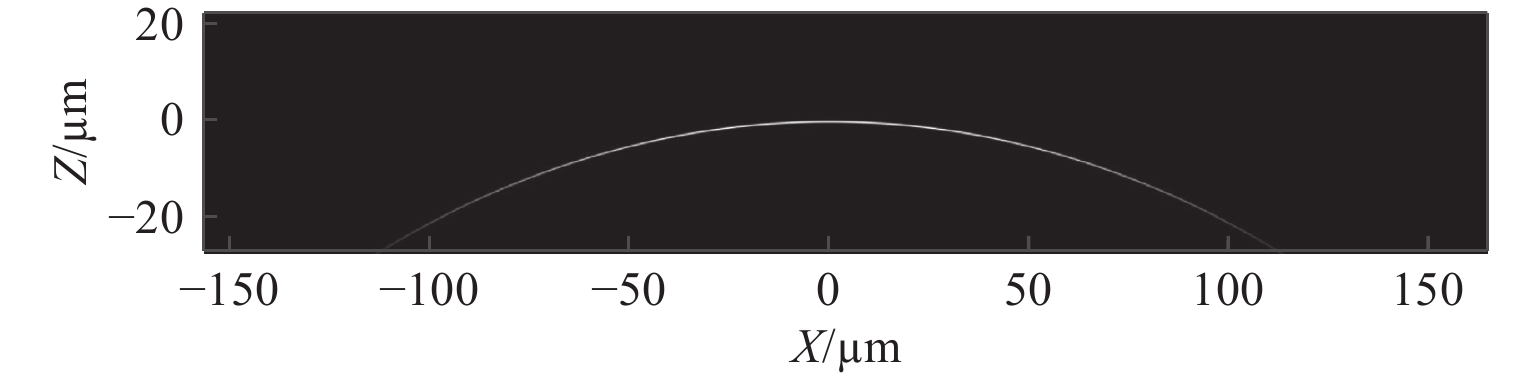
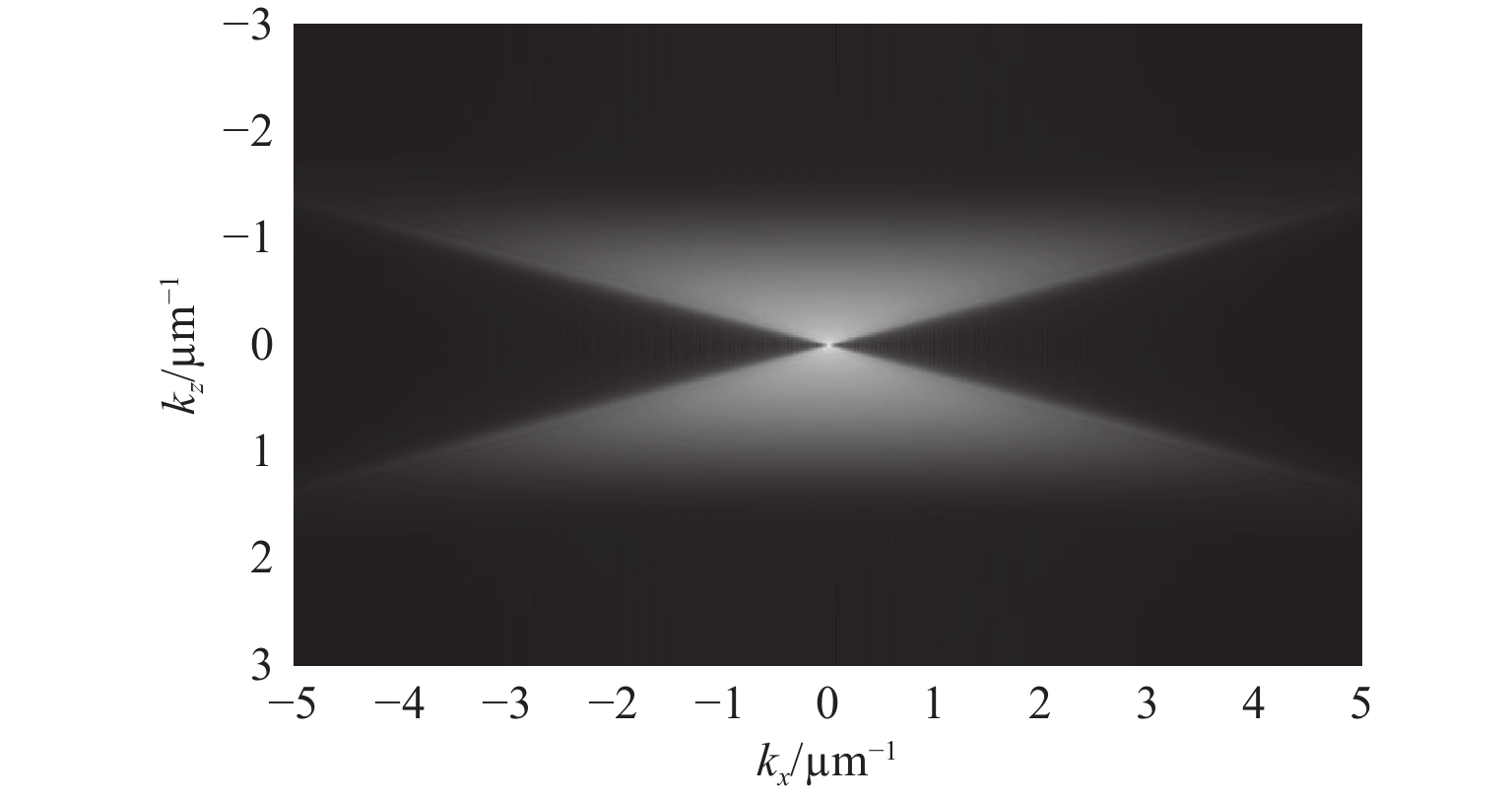
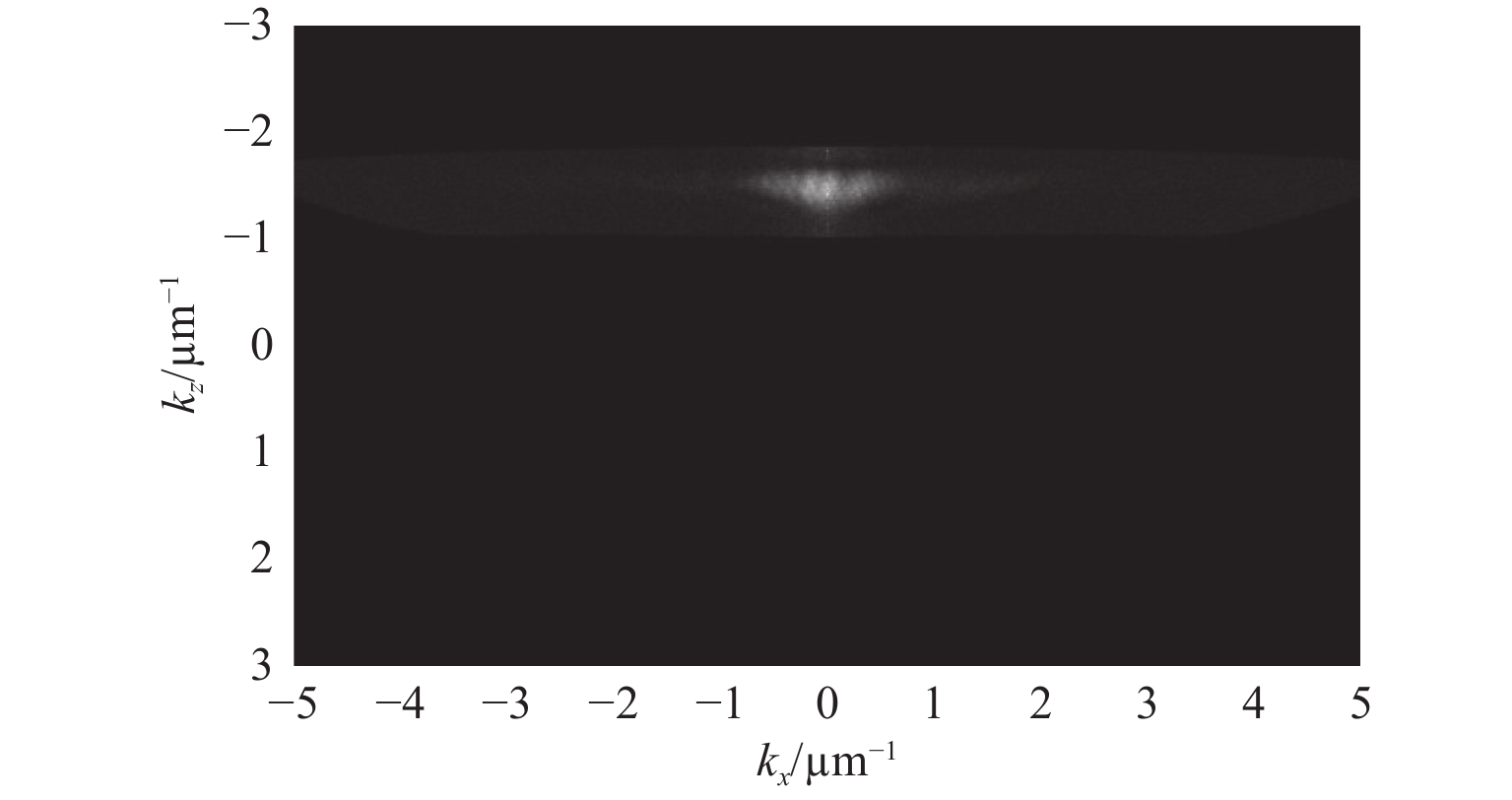
白光显微干涉术在平面阶跃型结构的形貌测量中具有显著优势。但在测量斜率变化的曲面样品时，由于物镜数值孔径的限制，样品表面反射光随着斜率的增大而减弱，干涉信号对比度降低，导致形貌测量结果的误差增大。基于表面传递函数（surface transfer function, STF）计算得到的逆滤波器可用于校正曲面样品的形貌测量误差，但现有方法的逆滤波器增益受限，无法有效提升频谱中的高频信号，对最大可测量斜率的提升有限。针对该问题，提取由白光干涉仪特性参数计算获得的虚拟STF的模作为振幅增益函数，由干涉图傅里叶变换得到的实测STF的相位作为相位补偿函数，形成虚实融合型逆滤波器，据此实现白光干涉仪曲面形貌测量误差的校正。应用该方法校正微球的形貌测量结果，校正后最大可测量斜率从8.09°提升到21.20°，均方根误差从0.545 5 μm降低至0.175 9 μm，实现了提升曲面样品的最大可测量斜率和减小测量误差的目的，有效提升了仪器针对曲面样品的测量范围。
Abstract:White light microinterferometry has obvious advantages in measuring the topography of planar step structures. However, due to the limitation of the numerical aperture of the objective lens, the reflected light on the surface of the sample is weakened with the increase of the slope when measuring the curved surface sample, and the contrast of the interference signal decreases, which leads to the increase of the error of topography measurement. Based on the theory of surface transfer function (STF), the inverse filter can be calculated to correct the topography measurement error of curved surface samples. However, the gain of the inverse filter of the existing method is limited, which is unable to elevate the high-frequency signal in the spectrum, and the improvement of the maximum measurable slope is limited. To address this issue, the modulus of the virtual STF calculated by the characteristic parameters of the white light interferometer was used as the amplitude gain function, and the phase of the measured STF obtained by the Fourier transform of the measured interferogram was used as the phase compensation function. A virtual-measured fusion inverse filter was formed, which realized the correction of the curved surface topography measurement error of white light interferometer. Using this method to correct the topography measurement results of the microsphere, the maximum measurable slope after correction is increased from 8.09° to 21.20°, and the root mean square error is reduced from 0.545 5 μm to 0.175 9 μm, which achieves the purpose of improving the maximum measurable slope of curved surface sample and reducing the measurement error, and effectively improves the measurement range of the instrument for the curved surface sample.
-
引言
陀螺是光电系统的重要传感器之一，其精度直接关系着光电系统最终的性能。采取陀螺冗余安装是提高角速率测量精度和系统可靠性的最有效方法，已广泛应用于惯性导航、故障检测与诊断等航空航天领域 [1-6]。在传感器数目确定的前提下，斜置冗余安装比正交冗余安装有更高的测量精度和可靠性[7]。富立等研究了基于可靠性的传感器冗余数量确定方法，梁海波等提出了9陀螺冗余配置的故障诊断与隔离，同时对系统精度和可靠性进行了分析。杜江松等研究了4陀螺冗余配置的传感器误差分析并对其可靠性和精度进行了验证。吴风喜等研究了基于斜装冗余传感器的分布式导航系统及信息融合方法。
本文提出将陀螺等传感器冗余安装应用于机载光电系统中，在考虑光电系统传感器安装空间、体积、重量和成本等因素的基础上，设计了八边形金字塔4陀螺斜置冗余安装方案。同时兼容4个磁流体动力学（magneto-hydrodynamics，MHD）角速率传感器冗余安装，便于后期将陀螺和MHD传感器数据融合。
1 冗余安装数量及方案
研究表明，冗余安装首先需要确定传感器冗余安装的数量。当传感器数量大于4时可构成冗余安装，用尽可能少的传感器冗余安装数量，达到系统所需要的测量精度和可靠性就十分重要[8-9]。当传感器数量增加到4时，冗余安装与无冗余安装比较，系统的可靠性增幅达到最大值[8]。结合光电系统实际安装空间、体积、重量和成本等因素，同时考虑到在3轴陀螺稳定光电系统中的移植，将陀螺冗余安装的数量确定为4。
常见的4个陀螺冗余安装方式有正交安装、斜置安装、圆锥安装和对称斜置安装等。根据陀螺安装方式，建立直角坐标系，由安装结构可得陀螺安装矩阵H。在陀螺冗余安装中，冗余陀螺的输出方程为
$$ {\boldsymbol{Z}} = {\boldsymbol{Hx}} + \xi $$ (1) 式中：${\boldsymbol{Z}} = {\left[ {\begin{array}{*{20}{c}} {{\omega _1}}&{{\omega _2}}&{{\omega _3}}&{{\omega _4}} \end{array}} \right]^{\rm{T}}}$为4个陀螺的测量值；${\boldsymbol{x}} = {\left[ {\begin{array}{*{20}{c}} {{\omega _x}}&{{\omega _y}}&{{\omega _{\textit{z}}}} \end{array}} \right]^{\rm{T}}}$为待测量的3轴角速度；$ \xi $为陀螺测量噪声，为零均值高斯白噪声。
陀螺冗余安装测量方程是一个含有随机误差的方程，根据最小二乘估计原理，要求估计值能使所有测量值相对估计值的偏差平方和最小。当4个陀螺均工作正常时，满足最小二乘判据的最佳值$\hat x $为[10]
$$ \hat x = {({{\boldsymbol{H}}^{\rm{T}}}{\boldsymbol{H}})^{ - 1}}{{\boldsymbol{H}}^{\rm{T}}}Z $$ (2) 当陀螺无冗余正交安装时，陀螺的3轴测量精度就是单个陀螺的测量精度。在陀螺数目确定的情况下，Harrison和Gai研究的性能指标评判标准[11]为
$$ {F_p} = \sqrt {|{{({{\boldsymbol{H}}^{\rm{T}}}{\boldsymbol{H}})}^{ - 1}}|} $$ (3) 根据以上评判标准，当安装矩阵H使得$ {F_p} $的值最小时，则系统由噪声所引起的误差最小，系统可获得最佳特性，即最优冗余安装。设陀螺安装个数为n，根据精度最优准则，可得以下结论[9]：
$${{\boldsymbol{H}}^{\rm{T}}}{\boldsymbol{H}} = \frac{n}{3}I$$ (4) 当光电系统仅使用光纤陀螺传感器时，采用4个光纤陀螺对称斜置的冗余安装方式[12]，如图1所示。
![]()
图 1 光纤陀螺对称斜置冗余安装Figure 1. Schematic diagram of symmetrical and oblique redundant installation of fiber optic gyroscopes由安装结构可得陀螺斜置冗余安装矩阵H：
$$ {\boldsymbol{H}} = \left[ {\begin{array}{*{20}{c}} {\cos \alpha }&{\cos \alpha }&{\cos \alpha } \\ { - \cos \alpha }&{\cos \alpha }&{\cos \alpha } \\ { - \cos \alpha }&{ - \cos \alpha }&{\cos \alpha } \\ {\cos \alpha }&{ - \cos \alpha }&{\cos \alpha } \end{array}} \right] $$ (5) 故
$$ {{\boldsymbol{H}}^{\rm{T}}}{\boldsymbol{H}} = \left[ {\begin{array}{*{20}{c}} {4{{\cos }^2}\alpha }&0&0 \\ 0&{4{{\cos }^2}\alpha }&0 \\ 0&0&{4{{\cos }^2}\alpha } \end{array}} \right] = 4{\cos ^2}\alpha I $$ (6) 结合（4）式，可得：
$$ \alpha = 54.735\;6{\text{°}} $$ 因此，采用4陀螺对称斜置冗余安装时，根据精度最优原则，得安装角$\alpha = 54.735\;6{\text{°}}$
在机载光电系统中，传感器冗余安装的主要目的是提升角速率测量精度，同时将陀螺和MHD角速率传感器的数据融合，以提升角速率测量带宽。由于设计的陀螺和MHD角速率传感器的敏感轴不一致，故应先解算3轴角速率，再进行数据融合。设计的4个MEMS陀螺和4个MHD传感器间隔安装，如图2所示。
根据传感器布局，建立工程应用中易于计算安装角度的直角坐标系，4个陀螺均匀分布，相互间夹角为$ {90^\circ } $；4个MHD与4个陀螺间隔分布，相互之间夹角为$ {90^\circ } $。陀螺与MHD在水平面内的夹角为$ {45^\circ } $。根据此结构布局，则可得陀螺与MHD的安装矩阵H：
$$ {\boldsymbol{H}} = \left[ {\begin{array}{*{20}{c}} {\cos \alpha }&0&{\sin \alpha } \\ {\cos \alpha \cos \dfrac{\pi }{4}}&{\cos \alpha \sin \dfrac{\pi }{4}}&{\sin \alpha } \\ 0&{\cos \alpha }&{\sin \alpha } \\ { - \cos \alpha \cos \dfrac{\pi }{4}}&{\cos \alpha \sin \dfrac{\pi }{4}}&{\sin \alpha } \\ { - \cos \alpha }&0&{\sin \alpha } \\ { - \cos \alpha \cos \dfrac{\pi }{4}}&{ - \cos \alpha \sin \dfrac{\pi }{4}}&{\sin \alpha } \\ 0&{ - \cos \alpha }&{\sin \alpha } \\ {\cos \alpha \cos \dfrac{\pi }{4}}&{ - \cos \alpha \sin \dfrac{\pi }{4}}&{\sin \alpha } \end{array}} \right] $$ (7) 故
$$ {{\boldsymbol{H}}^{\rm{T}}}{\boldsymbol{H}} = \left[ {\begin{array}{*{20}{c}} {4{{\cos }^2}\alpha }&0&0 \\ 0&{4{{\cos }^2}\alpha }&0 \\ 0&0&{4{{\cos }^2}\alpha } \end{array}} \right] = 4{\cos ^2}\alpha I $$ (8) 根据测量精度最优准则，可求得
$$ \alpha = 35.264{\text{°}} $$ 如果仅安装MEMS陀螺，则安装矩阵如下：
$$ {\boldsymbol{H}} = \left[ {\begin{array}{*{20}{c}} {\cos \alpha }&0&{\sin \alpha } \\ 0&{\cos \alpha }&{\sin \alpha } \\ { - \cos \alpha }&0&{\sin \alpha } \\ 0&{ - \cos \alpha }&{\sin \alpha } \end{array}} \right] $$ (9) 可得
$$ {{\boldsymbol{H}}^{\rm{T}}}{\boldsymbol{H}} = \left[ {\begin{array}{*{20}{c}} {2{{\cos }^2}\alpha }&0&0 \\ 0&{2{{\cos }^2}\alpha }&0 \\ 0&0&{2{{\cos }^2}\alpha } \end{array}} \right] = 2{\cos ^2}\alpha I $$ (10) 同理，根据测量精度最优准则，可求得
$$ \alpha = 35.264{\text{°}} $$ 由以上结论可得，仅安装MEMS陀螺，或将MEMS陀螺与MHD传感器同时安装，均可按照以下结构形式冗余安装陀螺与MHD：将陀螺与MHD均匀间隔分布在以底面为正八边形，传感器轴线与底面夹角为$ \alpha = 35.264 $°的多面体上。
2 系统精度仿真分析
假设4个陀螺精度一致，按照4陀螺冗余安装方案进行仿真。其中$w {x_ - }r$、$w {y_ - }r$、$w {{\textit{z}}_ - }r$为参考角速率，经过$m = {\boldsymbol{H}}w$变换，4个测量值均叠加白噪声，再经最小二乘估计得到瞄准线3个轴的角速率，并与参考角速率叠加噪声后的数据进行对比，如图3所示。
在仿真中，$w {x_ - }r$、$w {y_ - }r$、$w {{\textit{z}}_ - }r$为陀螺参考角速率，即陀螺测量值，其取值分别为$w {x_ - }r = {10^ \circ }/{\rm s}$，$w {y_ - }r = {20^ \circ }/{\rm s}$，$w {{\textit{z}}_ - }r = {30^ \circ }/{\rm s}$。按对称斜置冗余安装方式，经坐标变换，陀螺测量值及叠加白噪声后的速度曲线如图4所示。
仿真结果表明，瞄准线3个轴的噪声标准差有明显下降，噪声抑制率为12.37%～14.96%（见表1），仿真结论与理论相符。
![]()
图 4 陀螺测量值及叠加白噪声后信号图Figure 4. Signal diagram of gyroscope measurement results and white noise superposition表 1 3轴信号标准差Table 1. Triaxial standard deviation of signal坐标轴 参考信号
标准差/(°·s−1)估计信号
标准差/(°·s−1)噪声
抑制率/%X 0.308 478 78 0.270 322 51 12.37 Y 0.329 151 02 0.284 831 31 13.46 Z 0.321 488 73 0.273 385 59 14.96 3 可靠性分析
惯性器件可靠性的定义[8]：惯性测量单元元件在一定时间间隔内能正常工作的概率即为惯性测量单元的可靠性，可用平均故障间隔时间（MTBF）描述。
假设所有陀螺的失效分布一致，失效率为常数的指数分布，单个陀螺的可靠度为[13]
$$ R(t) = {{\rm{e}}^{ - \lambda t}} $$ 式中：$ \lambda $为单位时间内发生故障的次数，即故障率；t为陀螺正常工作时间。
陀螺的平均故障间隔时间MTBF为[13]
$$ t_{\rm{MTBF}} = \int\limits_0^\infty {R(t)} {\rm{d}}t = \frac{1}{\lambda } $$ 在3轴机载光电系统中，选取单轴高精度光纤陀螺。因需要对方位、俯仰和横滚3个轴向的角速度信息测量，故其可靠性计算如下。
4个光纤陀螺对称斜置冗余安装中，4个陀螺同时工作或最多允许一个陀螺失效，陀螺冗余安装系统属于$ \dfrac{3}{4} $表决模型系统，则其可靠度和MTBF分别为
$$ {R_1} = 4{R^3}(1 - R) + {R^4} = 4{R^3} - 3{R^4} = 4{{\rm{e}}^{ - 3\lambda t}} - 3{{\rm{e}}^{ - 4\lambda t}} $$ $$ t_{{\rm{MTB}}{{\rm{F}}_1}} = \int\limits_0^\infty {{R_1}(t)} {\rm{d}}t = \int\limits_0^\infty {(4{{\rm{e}}^{ - 3\lambda t}} - 3{{\rm{e}}^{ - 4\lambda t}})} {\rm{d}}t = \frac{7}{{12\lambda }} $$ 3个单轴光纤陀螺无冗余安装时，必须全部工作正常。无冗余安装陀螺系统属于串联系统模型，则陀螺系统可靠度和MTBF分别为
$$ {R_2} = 3R = {{\rm{e}}^{ - 3\lambda t}} $$ $$ t_{{\rm{MTB}}{{\rm{F}}_2}} = \int\limits_0^\infty {{R_2}(t)} {\rm{d}}t = \int\limits_0^\infty {{{\rm{e}}^{ - 3\lambda t}}} {\rm{d}}t = \frac{1}{{3\lambda }} $$ 因此，对称斜置安装4个陀螺的方案的可靠性是3轴无冗余配置可靠性的1.75倍。同理可得，八边形金字塔冗余安装的可靠性是3轴无冗余配置可靠性的1.75倍。另外，对称斜置4个陀螺冗余安装方案可保证在单陀螺故障时，依然有较高的解算精度[14]。
4 实验结果与分析
静态噪声输出：陀螺1：0.028 9°/s；陀螺2：0.028 3°/s；陀螺3：0.024 1°/s；陀螺4：0.027 5°/s。冗余安装解算噪声输出：X轴0.020 7°/s，Y轴0.020 9°/s，Z轴0.027 1°/s。
以X轴为例，当X轴转动时，冗余安装方式和无冗余安装方式数据输出如表2所示。
表 2 X轴测量数据表Table 2. Measurement data results of X-axis(°)/s 转台转速 冗余安装输
出数据标准差冗余安装
输出数据无冗余安装
数据标准差无冗余
安装数据60.0 0.024 6 60.664 5 0.035 1 58.800 6 30.0 0.022 8 30.357 8 0.030 1 29.480 1 10.0 0.021 8 10.126 3 0.028 5 9.837 9 1.0 0.021 1 1.070 5 0.028 4 1.147 5 0.1 0.021 2 0.158 0 0.027 9 0.263 7 −0.1 0.021 8 −0.036 3 0.028 8 0.074 5 −1.0 0.020 4 −0.950 1 0.027 8 −0.802 8 −10 0.021 1 −10.053 4 0.028 8 −9.599 3 −30 0.023 1 −30.265 3 0.031 6 −29.142 7 −60 0.025 2 −60.579 5 0.031 3 −58.451 4 由表2可以看出，冗余安装与无冗余安装相比较，测量精度有明显提升，满足光电系统实际需求。冗余安装与无冗余安装相比较，平均标准差下降约25.3%。另外，陀螺测量精度性能提升还受冗余安装倾角加工精度及4个陀螺精度一致性等因素的影响。
在不同转速匀速转动时，冗余安装与无冗余安装相对比，测量的速度波动和速度线性度分析结果如图5所示。
![]()
图 5 X轴速度波动与线性度对比图Figure 5. Comparison curves of velocity fluctuation and linearity in X-axis由图5可看出，冗余安装方式速度波动明显优于单陀螺无冗余安装方式，冗余安装方式与无冗余安装方式速度线性度相当。
5 结论
本文提出了一种传感器数目确定的冗余安装方法，从理论和仿真两方面分析了冗余安装的精度和可靠性，同时通过实验验证，实现了陀螺冗余安装精度性能的提升。与传统的无冗余安装比较，传感器测量精度和可靠性均得到明显提升。其中，陀螺角速度测量噪声标准差下降约25.3%，可靠性提升1.75倍，验证了该方法的有效性，满足系统的实际需求。下一步将通过卡尔曼滤波后，把陀螺与MHD数据信息融合[15]，以提升传感器的带宽。该方法可以提高系统角速率传感器的测量精度和带宽，提升系统可靠性。随着机载光电系统的广泛应用，该方法也可应用于3轴光电系统中，具有广泛的使用价值。
-
![]()
图 2 白光干涉仪测量倾斜表面的NA圆锥极限
Figure 2. NA cone limit of white light interferometer when measuring tilted surface
![]()
图 3 虚实融合型逆滤波校正算法流程图
Figure 3. Flow chart of virtual-measured inverse filtering correction method
![]()
图 6 经过带通滤波前后的干涉条纹和条纹中心的干涉信号
Figure 6. Interference fringes and signals at fringe center before and after bandpass filtering
![]()
图 7 微球的表层膜模型（$x {\text{-}} {\textit{z}} $截面）
Figure 7. Foil model of microsphere ($x {\text{-}} {\textit{z}} $ cross section)
![]()
图 8 表层膜模型的频谱（${k_x} {\text{-}} {k_{\textit{z}}}$截面）
Figure 8. Spectrum of foil model (${k_x} {\text{-}} {k_{\textit{z}}}$ cross section)
![]()
图 9 白光干涉仪实测STF（${k_x} {\text{-}} {k_{\textit{z}}}$截面）
Figure 9. Measured STF of WLI (${k_x} {\text{-}} {k_{\textit{z}}}$ cross section)
![]()
图 10 两种逆滤波校正后的干涉条纹及对应的干涉信号
Figure 10. Interference fringes after different inverse filtering correction and corresponding signals at center of fringe
![]()
图 11 普通逆滤波校正后不同表面斜率对应的干涉信号
Figure 11. Interference signals corresponding to different surface slopes corrected by ordinary inverse filtering
![]()
图 12 虚实融合型逆滤波校正后不同表面斜率对应的干涉信号
Figure 12. Interference signals corresponding to different surface slopes corrected by virtual-measured fusion inverse filtering
![]()
图 13 经过两种逆滤波校正的形貌测量结果和误差
Figure 13. Topography measurement results and errors corrected by different inverse filtering
表 1 两种逆滤波校正方法结果的对比
Table 1 Comparison of results of two inverse filtering correction methods
Calibration
methodRMSE/μm Lateral measuring
range/μmMaximum
measurable
slope/(°)Uncalibrated 0.545 5 70.49 8.09 Ordinary inverse
filtered0.336 3 145.87 16.93 Virtual-measured
inverse filtered0.175 9 181.14 21.20 
下载: 导出CSV
-
[1] 高志山, 袁群, 孙一峰, 等. 微结构显微光学无损检测方法(特邀)[J]. 光子学报,2022,51(8):23-40. doi: 10.3788/gzxb20225108.0851501 GAO Zhishan, YUAN Qun, SUN Yifeng, et al. Non-destructive test methods of microstructures by optical microscopy(invited)[J]. Acta Photonica Sinica,2022,51(8):23-40. doi: 10.3788/gzxb20225108.0851501
[2] 陈善勇, 薛帅, 熊玉朋, 等. 面向制造的光学面形超精密测量技术研究进展[J]. 激光与光电子学进展,2023,60(3):177-190. CHEN Shanyong, XUE Shuai, XIONG Yupeng, et al. Research progress of ultra-precision measurement of optical surfaces for manufacturing[J]. Laser & Optoelectronics Progress,2023,60(3):177-190.
[3] 尹智瑶, 郭仁慧, 杨鑫, 等. 基于白光干涉的ICF靶丸表面缺陷测量方法[J]. 光学学报,2022,42(10):79-87. doi: 10.3788/AOS202242.1012004 YIN Zhiyao, GUO Renhui, YANG Xin, et al. Measurement method for surface defects on ICF capsules based on white light interferometry[J]. Acta Optica Sinica,2022,42(10):79-87. doi: 10.3788/AOS202242.1012004
[4] 张超, 袁群, 张佳乐, 等. 白光显微干涉三维形貌测量中的移相误差校正方法[J]. 红外与激光工程, 2022, 51(7): 341-348. ZHANG Chao, YUAN Qun, ZHANG Jiale, et al. Calibration method of the phase-shifting error for the topography measurement utilizing white light interferometric microscopy[J]. Infrared and Laser Engineering, 2022, 51(7): 341-348.
[5] KÜNNE M, HAGEMEIER S, KÄKEL E, et al. Investigation of measurement data of low-coherence interferometry at tilted surfaces in the 3D spatial frequency domain[J]. Tm-Technisches Messen,2021,88(S1):65-70. doi: 10.1515/teme-2021-0051
[6] GAO F, LEACH R K, PETZING J, et al. Surface measurement errors using commercial scanning white light interferometers[J]. Measurement Science and Technology,2008,19(1):015303. doi: 10.1088/0957-0233/19/1/015303
[7] SU R, WANG Y H, COUPLAND J, et al. On tilt and curvature dependent errors and the calibration of coherence scanning interferometry[J]. Optics Express,2017,25(4):3297-3310. doi: 10.1364/OE.25.003297
[8] XIE W C, LEHMANN P, NIEHUES J. Lateral resolution and transfer characteristics of vertical scanning white-light interferometers[J]. Applied Optics,2012,51(11):1795-1803. doi: 10.1364/AO.51.001795
[9] COUPLAND J M, LOBERA J. Holography, tomography and 3D microscopy as linear filtering operations[J]. Measurement Science and Technology,2008,19(7):074012. doi: 10.1088/0957-0233/19/7/074012
[10] MANDAL R, COUPLAND J, LEACH R, et al. Coherence scanning interferometry: measurement and correction of three-dimensional transfer and point-spread characteristics[J]. Applied Optics,2014,53(8):1554-1563. doi: 10.1364/AO.53.001554
[11] SU R, THOMAS M, LIU M Y, et al. Lens aberration compensation in interference microscopy[J]. Optics and Lasers in Engineering,2020,128:106015. doi: 10.1016/j.optlaseng.2020.106015
[12] SU R, THOMAS M, LIU M Y, et al. High-accuracy surface measurement through modelling of the surface transfer function in interference microscopy[C]//Proceedings of Applied Optical Metrology III. San Diego: SPIE, 2019, 11102: 19-25.
[13] COUPLAND J, MANDAL R, PALODHI K, et al. Coherence scanning interferometry: linear theory of surface measurement[J]. Applied Optics,2013,52(16):3662-3670. doi: 10.1364/AO.52.003662
[14] 苏榕, 刘嘉宇, 乔潇悦, 等. 用于表面形貌测量的扫描白光干涉技术进展[J]. 激光与光电子学进展,2023,60(3):60-80. SU Rong, LIU Jiayu, QIAO Xiaoyue, et al. Advances in scanning white light interferometry for surface topography measurement[J]. Laser & Optoelectronics Progress,2023,60(3):60-80.
[15] SU R, LEACH R. Physics-based virtual coherence scanning interferometer for surface measurement[J]. Light: Advanced Manufacturing,2021,2(2):120-135. doi: 10.37188/lam.2021.009
-
期刊类型引用(4)
1. 林正煜，张继艳，孙丽婷，秦腾，曹天皓. 大面阵高分辨率三变倍双远心光学设计. 激光与红外. 2024(05): 796-803 . 
百度学术
2. 付秀华，席佩花，孟繁有，张功，张静. 多路谷物色选光学成像系统分光器件的研究. 长春理工大学学报(自然科学版). 2022(01): 1-8 . 百度学术
3. 杜国军，王春雨，欧宗耀，王聪，胡斌. 多基准轴透射式系统装调方法. 应用光学. 2021(02): 247-254 . 本站查看
4. 吴笑男，张瑞，王志斌，陈壮壮. 基于FP微阵列的压缩感知光谱重建研究. 应用光学. 2021(03): 436-442 . 本站查看
其他类型引用(10)
计量
- 文章访问数: 128
- HTML全文浏览量: 16
- PDF下载量: 59
- 被引次数: 14
陕公网安备 61011302001501号 