Control scheme of high working temperature based on dynamic temperature control in FOG
-
摘要:
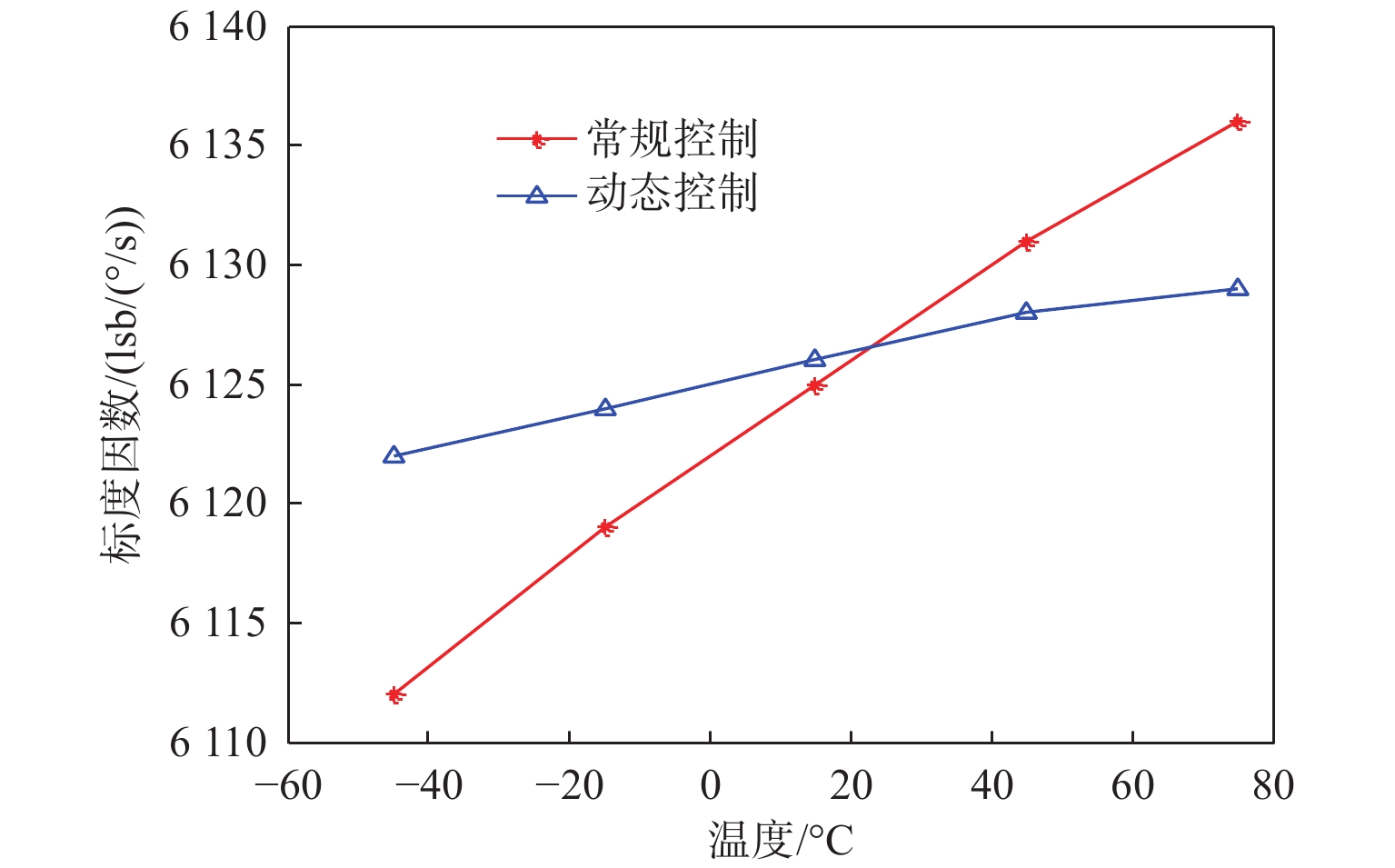
光纤陀螺工作温度的提升主要受制于光路系统，通过控制方案的优化可以提高光纤陀螺的工作温度。通过对光纤陀螺光路系统进行理论分析，提出了基于动态温控的光纤陀螺控制方案，通过试验验证了该方案的改进效果。光纤陀螺采用动态温度控制方案，一方面能够降低高温工作时的功耗，提高高温工作温度；另一方面可以降低光纤陀螺标度因数由于温度波动而引起的变化。
Abstract:The increase of working temperature of fiber-optic gyroscope (FOG) is mainly limited by the optical path system, which can be improved by optimizing the control scheme. Through the theoretical analysis of FOG optical system, the FOG control scheme based on dynamic temperature control was proposed. The improved effect of the was verified by experiments. On the one hand, the dynamic temperature control scheme of FOG can reduce the power consumption at high temperature and improve the high-temperature working temperature. On the other hand, it can reduce the change of FOG scale factor caused by the temperature fluctuations.
-
-
![]()
图 3 常规温控方案时升温条件下产品输出数据
Figure 3. Output data of gyro under conventional temperature control at rising temperature
![]()
图 4 动态温控方案时升温条件下产品输出数据
Figure 4. Output data of gyro under dynamic temperature control at rising temperature
![]()
图 5 不同控制方案下标度因数随温度变化
Figure 5. Scale factor varies with temperature under different control schemes
表 1 常规温控方案和动态温控方案试验结果对比
Table 1 Comparison of test results between conventional temperature control scheme and dynamic temperature control scheme
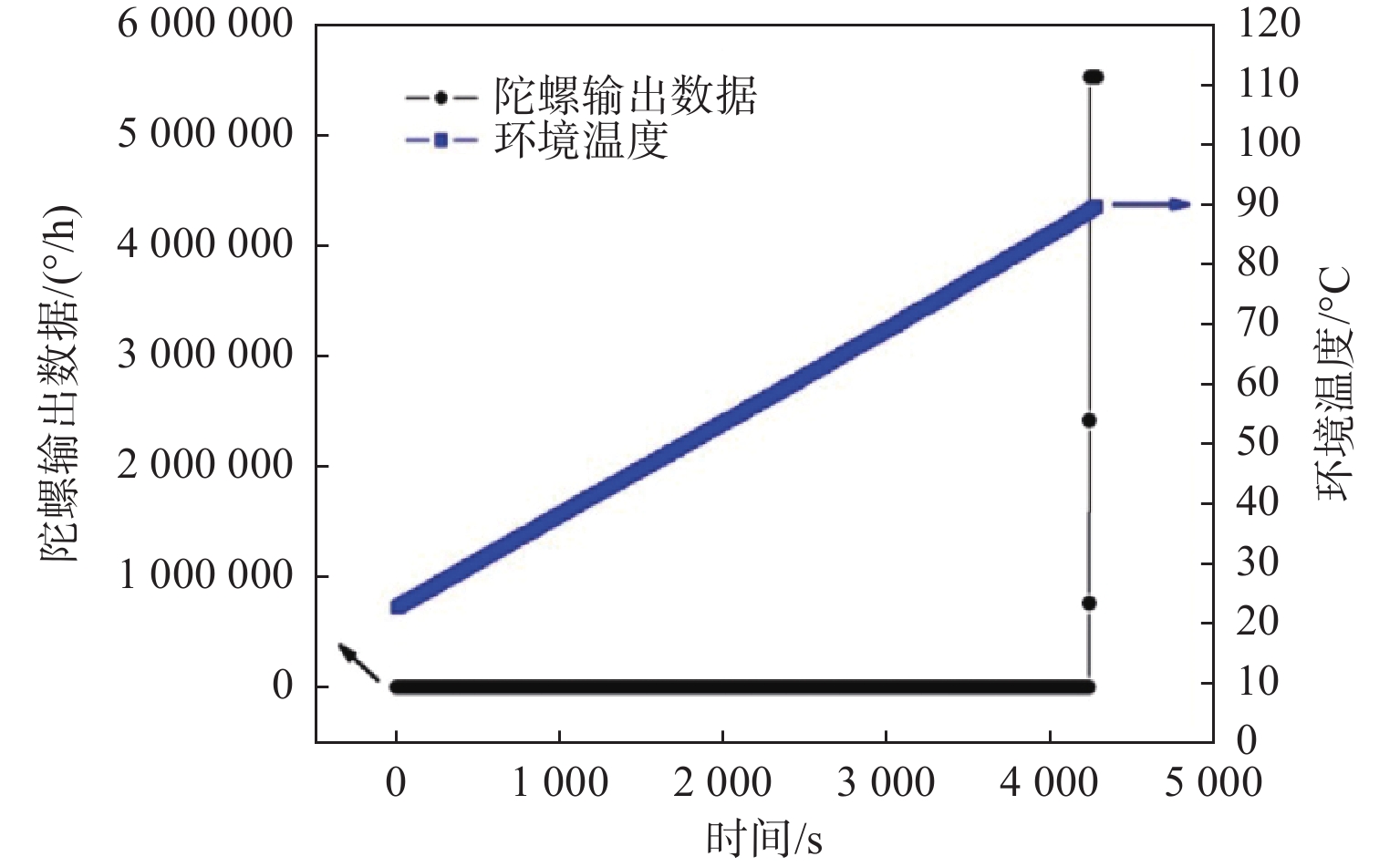
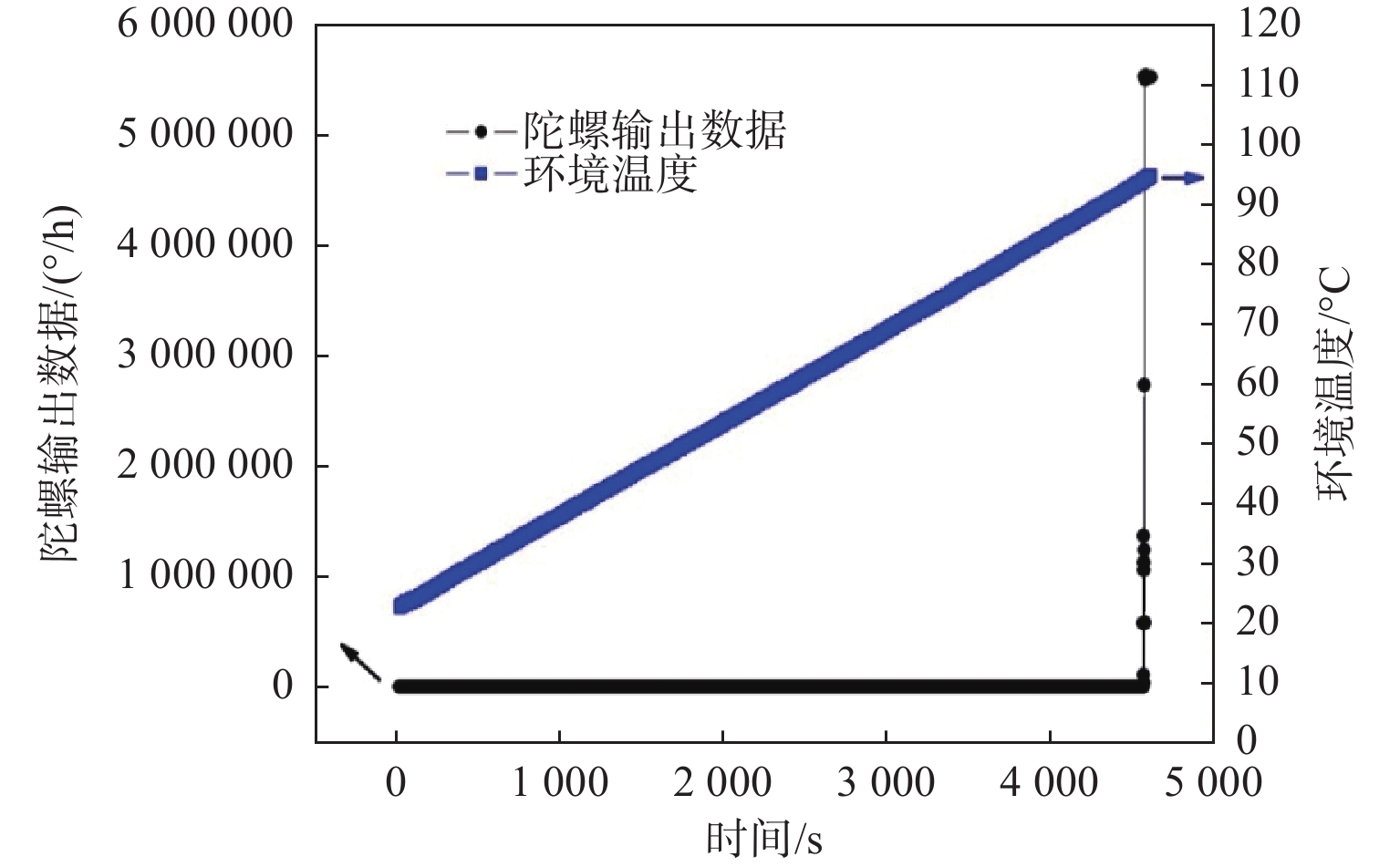
对比项目 高温75 ℃
功耗/W高温工作温度
耐受极值/℃未经补偿时标度
因数变化量常规温度控制 2.7 90.0 3 919×10−6 动态温度控制 2.4 94.8 1 143×10−6 改善 降低0.3 提高4.8 降低71% 
下载: 导出CSV
-
[1] JIN J, HE J L, SONG N F, et al. A compact four-axis interferometric fiber optic gyroscope based on multiplexing for space application[J]. Journal of Lightwave Technology,2020,38(23):6655-6663. doi: 10.1109/JLT.2020.3015713
[2] HAN S L, LUO S L, LU J Z, et al. A unified modeling approach of stochastic error in fiber optic gyro and application in INS initial alignment[J]. IEEE Sensors Journal,2020,20(13):7241-7252. doi: 10.1109/JSEN.2020.2978671
[3] ZAKIROV R, UMAROV A. Fiber optic gyroscope and accelerometer application in aircraft inertial system[C]//2020 International Conference on Information Science and Communications Technologies (ICISCT). Tashkent, Uzbekistan: IEEE, 2021: 1-3.
[4] 梁霄, 魏天啸, 谢良平, 等. 闭环光纤陀螺精度性能提升方法研究[J]. 红外技术,2019,41(9):819-823. LIANG Xiao, WEI Tianxiao, XIE Liangping, et al. Research on precision enhancement of closed-loop FOGs[J]. Infrared Technology,2019,41(9):819-823.
[5] 李彬, 谢良平, 刘卓, 等. 光纤陀螺启动过程标度因数补偿方法[J]. 中国惯性技术学报,2018,26(4):495-498. LI Bin, XIE Liangping, LIU Zhuo, et al. Compensation technique of scale factor during FOG start-up[J]. Journal of Chinese Inertial Technology,2018,26(4):495-498.
[6] 万洵, 谢良平. 光纤陀螺温度场仿真分析与陀螺外罩结构优化设计[J]. 应用光学,2016,37(3):353-358. doi: 10.5768/JAO201637.0301006 WAN Xun, XIE Liangping. Temperature field analysis and structure redesign of fiber optic gyroscope[J]. Journal of Applied Optics,2016,37(3):353-358. doi: 10.5768/JAO201637.0301006
[7] 周闻青, 费宇明, 洪桂杰, 等. 高精度光纤陀螺零位误差的磁温特性研究[J]. 应用光学,2020,41(1):220-227. doi: 10.5768/JAO202041.0108001 ZHOU Wenqing, FEI Yuming, HONG Guijie, et al. Research on magnetic temperature characteristics of zero error on high precision fiber-optic gyro[J]. Journal of Applied Optics,2020,41(1):220-227. doi: 10.5768/JAO202041.0108001
[8] 王夏霄, 冯志芳, 秦祎, 等. 光纤陀螺光纤环轴向磁敏感性研究[J]. 中国激光,2015,42(8):163-168. WANG Xiaxiao, FENG Zhifang, QIN Yi, et al. Study on the axial magnetic field sensitivity in optical fiber coil of fiber optic gyroscope[J]. Chinese Journal of Lasers,2015,42(8):163-168.
[9] KORKISHKO Y N, FEDOROV V A, PRILUTSKIY V E, et al. Highest bias stability fiber-optic gyroscope SRS-5000[C]//2017 DGON Inertial Sensors and Systems (ISS). Karlsruhe, Germany: IEEE, 2017: 1-23.
[10] LI H Z, LIN Y, LIU L, et al. Signal processing improvement of passive resonant fiber optic gyroscope using a reciprocal modulation-demodulation technique[J]. Optics Express,2020,28(12):18103. doi: 10.1364/OE.390605
[11] 张文帅, 申利梅, 涂志龙, 等. 微型层式热电模块制冷特性研究[J]. 工程热物理学报,2020,41(3):526-532. ZHANG Wenshuai, SHEN Limei, TU Zhilong, et al. Study on refrigeration characteristics of layered micro-thermoelectric module[J]. Journal of Engineering Thermophysics,2020,41(3):526-532.
[12] 谢良平, 宫晓宇, 张春熹. 使用滤波器和探测器阵列的光纤陀螺光源平均波长漂移监测[J]. 中国惯性技术学报,2019,27(6):799-803. XIE Liangping, GONG Xiaoyu, ZHANG Chunxi. Light source mean wavelength shift monitoring in fiber optic gyroscope with filter-detector array[J]. Journal of Chinese Inertial Technology,2019,27(6):799-803.
[13] LEFEVRE H C. The fiber-optic gyroscope [M]. 3rd ed. Norwood: Artech House, 2022: 23-24.
[14] 张桂才. 光纤陀螺原理与技术[M]. 北京: 国防工业出版社, 2010: 197-198. ZHANG Guicai. The principles and technologies of fiber-optic gyroscope[M]. Beijing: National Defense Industry Press, 2010: 197-198.
[15] 国防科学技术工业委员会. 光纤陀螺仪测试方法: GJB 2426A-2004[S]. 北京: 国防科工委军标出版发行部, 2004. Commission of Science, Technology and Industry for National Defense. Test method for fiber optic gyroscope. GJB 2426A-2004[S]. Beijing: Commission of Science, Technology and Industry for National Defense, 2004.
-
期刊类型引用(6)
1. 窦恩泽，杨鹏程，李小成，任拓. 改进PConvUNet图像修补的解包裹方法. 现代电子技术. 2024(05): 46-52 . 
百度学术
2. 康乐谦，杨鹏程，吕秋娟，连力平，朱新栋. Tsallis相对熵引导的包裹相位修补. 光学精密工程. 2024(08): 1130-1139 . 百度学术
3. 任拓，杨鹏程，李小成，窦恩泽，朱新栋. 激光干涉测量中数据驱动的齿轮装夹误差校正方法. 光子学报. 2024(12): 224-235 . 百度学术
4. 苏皓，刘冬冬，董太极，高彪. 基于法布里-珀罗干涉的双电机转速测量. 激光与红外. 2021(12): 1649-1653 . 百度学术
5. 李玥. 基于大数据分析技术的激光图像分类和识别研究. 激光杂志. 2020(08): 129-133 . 百度学术
6. 程志远，李治国，折文集，夏爱利. 激光相干场成像散斑噪声复合去噪方法. 物理学报. 2019(05): 154-160 . 百度学术
其他类型引用(4)
计量
- 文章访问数: 146
- HTML全文浏览量: 35
- PDF下载量: 37
- 被引次数: 10
陕公网安备 61011302001501号 