Design of high-resolution thin line structured light measurement system
-
摘要:
为实现物体表面轮廓高精度三维测量，提出一种视场、物距、测量范围均较大的远心光路测量系统。介绍了系统组成及光三角测量原理，论述了投影与成像双光路均与被测表面法线呈45 °夹角的光路搭建方式可降低较大深度测量范围对成像物镜景深的要求；建立系统深度标定理论模型，获得CCD单位像素深度理论值为0.554 4 μm；计算出放大倍率、数值孔径等光路参数并完成光路搭建；通过实验对所搭建测量系统的分辨力、测量范围、光条最小宽度及其边缘质量进行分析。实验结果表明，投影条纹最长2 mm，最小线宽可达8.96 μm，条纹边缘清晰平滑。系统成像畸变小，可实现1.62 µm的光学分辨率及0.54 µm的深度像素分辨率，工作距离65 mm，深度测量范围不低于140 µm，具有良好的测量性能。
Abstract:To achieve high-precision 3D measurement of surface profile of the object, a telecentric optical path measurement system with large field of view (FOV), object distance and measuring range was proposed. The composition of the system and the principle of optical triangulation were introduced, the construction of the optical path with the angle of 45 ° between the projection and double optical path and the normal line of the measured surface could reduce the requirement of depth of field of the imaging objective lens for large depth measurement range. The theoretical model of system depth calibration was established, and the theoretical value of CCD unit pixel depth was 0.554 4 μm. The magnification, numerical apertures and other optical parameters were calculated and the optical path was constructed. The resolution, measuring range, minimum width of light stripe and edge quality of the system were analyzed by experiments. The experimental results show that the longest projection stripe is 2 mm, the minimum line width is 8.96 μm, and the stripe edge is clear and smooth. The imaging distortion of the system is small, and the optical resolution of 1.62 µm and the depth pixel resolution of 0.54 µm can be achieved. The object distance is 65 mm, the depth measurement range is no less than 140 µm. The system has good measurement performances.
-
引言
在兵器靶场测试研究领域，对弹丸各项参数检测时能够得到弹丸清晰的飞行图像是非常重要的[1-2]。传统观测弹丸的飞行姿态有2种方法：一种是沿着外弹道方向放置多台高速CCD相机，通过分析每台摄像机单独拍摄弹丸图像，经过后续复杂的处理技术，得到较为完整的弹丸图像[1]。此方法受单个CCD相机视场范围的限制，且对多个相机所拍摄的图像进行分析时还涉及复杂的处理技术，更重要的是长距离测量时要花费很多的财力和物力[3-5]；另一种方法是将单个CCD相机系统固定在跟踪机架上，通过实时旋转CCD相机镜头来跟踪弹丸，但使用时，很难将设备用于测量小型高速飞行的弹丸。为了弥补这2种方法的缺陷，国外提出一种通过转镜和CCD相机实现对弹丸的实时跟踪技术，固定CCD相机位置，将它对准反射镜，通过拍摄反射镜中弹丸图像，以实现对高速弹丸的跟踪拍摄[6]。目前国外对此项技术的保密性非常高，国内相关产品还处于研发阶段，为了弥补我国在相关技术的空白，对于转镜跟踪测量系统的研究是非常有意义的[7]。基于高速CCD视场中点的转镜跟踪系统是通过计算机控制转镜转动来跟踪弹丸，同时弹丸的飞行姿态反射到CCD相机后，拍摄一组序列图像，从而实现对弹丸的同步跟踪[8-9]。因此，在跟踪系统中，建立弹丸运动以及转镜转动的数学模型对研究弹丸的飞行姿态有着重要的作用[10]。本文将研究基于高速CCD视场中点的转镜跟踪系统，主要包括建立弹丸和转镜的数学模型以及相关参数对系统的影响。
1 系统组成和工作原理
1.1 系统的结构组成
基于高速CCD相机视场中点的跟踪系统主要包含5个组成部分，如图 1所示。在系统能有效跟踪弹丸的视场前方设有测量弹丸速度的天幕靶，当弹丸穿过天幕靶后就能在计算机控制系统中获得弹丸的速度，此时计算机控制系统通过计算弹丸进入转镜的有效视场时间驱动伺服系统控制转镜在相应的时间段内转动。在整个跟踪过程中，转镜转动将运动弹丸的飞行姿态反射到CCD相机中，CCD相机拍摄的弹丸图像存储在计算机中，从而实现CCD相机对高速运动弹丸的同步跟踪测量。
1.2 系统的工作原理
一般情况下，转镜跟踪系统能跟踪90°视场的弹道线。跟踪系统在开始跟踪弹丸前有5°的缓冲角让反射镜预加速到一定速度，在跟踪结束时也有5°的缓冲角让反射镜停止。基于高速CCD相机视场中点的跟踪系统，工作原理图如图 2所示。当弹丸穿过天幕靶2后到达转镜-50°视场时，转镜缓慢加速转动。当弹丸到达-45°视场角时，转镜的条件满足同步跟踪弹丸的条件，系统开始同步跟踪弹丸运动。当弹丸到达+45°视场角时，反射镜开始缓慢减速，当到达转镜+50°视场时，系统停止工作，从而完成对90°转镜视场范围内弹丸的同步跟踪。
2 系统数学模型的建立
2.1 弹丸运动模型的建立
地球上任何飞行的物体都会受到地心引力和空气阻力的影响，在实际情况下弹丸发射后由于受到这2个力的作用，它不再是沿着弹道线的直线运动，而是作斜抛运动。由于弹丸的飞行速度很快，对弹丸实现实时跟踪的距离相比于整个运动过程较短，因此，在理论研究过程中，假设弹丸不受地心引力和空气阻力的影响，弹丸则沿着弹道线方向作匀速运动，且轨迹与地面平行。
假设弹丸沿弹道线方向作匀速直线运动，转镜视场中点跟踪弹丸的原理图如图 3所示。CCD相机主光轴与弹道线平行且与转镜相交于O点，OQ为相机主光轴到弹道线之间的垂直距离，记为H，垂足Q为坐标原点，规定弹丸沿弹道线运动的方向为x正方向，W点为要跟踪的弹丸，则弹丸距坐标原点的距离为|WQ|= S。CCD相机的视场角为2θ，转镜与主光轴的夹角为ϕ，OP=L1, AB段为转镜跟踪弹丸的有效范围。
由反射定理和几何关系并根据图 3可以得到弹丸运动模型：
$$ \begin{array}{*{20}{l}} {S\left( t \right) = \frac{H}{{\tan {\Phi _{\left( t \right)}}}} + } \\ {\;\;\;\;\;\;\;\;\;\;\frac{{\left( {0.5{L_1}\sin 2{\Phi _{\left( t \right)}}} \right) + H\cos {\Phi _{\left( t \right)}}{{\sin }^2}\theta }}{{\sin {\Phi _{\left( t \right)}} \times \sin \lambda \times \sin \beta }}} \end{array} $$ (1) 同时, A点坐标：
$$ {X_A} = \frac{H}{{\tan {\mathit{\Phi }_{\left( t \right)}}}} - \frac{{\left( {{L_1} + \frac{H}{{\sin {\mathit{\Phi }_{\left( t \right)}}}}} \right)\sin \theta }}{{\sin \beta }} $$ (2) B点坐标：
$$ {X_B} = \frac{H}{{\tan {{\Phi }_{\left( t \right)}}}} + \frac{{\left( {{L_1} + \frac{H}{{\sin {{\Phi }_{\left( t \right)}}}}} \right)\sin \theta }}{{\sin \lambda }} $$ (3) 由(2)、(3)式可得整个跟踪视场的范围为
$$ AB\left( t \right) = \left( {{L_1} + \frac{H}{{\sin {\mathit{\Phi }_{\left( t \right)}}}}} \right)\left( {\frac{{\sin \beta + \sin \lambda }}{{\sin \beta \sin \lambda }}} \right)\sin \theta $$ (4) (1) 式两边同时对t求导，则得出弹丸速度和转镜角速度的关系为
$$ \begin{array}{l} \frac{v}{w} = H\csc {\mathit{\Phi }_{\left( t \right)}}[ - 2\csc {\mathit{\Phi }_{\left( t \right)}} - \cot {\mathit{\Phi }_{\left( t \right)}} \cdot \\ \;\;\;\;\;\;\;\frac{{\sin \theta \left( {\sin \beta - \sin \lambda } \right)}}{{\sin \lambda \sin \beta }}] + \left( {{L_1} + H\csc {\mathit{\Phi }_{\left( t \right)}}} \right) \cdot \\ \;\;\;\;\;\;\;\frac{{\sin \theta \left( {{{\sin }^2}\lambda \cos \beta + {{\sin }^2}\beta \cos \lambda } \right)}}{{{{\sin }^2}\beta {{\sin }^2}\lambda }} \end{array} $$ (5) 式中：β=2ϕ(t)+θ；λ=2ϕ(t)-θ；Φ(t)=2ϕ(t)。
利用MATLAB对系统的数学模型进行仿真, 由于在对不同速度的弹丸进行跟踪时，系统开始工作的时间不同，则弹道宽度AB也不同，弹道宽度曲线随时间的变化曲线可由MATLAB仿真得到。当H=200 m, v=100 m/s时，转镜视场范围内的有效视场AB随时间变化的曲线如图 4所示。
![]()
图 4 转镜视场范围内有效跟踪范围随时间变化曲线Figure 4. Curves of effective tracking range over time within rotation mirror FOV根据图 4可以看出A、B点的坐标随着时间的变化而变化，系统能有效跟踪弹丸的范围也在随时间变化，它的曲线呈开口向上的抛物线，当弹丸到达转镜正上方时有效跟踪范围最小，且最小值约为14 m，远大于被观测高速目标的尺寸，可以满足系统跟踪弹丸的视场要求。
2.2 扫描速率的大小
扫描速率R定义为被测弹丸速度v和相机主光轴到弹道线的垂直距离H的比值，它可以表示为
$$ R = \frac{v}{H} $$ (6) 对于不同速度的弹丸，不同的相机位置，只要保证它们的比值相同，那么转镜的扫描速率都是相同的，可以采用同一扫描速率。联立(5)式和(6)式，可以得出在ϕ=45°时，转镜的最大角速度ωmax和R之间的关系为
$$ {\omega _{\max }} = \left| { - \frac{v}{{2H}}} \right| = \frac{R}{2} $$ (7) 由(7)式可知，转镜的最大角速度仅跟扫描速率相关。
在跟踪系统中，扫描速率R是有一定范围的，它跟CCD相机所能接收物体像大小的能力有关。它的最大值与电机的转速有关，最小值与CCD的视场范围有关。在对弹丸的跟踪过程中，选择合适的扫描曲线控制转镜转动，在CCD中对连续弹丸图像的成像效果有着极大的提高。
3 设置参数对跟踪效果影响
根据(1)式可知，同一个跟踪系统在跟踪弹丸时(即相机视场角和转镜转动角度相同)，CCD相机、转镜的所处位置对整个系统的跟踪效果有影响，也就是转镜到弹道线的垂直距离OQ(H)和CCD相机到转镜的距离OP(L1)对跟踪视场有影响。
3.1 弹道线到转镜的垂直距离H
根据(1)和(4)式可知H越大，能有效跟踪弹丸的范围就越大。如果想得到弹丸长时间的飞行轨迹，可以通过一直增大H来实现，但是转镜转动和相机的视场角都是有限制的，H不会一直增大。
对于图 3，现在假设相机的焦距为f，弹丸长度为d，弹丸成像在CCD上的长度为L, CCD的光敏面尺寸为l×ħ。根据物像关系可知：
$$ \frac{d}{L} = \frac{{{L_1} + H}}{f} $$ (8) 为了保证在跟踪过程中弹丸的像不脱离CCD的光敏面，一般要求弹丸长度占CCD光敏面长度的1/5~4/5，由此关系和(8)式可以得到转镜和相机的位置关系为
$$ \frac{{5df}}{l} - {L_1} \le H \le \frac{{5df}}{{4l}} - {L_1} $$ (9) 由(9)式可知：已知相机参数和弹丸长度，通过调整转镜到弹道线的距离H和相机到转镜的距离L1就可以使弹丸清晰成像。
3.2 最大离散速度的间隔值
假设天幕靶测得弹丸的速度为v0，CCD视场大小为l×ħ，弹丸的长度为d，在必须考虑地球引力对弹丸的影响时，弹丸脱离CCD视场范围的情况有2种，其示意图如图 5所示。
如图 5所示，曲线1是弹丸在水平方向上发生偏离的情况，曲线2是弹丸在竖直方向上发生偏离的情况。通过计算得到弹丸在水平方向上的离散速度为
$$ \Delta v = \frac{{\left( {3l + 5d} \right){v_0}}}{{5S}} $$ (10) 弹丸在竖直方向上的离散速度为
$$ \Delta v = \frac{ħ}{{\sqrt {\frac{{8\left( {{H_1} - H} \right)}}{8}} }} $$ (11) 式中：S为弹丸在CCD视场内飞行的距离；H1为弹丸偏移后距离相机主光轴的垂直距离。
4 系统跟踪效果误差分析
基于高速CCD相机视场中点跟踪弹丸的转镜系统，在弹道线方向跟踪弹丸时(图 3所示)，取L=300 mm, H=200 m, θ=2°，利用MATLAB得到的跟踪效果表如表 1所示。
表 1 相机中点跟踪效果表Table 1. Camera midpoint tracking effect table时间T/s 转镜角度/(°) 视场前点B/m 视场后点A/m 理论跟踪点/m 实际跟踪点/m Δ/m 2.0 66.5203 -174.0924 -200.2985 -187.1954 -187.1924 -0.003 2.5 63.1024 -135.9317 -157.4249 -146.6783 -146.6763 -0.002 3.0 57.8523 -87.8016 -105.0347 -96.4182 -96.4160 -0.0022 3.5 53.0235 -50.0291 -65.1764 -57.6028 -57.6012 -0.0016 4.0 45.0000 6.9946 -6.9946 0 0.002 -0.002 4.5 38.8521 50.9746 36.3204 43.6484 43.6463 0.0021 5.0 32.1578 104.9447 87.7175 96.3311 96.3283 0.0028 5.5 27.0357 155.8694 134.5268 145.1981 145.1956 0.0025 6.0 22.5278 214.0722 186.1262 200.0992 200.0962 0.003 由(1)式可知：在相机和电机的参数相同的条件下(即相机视场角和转镜的转动角度相同)，弹丸的位置只跟相机主光轴到弹道线的垂直距离H和OP的大小相关。弹丸的跟踪点坐标是按照(1)式的函数关系计算出的，根据函数的系统误差公式可以得到弹丸坐标的系统误差公式为
$$ \begin{array}{l} \Delta {S_{\left( t \right)}} = \left( {\frac{1}{{{\rm{tan}}{\mathit{\Phi }_{\left( t \right)}}}} + \frac{{\cos {\mathit{\Phi }_{\left( t \right)}} \times {{\sin }^2}\theta }}{{\sin {\mathit{\Phi }_{\left( t \right)}} \times \sin \lambda \times \sin \beta }}} \right)\Delta H + \\ \;\;\;\;\;\;\;\;\;\;\;\left( {\frac{{0.5\sin 2{\mathit{\Phi }_{\left( t \right)}} \times {{\sin }^2}\theta }}{{\sin {\mathit{\Phi }_{\left( t \right)}} \times \sin \lambda \times \sin \beta }}} \right)\Delta {L_1} \end{array} $$ (12) 已知ΔH=0.003 m, ΔL1=0.001 m，利用MATLAB得到弹丸坐标的系统误差随时间变化的曲线如图 6所示。
由上图看出系统的误差范围在±0.003 m的范围内变化，结合表 1所示的Δ值可知，测量误差在系统误差的范围内。也就是说，基于高速CCD相机视场中点的跟踪系统可以实现对弹丸的实时跟踪性能。
5 结论
通过建立高速CCD相机视场中点的转镜弹道跟踪系统的数学模型，并对其运动曲线进行了仿真，得出了能有效跟踪弹丸的视场范围。分析了主要参数转镜到弹道线的距离H和转镜到相机的距离L1对跟踪效果的影响，计算了扫描速率和速度的最大离散间隔值以及它们对成像效果造成的影响，进一步得到了转镜和相机空间布置的关系。在理想条件下，当弹丸飞行速度为100 m/s，转镜到弹道线的垂直距离H为200 m时，分析了系统存在的误差，结果证明该系统可以实现对弹丸的同步跟踪。
-
![]()
图 5 第7组元素1灰度对比度随拍摄物距变化曲线
Figure 5. Variation curves of gray contrast of group 7 element 1 with object distance
![]()
图 6 成像系统拍摄分辨率靶所得图片
Figure 6. Image obtained from resolution target taken by imaging system
![]()
图 8 视场不同区域拍摄包含高度差信息的条纹图
Figure 8. Fringe patterns containing height difference information taken in different areas of field of view
表 1 第7组各元素成像对比度数据表
Table 1 Imaging contrast data for each element in group 7
元素序号 分辨
率/µm解析
力/lp/mm水平条
纹对比度竖直条
纹对比度平均
对比度7.1 3.91 128.00 0.405 0.538 0.452 7.2 3.48 144.00 0.430 0.596 0.497 7.3 3.10 161.00 0.440 0.617 0.512 7.4 2.76 181.00 0.475 0.639 0.538 7.5 2.46 203.00 0.512 0.662 0.569 7.6 2.19 228.00 0.525 0.689 0.596 
下载: 导出CSV
表 2 CCD单位像素深度计算数据表
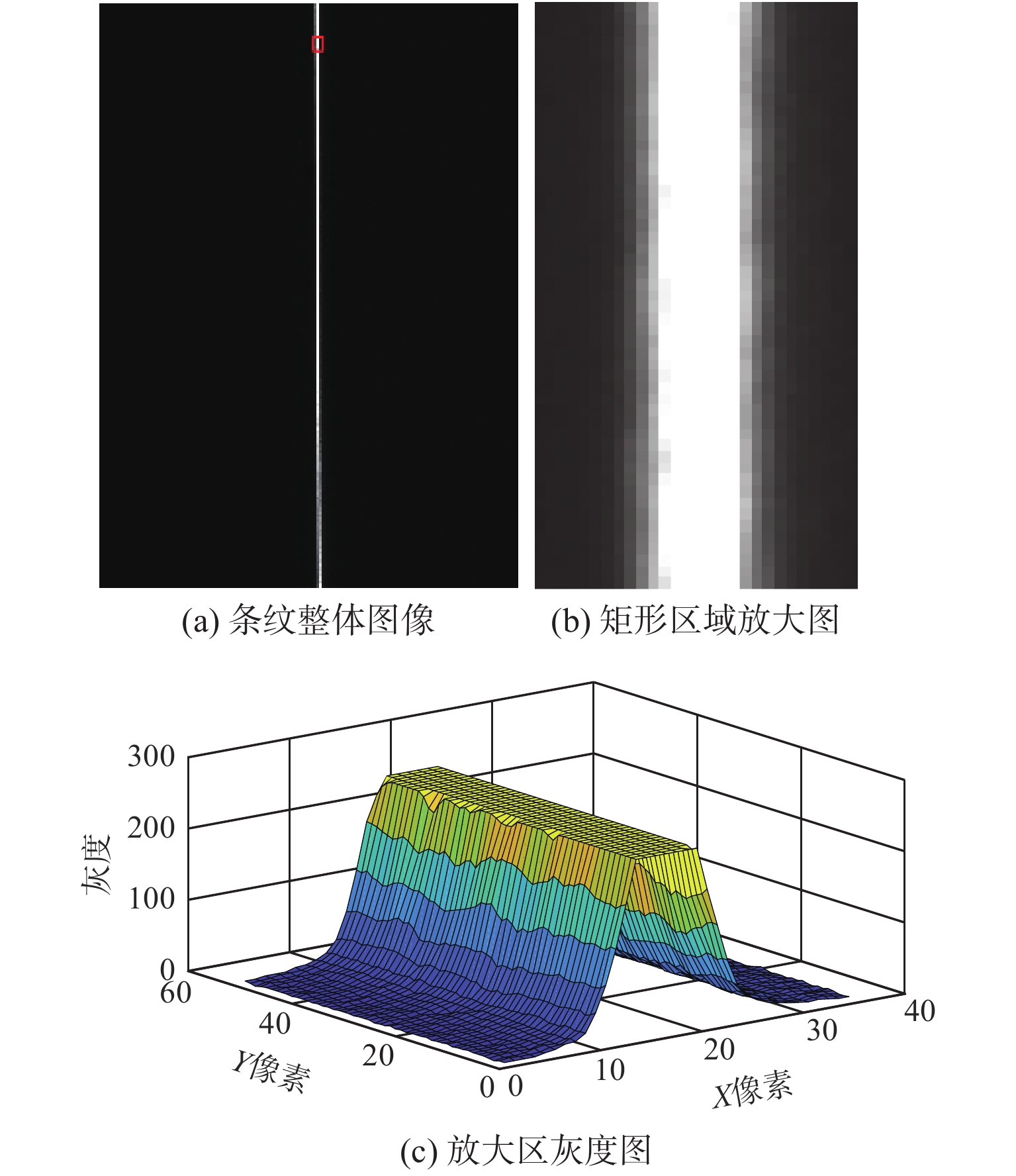
Table 2 Data of computed CCD unit pixel depth
视场序号 1 2 3 4 5 均值 条纹间距占像素数 955.4 955.1 955.3 955.4 955.2 955.3 单位像素深度/µm 0.544 3 0.544 4 0.544 3 0.544 3 0.544 4 0.544 3
下载: 导出CSV
表 3 条纹宽度随投影距离变化情况
Table 3 Variation of fringe width with projection distance
位置读数/mm 条纹宽度/µm 条纹宽度变化率/% 10.62 13.05 45.61 10.64 11.63 29.82 10.66 11.32 26.32 10.68 10.53 17.54 10.70 9.59 7.02 10.72 9.43 5.26 10.74 8.96 0.00 10.76 8.96 0.00 10.78 9.12 1.75 10.80 9.27 3.51 10.82 9.43 5.26 10.84 9.43 5.26 10.86 10.69 19.30 10.88 11.79 31.58
下载: 导出CSV
-
[1] 王颖, 张瑞, 张圆. 基于圆结构光视觉传感器的标定特征点获取方法[J]. 应用光学,2012,33(5):884-888. WANG Ying, ZHANG Rui, ZHANG Yuan. Constructing method of calibration feature points used for circle structure light vision sensor[J]. Journal of Applied Optics,2012,33(5):884-888.
[2] 符钦伟, 杜宣, 张燕, 等. 半透明胶条表面光条中心线提取[J]. 光学精密工程,2021,29(4):701-709. doi: 10.37188/OPE.20212904.0701 FU Qinwei, DU Xuan, ZHANG Yan, et al. Extraction of laser stripe center on translucent glue strip surface[J]. Optics and Precision Engineering,2021,29(4):701-709. doi: 10.37188/OPE.20212904.0701
[3] XU Xiaobin, FEI Zhongwen, YANG Jian, et al. Line structured light calibration method and centerline extraction: a review[J]. Results in Physics,2020,19(1):103637.
[4] LYU Shenzhen, SUN Qiang, ZHANG Yuyuan, et al. Projector distortion correction in 3D shape measurement using a structured-light system by deep neural networks[J]. Optics Letters,2020,45(1):204-207. doi: 10.1364/OL.45.000204
[5] 朱统晶, 周平, 刘欣冉, 等. 结构光三维测量系统标定的关键算法研究[J]. 应用光学,2014,35(5):848-852. ZHU Tongjing, ZHOU Ping, LIU Xinran, et al. Crucial algorithms for structural light 3D measurement system calibration[J]. Journal of Applied Optics,2014,35(5):848-852.
[6] 王永红, 张倩, 胡寅, 等. 显微条纹投影小视场三维表面成像技术综述[J]. 中国光学,2021,14(3):447-457. doi: 10.37188/CO.2020-0199 WANG Yonghong, ZHANG Qian, HU Yin, et al. 3D small-field surface imaging based on microscopic fringe projection profilometry: a review[J]. Chinese Optics,2021,14(3):447-457. doi: 10.37188/CO.2020-0199
[7] 李文涛, 王培俊, 唐晓敏. 钢轨磨损视觉测量的轮廓精确快速提取[J]. 光学精密工程,2018,26(1):238-244. doi: 10.3788/OPE.20182601.0238 LI Wentao, WANG Peijun, TANG Xiaomin. Accurate and rapid contour extraction of visual measurement for rail wear[J]. Optics and Precision Engineering,2018,26(1):238-244. doi: 10.3788/OPE.20182601.0238
[8] GUO Xiaozhong, SHI Zhaoyao, YU Bo, et al. 3D measurement of gears based on a line structured light sensor[J]. Precision Engineering,2020,61:160-169. doi: 10.1016/j.precisioneng.2019.10.013
[9] WU Xiaojun, TANG Na, LIU Bo, et al. A novel high precise laser 3D profile scanning method with flexible calibration[J]. Optics and Lasers in Engineering,2020,132(3):105938.
[10] JOSÉ S, AMALIA G, JUAN R, et al. LED source interferometer for microscopic fringe projection profilometry using a gates' interferometer configuration[J]. Optics and Lasers in Engineering,2022,149(2):106822.
[11] KUMAR V, DUBEY A K, GUPTA M, et al. Speckle noise reduction strategies in laser-based projection imaging, fluorescence microscopy, and digital holography with uniform illumination, improved image sharpness, and resolution[J]. Optics & Laser Technology,2021,141(9):107079.
[12] 夏灵林. 激光毛化冷轧辊表面三维形貌测量及其评价方法研究[D]. 武汉: 华中科技大学, 2012. XIA Linglin. Measurement and evaluation for surface topography of laser roughen steel rolls [D]. Wuhan: Huazhong University of Science and Technology, 2012.
[13] CHEN K P, SHI T L, LIU Q, et al. Microscopic three-dimensional measurement based on telecentric stereo and speckle projection methods[J]. Sensors (Basel, Switzerland),2018,18(11):3882. doi: 10.3390/s18113882
[14] ZHANG J, CHEN X, XI J, et al. Paraxial analysis of double-sided telecentric zoom lenses with four components[J]. Optical Engineering,2014,53(11):115103. doi: 10.1117/1.OE.53.11.115103
[15] SHANG Z Y, WANG J H, ZHAO L, et al. a Measurement of gear tooth profiles using incoherent line structured light[J]. Measurement,2022,189(15):110450.
[16] PEI Yongchen, XIE Hailiang, TAN Qingchang. A non-contact high precision measuring method for the radial runout of cylindrical gear tooth profile[J]. Mechanical Systems and Signal Processing,2020,138(7):106543.
[17] YAN Z H, HU F T, FANG J, et al. Multi-line laser structured light fast visual positioning system with assist of TOF and CAD[J]. Optik,2022,269(11):169923.
[18] 王乐, 方玥, 王胜春, 等. 基于Zemax的钢轨轮廓测量系统仿真模型研究[J]. 中国激光,2021,48(16):191-103. WANG Le, FANG Yue, WANG Shengchun, et al. Research on simulation model of rail contour measurement system based on Zemax[J]. Chinese Journal of Lasers,2021,48(16):191-103.
[19] WANG Y Z, CHEN J Q, PENG Y F. Surface reconstruction of microscale objects based on grid-patterned structured-light measurements[J]. Microscopy and Microanalysis,2022,28(1):152-172. doi: 10.1017/S1431927621013829
[20] 姚敏. 基于线结构光的视频内窥镜测量技术[J]. 应用光学,2016,37(1):91-95. doi: 10.5768/JAO201637.0103004 YAO Min. Measurement method for video probe based on line-structured light[J]. Journal of Applied Optics,2016,37(1):91-95. doi: 10.5768/JAO201637.0103004
[21] LIU J H, WANG Y Z. 3D surface reconstruction of small height object based on thin structured light scanning[J]. Micron,2021,143:103022. doi: 10.1016/j.micron.2021.103022
[22] WANG Y, GENG B, LONG C. Contour extraction of a laser stripe located on a microscope image from a stereo light microscope[J]. Microscopy Research and Technique,2019,82(3):260-271. doi: 10.1002/jemt.23168
[23] HOLST G C. Imaging system performance based upon Fλ/d[J]. Optical Engineering,2007,46(10):103204. doi: 10.1117/1.2790066
[24] 汤东亮. 基于超振荡光场的远场超分辨成像原理和方法研究[D]. 成都: 中国科学院研究生院(光电技术研究所), 2016. TANG Dongliang. Investigation of far-field super-resolution imaging method based on super-oscillatory phenomenon[D]. Chengdu: University of Chinese Academy of Sciences (The Institute of Optics and Electronics).
-
期刊类型引用(1)
1. 戴宗武，张少甫，刘乃强，胡月，王喆，段岑薇，孙宇哲. 用于自主导航的SAR/多普勒雷达一体化技术设计. 兵工学报. 2022(12): 3093-3102 . 
百度学术
其他类型引用(1)
计量
- 文章访问数: 191
- HTML全文浏览量: 54
- PDF下载量: 65
- 被引次数: 2
陕公网安备 61011302001501号 