New defect characterization mass map in fringe reflection method
-
摘要:
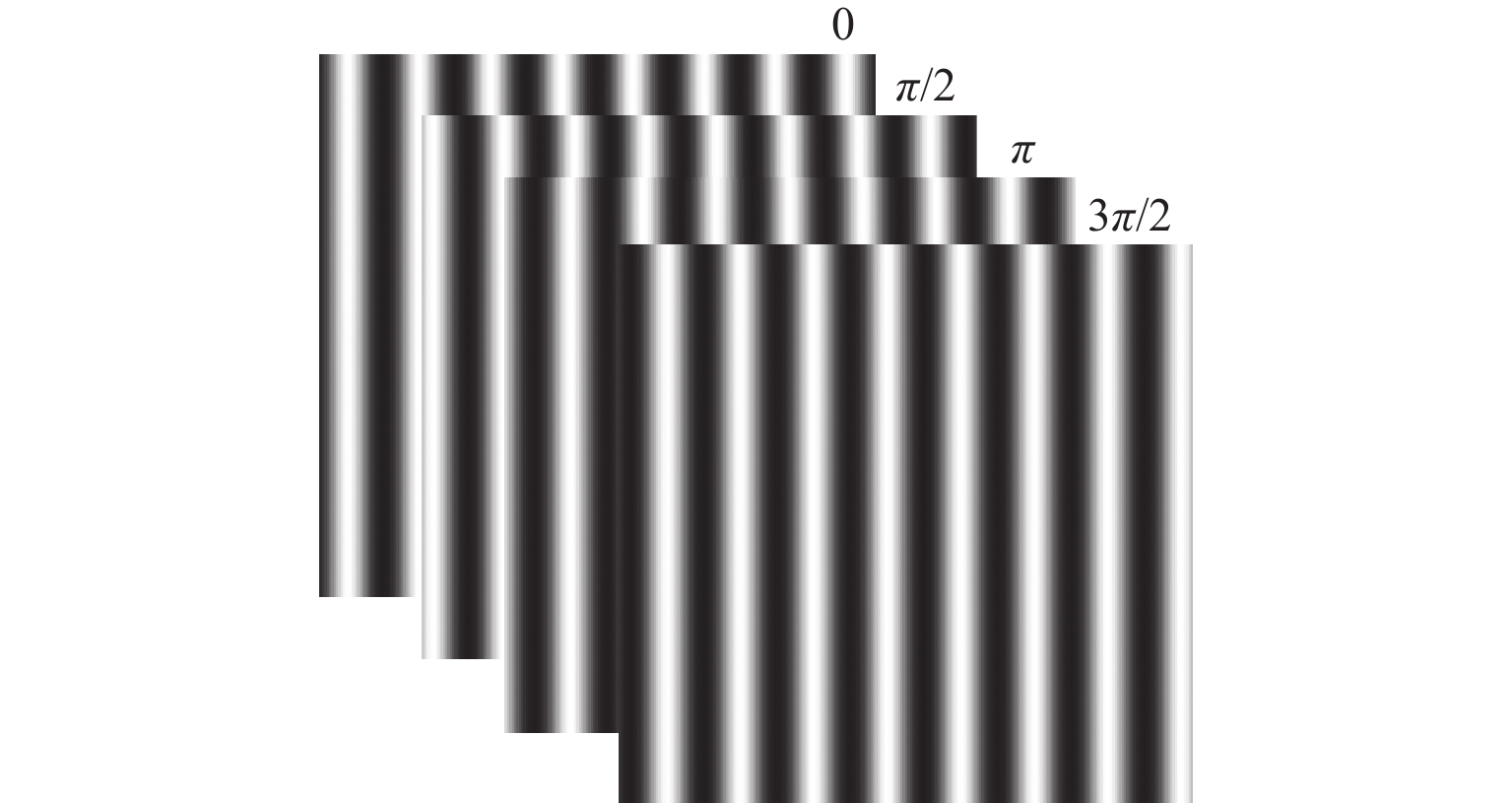
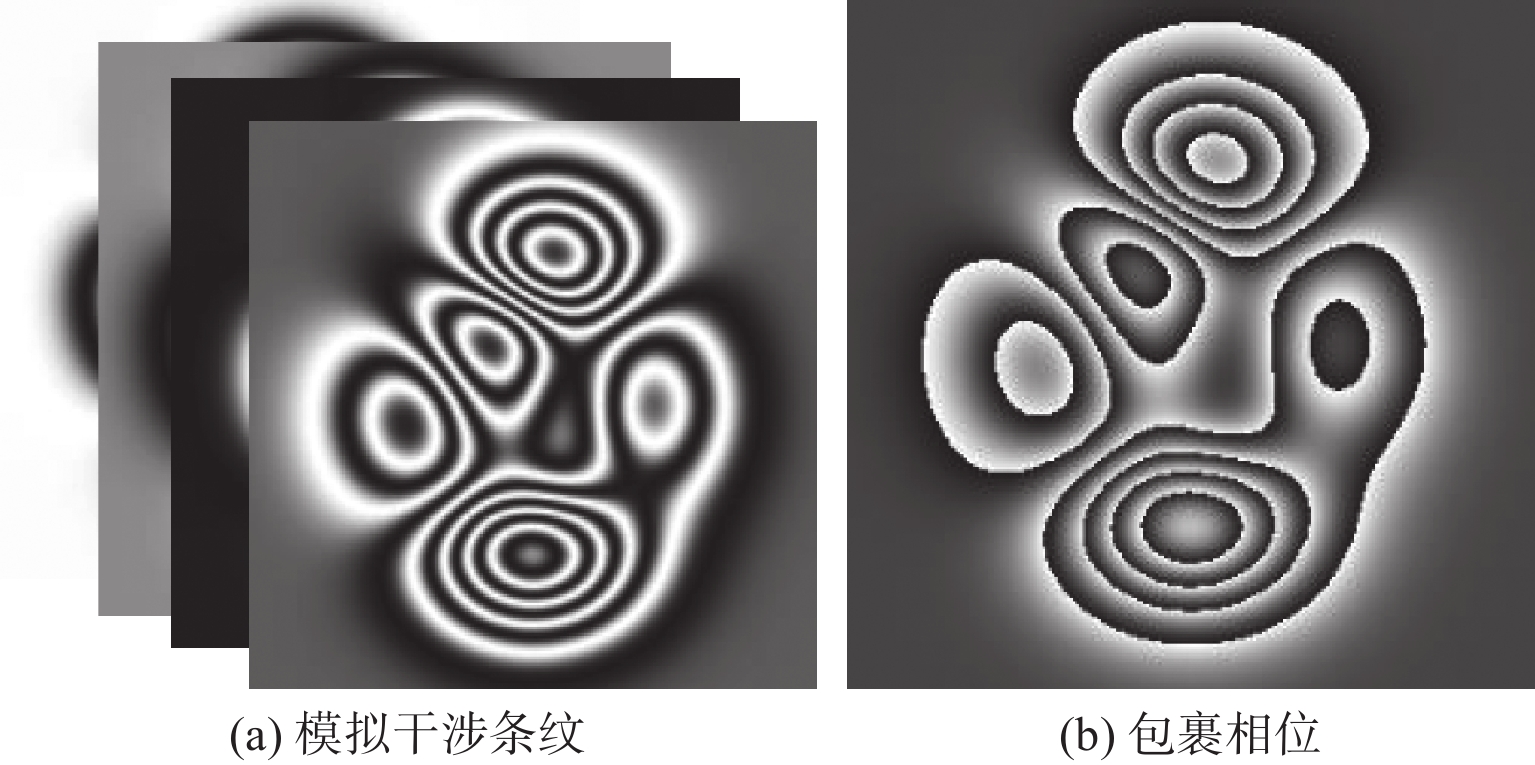
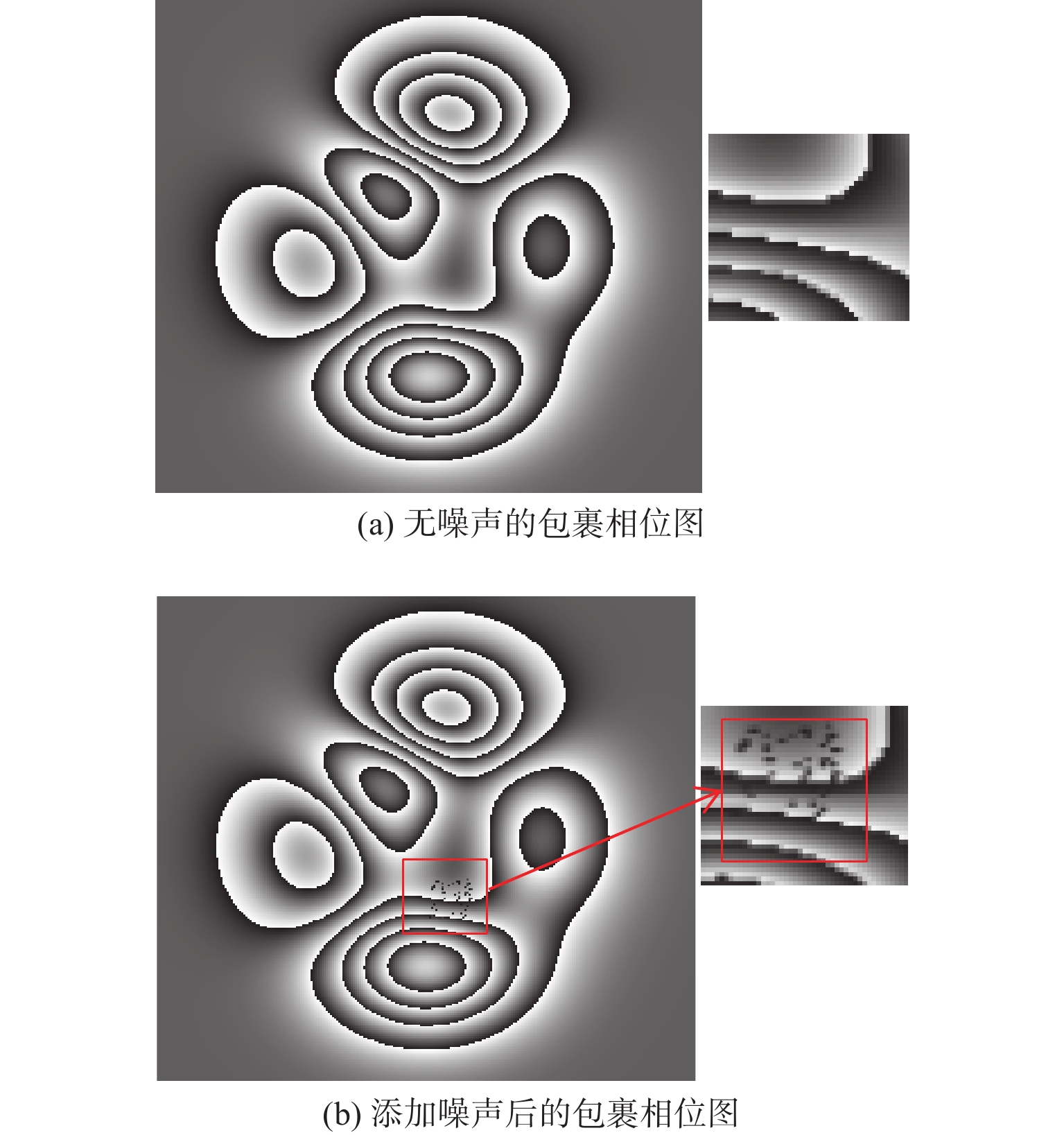
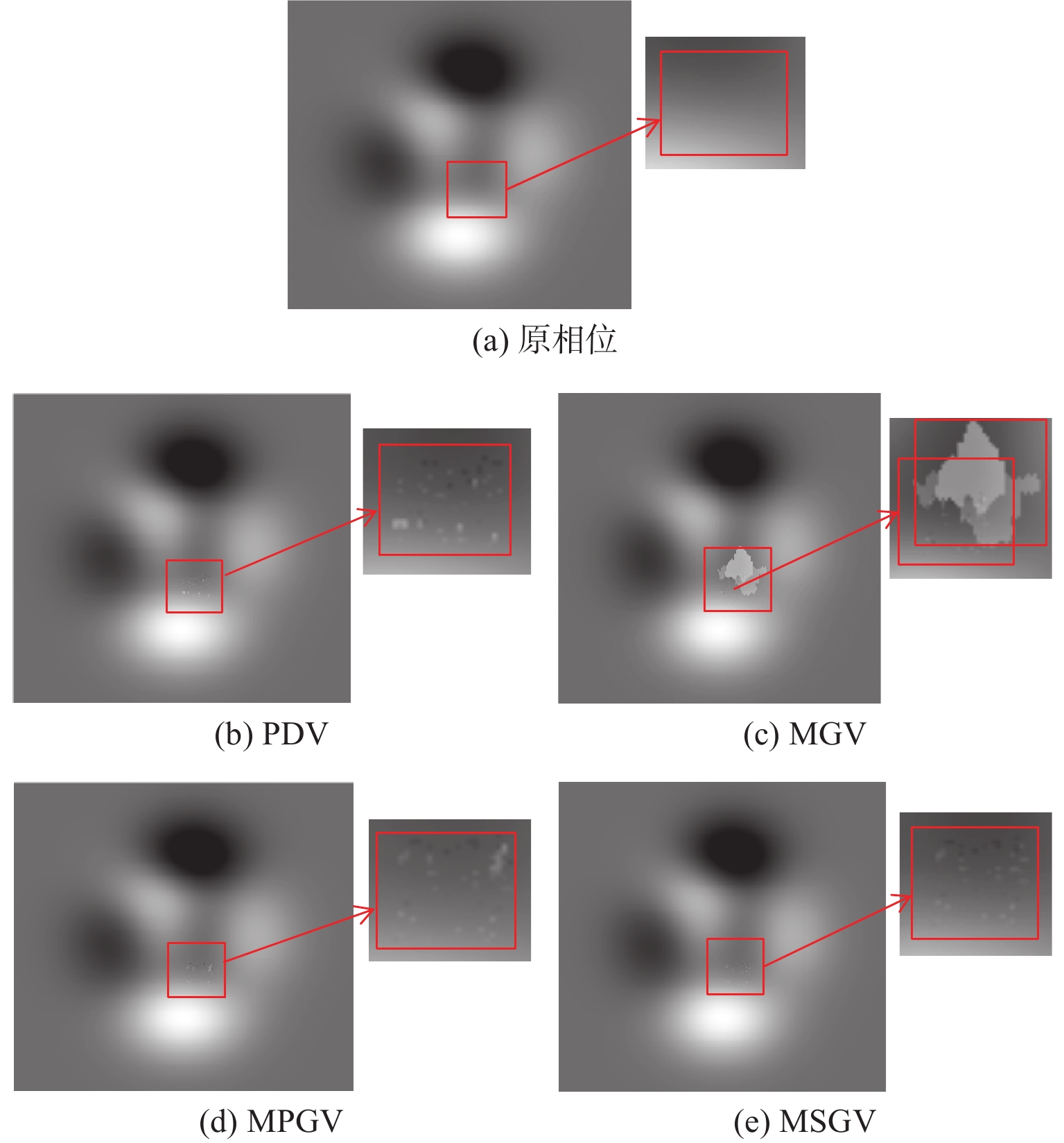
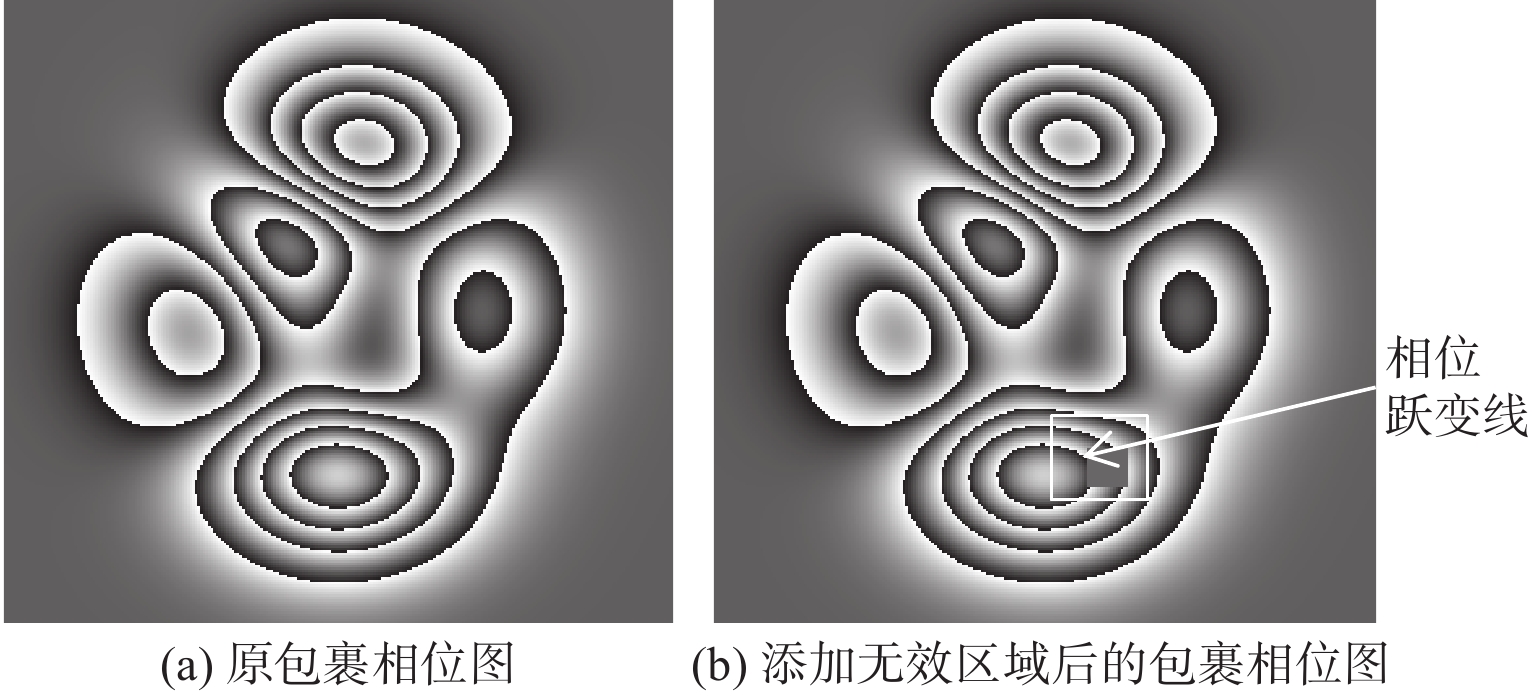
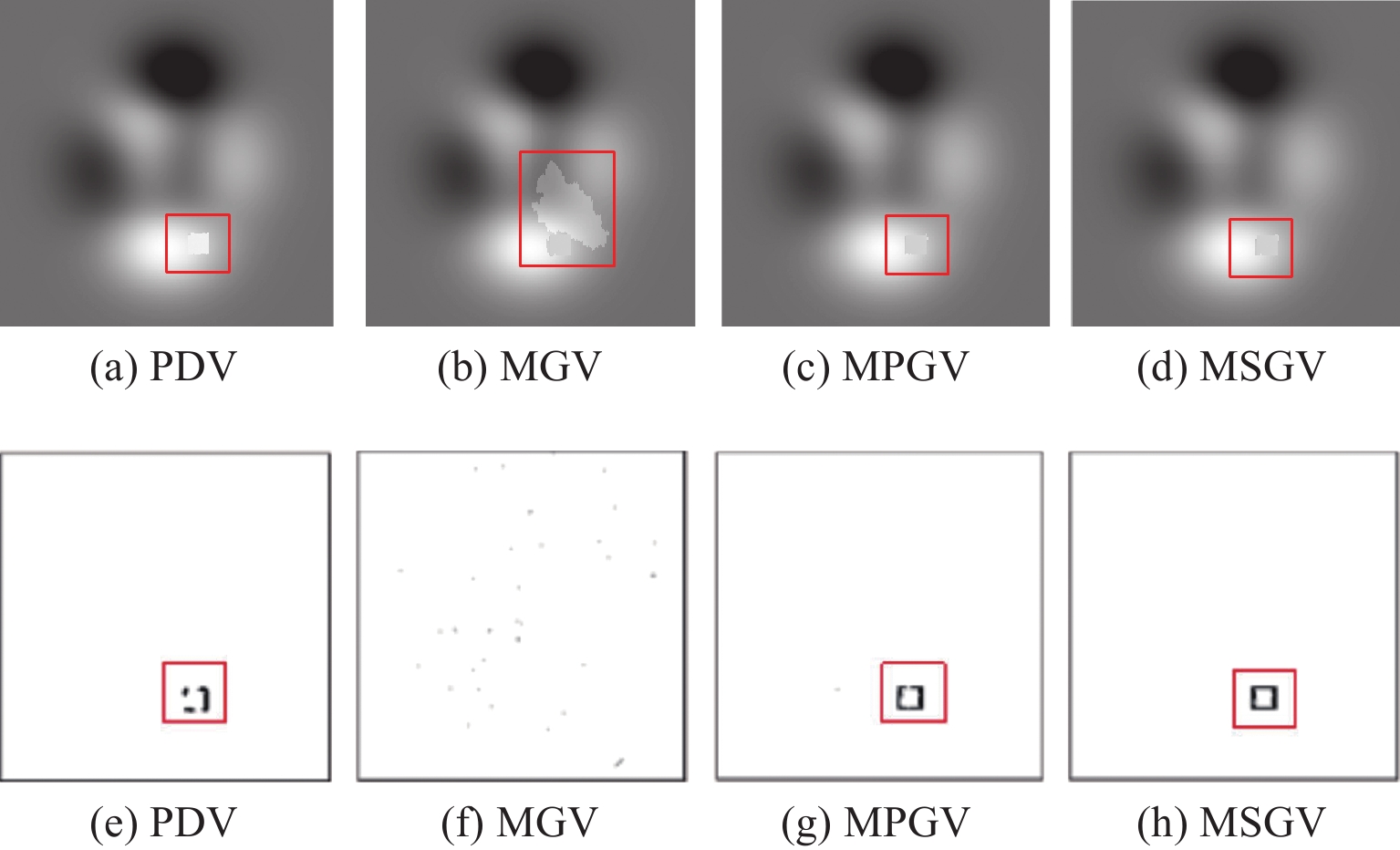
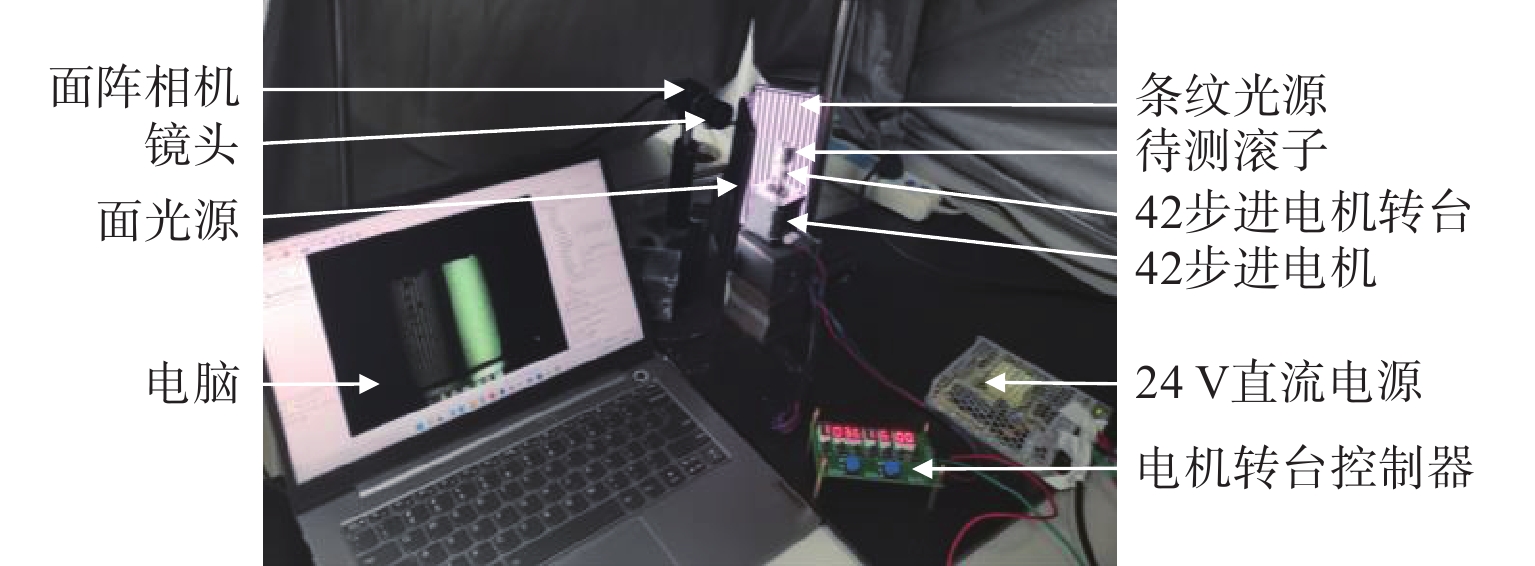
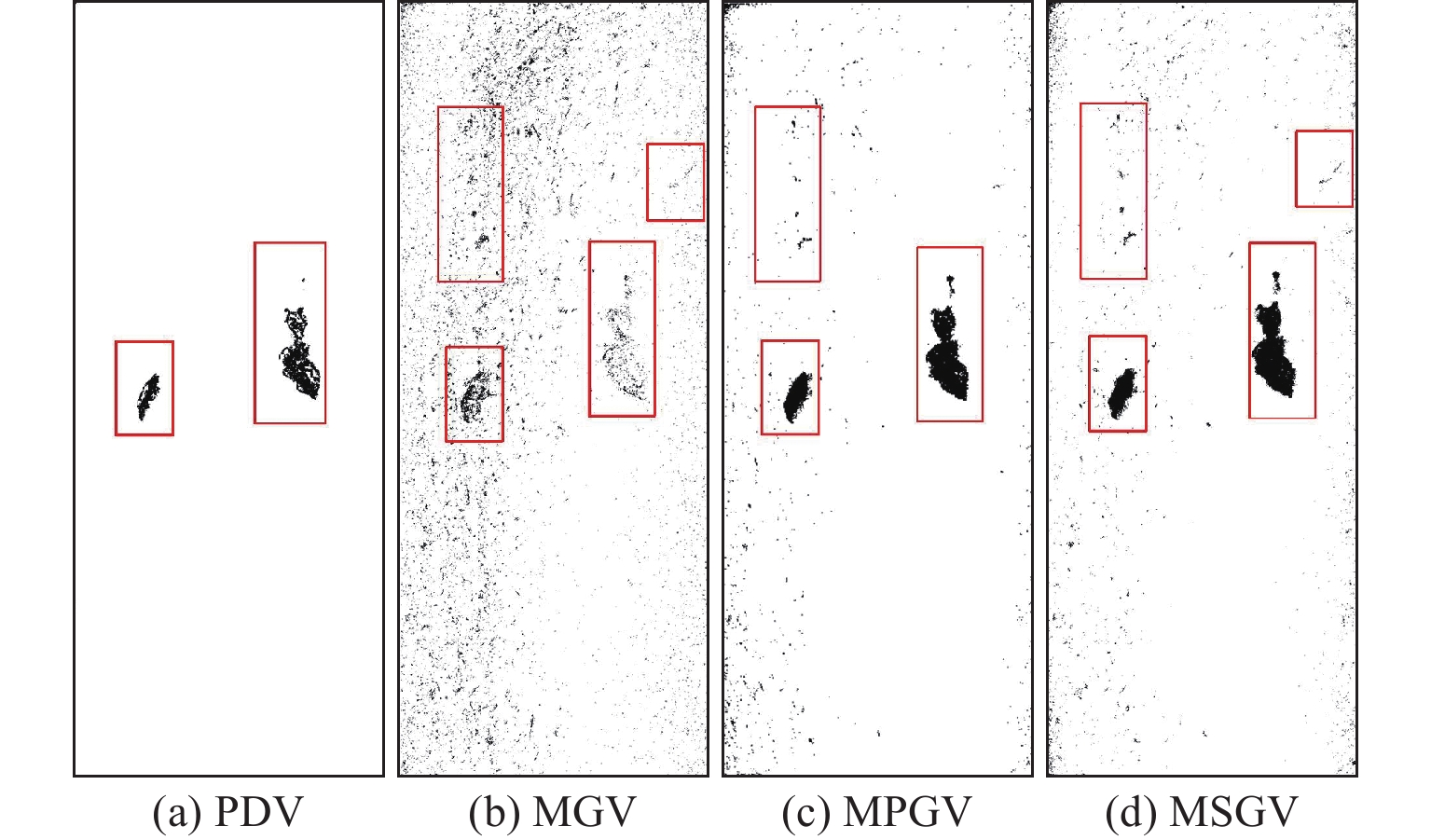
使用相移法测量物体形貌时，质量导引法在相位解包裹中起着重要作用。在检测高反光待测物表面缺陷时，相移条纹调制度常用来识别表面缺陷。本文通过将Sobel梯度与条纹调制相结合，提出了新的缺陷表征质量图，即调制度-Sobel梯度偏差质量图（modulation-sobel gradient variance，MSGV）。将解包裹相位图和原始相位图的均方根误差、峰值信噪比、信噪比3个性能作数据对比，对相位解包裹数据进行模拟测试。结果显示，MSGV的平均峰值信噪比较调制度-相位梯度偏差质量图（modulation-phase gradient variance，MPGV）提升了约1.97%，均方根误差减小了约2.91%。表明本文提出的MSGV算法相对于传统算法有一定优越性，具有更高的抗噪性和更低的解包裹误差。将所提出的方法对鼓型滚子曲面进行缺陷检测与定位，结果表明，MSGV能更好地显示出缺陷的轮廓信息。
Abstract:In the process of measuring the topography of objects by the phase-shift method, the mass-guided method plays an important role in the phase unwrapping. In the detection of surface defects of high-reflective object, the phase-shift fringe modulation is often used to identify surface defects. A new defect characterization mass map was proposed by combining Sobel gradients with fringe modulation, namely modulation-sobel gradient variance (MSGV) mass map. The root-mean-square error, peak signal-to-noise ratio, and signal-to-noise ratio of the unwrapped phase map and the original phase map were compared to simulate and test the phase unwrapped data. The results show that the average peak signal-to-noise ratio of MSGV is improved by about 1.97% compared to the modulation-phase gradient variance (MPGV) mass map, and the root-mean-square error is reduced by about 2.91%, which indicates that the proposed MSGV algorithm has certain advantages compared with the traditional algorithms, with higher noise immunity and lower unwrapping error. The proposed method was used to detect and locate the defects on the surface of the drum roller. The results show that the MSGV can better display the contour information of defects.
-
Keywords:
- phase-shift fringes /
- mass map /
- modulation degree /
- defect detection
-
-
![]()
图 2 模拟干涉条纹图和包裹相位图
Figure 2. Simulated interference fringe pattern and wrapped phase image
![]()
图 5 添加无效区域前后的包裹相位图
Figure 5. Wrapped phase images before and after adding invalid regions
表 1 四种相位展开算法的性能参数对比
Table 1 Comparison of performance parameters of four phase-unwrapping algorithms
PDV MGV MPGV MSGV SNR 6.4643 6.0755 37.2173 38.2827 PSNR 23.2293 22.8405 53.9823 55.0476 RMSE 3.0913 3.3809 0.2095 0.2034 
下载: 导出CSV
-
[1] 宋一平. 基于条纹反射的手机玻璃盖板三维面形检测方法研究[D]. 成都: 电子科技大学, 2019. SONG Yiping. Research on three dimensional shape measurement method of mobile phone glass panel based on fringe reflection[D]. Chengdu: University of Electronic Science and Technology of China, 2019.
[2] 岳慧敏. 基于时间相位展开的三维轮廓测量研究[D]. 成都: 四川大学, 2005. YUE Huimin. Research on three-dimensional profilometry based on temporal phase unwrapping[D]. Chengdu: Sichuan University, 2005.
[3] 苏显渝, 苏礼坤, 李万松. 基于傅里叶变换的调制度测量轮廓术[J]. 激光与光电子学进展,1999,36(增刊1):128-131. SU Xianyu, SU Likun, LI Wansong. Modulation measurement profilometry based on Fourier transform[J]. Laser and Optronics Progress,1999,36(S1):128-131.
[4] 宋雷. 基于条纹投影和条纹反射的三维形貌测量[D]. 成都: 电子科技大学, 2012. SONG Lei. Three-dimensional shape measurement based on fringe projection and fringe reflection[D]. Chengdu: University of Electronic Science and Technology of China, 2012.
[5] FLYNN T J. Consistent 2-D phase unwrapping guided by a quality map[C]//IEEE International Symposium on Geoscience and Remote Sensing (IGARSS). Lincolnshire: IEEE, 2002: 2057-2059.
[6] JUDGE T R, BRYANSTON-CROSS P J. A review of phase unwrapping techniques in fringe analysis[J]. Optics and Lasers in Engineering,1994,21(4):199-239. doi: 10.1016/0143-8166(94)90073-6
[7] 王霖, 韩旭, 伏燕军, 等. 用于三维测量的快速相位解包裹算法[J]. 应用光学,2019,40(2):271-277. WANG Lin, HAN Xu, FU Yanjun, et al. Fast phase unwrapping algorithm for 3D measurement[J]. Journal of Applied Optics,2019,40(2):271-277.
[8] ZHENG D L, DA F P. A novel algorithm for branch cut phase unwrapping[J]. Optics and Lasers in Engineering,2011,49(5):609-617. doi: 10.1016/j.optlaseng.2011.01.017
[9] GOLDSTEIN R M, ZEBKER H A, WERNER C L. Satellite radar interferometry: two-dimensional phase unwrapping[J]. Radio Science,1988,23(4):713-720. doi: 10.1029/RS023i004p00713
[10] GHIGLIA D C, ROMERO L A. Robust two-dimensional weighted and unweighted phase unwrapping that uses fast transforms and iterative methods[J]. Journal of the Optical Society of America A,1994,11(1):107-117. doi: 10.1364/JOSAA.11.000107
[11] 刘景峰, 李艳秋, 刘克. 一种新的质量图导引路径积分相位展开算法[J]. 光电工程,2007,34(12):104-107. LIU Jingfeng, LI Yanqiu, LIU Ke. New path-following phase unwrapping algorithm based on quality-guided[J]. Opto-Electronic Engineering,2007,34(12):104-107.
[12] GAO D P, YIN F L. Mask cut optimization in two-dimensional phase unwrapping[J]. IEEE Geoscience and Remote Sensing Letters,2012,9(3):338-342. doi: 10.1109/LGRS.2011.2168940
[13] ZHONG H P, TANG J S, ZHANG Sen, et al. A quality-guided and local minimum discontinuity based phase unwrapping algorithm for InSAR/InSAS interferograms[J]. IEEE Geoscience and Remote Sensing Letters,2014,11(1):215-219. doi: 10.1109/LGRS.2013.2252880
[14] GHIGLIA D, PRITT M D. Robust two-dimensional weighted and unweighted phase unwrapping that uses fast transforms and iterative methods[J]. JOSA A, 1994, 11(1): 107-117.
[15] HE Y T, ZHU Y J, QIN G F, et al. New quality weight used for phase unwrapping in color fringe reflection method[J]. Measurement,2021,176:109187. doi: 10.1016/j.measurement.2021.109187
[16] 朱勇建, 刘立人, 栾竹, 等. 相位解包裹算法中基于调制度的新质量图[J]. 中国激光,2006,33(5):667-672. doi: 10.3321/j.issn:0258-7025.2006.05.020 ZHU Yongjian, LIU Liren, LUAN Zhu, et al. New quality map based on modulation for phase unwrapping algorithm[J]. Chinese Journal of Lasers,2006,33(5):667-672. doi: 10.3321/j.issn:0258-7025.2006.05.020
[17] 王吉元, 田爱玲, 王红军. 四步相移干涉测量的相位补偿及仿真[J]. 西安工业学院学报,2005,25(1):23-27. WANG Jiyuan, TIAN Ailing, WANG Hongjun. Phase error compensation algorithm and simulation four-step phase shifting interferometry[J]. Journal of Xi'an Institute of Technology,2005,25(1):23-27.
-
期刊类型引用(1)
1. 王中，赵士桢，郑拓. PWM方波边沿时间对机动车流量输出调节系统电磁辐射发射的影响. 上海计量测试. 2023(03): 2-5 . 
百度学术
其他类型引用(1)
计量
- 文章访问数: 183
- HTML全文浏览量: 63
- PDF下载量: 44
- 被引次数: 2
陕公网安备 61011302001501号 