Inter-partition phase unwrapping method based on Gray code
-
摘要:
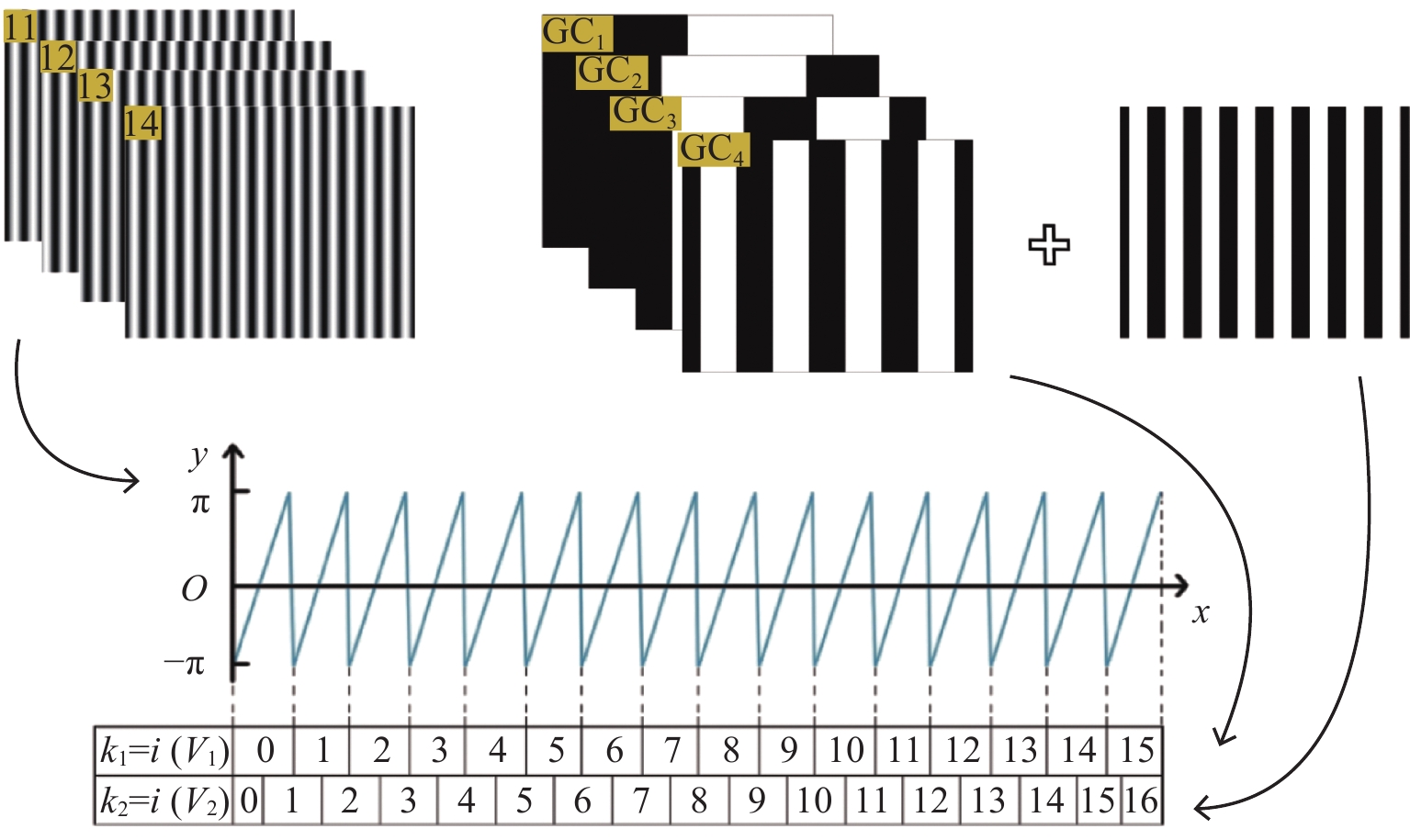
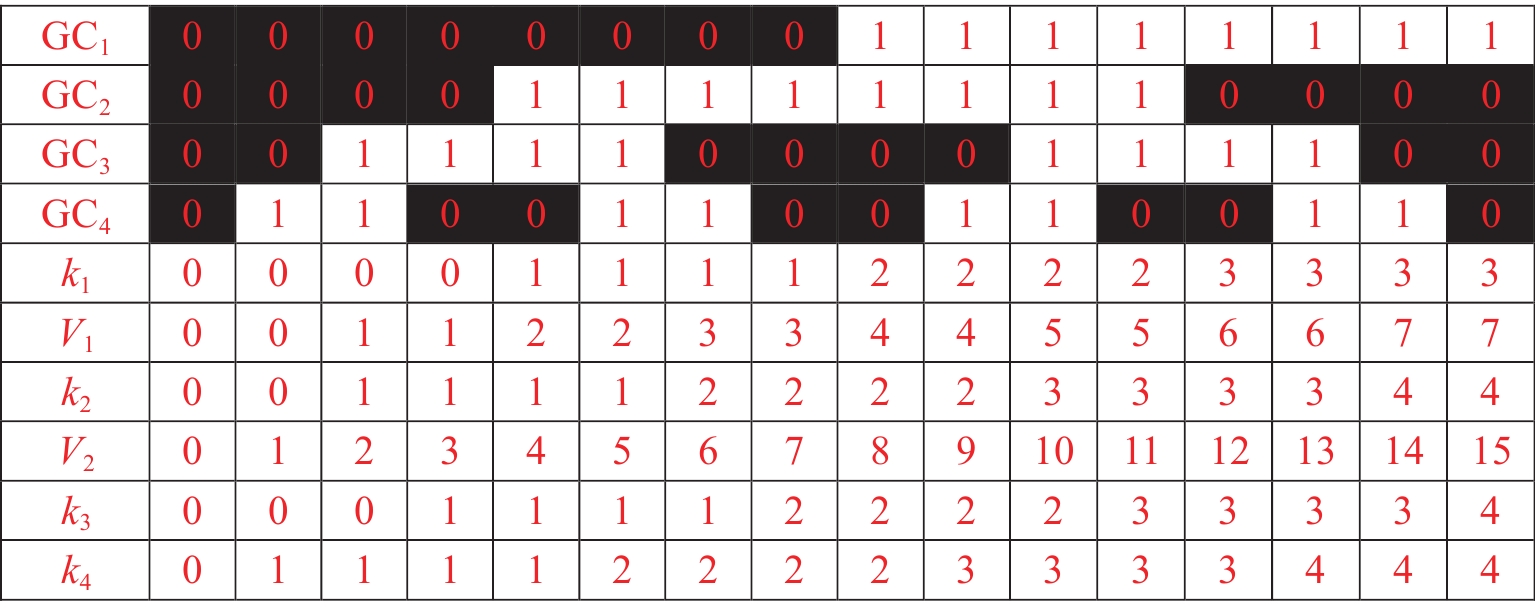
格雷码因具有良好的鲁棒性和抗噪性,被广泛应用到结构光投影三维成像方法中。在三维测量过程中,由于设备以及其他环境噪声的影响,格雷码方法解码条纹级次边沿和截断相位边沿通常无法处于理想的对齐状况,使得展开的相位出现跳变现象。为了更好地避免级次跳变误差,使得边沿跳变区域的容错宽度更大,提出基于格雷码的分区间相位展开方法。在互补格雷码基础上增加一幅格雷码图像,利用所有格雷码解得附加码字,通过对附加码字进行不同位移量的条纹级次映射,得到2个辅助条纹级次。利用所有条纹级次,对截断相位进行分区间相位展开,在边沿跳变区域错误大于半个周期时,仍能获取到无跳变现象的展开相位。实验结果表明,当边沿错误区域宽度小于3/4个条纹周期宽度时,可以有效避免级次跳变产生的误差。
Abstract:Gray codes are widely used in structured-light projection three-dimensional (3D) imaging because of good robustness and noise immunity. During the process of 3D measurement, due to the influence of equipment and other environmental noises, the gray-code decoding fringe-order edge and the truncated phase edge usually cannot be in an ideal alignment state, which causes the unwrapped phase to jump. In order to better avoid the level hopping error and make the error tolerance width of the edge hopping region larger, an inter-partition phase unwrapping method based on Gray codes was proposed. A Gray code image was added on the basis of the complementary Gray code, the additional code words were obtained by decoding all the Gray codes, and two auxiliary fringe orders were obtained by performing fringe order mapping with different displacements on the additional code words. Using all the fringe orders, the inter-partition phase unwrapping was performed on the truncated phase. When the error in the edge hopping region was greater than half a period, the unwrapping phase without hopping could still be obtained. The experimental results show that when the width of the edge error region is less than 3/4 of the stripe period width, the error caused by the level hopping can be effectively avoided.
-
Keywords:
- three-dimensional measurement /
- Gray code /
- phase unwrapping /
- inter-partition /
- level hopping error
-
引言
水下视觉成像在海底资源勘探、海洋开发、水下探测、水下反恐等方面有广泛的应用[1-2]。众所周知,水下声成像[3]的图像分辨率低,目标细节无法从图像中获取,难以满足上述应用需要。水下光成像,尤其是水下主动激光成像技术,相比于水下声成像有较高的成像分辨率,因而在海洋探索以及海洋军事方面具有重要的应用价值[4-6]。
激光逐点扫描三角测距成像[7-8]因其简单易行、成本低,受到人们的关注,但存在的问题是系统的横向扫描测量范围和纵向测距范围相互制约。M.Rioux等[9-10]提出的同步扫描三角测距成像方法,通过扫描光路与接收成像光路共用一扫描器,使得接收视场与扫描光束方向同步,扩大了系统横向扫描范围和纵向景深。文献[11]和[12]进一步研究了激光同步扫描三角测距成像系统的特点,从理论上推出了一般意义轨迹圆方程,并得出系统所能测量的最大和最小范围、距离测量分辨率等与系统参数的关系规律。对于激光同步扫描三角测距成像系统水下应用而言,由于光线在“空气-玻璃-水”发射过程,以及“水-玻璃-空气”接收过程中不同界面的多次折射,其测量方程、系统特性与系统参数的关系等,与文献[13]和[14]得到的空气中应用情况完全不同。因此,有必要对其作专门研究和讨论。文献[15]和[16]研究了使用同步扫描技术获取水下目标的二维图像,但无法对目标物体精确测量。
本文针对激光同步扫描三角测距成像系统水下应用,通过理论建模,得出水下同步扫描三角测距成像系统的空间三维坐标测量关系表达式,进一步分析了系统主要参数对系统测量范围、距离测量分辨率的影响,为水下同步扫描三角测距成像系统的设计提供了理论基础。
1 水下同步扫描三角测量系统建模
水下同步扫描三角测量系统工作原理如图 1(a)所示。激光器发出准直激光束经双面反射镜M3、反射镜M1反射后,通过密封窗玻璃M4进入水中,到达被测物体某一点$ \widetilde Q$,部分反射光通过密封窗玻璃M4进入空气中,经反射镜M2、双面反射镜M3另一反射面,由接收透镜接收,汇聚成像在成像探测器上。根据三角测距原理,汇聚成像的光点在成像探测器上的位置可以确定相对应的$ \widetilde Q$点的坐标。当M3绕轴往复摆动时,光点$ \widetilde Q$在被测物体表面上沿X轴方向行扫描;将该装置摆动或平移(本文采用平移方式),光点$ \widetilde Q$在被测物体表面作光栅轨迹扫描,可得整个物面的三维坐标。
图 1(b)系统二维图中,双面反射镜M3位置坐标为系统坐标原点O=(0 0),初始角度X轴方向为θ3。反射镜M1、M2位置坐标分别是:PM1=(d13 0)、PM2=(d23 0),相对X轴的角度分别为θ1、θ2。焦距f0的接收透镜孔径为D,水平安放位置c=(0 -s);成像探测器沿光轴左右对称,与透镜主平面的倾斜角度为0°;密封窗玻璃M4安放位置PM4=(0 h),垂直于X-Y平面,厚度为e。系统基线距离为d=d13+d32。在图 1(b)中虚线为不发生界面折射时的光路,实线为发生界面折射后的光路。
图 2的光路展开中点P为初始位置点,在成像探测器上对应点为p0;P′点为M3转动一定角度,成像探测器上对应点未发生改变仍为p0的位置点;对于任意一点Q(x y),M3转动一定角度且成像面光点位置发生改变,在成像探测器上对应点为p点,p点相对成像探测器主点p0的位置,记为q,是有方向的实数,与成像探测器转角同向为正,反向为负。
M3的转角为θ3时,发射光路激光在空气中方向向量为$ di{r_{\overline {E'{A_{p'}}} }} = 2{\theta _1} - 2{\theta _3} - \left( {{\rm{ \mathit{ π} }}/2} \right)$,接收光路激光在空气中方向向量为$di{r_{\overline {{W_{P'}}H'} }} = 2{\theta _2} - 2{\theta _3} - \left( {{\rm{ \mathit{ π} }}/2} \right) $,M3在展开光路中分别为M3′和M3″,O点位置分别对应展开光路的O′和O″点。O′和O″分别表示为
$$ \begin{array}{l} O' = {P_{{\rm{M1}}}} - \overline {O'{P_{{\rm{M1}}}}} = {\left[ {\begin{array}{*{20}{c}} { - {d_{13}} - {d_{13}}\cos \left( {2{\theta _1} - {\rm{ \mathit{ π} }}} \right)}\\ { - {d_{13}}\sin \left( {2{\theta _1} - {\rm{ \mathit{ π} }}} \right)} \end{array}} \right]^{\rm{T}}}\\ O'' = {P_{{\rm{M2}}}} + \overline {{P_{{\rm{M2}}}}O''} = {\left[ {\begin{array}{*{20}{c}} {{d_{32}} + {d_{32}}\cos \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right)}\\ {{d_{32}}\sin \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right)} \end{array}} \right]^{\rm{T}}} \end{array} $$ (1) 由(1)式可知,O′和O″点位置不随M3转动而变化。发射光线在展开光路中由于M3的转动由实线变化为点划线,转动中心为O′,如图 3所示。
如图 1(b)所示,设M3镜片厚度为ThM3,坐标原点O到M3上反射面的距离为ThM3_up,E、H两点的坐标分别为
$$ \begin{array}{l} E = {\left[ {\begin{array}{*{20}{c}} 0&{\frac{{T{h_{{\rm{M3\_up}}}}}}{{\cos \left( {{\theta _3}} \right)}}} \end{array}} \right]^{\rm{T}}}\\ H = {\left[ {\begin{array}{*{20}{c}} 0&{\frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}}} \end{array}} \right]^{\rm{T}}} \end{array} $$ (2) E、H在展开光路中对应点E′、H′的坐标为
$$ E' = O' + \overline {O'E'} = {\left[ {\begin{array}{*{20}{c}} { - {d_{13}} - {d_{13}}\cos \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}}}}{{\cos \left( {{\theta _3}} \right)}}\cos \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right)}\\ { - {d_{13}}\sin \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}}}}{{\cos \left( {{\theta _3}} \right)}}\sin \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right)} \end{array}} \right]^{\rm{T}}} $$ (3) $$ H' = O'' + \overline {O''H'} = {\left[ {\begin{array}{*{20}{c}} {{d_{32}} + {d_{32}}\cos \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}}\cos \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right)}\\ {{d_{32}}\sin \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}}\sin \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right)} \end{array}} \right]^{\rm{T}}} $$ (4) 由于M3具有一定厚度,因此光路行程会发生微小改变。透镜光心c到H点的行程为$s' = \left\| {\overline {cH} } \right\| = s + \frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}} $。透镜光心c在展开光路中的对应点c′坐标为
$$ c' = H' + \overline {H'c'} = \left[ {\begin{array}{*{20}{c}} {{d_{32}} + {d_{32}}\cos \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}}\cos \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right) + s'\cos \left( {2{\theta _2}{\rm{ - }}2{\theta _3}{\rm{ - }}\frac{{\rm{ \mathit{ π} }}}{2}} \right)}\\ {{d_{32}}\sin \left( {2{\theta _2} - {\rm{ \mathit{ π} }}} \right) + \frac{{T{h_{{\rm{M3\_up}}}} - T{h_{{\rm{M3}}}}}}{{\cos \left( {{\theta _3}} \right)}}\sin \left( {2{\theta _1} - \frac{{\rm{ \mathit{ π} }}}{2}} \right){\rm{ + }}s'{\rm{sin}}\left( {2{\theta _2}{\rm{ - }}2{\theta _3}{\rm{ - }}\frac{{\rm{ \mathit{ π} }}}{2}} \right)} \end{array}} \right] $$ (5) 图 3为成像光束主光线方向。在三角形Δc′p0p中,已知$ \angle c'{p_0}p = \frac{{\rm{ \mathit{ π} }}}{{\rm{2}}}$,$\left| {{p_0}c'} \right| = {f_0} $,$\left| {{p_0}p} \right| = q $,由正弦定理可得:$ \alpha = \arctan \left( {\frac{q}{{{f_0}}}} \right)$,因此成像光线方向为
$$ di{r_{\overline {WQP} }} = di{r_{\overline {c'p} }} = 2{\theta _2}{\rm{ - }}2{\theta _3}{\rm{ - }}\frac{{\rm{ \mathit{ π} }}}{2} + \alpha $$ (6) 如图 2所示,点$\widetilde Q $对应的Q点既在投射激光方向上又在入射成像光线上,由于光线经“空气-玻璃-水”界面的多次折射,Q点为这两条折线的交点。当M3的转角为θ3,成像探测器上成像点位置为p时,折线各段的方向向量如表 1所示。其中n1,n2,n3分别为光在空气、玻璃和水中的折射率。
表 1 各段光路方向向量Table 1. Direction vector for each section of light path线段 方向向量 线段 方向向量 E′AQ $di{r_{\overline {E'{\mathit{A}_\mathit{Q}}} }} = 2{\theta _1}{\rm{ - }}2{\theta _3}{\rm{ - }}\left( {{\rm{ \mathit{ π} }}/2} \right) $ WQH′ $di{r_{\overline {{W_Q}H'} }} = 2{\theta _2}{\rm{ - }}2{\theta _3}{\rm{ - }}\left( {{\rm{ \mathit{ π} }}/2} \right) + \alpha $ AQA′Q $di{r_{\overline {{A_Q}A{'_Q}} }} = \arccos \left( {\frac{{{n_1}}}{{{n_2}}}\cos \left( {di{r_{\overline {E'{\mathit{A}_{P'}}} }}} \right)} \right) $ WQW′Q $di{r_{\overline {{W_Q}W{'_Q}} }} = \arccos \left( {\frac{{{n_1}}}{{{n_2}}}\cos \left( {di{r_{\overline {{W_{P'}}H'} }}} \right)} \right) $ AQQ $di{r_{\overline {A{'_Q}Q} }} = \arccos \left( {\frac{{{n_2}}}{{{n_3}}}\cos \left( {di{r_{\overline {{A_{P'}}A{'_{P'}}} }}} \right)} \right) $ WQQ $di{r_{\overline {W{'_Q}Q} }} = \arccos \left( {\frac{{{n_2}}}{{{n_3}}}\cos \left( {di{r_{\overline {{W_{P'}}W{'_{P'}}} }}} \right)} \right) $ $$ \left\{ \begin{array}{l} \cot \left( {di{r_{\overline {E'{\mathit{A}_\mathit{Q}}} }}} \right) = \frac{{{A_{{Q_x}}} - O{'_x}}}{{{A_{{Q_y}}} - O{'_y}}}\\ \cot \left( {di{r_{\overline {c'{W_Q}} }}} \right) = \frac{{{W_{{Q_x}}} - c{'_x}}}{{{W_{{Q_y}}} - c{'_y}}} \end{array} \right. $$ (7) 解(7)式中2个方程,可得投射激光和入射成像光线与密封罩下表面的交点坐标:
$$ \left\{ \begin{array}{l} {A_Q} = {\left[ {\begin{array}{*{20}{c}} {\cot \left( {di{r_{\overline {E'{\mathit{A}_\mathit{Q}}} }}} \right)\left( {h - Q{'_y}} \right) - O{'_x}}&h \end{array}} \right]^{\rm{T}}}\\ {W_Q} = {\left[ {\begin{array}{*{20}{c}} {\cot \left( {di{r_{\overline {c'{W_Q}} }}} \right)\left( {h - c{'_y}} \right) - c{'_x}}&h \end{array}} \right]^{\rm{T}}} \end{array} \right. $$ (8) $$ \left\{ \begin{array}{l} \cot \left( {di{r_{\overline {{\mathit{A}_\mathit{Q}}A{'_Q}} }}} \right) = \frac{{{A_{{Q_x}}} - A{'_{{Q_x}}}}}{{{A_{{Q_y}}} - A{'_{{Q_y}}}}}\\ \cot \left( {di{r_{\overline {{W_\mathit{Q}}W{'_Q}} }}} \right) = \frac{{{W_{{Q_x}}} - W{'_{{Q_x}}}}}{{{W_{{Q_y}}} - W{'_{{Q_y}}}}} \end{array} \right. $$ (9) 解(9)式2个方程,可得投射激光和入射成像光线与密封罩上表面的交点坐标:
$$ \left\{ \begin{array}{l} A{'_Q} = {\left[ {\begin{array}{*{20}{c}} {\cot \left( {di{r_{\overline {{\mathit{A}_\mathit{Q}}A{'_Q}} }}} \right)\left( {h + e - {A_{{Q_y}}}} \right)}&{h + e} \end{array}} \right]^{\rm{T}}}\\ W{'_Q} = {\left[ {\begin{array}{*{20}{c}} {\cot \left( {di{r_{\overline {{W_\mathit{Q}}W{'_Q}} }}} \right)\left( {h + e - {W_{{Q_y}}}} \right)}&{h + e} \end{array}} \right]^{\rm{T}}} \end{array} \right. $$ (10) $$ \left\{ \begin{array}{l} \cot \left( {di{r_{\overline {A{'_Q}Q} }}} \right) = \frac{{{Q_x} - A{'_{{Q_x}}}}}{{{Q_y} - {A_{{Q_y}}}}}\\ \cot \left( {di{r_{\overline {W{'_Q}Q} }}} \right) = \frac{{{Q_x} - W{'_{{Q_x}}}}}{{{Q_y} - W{'_{{Q_y}}}}} \end{array} \right. $$ (11) 解方程组(11),可得Q点在光路展开图中的坐标:
$$ Q = \left[ {\begin{array}{*{20}{c}} {\frac{{\left( {h + h' + e} \right)\left[ {\cot \left( {di{r_{\overline {W{'_Q}Q} }}} \right) - \cot \left( {di{r_{\overline {A{'_Q}Q} }}} \right)} \right] + A{'_{{Q_x}}} - W{'_{{Q_x}}}}}{{\cot \left( {di{r_{\overline {W{'_Q}Q} }}} \right) - \cot \left( {di{r_{\overline {A{'_Q}Q} }}} \right)}}}\\ {\left( {{Q_y} - h - h' - e} \right)\cot \left( {di{r_{\overline {A{'_Q}Q} }} + A{'_{{Q_x}}}} \right)} \end{array}} \right] $$ (12) 本文采用图 1整个装置平移方式实现对物面的光栅轨迹扫描,取该装置的在导轨上的位移为Qz,测量点三维坐标为
$$ \widetilde Q = \left[ {\begin{array}{*{20}{c}} {\frac{{\left( {h + h' + e} \right)\left[ {\cot \left( {di{r_{\overline {W{'_Q}Q} }}} \right) - \cot \left( {di{r_{\overline {A{'_Q}Q} }}} \right)} \right] + A{'_{{Q_x}}} - W{'_{{Q_x}}}}}{{\cot \left( {di{r_{\overline {W{'_Q}Q} }}} \right) - \cot \left( {di{r_{\overline {A{'_Q}Q} }}} \right)}}}\\ {\left( {{Q_y} - h - h' - e} \right)\cot \left( {di{r_{\overline {A{'_Q}Q} }} + A{'_{{Q_x}}}} \right)}\\ {{Q_z}} \end{array}} \right] $$ (13) 2 水下同步扫描三角测距成像系统分析
2.1 距离测量分辨率分析
系统采用的成像探测器为线阵CCD或PSD,根据三角测距原理,距离测量分辨率由成像探测器上最小可分辨单元决定,采用的成像探测器长度L为30 mm,最小分辨单元为0.005 mm。根据公式(12),采用Matlab对系统进行仿真分析,考察基线距离d、物镜焦距f0、接收透镜到原点坐标的距离s、密封窗玻璃到原点坐标的距离h等系统参数改变对距离测量分辨率的影响情况。图 4(a)为基线距离d、物镜焦距f0改变,测量距离分辨率变化情况,此时取s为30 mm,h为60 mm;图 4(b)为接收透镜到原点坐标的距离s、密封窗玻璃到原点坐标的距离h改变,测量距离分辨率变化情况,此时取f0为75 mm,d为80 mm。从图 4可见,基线距离d和物镜的焦距f0对分辨率影响较大,而接收透镜到原点坐标的距离s和密封窗玻璃到原点坐标的距离h对分辨率影响较小。
图 5(a)为物镜焦距f0=75 mm,基线距离d为80 mm、90 mm、100 mm时,距离分辨率在不同测量距离上的变化情况,此时s=30 mm,h=60 mm。从图 5(a)中可见,距离测量分辨率随基线距离增大而提高,随测量距离增大而降低。图 5(b)为取基线距离d=80 mm,物镜焦距f0为70 mm、80 mm、90 mm时,距离测量分辨率在不同测量距离上的变化情况,此时s=30 mm,h=60 mm。从图 5中可见,距离分辨率随物镜焦距f0增大而提高,随测量距离增大而降低。
2.2 测量范围分析
与系统测量范围有关的参数主要有:基线距d、接收透镜到原点坐标的距离s、接收透镜焦距f0、密封窗玻璃到原点坐标的距离h、成像探测器长度L,取d、s、f0、h、L分别为75 mm、30 mm、85 mm、60 mm、25 mm。将其中一个参数分别改变5 mm、10 mm,其余参数保持不变,系统测量范围变化量如表 2所示。根据表 2可知,成像探测器长度L对系统测量范围的影响远大于其他参数。因此,成像探测器长度L是影响系统测量范围的主要参数。
表 2 系统测量范围变化Table 2. Change of system measurement range任一参数改变5 mm,其他参数不变 d s f0 h L 测量范围变化 横向/mm 25.45 4.37 -37.05 -0.08 176.83 纵向/mm 47.26 12.23 -98.63 -0.33 461.65 任一参数改变5 mm,其他参数不变 d s f0 h L 测量范围变化 横向/mm 51.62 8.23 -64.91 -0.17 575.09 纵向/mm 95.22 24.26 -174.62 -0.66 1473.76 根据同步扫描三角测距原理,成像探测器上任意点p所对应的物点为一曲线,左右极点决定系统最大、最小曲线轨迹,系统测量范围由最大、最小曲线轨迹决定。不同成像探测器长度下,系统最大、最小曲线轨迹如图 6所示,其中横坐标是横向扫描范围,纵坐标为纵向测距范围。图 6(a)表示L=20 mm时,系统最大、最小曲线轨迹;图 6(b)表示L=30 mm时,系统最大、最小曲线轨迹;图 6(c)表示L=40 mm时,系统最大、最小曲线轨迹。计算结果表明,当L从20 mm增加到40 mm时,纵向测量范围增加了1 787 mm,横向测量范围增加了689 mm。
2.3 设计实例
作为设计实例,取基线距离d为100 mm、物镜焦距f0为75 mm、接收透镜到原点坐标的距离s为25 mm、密封窗玻璃到原点坐标的距离h为50 mm、成像探测器长度L为37 mm,系统扫描测量范围和扫描测量范围内的距离测量分辨率如图 7所示。由图 7可知,在该参数条件下,最远处的距离测量分辨率为1.97 mm,最近处的距离测量分辨率为0.04 mm,系统横向扫描范围为864 mm,系统纵向测量范围为1 899 mm。
3 结论
本文考虑水下同步扫描三角测量系统中, 光线在不同介质界面的折射效应, 通过理论建模,得出成像系统测量方程。分析了基线距离d、接收透镜焦距f0、成像探测器长度L等参数对距离测量分辨率及测量范围的影响。通过仿真分析可知,接收透镜焦距f0和基线距离d是影响距离测量分辨率的主要系统参数,成像探测面长度L决定系统测量范围。因此,在水下同步扫描三角测距成像系统设计时,可从系统性能要求出发,调整接收透镜焦距f0和基线距离d以满足距离测量分辨率要求。选取合适的成像探测面长度L,以满足系统测量范围要求,从而达到优化系统设计的目的,为水下同步扫描三角测距成像系统的设计提供参考。
-
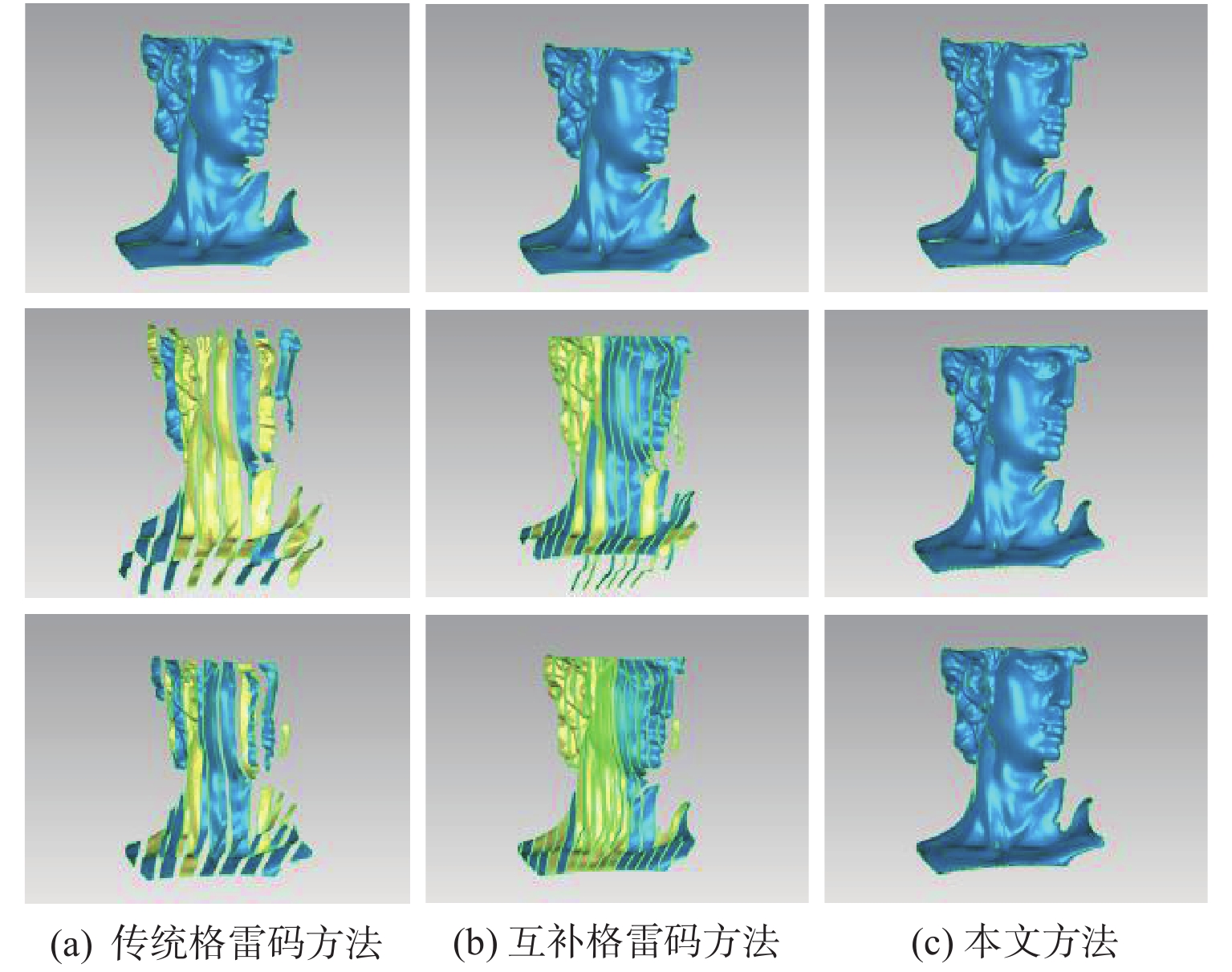
表 1 展开相位准确性分析结果
Table 1 Analysis results of unwrapped phase accuracy
相位展开方法 总点数 错误点个数 错误点占比/% 传统格雷码方法 208 458 73 227 35.13 互补格雷码方法 208 458 42 127 20.21 本文方法 208 658 2 263 1.08 -
[1] GORTHI S S, RASTOGI P. Fringe projection techniques: whither we are?[J]. Optics and Lasers in Engineering,2010,48(2):133-140. doi: 10.1016/j.optlaseng.2009.09.001
[2] 刘路, 潘艳娟, 奚冬冬, 等. 相位编码条纹投影轮廓术的相位展开误差校正方法[J]. 应用光学,2020,41(5):978-983. doi: 10.5768/JAO202041.0502007 LIU Lu, PAN Yanjuan, XI Dongdong, et al. Phase unwrapping errors correction for phase-encoding based on fringe projection profilometry[J]. Journal of Applied Optics,2020,41(5):978-983. doi: 10.5768/JAO202041.0502007
[3] CHEN F, BROWN G M, SONG M. Overview of 3-D shape measurement using optical methods[J]. Optical Engineering,2000,39(1):10-22. doi: 10.1117/1.602438
[4] 朱勇建, 黄振, 马俊飞, 等. 光栅投影三维测量系统标定技术研究[J]. 应用光学,2020,41(5):938-946. doi: 10.5768/JAO202041.0502002 ZHU Yongjian, HUANG Zhen, MA Junfei, et al. Study on calibration method of grating projection 3D measuring system[J]. Journal of Applied Optics,2020,41(5):938-946. doi: 10.5768/JAO202041.0502002
[5] SU X, CHEN W. Reliability-guided phase unwrapping algorithm: a review[J]. Optics and Lasers in Engineering,2004,42(3):245-261. doi: 10.1016/j.optlaseng.2003.11.002
[6] LI B Y, TANG C, ZHOU Q L, et al. Weighted least-squares phase-unwrapping algorithm based on the orientation coherence for discontinuous optical phase patterns[J]. Applied Optics,2019,58(2):219-226. doi: 10.1364/AO.58.000219
[7] 钱晓凡, 张永安, 李新宇, 等. 基于掩膜和最小二乘迭代的相位解包裹方法[J]. 光学学报,2010,30(2):440-444. doi: 10.3788/AOS20103002.0440 QIAN Xiaofan, ZHANG Yong'an, LI Xinyu, et al. Phase unwrapping algorithm based on mask and least-squares iteration[J]. Acta Optica Sinica,2010,30(2):440-444. doi: 10.3788/AOS20103002.0440
[8] CHENG Y Y, WYANT J C. Two-wavelength phase shifting interferometry[J]. Applied Optics,1984,23(24):4539. doi: 10.1364/AO.23.004539
[9] WANG Y J, ZHANG S. Novel phase-coding method for absolute phase retrieval[J]. Optics Letters,2012,37(11):2067-2069. doi: 10.1364/OL.37.002067
[10] SANSONI G, CORINI S, LAZZARI S, et al. Three-dimensional imaging based on Gray-code light projection: characterization of the measuring algorithm and development of a measuring system for industrial applications[J]. Applied Optics,1997,36(19):4463-4472. doi: 10.1364/AO.36.004463
[11] LAUGHNER J I, ZHANG S, LI H, et al. Mapping cardiac surface mechanics with structured light imaging[J]. American Journal of Physiology Heart and Circulatory Physiology,2012,303(6):712-720. doi: 10.1152/ajpheart.00269.2012
[12] ZHENG D L, QIAN K M, DA F P, et al. Ternary Gray code-based phase unwrapping for 3D measurement using binary patterns with projector defocusing[J]. Applied Optics,2017,56(13):3660-3665. doi: 10.1364/AO.56.003660
[13] LEI S Y, ZHANG S. Flexible 3-D shape measurement using projector defocusing[J]. Optics Letters,2009,34(20):3080-3082. doi: 10.1364/OL.34.003080
[14] WU Z J, ZUO C, GUO W B, et al. High-speed three-dimensional shape measurement based on cyclic complementary Gray-code light[J]. Optics Express,2019,27(2):1283-1297. doi: 10.1364/OE.27.001283
[15] 孙学真, 苏显渝, 邹小平. 基于互补型光栅编码的相位展开[J]. 光学学报,2008,28(10):1947-1951. doi: 10.3321/j.issn:0253-2239.2008.10.020 SUN Xuezhen, SU Xianyu, ZOU Xiaoping. Phase-unwrapping based on complementary structured light binary code[J]. Acta Optica Sinica,2008,28(10):1947-1951. doi: 10.3321/j.issn:0253-2239.2008.10.020
[16] ZHANG Q C, SU X Y, XIANG L Q, et al. 3-D shape measurement based on complementary Gray-code light[J]. Optics and Lasers in Engineering,2012,50(4):574-579. doi: 10.1016/j.optlaseng.2011.06.024
[17] 张启灿, 吴周杰. 基于格雷码图案投影的结构光三维成像技术[J]. 红外与激光工程,2020,49(3):78-90. ZHANG Qican, WU Zhoujie. Three-dimensional imaging technique based on Gray-coded structured illumination[J]. Infrared and Laser Engineering,2020,49(3):78-90.
[18] SRINIVASAN V, LIU H C, HALIOUA M. Automated phase-measuring profilometry of 3-D diffuse objects[J]. Applied Optics,1984,23(18):3105. doi: 10.1364/AO.23.003105
-
期刊类型引用(6)
1. 王宇航,王新宇,张景辉,卜璐杰,张韬. 水下三维光学成像探测技术综述及展望. 激光与光电子学进展. 2024(22): 29-42 .  百度学术
百度学术
2. 张伟,李忠,袁四化,李梵若. 数据挖掘的单光束脉冲激光方位识别精度分析. 激光杂志. 2022(08): 180-184 .  百度学术
百度学术
3. 吉勇,屠大维,张旭,李晨. 水下主动激光扫描系统中光场记录及散射背景分离研究. 电子测量与仪器学报. 2020(05): 58-64 .  百度学术
百度学术
4. 卢治功,贺鹏,职连杰,陈文建. 基于最小二乘法多项式拟合三角测量模型研究. 应用光学. 2019(05): 853-858 .  本站查看
本站查看
5. 田锋,黄秀祥,姜捷来,李仲阳. 反求工程关键问题研究综述. 装备制造技术. 2019(08): 18-20 .  百度学术
百度学术
6. 赵丽花. 基于大数据分析的激光位移传感器测距自动校准方法研究. 激光杂志. 2019(11): 191-194 .  百度学术
百度学术
其他类型引用(5)




 下载:
下载:













 陕公网安备 61011302001501号
陕公网安备 61011302001501号 