Analysis of influence of acceleration field on deformation of gyro fiber-optical ring
-
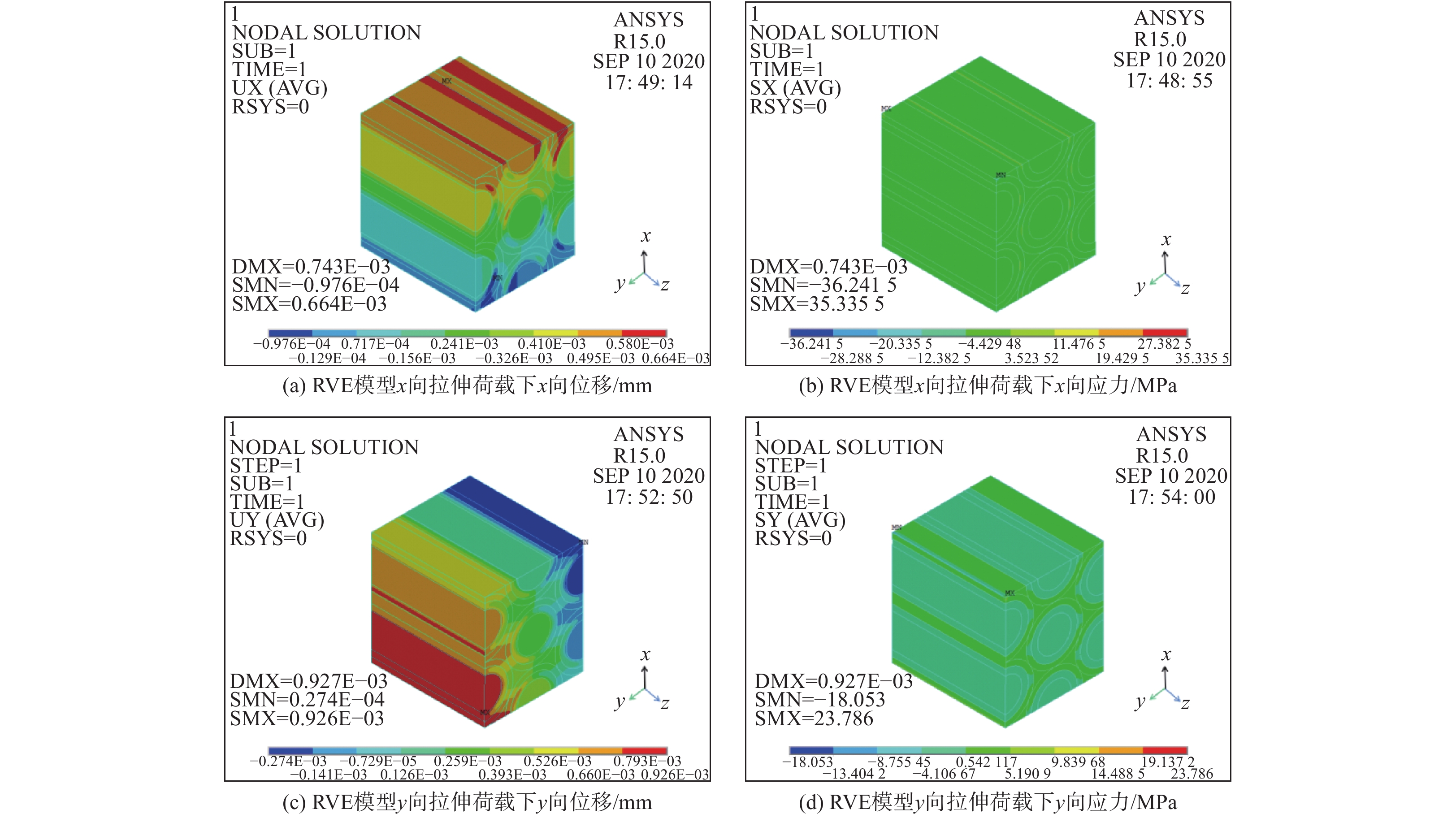
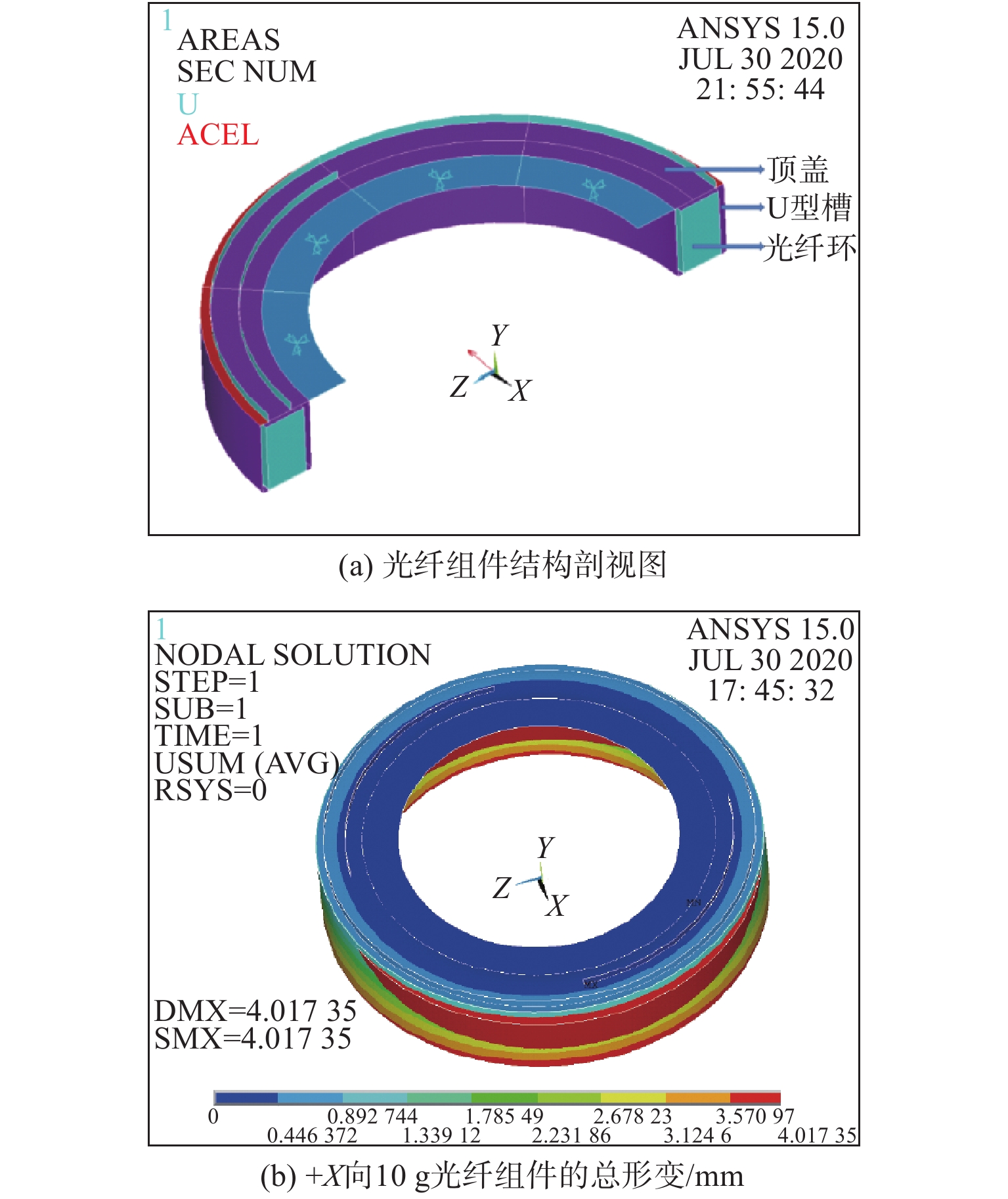
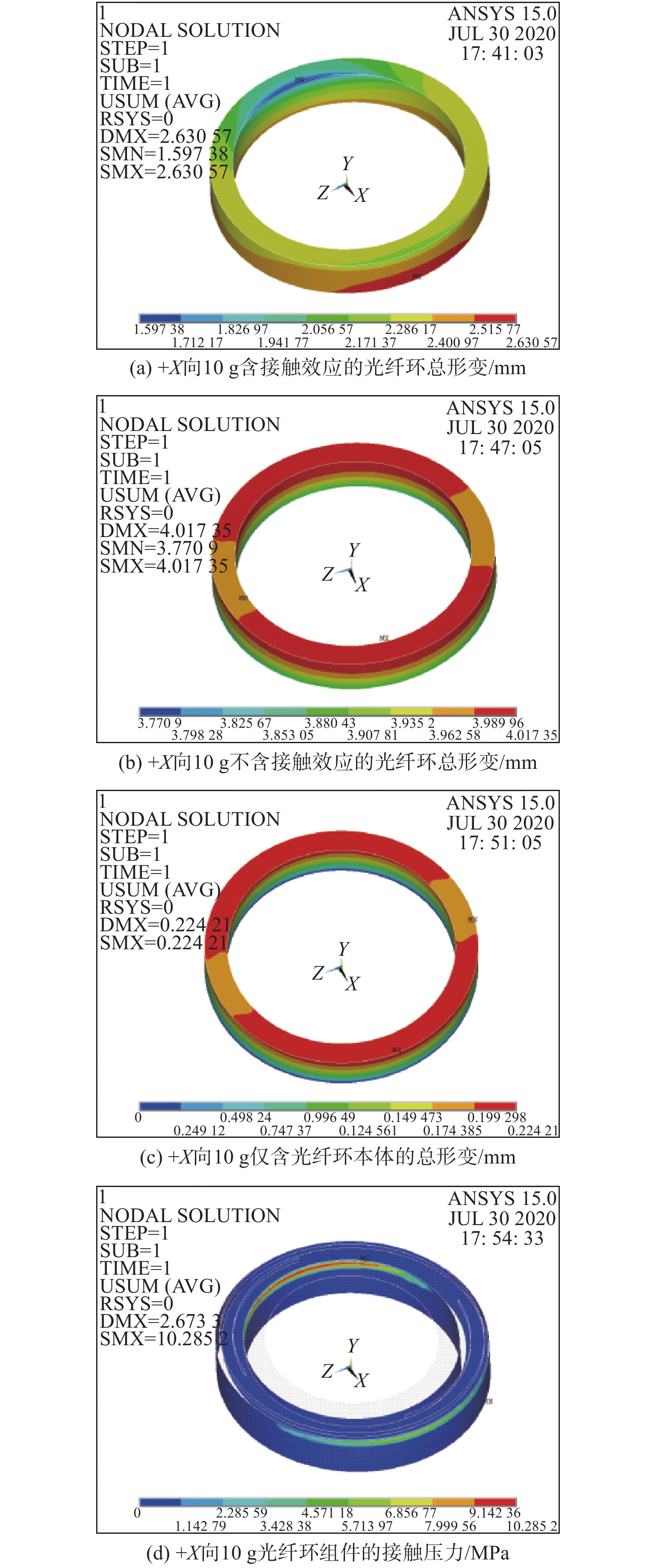
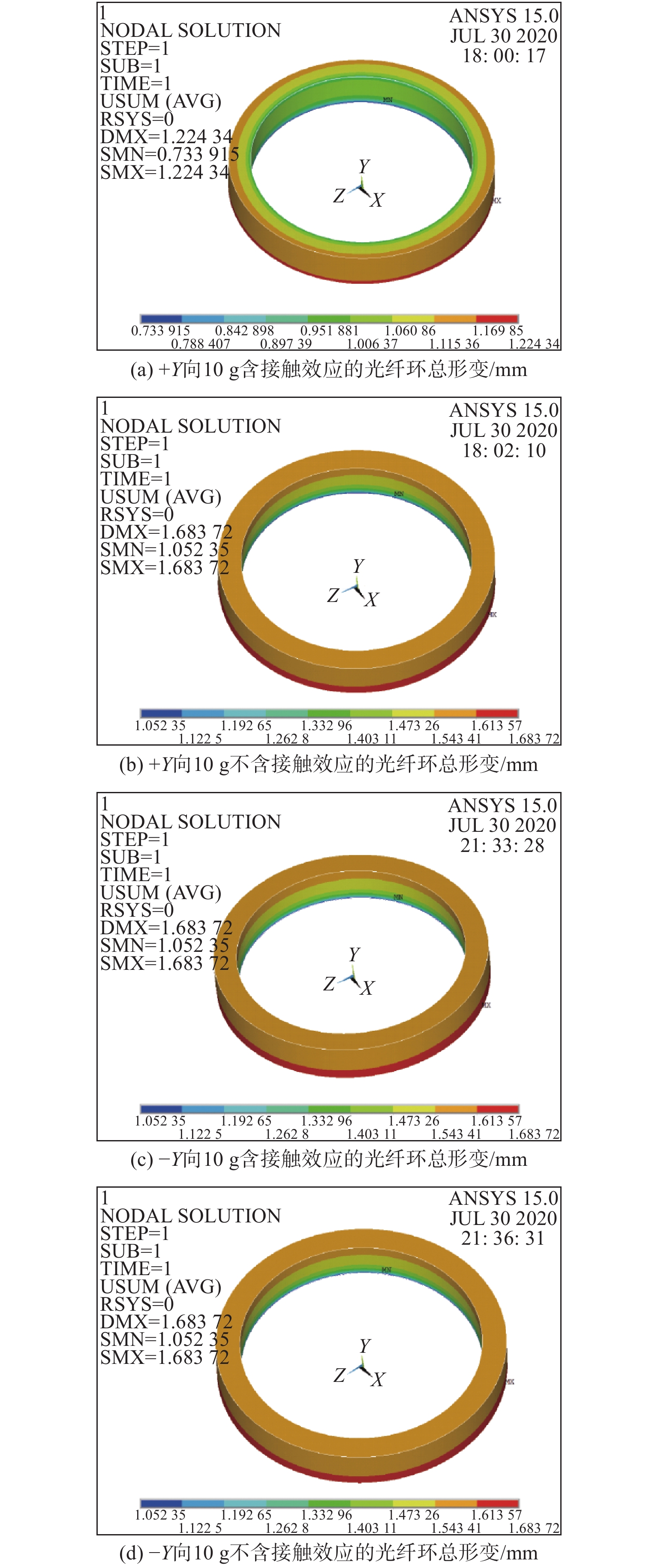
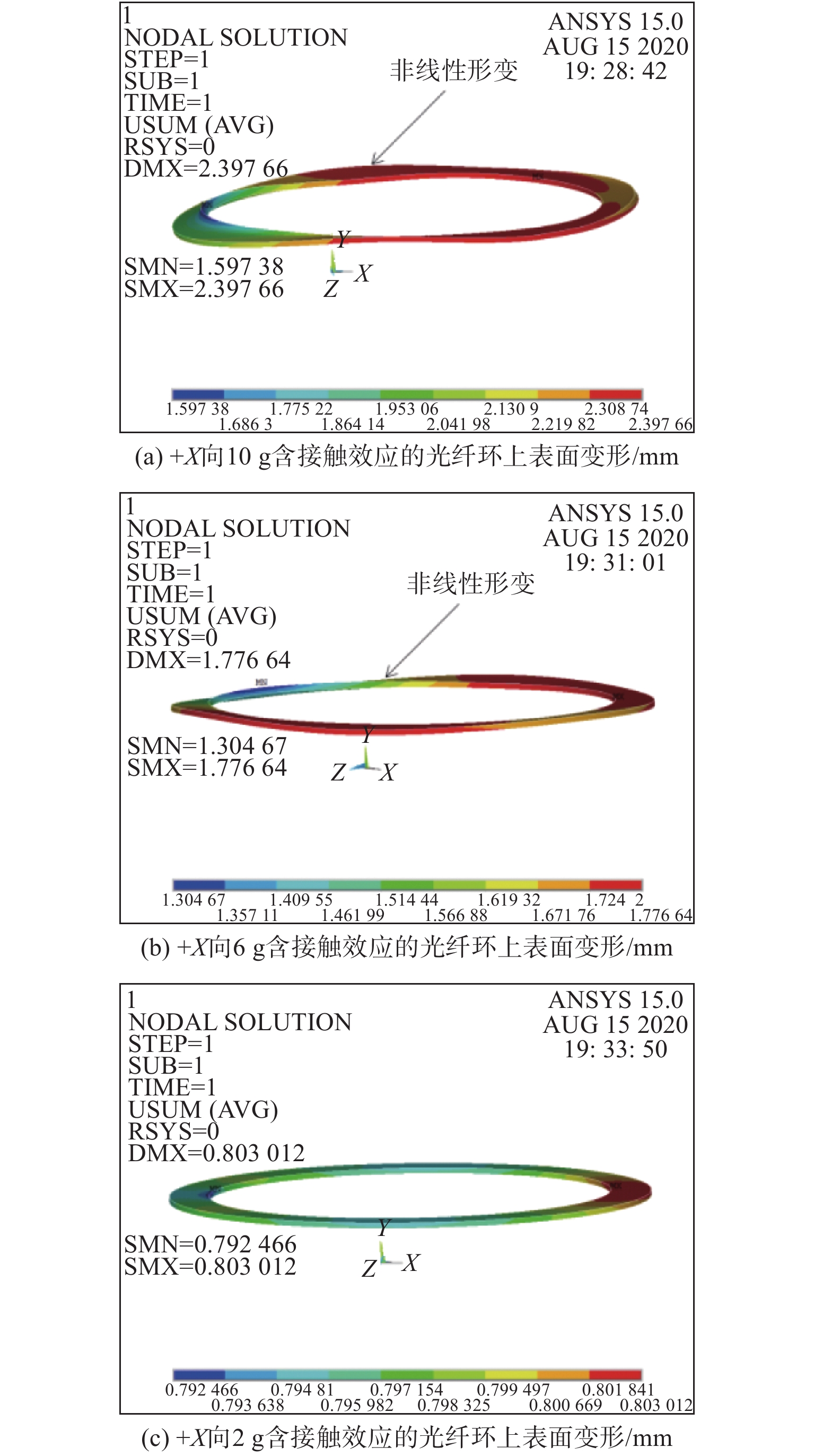
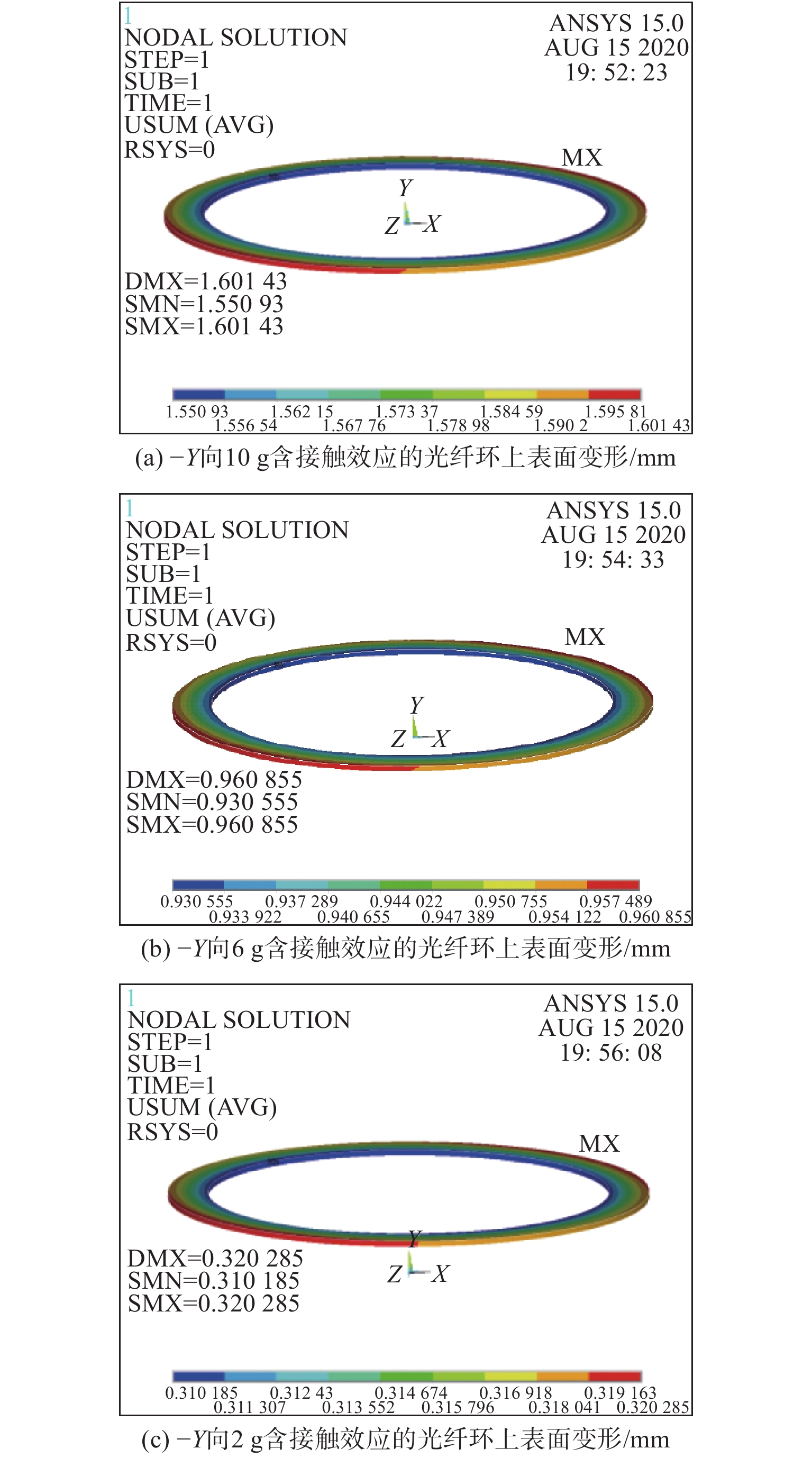
摘要: 通过航天器大机动时直线加速场下陀螺光纤环的有限元分析，得出光纤环在加速场下的形变机理。基于各向异性复合材料理论，采用细观力学有限元方法，对光纤环代表性体积单元施加周期边界条件，分析得出光纤环的等效材料参数。再利用有限元程序ANSYS建立光纤环组件的空间有限元模型，施加加速度场，并建立接触单元分析形变过程中的结构接触耦合影响。分析结果表明：在加速场的作用下，光纤环形变的主要原因包括光纤环组件中的U型槽和顶盖形变后与光纤环的接触耦合作用，以及U型槽和顶盖结构的形变引起光纤环和光纤环本体结构的形变。Abstract: Through the finite element analysis of gyroscope fiber-optical ring under the linear acceleration field of spacecraft in large maneuvering motion, the deformation mechanism of the fiber-optical ring was obtained. Based on the theoretical knowledge of anisotropic materials, the micro-mechanics finite element method was used to apply periodic boundary conditions to the representative volume element (RVE) of the fiber-optical ring. Therefore, the equivalent material parameters of the fiber-optical ring were analyzed. The finite element program ANSYS was adopted to establish the spatial finite element model of the fiber-optical ring assembly and apply the acceleration field. The contact element was established to analyze the structural contact coupling effect during the deformation process. The analysis results show that the U-shaped structure and top cover of the fiber-optical ring assembly structure will contact with the fiber-optical ring after deformation under the action of acceleration field. In addition, the U-shaped structure and top cover structure will deform under the action of inertial force, which will affect the deformation of the fiber-optical ring.
-
引言
随着我国交通大发展的持续深入推进，用户对汽油的需求呈现爆炸式增长。然而，大量不法企业为追求最大限度利润，擅自用化工原料和添加剂兑制、混配“调和汽油”，给消费安全带来了极大的隐患。因此亟需研究对掺混成品油进行快速鉴别的方法。
由于红外光谱分析技术具有检测速度快、效率高、成本低等特点[1]，已被广泛应用于成品油分析领域[2]。Veras等人利用主成分分析（principal component analysis，PCA）结合聚类分析的方法对108个柴油样品的原产地进行分类[3]；姜黎等人利用主成分分析结合马氏距离的方法比较汽油的2个特征波段建模的分类效果[4]；王丽等人利用主成分分析结合模糊聚类实现了对海洋溢油样本的快速分类[5]。然而主成分分析属于线性降维方法，其不能准确提取光谱数据中的非线性特征，导致光谱数据在降维的过程中部分有用信息丢失及鉴别模型精准度下降。鉴于此，本文采用非线性降维方法中的t分布邻域嵌入（t-distributed stochastic neighbor embedding，t-SNE）算法[6]对光谱数据进行降维处理，同时结合深度置信网络方法[7]建立汽油鉴别模型，并与极限学习机鉴别算法[8]进行识别精度对比分析，以解决掺混汽油红外光谱鉴别技术中线性降维方法缺陷和高精度识别模型选择问题。
1 材料与方法
1.1 样品来源与光谱测试
本实验所使用的92#、95#以及98#汽油样品均购置于大庆中石化加油站，掺混汽油样品由92#、95#、98#汽油按照1∶1∶1配制而成，每种样品各50份用于红外光谱测量实验。其中，每种类型取其40份作为训练集，10份作为测试集。
1.2 光谱数据处理
通过实验所采集到的光谱信息不仅包含样本特征信息，还包含外界的干扰因素[9]，这些干扰因素会对模型建立造成一定的影响。因此，有必要对原始光谱数据进行预处理[10]。分别采用多元散射校正（multiplication scattering correction，MSC）、标准正态变换（standard normal variate，SNV）以及一阶导数对原始光谱数据进行预处理，从而选择最适合本文的预处理方法。
红外光谱数据通常维度很高，如若将全部光谱数据参与模型构建，将会导致该模型识别效率下降，通常在建立模型之前需对光谱数据进行降维处理，本文采用t分布邻域嵌入（t-distributed stochastic neighbor embedding，t-SNE）算法对光谱数据进行降维[5]。
t-SNE算法具体步骤如下：
a） 用条件概率pj|i表示高维空间中邻近数据点xi与xj的相似度，邻近数据点之间的相似度越高，则条件概率pj|i值也就越大，且其服从高斯分布[11]，条件概率pj|i计算公式为
$$ {p_{j\left| i \right.}} = \dfrac{{\exp \left( { - \left\| {{x_i} - {{\left. {{x_j}} \right\|}^2}} \right.2\sigma _i^2} \right)}}{{\displaystyle\sum\nolimits_{k \ne i} {\exp \left( { - \left\| {{x_i} - {{\left. {{x_k}} \right\|}^2}} \right./2\sigma _i^2} \right)} }} $$ (1) 式中
$ {\sigma }_{i} $ 为高斯分布标准差。将高维中邻近数据点xi与xj在低维中的映射点记为yi与yj，并计算其相似的条件概率qj|i
$$ {q_{j\left| i \right.}} = \dfrac{{\exp \left( { - \left\| {{y_i} - {{\left. {{y_j}} \right\|}^2}} \right.} \right)}}{{\displaystyle\sum\nolimits_{k \ne i} {\exp \left( { - \left\| {{y_i} - {{\left. {{y_k}} \right\|}^2}} \right.} \right)} }} $$ (2) b） pj|i与qj|i分别表示高维空间中数据点xi、xj与低维空间中数据点yi、yj之间的联合概率，如（3）式和（4）式所示：
$${p_{ij}} = \dfrac{{{p_{j\left| i \right.}} + {p_{i\left| j \right.}}}}{{2n}}$$ (3) $${q_{ij}} = \dfrac{{{{\left( {1 + \left\| {{y_i} - {{\left. {{y_j}} \right\|}^2}} \right.} \right)}^{ - 1}}}}{{\displaystyle\sum\nolimits_{k \ne l} {{{\left( {1 + \left\| {{y_k} - {{\left. {{y_l}} \right\|}^2}} \right.} \right)}^{ - 1}}} }}$$ (4) c） 此时新的代价函数C可以表示为
$${{{\rm{C}}}} = {{KL}}\left( {\left. {{P}} \right\|{{Q}}} \right) = \displaystyle\sum \limits_{{i}} \displaystyle\sum \limits_{{j}} {{{p}}_{{{ij}}}}\log \dfrac{{{{{p}}_{{{ij}}}}}}{{{{{q}}_{{{ij}}}}}}$$ (5) 式中：KL为K-L散度（Kullback-Leibler divergence）；P与Q分别为高维空间和低维空间中度量点对分布概率分布。
d） 在低维空间中，t-SNE算法将使用t分布（student t-distribution）代替高斯分布以表示两个点之间的相似度。t分布在低维空间中使用更注重长尾分布，使同类的样本点在低维空间中相隔距离较近，不同类型的样本点相隔距离较远[12]。t-SNE梯度计算式可以表示为
$$\dfrac{{\delta y}}{{\delta x}}{\rm{ = }}4\displaystyle\sum\nolimits_{\rm{j}} {\left( {{p_{ij}} - {q_{ij}}} \right)} \left( {{y_i} - {y_j}} \right){\left( {1 + \left\| {{y_i} - {{\left. {{y_j}} \right\|}^2}} \right.} \right)^{ - 1}}$$ (6) 1.3 基于深度置信网络的汽油种类鉴别方法
1.3.1 数据集处理
数据集中标记的鉴别汽油种类是离散型数据，不能直接参与DBN模型计算，因此在构建DBN模型之前需要利用One-Hot编码进行转换处理。One-Hot编码使用0或1对多个分类或状态进行编码，将每个分类或状态作为独立属性，任意时刻只有其中一个属性有效，将对应的有效属性设置为1[13]，4种类型的汽油对应的编码如表1所示。
表 1 同类型汽油One-Hot编码Table 1. One-Hot coding of different types of gasoline类型 92# 95# 98# 掺混 1 1 0 0 0 2 0 1 0 0 3 0 0 1 0 4 0 0 0 1 1.3.2 深度置信网络鉴别模型
深度置信网络既可以用于非监督学习，也可以用于监督学习，其由多层受限玻尔兹曼机（restricted Boltzmann machines，RBM）组成，通过训练各个神经元之间的权重和偏置，可使整个神经网络以最大概率生成训练数据[14]。DBN一般由3层或3层以上神经元构成，神经元分为显性神经元和隐性神经元。显性神经元接受输入数据，隐性神经元提取数据的特征，其中每一个神经元代表数据向量的一维。与传统方法相比，DBN不仅有多隐层的深度结构，而且通过逐层训练学习以获取特征，能够刻画出数据更丰富的内在信息，使分类和预测更加容易[15]。
DBN模型如图1所示，第一层为输入数据的可见层，输入不同类型汽油光谱特征向量，数据经过2个隐层逐层训练后到达最后Softmax分类器，Softmax分类器输出汽油种类。
DBN模型中核心部分是RBM，RBM是一种层内无连接、层间全连接的两层神经网络[16]，其结构如图2所示。
图2中，ai和bj分别为可见层神经元和隐层神经元的偏置值，wij 为
层间相连的神经元的权值。 RBM中状态（v，h）的能量函数如（7）式所示，其函数值越小，则表示此时的RBM处于理想状态，汽油类型鉴别的错误率也就越低。
$$E\left\{ {v,\left( {h\left| \theta \right.} \right)} \right\} = - \displaystyle\sum\limits_{i = 1}^n {{a_i}} {v_i} - \displaystyle\sum\limits_{j = 1}^m {{b_j}} {h_j} - \displaystyle\sum\limits_{i = 1}^n {\displaystyle\sum\limits_{j = 1}^m {{v_i}} } {w_{ij}}{h_j}$$ (7) 此时，RBM的可见层与隐层对应的神经元激活概率可以表示为（8）式和（9）式：
$$p\left\{ {{h_j} = \left( {1\left| v \right.} \right),\theta } \right\} = \sigma \left( {{b_j} + \displaystyle\sum\limits_i {{v_i}{w_{ij}}} } \right)$$ (8) $$p\left\{ {{v_j} = \left( {1\left| v \right.} \right),\theta } \right\} = \sigma \left( {{a_i} + \displaystyle\sum\limits_j {{v_j}{w_{ij}}} } \right)$$ (9) 式中，σ为sigmoid激活函数，计算方法如（10）式所示：
$$\sigma \left( x \right) = \dfrac{1}{{1 + {e^{ - x}}}}$$ (10) 为了提高DBN模型的训练速度，Hionton等人提出了通过对比散度算法（CD-K）来构建可见层节点概率分布，其发现当K=1时，即只进行一步Gibbs采用便获得比较好的学习效果[17]。
2 结果与讨论
2.1 透射光谱分析
汽油样品红外光谱图如图3所示，从中可以看出，不同型号汽油样品的红外光谱大致相同，很难用肉眼进行区分。但红外光谱记录物质分子振动情况，而分子振动频率取决于组成原子的质量、化学键以及物质内部结构基团，所以原子的种类和结构基团的组合都可以在红外光谱图上表现出来，即不同物质的吸收谱带也不相同[18]。因此，借助化学计量学的方法可以对不同型号的汽油进行聚类分析。
2.2 原始光谱预处理分析
部分汽油样品原始光谱数据经预处理后的光谱图如图4所示。其中，导数处理虽然可以有效地消除基线和其他背景干扰，使某些未分辨开的重叠光谱分辨开，但是会引入噪声，降低信噪比[19]。MSC主要是消除由于颗粒分布不均匀及颗粒大小不同产生的散射对光谱的影响，其认为每条光谱与“理想光谱”都成线性关系，但在大多数情况下这种情形并不存在，而且光散射引起的背景非常复杂，仅靠校正集的平均光谱作为标准光谱是存在误差的[20]。SNV主要用来消除固体颗粒大小、表面散射以及光程变化对光谱的影响，但是假设乘法效应在整个光谱范围内是均匀的，并不一定能实现[21]。因此，本文所选择的3种预处理方法都有各自优劣之处，需要进一步分析来选择最适合本文的预处理方法。
为确定最适合本文的预处理方法，将MSC、SNV和一阶导数预处理后的光谱数据利用t-SNE算法进行数据降维并将前3个特征向量进行可视化处理，最终得出的结论为经MSC预处理后的4种汽油光谱特征数据不仅各自聚集在一起，而且还互不相交，能够很好地将这4种汽油区分开。因此选择多元散射校正作为建模前的原始光谱数据预处理方法。
2.3 光谱数据降维方法比较及分析
为验证所选择的t-SNE算法具有一定的优越性，因此将PCA算法与t-SNE算法提取到的汽油光谱特征进行特征可视化图以比较分类效果。
选择累积贡献率超过90%的前10个特征代表汽油光谱特征，即将汽油红外光谱数据维度降至10维，将其前3个特征向量进行可视化，结果如图5所示。
由图5可以看出，经PCA算法提取的汽油光谱特征数据分类效果比较差，这是因为汽油的红外光谱中含有非线性特征信息，而PCA属于线性降维方法，不能准确提取红外光谱数据中的非线性特征信息，从而导致光谱数据在降维的过程中部分有用信息丢失，造成模型鉴别精准度下降。
2.4 DBN模型的建立及对比分析
DBN模型中的迭代次数和网络深度会对模型预测精准度产生较大的影响，以模型最终的预测精准度为判断标准来确定其参数。
DBN结构主要分为输入层、隐含层和输出层，其网络深度主要体现在隐含层数量上。又因为t-SNE将汽油样品光谱数据降维至5维，因此将输出层节点数设置为5，输出层神经元的个数需要根据成品油分类数量决定，需要将92#、95#、98#以及掺混成品油区分开，因此输出层神经元的节点数设置为4。为了确定最合适的模型迭代次数，分别将迭代次数设置为50、60、70、80、90、100、110、120、130、140、150和160进行模型构建，当迭代次数为100时，DBN网络模型的识别准确度最高，而其他迭代次数的识别准确度都低于迭代次数为100时的识别准确度，将DBN网络模型的迭代次数设置为100。为了确定模型的最佳隐含层数量，分别建立1至4层隐含层的DBN网络模型，以比较不同隐含层数对模型预测精准度影响。当隐含层数设置为1时，DBN网络模型的识别准确度仅为75%，当隐含层数量增加到2时，DBN模型的识别准确度相较一个隐含层时有较大的提升，识别准确度已到达92.5%，然而继续增加网络模型的隐含层数时，其模型识别度开始降低。因此，所建立的DBN网络模型的隐含层数量为2。因此，构建一个结构为5-10-20-4的DBN网络模型对4种类型汽油样本进行特征学习和分类。
为了进一步验证分类算法有效性，分别利用深度置信网络算法与极限学习机算法建立汽油鉴别模型并比较这两种模型在测试集中的鉴别精准度。ELM模型的识别精准度为80%，而DBN模型的识别精准度为92.5%。由此可见，DBN 模型分类效果更加良好。这是由于采用非线性算法t-SNE对光谱数据进行降维处理，降低了数据在降维过程中有用信息丢失的可能性，再者DBN模型拥有更深层次的网络学习结构，训练网络时采用反向传播微调方法，使得训练后的网络具有更好的识别能力，因而汽油种类鉴别精准度更高。
3 结论
本文提出了一种t-SNE和DBN二者相结合的汽油种类鉴别方法。在对汽油原始光谱数据进行多元散射校正预处理后，利用t-SNE算法对预处理后的光谱数据进行降维以提取光谱特征信息，最后将光谱特征信息作为DBN网络的输入并构建汽油种类鉴别模型，通过在MATLAB上进行测试，最终的实验结果表明优选的方法具有更好的鉴别效果。
-
![]()
图 3 光纤环结构示意图和横截面视图
Figure 3. Schematic diagram and cross-sectional view of fiber-optical ring structure
![]()
图 5 RVE模型在拉伸与剪切载荷下的应力和位移云图
Figure 5. Stress and displacement cloud diagram of RVE model under tensile and shear loads
![]()
图 6 +X向10 g光纤环组件的有限元模型和总形变图
Figure 6. Finite element model and total deformation diagram of fiber-optical ring assembly structure at 10 g in + X direction
![]()
图 7 +X向10 g 3种不同接触效应的光纤环总形变和接触压力图
Figure 7. Total deformation and contact pressure of optical fiber ring with three contact effects from 10 g in + X direction
![]()
图 8 +Y向10 g和−Y向10 g的光纤环总形变
Figure 8. Total fiber ring deformation of 10 g in + Y direction and 10 g in − Y direction
![]()
图 9 +X向10 g、+X向6 g和+X向2 g含接触效应的光纤环上表面总位移
Figure 9. Total displacement of upper surfaces of optical fiber ring with contact effect at 10 g in + X direction, 6 g in + X direction and 2 g in + X direction
![]()
图 10 +Y向10 g、+Y向6 g和+Y向2 g含接触效应的光纤环上表面总位移
Figure 10. Total displacement of upper surfaces of optical fiber ring with contact effect at 10 g in + Y direction, 6 g in + Y direction and 2 g in + Y direction
![]()
图 11 −Y向10 g、Y向6 g和−Y向2 g含接触效应的光纤环上表面总位移
Figure 11. Total displacement of upper surfaces of optical fiber ring with contact effect at 10 g in − Y direction, 6 g in Y direction and 2 g in −Y direction
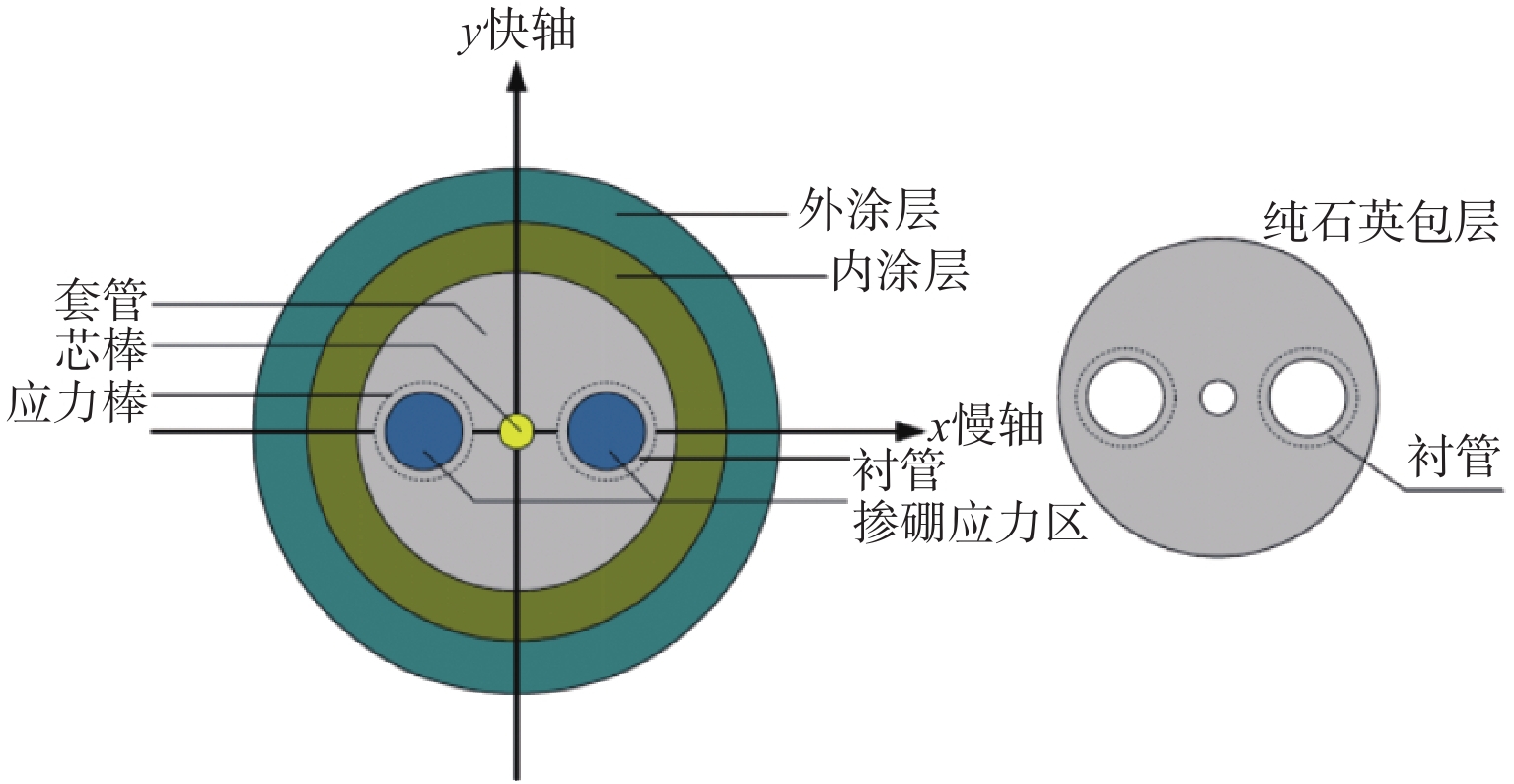
表 1 光纤所含材料参数
Table 1 Material parameters contained in fiber-optical
名称 弹性模量 E/MPa 泊松比 μ 密度 ρ/kg·m−3 光纤内涂层 2 0.49 1 179 光纤外涂层 1 200 0.33 1 179 石英 73 103.448 28 0.18 2 193.5 胶粘剂 584.62 0.3 1 179 
下载: 导出CSV
表 2 本文与算例的B/Al复合材料等效弹性性能参数计算结果对比
Table 2 Comparison of calculation results of effective elastic performance parameters of B/Al composites in this paper and reference
参数 Et1/GPa Ea/GPa Et2/GPa Ga1/GPa Ga2/GPa Gt/GPa vt1 vt2 va 算例 143.9 215 143.9 54.34 54.34 45.8 0.255 0.255 0.195 本文 145 215 139 54.2 54.2 45.5 0.251 0.223 0.165
下载: 导出CSV
表 3 光纤环等效材料参数
Table 3 Equivalent material parameters of fiber-optical ring
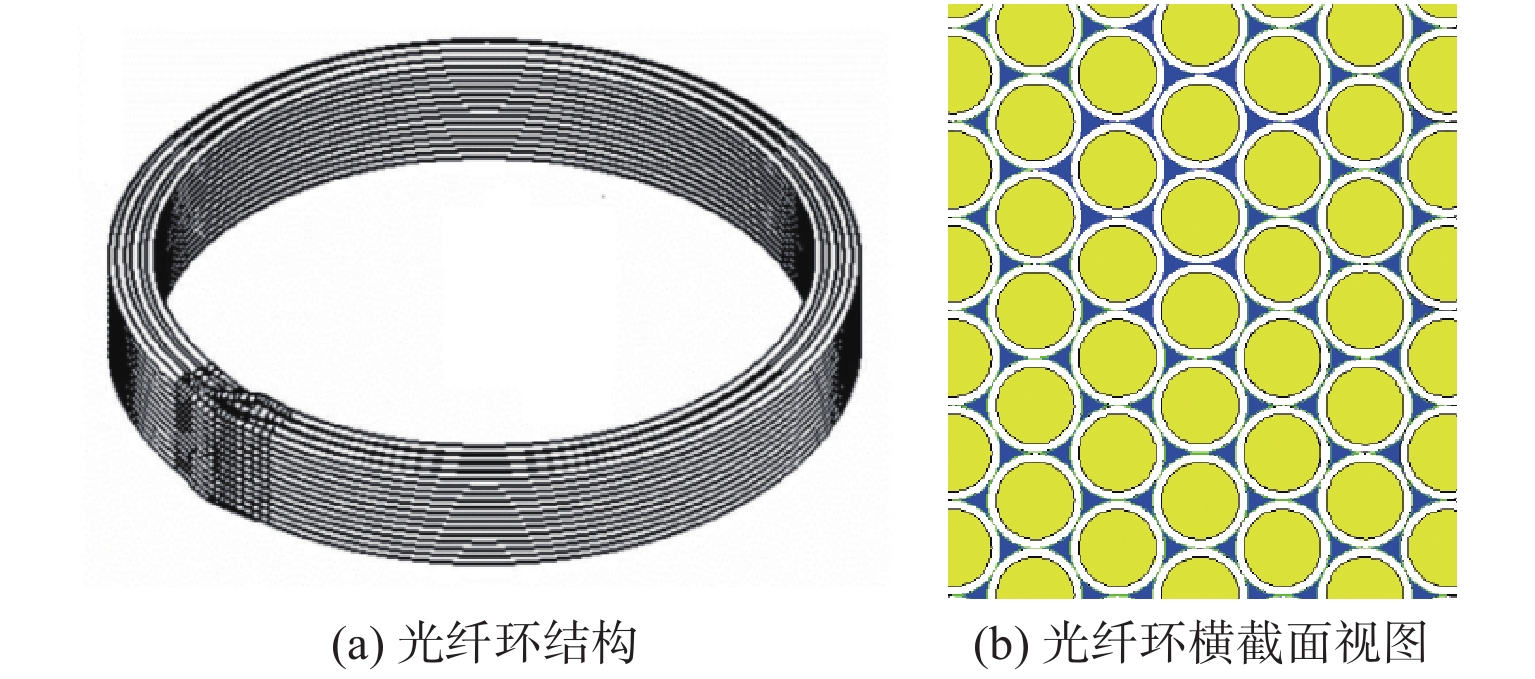
参数 值 参数 值 E1/GPa 0.262 G31/GPa 0.0654 E2/GPa 23.7 v12 0.004682 E3/GPa 0.214 v23 0.145771 G12/GPa 0.102 v31 0.317134 G23/GPa 0.102 密度/kg·m−3 1501.8148
下载: 导出CSV
-
[1] 杨盛林, 马林, 陈桂红, 等. 4J32芯轴式环圈骨架对光纤陀螺性能的改善[J]. 中国惯性技术学报,2016,24(1):88-92. YANG Shenglin, MA Lin, CHEN Guihong, et al. Improvement on FOG performance by 4J32 skeleton of mandrel coil[J]. Journal of Chinese Inertial Technology,2016,24(1):88-92.
[2] 王新龙, 马闪. 光纤陀螺随机漂移误差补偿适用性方法[J]. 北京航空航天大学学报,2008,34(6):681-685. WANG Xinlong, MA Shan. Applicability compensation method for random drift of fiber optic gyroscopes[J]. Journal of Beijing University of Aeronautics and Astronautics,2008,34(6):681-685.
[3] 高鑫, 严吉中, 谢良平, 等. 光纤陀螺的角加速度误差分析与实验研究[J]. 压电与声光,2013,35(6):821-823. GAO Xin, YAN Jizhong, XIE Liangping, et al. Analysis and experimental research on angular acceleration error of fOG[J]. Piezoelectrics & Acoustooptics,2013,35(6):821-823.
[4] 舒建涛, 李绪友, 吴磊. 高精度光纤陀螺振动误差抑制技术[J]. 红外与激光工程,2011,40(11):2201-2206. doi: 10.3969/j.issn.1007-2276.2011.11.026 SHU Jiantao, LI Xuyou, WU Lei. Vibration error restrain technology for high-precision fiber optic gyroscope[J]. Infrared and Laser Engineering,2011,40(11):2201-2206. doi: 10.3969/j.issn.1007-2276.2011.11.026
[5] 苏晓东, 宋蔚阳, 吴昭辉, 等. 光纤陀螺跟踪角加速度模型建模与研究[J]. 电子技术与软件工程,2019(5):169-171. SU Xiaodong, SONG Weiyang, WU Zhaohui, et al. Modeling and research on tracking angular acceleration model of fiber optic gyroscope[J]. Electronic Technology & Software Engineering,2019(5):169-171.
[6] 高鑫, 严吉中, 谢良平, 等. 光纤陀螺在摇摆状态下的误差建模与补偿[J]. 光电工程,2013,40(5):58-63. GAO Xin, YAN Jizhong, XIE Liangping, et al. Error Modeling and Compensation of FOG in Swaying Motion[J]. Opto-Electronic Engineering,2013,40(5):58-63.
[7] 王锴, 冯喆, 刘淑荣, 等. 电磁干扰对光纤陀螺零偏的影响分析及验证: 中国惯性技术学会2019科技工作者研讨会[C]. 昆明: 中国惯性技术学会, 2019: 42-44. WANG Kai, FENG Zhe, LIU Shurong, et al. Analysis and verification of the influence of electromagnetic interference on the bias of fiber optic gyroscope[C]//China Institute of Inertial Technology 2019 Symposium for Scientific and Technical Workers. Kunming, Yunnan, China: Society of Chinese Inertial Technology, 2019: 42-44.
[8] 周闻青, 费宇明, 洪桂杰, 等. 高精度光纤陀螺零位误差的磁温特性研究[J]. 应用光学,2020,41(1):220-226. ZHOU Wenqing, FEI Yuming, HONG Guijie, et al. Error modeling and compensation of FOG in swaying motion[J]. Journal of Applied Optics,2020,41(1):220-226.
[9] 宋昱寰, 管练武, 高延滨, 等. 光路偏振串扰误差对闭环光纤陀螺精度影响[J]. 哈尔滨工程大学学报,2020,41(8):1210-1215. SONG Yuhuan, GUAN Lianwu, GAO Yanbin, et al. Influence of polarization crosstalk error of optic path on the accuracyof a closed-loop fiber optic gyroscope[J]. Journal of Harbin Engineering University,2020,41(8):1210-1215.
[10] 白俊卿, 张科, 卫育新. 光纤陀螺随机漂移建模与分析[J]. 中国惯性技术学报,2012,20(5):621-624. doi: 10.3969/j.issn.1005-6734.2012.05.024 BAI Junqing, ZHANG Ke, WEI Yuxin. Modeling and analysis of fiber optic gyroscope random drifts[J]. Journal of Chinese Inertial Technology,2012,20(5):621-624. doi: 10.3969/j.issn.1005-6734.2012.05.024
[11] 梁霄, 魏天啸, 谢良平. 闭环光纤陀螺精度性能提升方法研究[J]. 红外技术,2019,41(9):819-923. LIANG Xiao, WEI Tianxiao, XIE Liangping. Research on precision enhancement of closed-loop FOGs[J]. Infrared Technology,2019,41(9):819-923.
[12] 潘雄, 张春生, 王夏霄, 等. 反馈延迟对光纤陀螺振动误差特性的影响[J]. 红外与激光工程,2014,43(8):2607-2612. doi: 10.3969/j.issn.1007-2276.2014.08.035 PAN Xiong, ZHANG Chunsheng, WANG Xiaxiao, et al. Impact on vibration error characteristics of FOG with feedback delay[J]. Infrared and Laser Engineering,2014,43(8):2607-2612. doi: 10.3969/j.issn.1007-2276.2014.08.035
[13] 姜梦茹, 李彦, 侯智懿, 等. 光纤环温度特性对光纤陀螺零偏稳定性的影响[J]. 激光杂志,2018,39(11):39-42. JIANG Mengru, LI Yan, HOU Zhiyi, et al. The influence of the temperature characteristics of the fiber ring on the bias stability of the fiber optic gyroscope[J]. Laser Journal,2018,39(11):39-42.
[14] 杨远洪, 伊小素, 孟照魁, 等. 光纤陀螺用光纤环的应力分布实验研究[J]. 压电与声光,2005,27(2):98-101. doi: 10.3969/j.issn.1004-2474.2005.02.004 YANG Yuanhong, YI Xiaosu, MENG Zhaokui, et al. Experimental study on strain distribution in fiber coil used in fiber optic gyroscope[J]. Piezoelectrics & Acoustooptics,2005,27(2):98-101. doi: 10.3969/j.issn.1004-2474.2005.02.004
[15] 王海波, 周爱军, 陈荣娟, 等. 惯性器件原理与应用[M]. 1版. 长春: 吉林大学出版社, 2017: 16. WANG Haibo, ZHOU Aijun, CHEN Rongjuan, et al. Principle and application of inertial devices[M]. 1st ed.Changchun: Jilin University Press, 2017: 16.
[16] 张超, 许希武, 严雪. 纺织复合材料细观力学分析的一般性周期性边界条件及其有限元实现[J]. 航空学报,2013,34(7):1636-1645. ZHANG Chao, XU Xiwu, YAN Xue. General periodic boundary conditions and their application to micromechanical finite element analysis of textile composites[J]. Acta Aeronautica et Astronautica Sinica,2013,34(7):1636-1645.
[17] 吕毅, 吕国志, 孙龙生. 基于有限元计算细观力学的RVE库的建立与应用[J]. 机械强度,2009,31(5):851-856. LYU Yi, LYU Guozhi, SUN Longsheng. Construction and application of RVE library based on finite element computation micromechanics[J]. Journal of Mechanical Strength,2009,31(5):851-856.
[18] 雷友锋, 魏德明, 高德平. 细观力学有限元法预测复合材料宏观有效弹性模量[J]. 燃气涡轮试验与研究,2003,16(3):11-15, 18. LEI Youfeng, WEI Deming, GAO Deping. Predicting macroscopic effective elastic moduli of composites by micro-mechanics FEM[J]. Gas Turbine Experiment and Research,2003,16(3):11-15, 18.
[19] SMIT R J M, BREKELMANS W A M, MEIJER H E H. Prediction of the large-strain mechanical response of heterogeneous polymer systems: local and global deformation behaviour of a representative volume element of voided polycarbonate[J]. Journal of the Mechanics and Physics of Solids,1999,47(2):201-221. doi: 10.1016/S0022-5096(98)00089-1
[20] 沙云东, 丁光耀, 田建光, 等. 纤维增强复合材料力学性能预测及试验验证[J]. 航空动力学报,2018,33(10):29-37. SHA Yundong, DING Guangyao, TIAN Jianguang, et al. Prediction and test verification of mechanical properties of fiber reinforced composites[J]. Journal of Aerospace Power,2018,33(10):29-37.
[21] 王耀先. 复合材料力学与结构设计[M]. 1版.上海: 华东理工大学出版社, 2012: 65. WANG Yaoxian. Composite material mechanics and structure design[M]. 1st ed.Shanghai: East China University of Science and Technology Press, 2012: 65.
-
期刊类型引用(1)
1. 伍秋林，冯新凯，陈怀熹，陈家颖，马翠坪，梁万国. 基于紧凑型Nd∶YAG/PPMgLN模组的561 nm基模激光器. 人工晶体学报. 2024(09): 1512-1518 . 
百度学术
其他类型引用(4)
计量
- 文章访问数: 653
- HTML全文浏览量: 416
- PDF下载量: 26
- 被引次数: 5
陕公网安备 61011302001501号 