Distortion correction research by gravity for 300-mm-aperture flat based on multi-point adjustable support
-
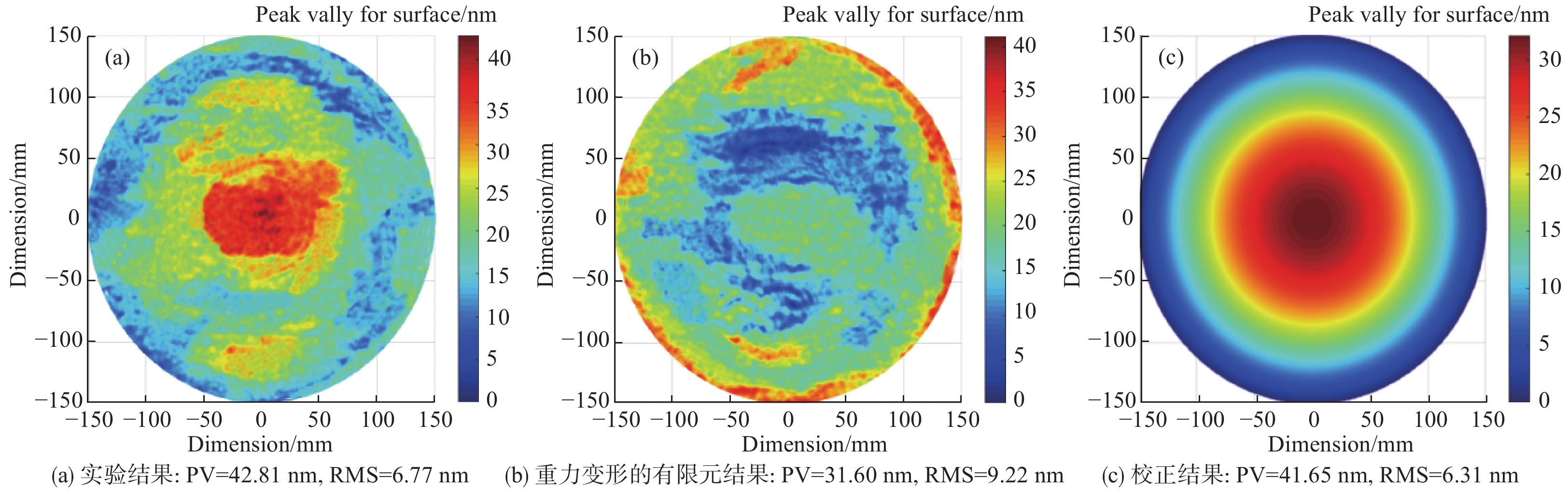
摘要: 在高精度光学元件领域，特别是大口径的平面光学元件，随着对测试精度和口径要求的提高，重力变形成为其不可避免的问题。介绍了立式斐索型干涉仪的系统设计和多点可调支撑方法的变形理论，通过有限元分析（FEM），优化得到了合理的支撑结构。采用液面基准，在300 mm口径的立式斐索型干涉仪上进行了平面的重力变形校正。与三平面法检测结果比较，两者的残差峰谷（PV）值为10.20 nm，均方根（RMS）值为1.56 nm。Abstract: In the field of high-precision optical elements, especially the planar optical elements with large aperture, the deformation by gravity becomes an inevitable problem with the improvement of requirements for higher measuring accuracy and larger aperture. The system design of vertical Fizeau interferometer and the deformation theory of multi-point adjustable support method were introduced.By finite element method (FEM) analysis, the reasonable support structure is optimized and derived. Calibration of deformation of flat by gravity was obtained on 300-mm-diameter vertical Fizeau interferometer with liquid surface datum. Comparing it with detection result by three-flat method, the residual between two methods can reach 10.20 nm for peak valley (PV), and 1.56 nm for root mean square (RMS).
-
引言
自20世纪末以来，国内外科研人员通过理论和实验对水下人造目标识别的可行性进行探索，曹念文[1]等人验证了用圆偏振和线偏振技术能够大大提高水下目标成像的图像清晰度和图像分辨率。秦琳[2]等人结合距离选通技术和偏振成像技术的各自特点，提出基于距离选通的偏振成像方式来抑制后向散射光的干扰，从而提高图像对比度。赵泓扬[3]等人通过暗原色先验知识对图像进行背景区域划分，结合光强和偏振度计算出各像素点的后向散射光强，进行融合处理可以精确得到水下目标深度信息。韩捷飞[4]等人设计了一套基于LED辅助照明的偏振成像实验系统，针对不同性质的目标，可以通过恰当的处理方式获得高分辨率的图像。王马华[5]等人结合折射率谱，改进水下湍流退化模型来提高复杂水流环境下水下退化图像复原方法。管今哥[6]等人通过分析偏振差分探测原理建立偏振差分模型，提高了水下物体探测与识别的效率。李明杰[7]等人对激光水下偏振成像的特征融合处理，提高了激光成像的识别能力。以上研究大多是围绕偏振成像的获取以及图像清晰度提高的研究，而缺乏从本质上分析水下目标物的偏振成像特性。本文从偏振分量角度分析图像的偏振特性，探究目标物在水下的偏振成像特性。
理论上, 利用已有的斯托克斯矢量对偏振态进行描述, 给出了最终识别目标的测量计算方法。模拟水下环境, 通过SALSA采集包含偏振信息的目标图像并与强度图像的效果比较。利用Matlab软件对图像进行处理, 计算比较不同材料在各试验场景下DOP、DOLP(线偏振度)、DOCP(圆偏振度)及强度图像，量化结果显示偏振成像与强度成像的差异。通过识别不同材料的目标, 发现其图像中包含不同的偏振特性, 对水下探测目标物具有深远意义，未来可以应用于水下复杂背景下对敌潜艇等军事目标侦测、识别和搜救工作。
1 偏振成像的基本原理
一般来说，完整地描述偏振光的状态需要3个参数：光强、偏振度和偏振角。光强描述了光矢量的平均振动幅度; 偏振度描述了完全偏振光强度在整个光强中的比例; 偏振角描述了偏振光的振动方向。对于部分偏振光，通常将其分解成完全偏振光与非偏振光之和的形式，即部分偏振光的斯托克斯矢量为
$$ S_{部分}=\left(\begin{array}{c} \sqrt{Q^{2}+U^{2}+V^{2}} \\ Q \\ U \\ V \end{array}\right)+\left(\begin{array}{c} I-\sqrt{Q^{2}+U^{2}+V^{2}} \\ 0 \\ 0 \\ 0 \end{array}\right) $$ 一束光经过光学系统后，入射光的斯托克斯矢量Sin与出射光的斯托克斯矢量Sout之间有如下关系：
$$ S_{\text {out }}=M \cdot S_{\text {in }} $$ 式中M表示穆勒矩阵，用其表述光学系统中元器件特性，若其经过n个器件，则有：
$$ S_{\text {out }}=M_{n} M_{n-1} \cdots M_{2} M_{1} \cdot S_{\text {in }} $$ 当偏振片的偏振方向与水平方向的夹角为θ时，此时偏振片的穆勒矩阵Mθ可以表示为
$$ M_{\theta}=\frac{1}{2}\left[\begin{array}{cccc} 1 & \cos 2 \theta & \sin 2 \theta & 0 \\ \cos 2 \theta & \cos ^{2} 2 \theta & \cos 2 \theta \sin 2 \theta & 0 \\ \sin 2 \theta & \cos 2 \theta \sin 2 \theta & \sin ^{2} 2 \theta & 0 \\ 0 & 0 & 0 & 0 \end{array}\right] $$ 于是，斯托克斯矢量表达式为
$$ \left[\begin{array}{l} I \\ Q \\ U \\ V \end{array}\right]=\left[\begin{array}{c} I^{\prime}\left(0^{\circ}\right)+I^{\prime}\left(90^{\circ}\right) \\ I^{\prime}\left(0^{\circ}\right)-I^{\prime}\left(90^{\circ}\right) \\ I^{\prime}\left(45^{\circ}\right)-I^{\prime}\left(-45^{\circ}\right) \\ I_{r}^{\prime}-I_{t}^{\prime} \end{array}\right]=\left[\begin{array}{c} I^{\prime}\left(0^{\circ}\right)+I^{\prime}\left(90^{\circ}\right) \\ 2 I^{\prime}\left(0^{\circ}\right)-I \\ 2 I^{\prime}\left(45^{\circ}\right)-I \\ 2 I_{r}^{\prime}-I \end{array}\right] $$ 式中I′(0°)，I′(0°)，I′(0°)，I′(0°)为在不同角度下探测到的线偏振光强。
偏振度(degree of polarization, DOP)指偏振光在总光强中所占的比例或光束中偏振部分的光强度和整个光强度之比，表达式为
$$ \mathrm{DOP}=\frac{\sqrt{Q^{2}+U^{2}+V^{2}}}{I} $$ 反射光中线偏振度(degree of linear polarization, DOLP)为${\rm{DOLP}} = \frac{{\sqrt {{Q^2} + {U^2}} }}{I} $，圆偏振度(degree of circular polarization, DOCP) $ {\rm{DOCP}} = |\frac{V}{I}|$。
2 水下目标偏振成像实验
2.1 实验设备
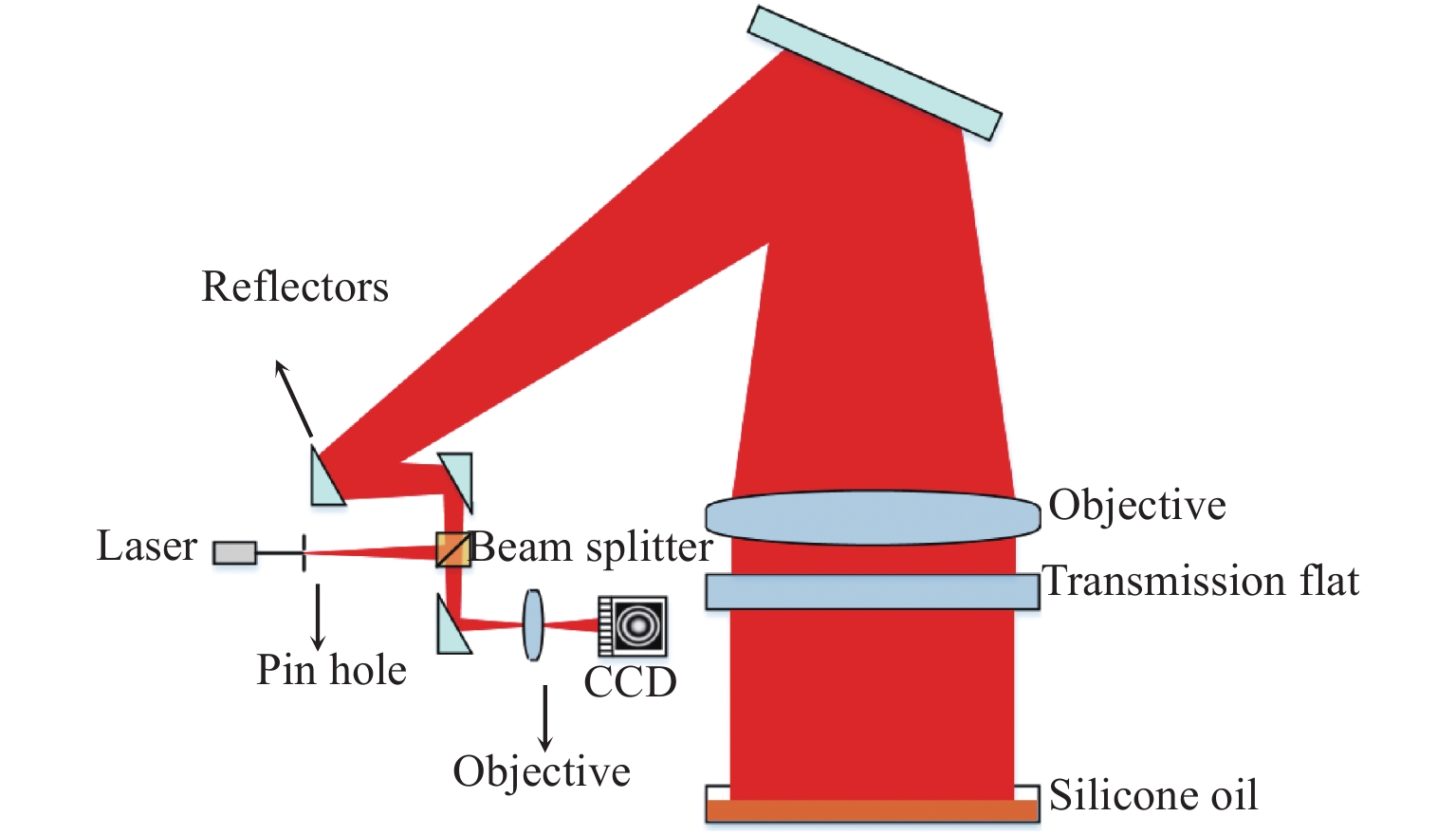
实验需要用到的元器件及材料有：SALSA相机、滤光片、偏振片、镜头、目标物、计算机等。实验采用SALSA偏振相机，结合专用软件实现对目标进行偏振成像，并获得强度图像I、线偏振度DOLP、圆偏振度DOCP和偏振度DOP。此外，实验采用长宽高100 cm*45 cm*45 cm的长方形水槽，并将四周用黑布完全遮住，避免内壁反射光的影响。图 1为偏振成像的实验示意图。
2.2 实验方法
为了探测不同材质的目标物在复杂水下的偏振成像特性，需要对各类金属片(如铁、铜、铝等)、塑料、木头，泡沫等共同进行偏振成像。实验时，摆放好仪器设备，将水流注入到水槽中达到水深45 cm时停止注水，注水时长0.5 h。将SALSA相机垂直固定于水面上，并将目标物放置于水下40 cm深处，将相机镜头对准水面的目标物，调节光圈、焦距使成像清晰。本次实验在水下进行，分别以黄铜、紫铜、铁、铝作为目标物。对目标物在清水条件下进行偏振成像，得到强度I图像以及DOP、DOLP、DOCP图像。然后同样将其他材料和金属片一同放到拍摄效果最佳的区域进行数据采集。采集图像时，分别将绿色、蓝色、红色3种不同波段的滤光片加到镜头前分别进行拍摄。通过计算图像的DOP、DOCP、DOLP大小综合比较选择成像效果最佳的波段; 调节水下目标物的深度，计算偏振度对比度与强度对比度显示探测不同深度下偏振成像效果。选择偏振成像效果最佳的深度，适当改变水体的浑浊度，对所成图像提取熵值，根据所包含的信息量判断成像效果，根据不同浓度下目标物DOP大小，探究不同浑浊度的水体对偏振成像的影响。分析对比偏振图像，总结复杂水下目标物的偏振成像特性。图 2是数据采集场景图。
3 实验结果分析
3.1 对目标进行不同波段的偏振成像
偏振成像具有很多强度成像所不具备的优势，在某种特定背景下能够更加突显目标。在清水条件下，分别给镜头加红(波长620 nm~750 nm)、绿(波长495 nm~570 nm)、蓝(波长476 nm~495 nm)3种不同的滤光片对深度为40 cm的目标物进行偏振成像。根据先验知识：由于水体吸收和散射具有显著的波长选择性，不同波段水下偏振成像，其成像效果也存在显著的差异。图 3是一组3种波段的强度图像，图 4是对应的偏振度图像，发现偏振度图像比强度图像对比度高，边缘轮廓更加清晰，尤其在蓝色波段的图像最为清晰，因为该波段水体吸收相对较小。图 5是3种波段的DOP、DOLP、DOCP比较图，可以看出：当光照射到光滑的目标物时，其DOCP在红色波段和绿色波段都趋于0，说明反射光中的偏振光以线偏振光为主。而在蓝色波段下，圆偏振光却比其他2种波段下所占比例更重，最多可达近50%。在绿色和红色波段下，黄铜和紫铜的DOP都要高于铁的DOP，而在蓝色波段下，铁的DOP比黄铜和紫铜的DOP分别高出0.3和0.48。在可见光谱内，蓝色(476 nm~495 nm)在水中的衰减系数最小，穿透力最强，而金属目标物表面反射率较高，因此该波段范围内的偏振成像效果比较好，目标物较清晰。
![]()
图 5 三种波段下目标物的DOP、DOLP、DOCP比较图Figure 5. DOP, DOLP and DOCP comparison of objects in three bands3.2 不同材质的目标物在平静水面下的偏振成像特性
3.2.1 同一深度下, 不同材质的目标物
图 6为目标物偏振度图像。图 7为计算图 6所得各目标物的偏振度，可见铜和铁的偏振度较高，瓷片的DOP最高达0.8，而布片DOP最低仅0.12。这是因为布片表面反射率较高，辐射强度图像灰度值更大，光在经历多次反射或者散射，导致偏振度离散型变大，DOP值变小; 而金属等光滑表面较易形成镜面反射，使偏振度的离散程度变小，DOP值变大。因此，瓷片、PVC、金属等表面光滑的目标物在水下的偏振度平均要比木头、橡胶、布片这一类的目标物要高得多。
3.2.2 不同深度下，同材质的目标物
图 8为不同深度下各目标物在水下的偏振度对比度和强度对比度实验结果。(a)~(d)分别表示目标物在水下深度分别为10 cm，20 cm，30 cm，40 cm的偏振度对比度和强度对比度的计算结果。由此可以看出，随着深度的不断加大，目标物在水下的成像逐渐变小，对比度逐渐降低，如铁的偏振对比度从10.35%降低到9.24%，降低了1.11%;铁的强度对比度从4.86%降低到3.98%，降低了0.88%。但是在水深40 cm处，铁的偏振度对比度依然比强度对比度高5.26%，说明偏振度图像比强度图像更加清晰。而且由于强度图像受水体对光的吸收作用和水中粒子的散射作用，强度图像的成像效果会表现出更多的背景噪音。因此，相比于强度图像，偏振成像获取的图像轮廓更为清晰。
3.3 不同浑浊度的水下目标物偏振成像效果
配制牛奶浓度分别为0.35 mg/L、0.70 mg/L、1.05 mg/L、1.40 mg/L的浑浊水并与清水(牛奶浓度为0)对比，得到一系列随着牛奶浓度变化的水下目标物偏振成像结果，表 1中给出了不同泥沙浓度下，偏振分量S0、S1、S2、S3图像的信息熵值指标。强度图像在各种浓度下都表现出了比偏振分量图像包含更多的图像信息。
表 1 熵Table 1. Entropy偏振分量 浓度 0 mg/L 0.35 mg/L 0.70 mg/L 1.05 mg/L 1.40 mg/L S0 3.69 3.46 3.04 2.81 2.57 S1 3.14 2.95 2.64 2.12 1.81 S2 2.81 2.57 2.02 1.87 1.64 S3 1.83 1.52 1.33 1.05 0.92 图 9描述了不同的目标物在各浓度下的DOP的值变化情况，如瓷片的DOP在1.40 mg/L浑浊度水下仅降低了0.31，尽管牛奶浓度对偏振图像的影响比辐射强度图像大，但偏振图像仍然可以在高浑浊度水体中检测出目标物的边缘轮廓，且比传统辐射强度图像更为清晰; 牛奶浓度对水下偏振探测的影响主要体现在对光的散射作用上，牛奶浓度增大，水中粒子后向散射系数增大，图像背景噪声增强，目标物不清晰，根据图像的清晰度计算可知，偏振图像可以较好地提取水下物体的边界轮廓。
4 结论
分别从水下物体的材质、深度、观测波段、不同浑浊度水体等方面进行了偏振成像实验。在光谱蓝色波段(476 nm~495 nm)水体衰减系数最小，该波段下偏振图象中，铁片的DOP要明显高于其他目标物。其中，圆偏振光比其他波段下所占比重高50%，水下识别度也更高。不同材质的目标物在水下的偏振特性表现出较大的差异，金属片等光面的目标物DOP的值要普遍比布片等其他粗面的目标物要高出许多，依此可判断目标物的表面粗糙。随着深度的不断加深，强度图像的对比度明显下降，而偏振度图像的对比度下降缓慢，在较深的水下，目标物的偏振度对比度明显高于强度图像对比度强度。牛奶浓度对偏振图像的影响比辐射强度图像大，在浑浊的水下，目标物强度图像的熵值更高，但依然可以获得客观的偏振度。综上所述，偏振成像可以在复杂的水下获取信息量更大的偏振图像，对水下作业和探测资源有着更好的前景和发展。
-
![]()
图 2 立式斐索型干涉仪的三维结构
Figure 2. Tridimensional structure of vertical Fizeau interferometer
![]()
图 3 多点可调支撑的水平放置平晶
Figure 3. Optical flat of horizontal placement with multi-point adjustable support
![]()
图 4 不同因素影响下的300 mm范围内平晶的重力变形PV值
Figure 4. PV value of optical flat gravity deformation with 300 mm-aperture by different factors
![]()
图 6 72 h内的温度和湿度变化及测试实验室中的垂直振动
Figure 6. Temperature & humidity variation within 72 h and vertical vibration in test laboratory
![]()
图 7 在液面基准下的多点可调支撑法的结果图
Figure 7. Diagrams of multi-point adjustable support method under liquid surface datum
![]()
图 8 三平面法与多点可调支撑法的比较
Figure 8. Comparison of three-plane method and multi-point adjustable support method
表 1 所需材料的各项属性值
Table 1 Properties of required materials
材料 杨氏模量/GPa 泊松比 密度/（kg/m3） 石英 72.7 0.17 $2.{\rm{201}} \times {10^3}$ 铝合金 70.6 0.33 $2.7 \times {10^3}$ 聚乙烯塑料 3.2 0.35 $1.1{\rm{9}} \times {10^3}$ 
下载: 导出CSV
表 2 仿真参数
Table 2 Simulation parameters
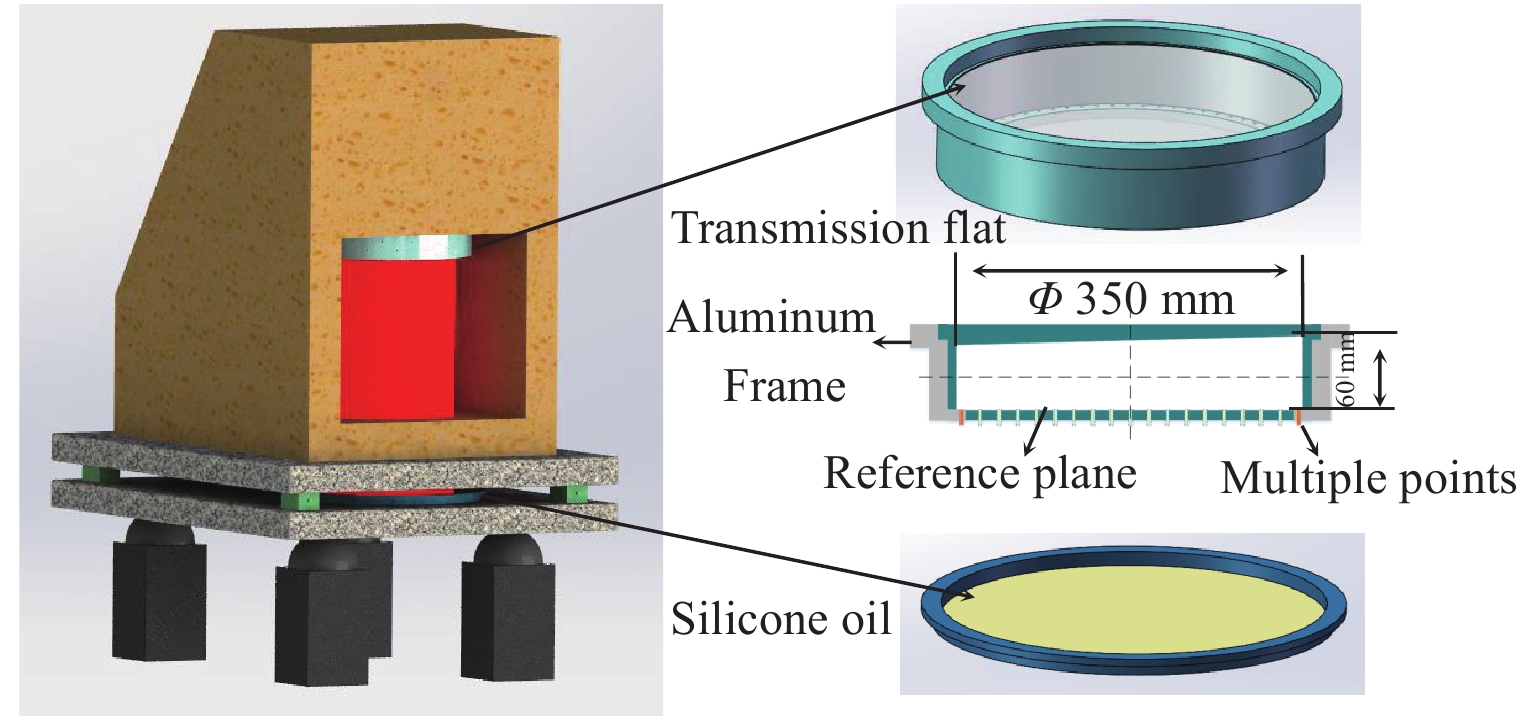
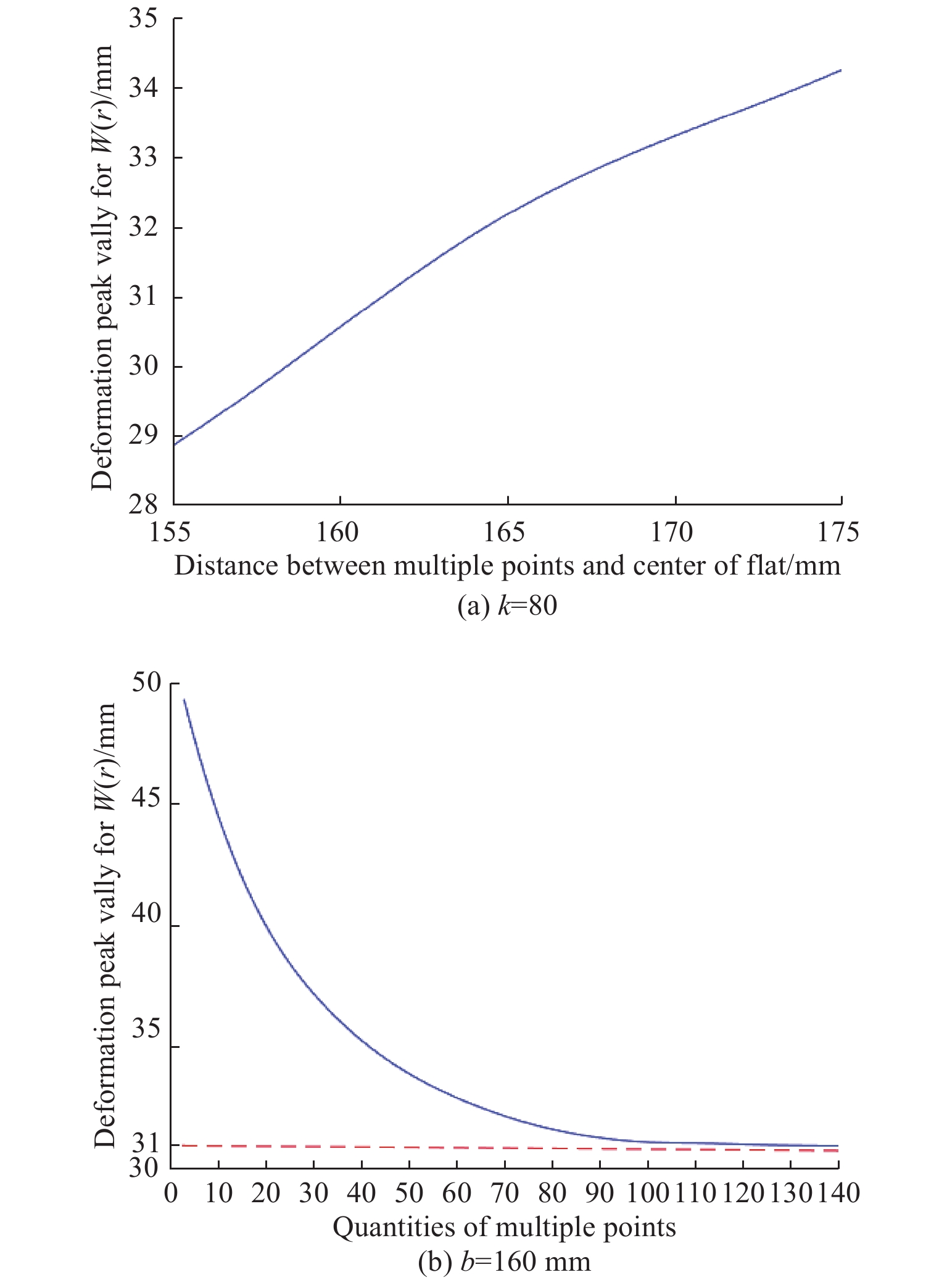
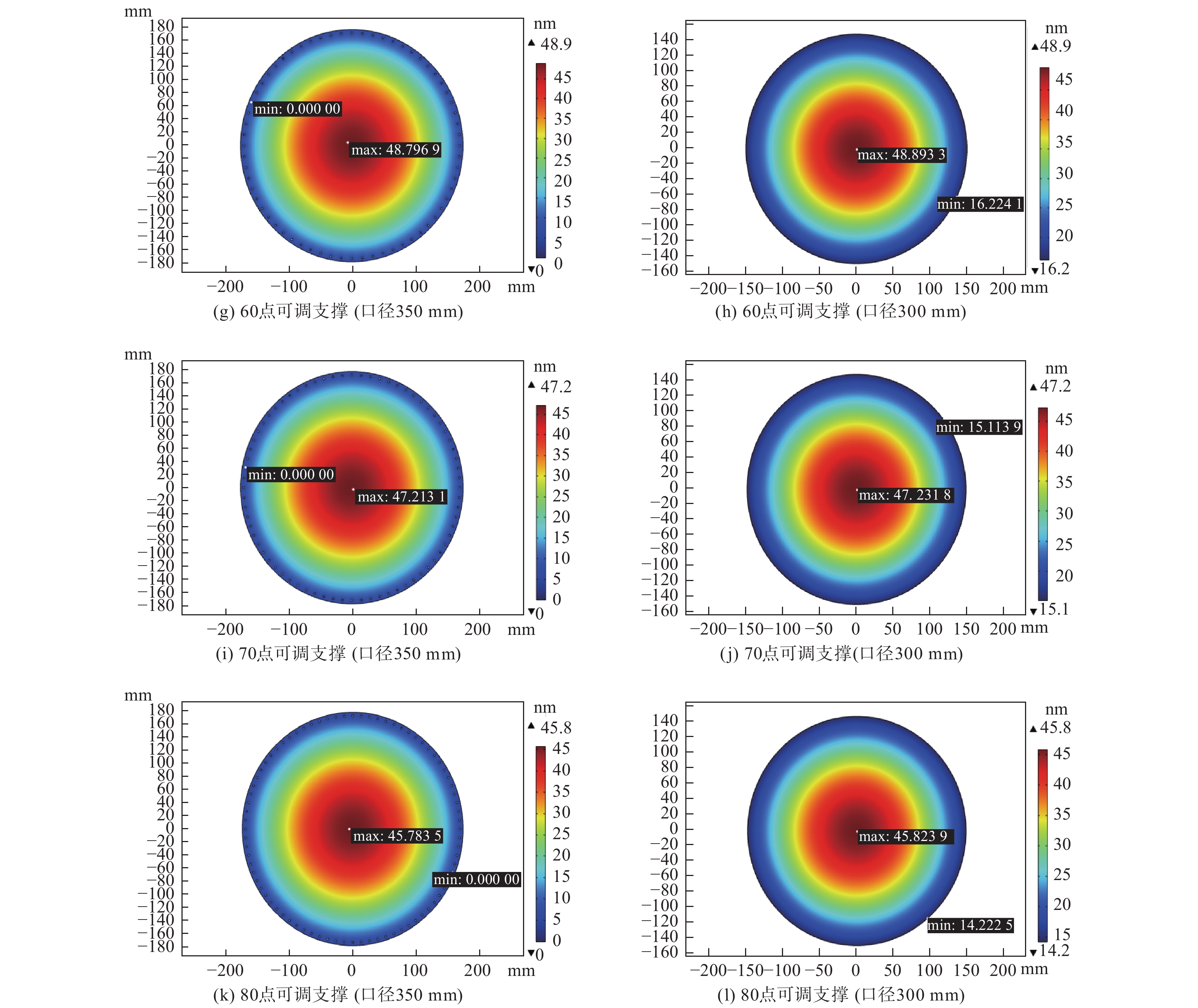
参数 量值 支撑点数量 ${\rm{80}}$ 单个支撑点直径 ${\rm{2}}{\rm{.5\;mm}}$ 支撑点距平晶中心的距离 ${\rm{160\;mm}}$ 支撑点分布方式 均匀分布 平晶直径 ${\rm{350\;mm}}$ 平晶厚度 ${\rm{60\;mm}}$
下载: 导出CSV
表 3 在不同口径下参考平面变形的PV值
Table 3 PV value of reference plane deformation under different apertures
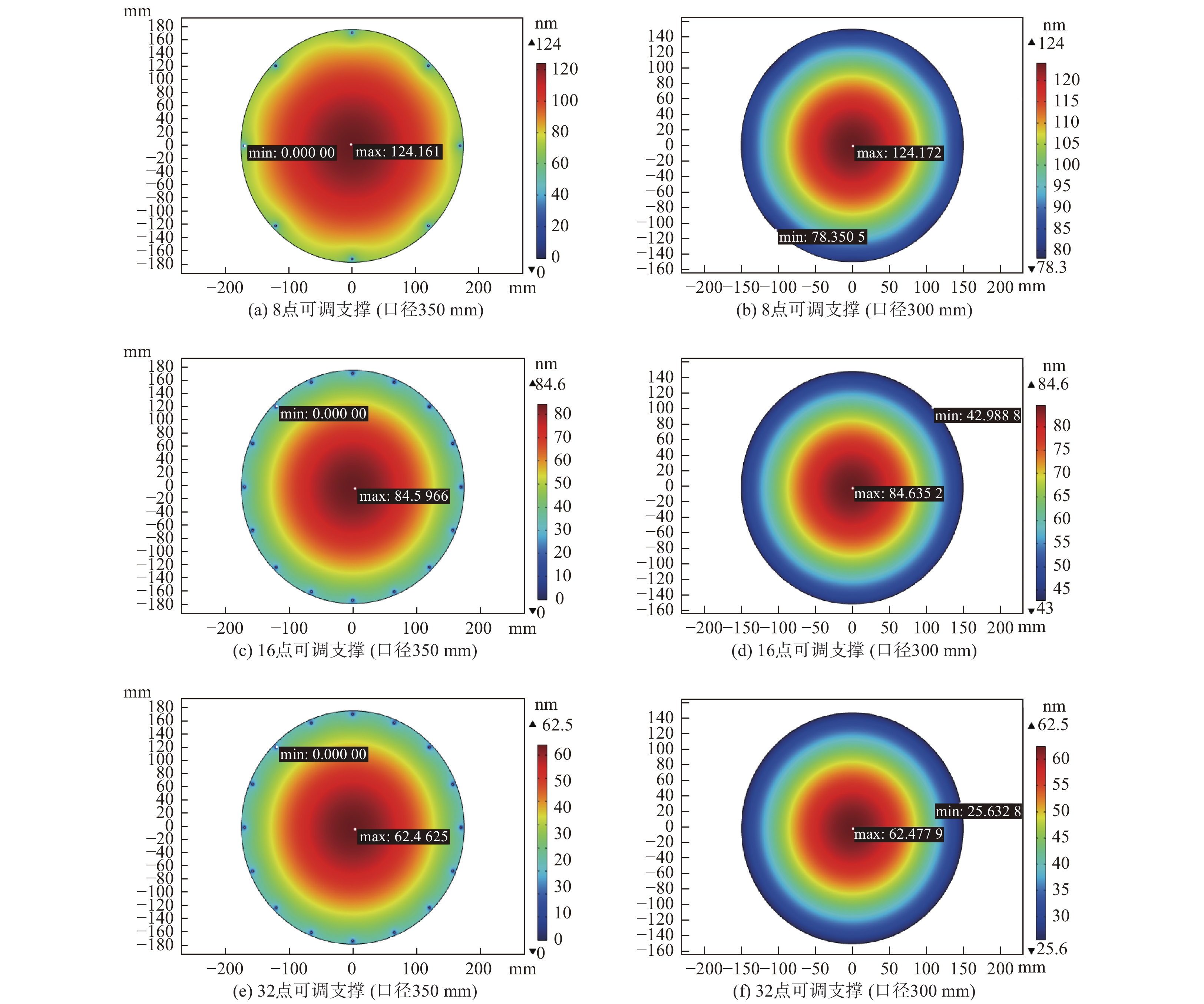
支撑方式 变形值PV（350 mm） 变形值PV（300 mm） 8点可调支撑 124.16 45.82 16点可调支撑 84.60 41.65 32点可调支撑 62.46 36.85 60点可调支撑 48.80 32.67 70点可调支撑 47.21 32.12 80点可调支撑 45.78 31.60
下载: 导出CSV
-
[1] EVANS C J. Certification, self-calibration, and uncertainty in testing flats[J]. Proceeding of SPIE,2010,76560:1-6.
[2] BURKE J, OREB B, NETTERFIELD R. Customized three-flat calibration method for a 320 mm aperture Fizeau interferometer with vertical optical axis[J]. Proc. ASPE,2005,31:1-6.
[3] SCHULZ M, EHRET G, KŘEN P. High accuracy flatness metrology within the European metrology research program[J]. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment,2013,710:37-41.
[4] SIEWERT F, BUCHHEIM J, ZESCHKE T, et al. On the characterization of ultra-precise X-ray optical components: advances and challenges inex situmetrology[J]. Journal of Synchrotron Radiation,2014,21(5):968-975. doi: 10.1107/S1600577514016221
[5] DEW G D. The measurement of optical flatness[J]. Journal of Scientific Instruments,1966,43(7):409-415. doi: 10.1088/0950-7671/43/7/301
[6] International Bureau of Weights and Measures. Evaluation of measurement data — Guide to the expression of uncertainty in measurement[M]. France: First edition, 2008.
[7] TOSHIYUKI T, OSAWA S, KURIYAMA Y, et al. Stability of the reference flat used in Fizeau interferometer and its contribution to measurement uncertainty[J].SPIE, 2003, 5190:431-439.
[8] BURKE J, GRIESMANN U. Calibrating the sag due to gravity of horizontal interferometer reference flats[J]. Proceeding of SPIE,2012,84930:1-9.
[9] KONDO Y, BITOU Y. Evaluation of the deformation value of an optical flat under gravity[J]. Measurement Science and Technology,2014,25(6):064007. doi: 10.1088/0957-0233/25/6/064007
[10] POKORNÝ P. Gravity induced deflection of a reference plate and tested plane surfaces and its influence on optical measurement with the Fizeau interferometer[J]. Proceeding of SPIE,2015,944210:1-12.
[11] ZHU Xueliang. Research on the calibration method of reference flat based on deformation by gravity[J]. Proceeding of SPIE,2018,10827:108272Q.
[12] ZHENG D H, CHEN L, KONG L, et al. System design and error correction for 300 mm aperture vertical Fizeau spatial-temporal phase-shifting interferometer[J]. Applied Optics,2020,59(8):2512-2520.
[13] DECK L L, DE GROOT P J, SOOBITSKY J A. Large-aperture, equal-path interferometer for precision measurements of flat transparent surfaces[J]. Applied Optics,2014,53(8):1546. doi: 10.1364/AO.53.001546
[14] GU Wei. Removal of surface figure deformation due to gravity in optical test[J]. Proceeding of SPIE,2014,928209:1-7.
[15] QUAN H Y, GU W, HOU X, et al. Model-based optimization of gravity sagging for a horizontally mounted optical flat[J]. Applied Optics,2016,55(5):993. doi: 10.1364/AO.55.000993
[16] VENTSEL E, KRAUTHAMMER T. Thin plates and shells[M]. Boca Raton: CRC Press, 2001.
[17] ARTEMIEV N A, CHOW K P, LA CIVITA D, et al. Optimal setting of bendable optics based on FEA calculations[J]. Proceeding of SPIE,2012,8501:850107. doi: 10.1117/12.929098
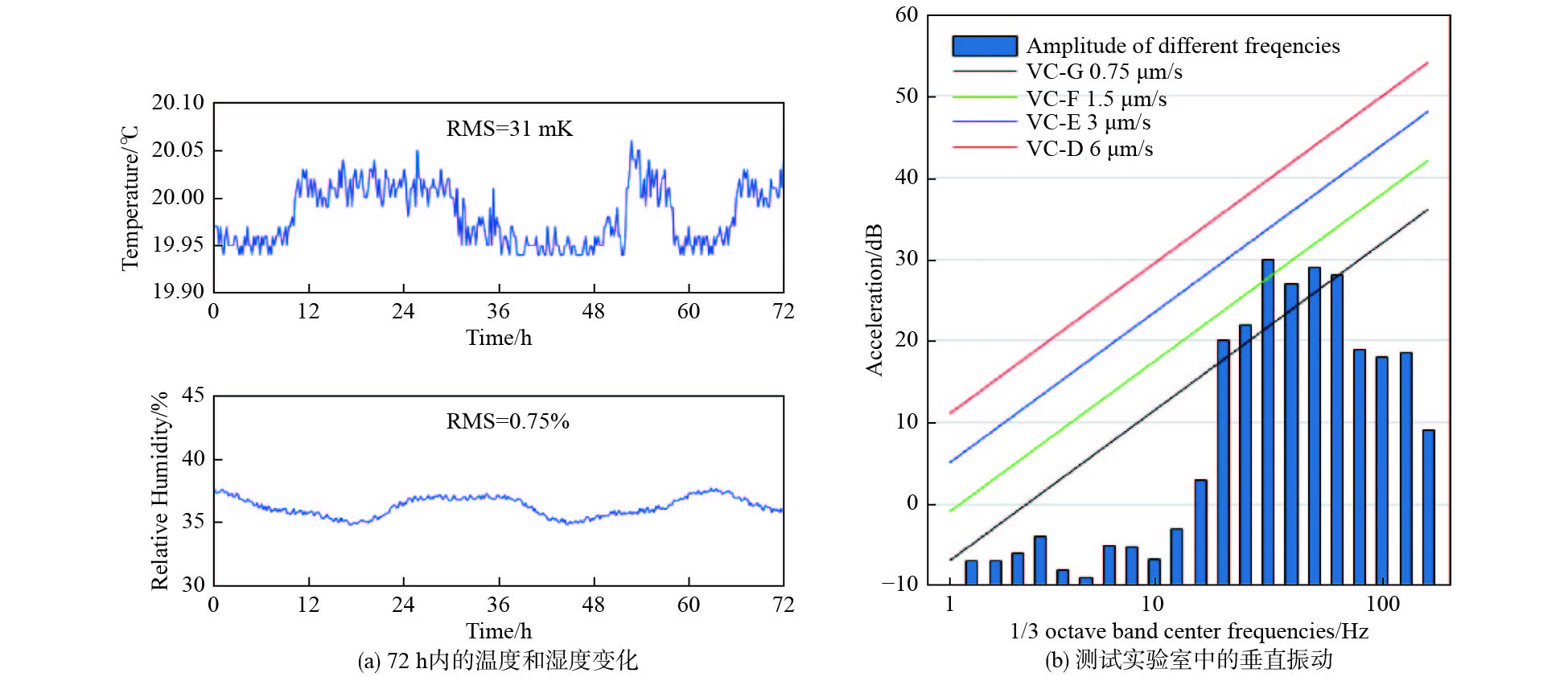
[18] YUMOTO H, MATSUYAMA S, MIMURA H, et al. Absolute calibration of optical Flats using the three-flat test by considering the relative humidity change[J]. Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment,2013,710:2-6.
[19] YASHCHUK V V, ARTEMIEV N A, LACEY I, et al. Advanced environmental control as a key component in the development of ultrahigh accuracyex situmetrology for X-ray optics[J]. Optical Engineering,2015,54(10):104104. doi: 10.1117/1.OE.54.10.104104
[20] AMICK H, GENDREAU M, BUSCH T, et al. Evolving criteria for research facilities: vibration[J]. Proceeding of SPIE,2005,5933:593303. doi: 10.1117/12.617970
[21] CHATTERJEE S, PAVAN KUMAR Y, SINGH R, et al. Measurement of the surface form error of large aperture plane optical surfaces with a polarization phase-shifting liquid reference reflection Fizeau interferometer[J]. Applied Optics,2016,55(2):310. doi: 10.1364/AO.55.000310
[22] CHU J, GRIESMANN U, WANG Q D, et al. Deformation-free form error measurement of thin, plane-parallel optics floated on a heavy liquid[J]. Applied Optics,2010,49(10):1849. doi: 10.1364/AO.49.001849
[23] GRIESMANN U. Three-flat tests including mounting-induced deformations[J]. Optical Engineering,2007,46(9):093601. doi: 10.1117/1.2784531
[24] ZHOU Y, LIU S J, LU Q, et al. In situ absolute surface metrology for a 600 mm aperture interferometer[J]. Optics and Lasers in Engineering,2020,129:106054. doi: 10.1016/j.optlaseng.2020.106054
[25] 王贵林, 朱俊辉, 李嘉祥, 等. 大口径光学元件表面疵病在位检测与评价研究[J]. 应用光学,2019,40(6):1167-1173. doi: 10.5768/JAO201940.0605005 WANG Guilin, ZHU Junhui, LI Jiaxiang, et al. In situ detection and evaluation of surface defects for large-aperture optical elements[J]. Journal of Applied Optics,2019,40(6):1167-1173. doi: 10.5768/JAO201940.0605005
-
期刊类型引用(0)
其他类型引用(1)
计量
- 文章访问数: 1101
- HTML全文浏览量: 378
- PDF下载量: 49
- 被引次数: 1
陕公网安备 61011302001501号 