Progress of terahertz metrology research and standard construction
-
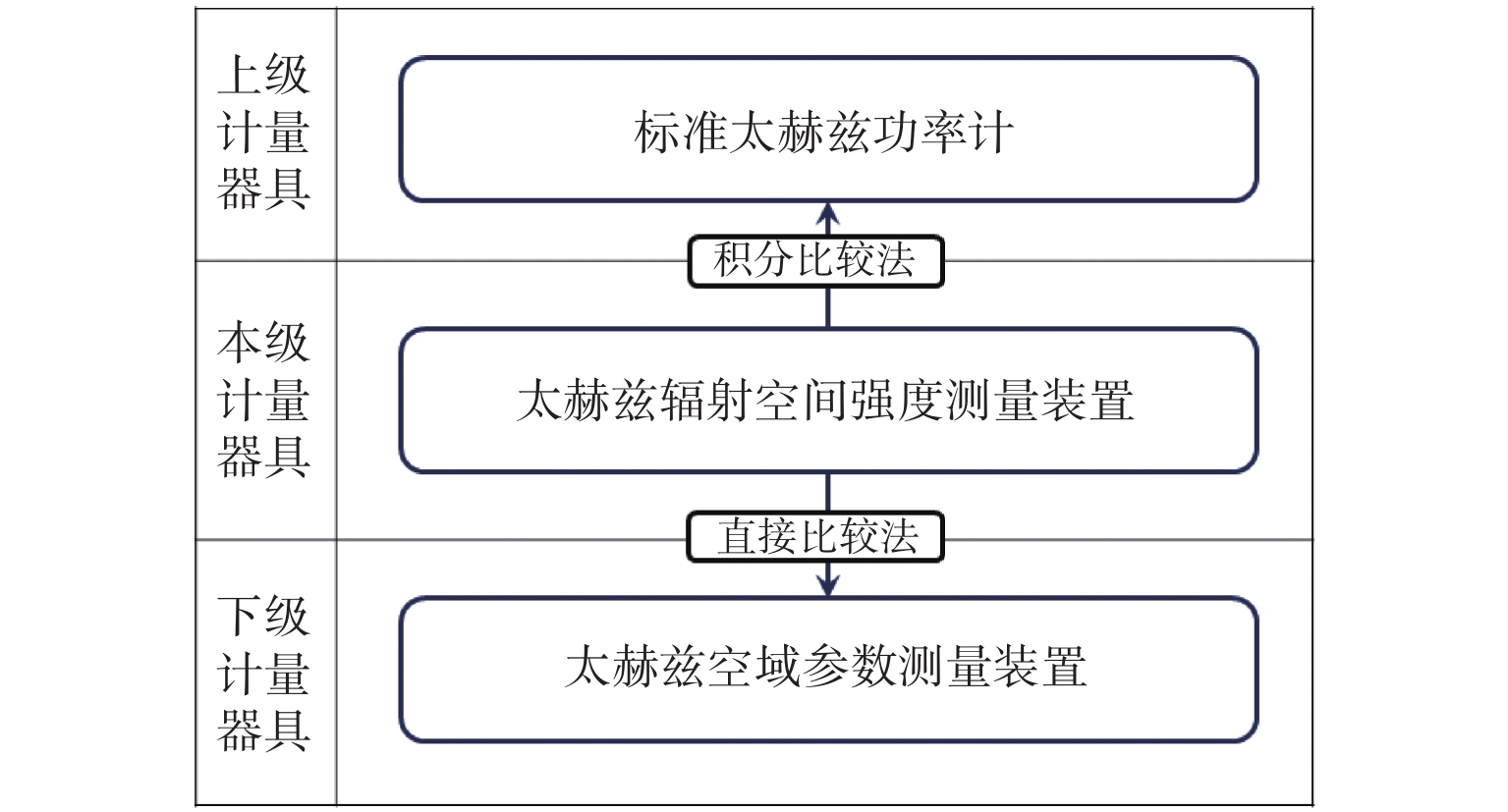
摘要: 太赫兹作为新的技术手段在物质成分识别、高速通信、生物医学、安检成像和军事国防等领域具有重要的作用。太赫兹技术的各种应用都建立在对太赫兹本身及其与物质相互作用测量的基础上，因此准确的太赫兹参数测量及相关量值溯源是太赫兹应用的技术支撑和保障。介绍中国计量科学研究院在太赫兹辐射参数计量标准研究中形成的测量技术和溯源方法、研制的测量仪器和测量装置、制定的计量校准法规和建立的计量标准装置，对太赫兹辐射时域、频域、空域和强度等参数给出了量值溯源传递图并进行了测量不确定度分析。提出的太赫兹计量方法和标准装置可保障太赫兹技术研究和应用中的量值可靠，也可为其他太赫兹参数的测量提供参考。Abstract: Terahertz, as a novel technology, has been widely used in the field of material component recognition, high-speed communication, biomedicine, security imaging and military defense. Various applications of terahertz technology are based on the measurement of terahertz itself and its interaction with substances, therefore the accurate terahertz parameter measurement and relevant magnitude tracing are the technical support and guarantee of terahertz application. The measurement technology and tracing method, developed measuring instruments and devices, and established metrology calibration regulations, and metrology standard device formed in the terahertz radiation parameters metrology standard research by National Institute of Metrology were introduced. The magnitude tracing transitive graphs of terahertz radiation time domain, frequency domain, space domain and intensity were presented, and the measurement uncertainty analysis was carried out. The proposed terahertz metrology method and standard devices can guarantee the reliable magnitude in terahertz technology research and application, and can also provide a reference for other terahertz parameters measurement.
-
Keywords:
- terahertz metrology /
- terahertz technology /
- measurement standard
-
引言
某型号星载光谱仪聚焦于陆地生态系统植被和森林蓄积量探测，利用670 nm～780 nm谱段的光谱获取空间连续分布的植被荧光信号，得到太阳诱导植被荧光遥感数据，从而准确绘制植被荧光时空分布规律，满足全球植被定量监测、森林植被生产力评估的需求。光谱仪采用光栅作为色散元件，由于分光系统的存在，系统会残余较大畸变，导致数据处理困难，星载光谱仪为了校正系统的畸变，成像透镜设计为离轴透射式光学系统，系统存在3个光轴，光轴之间倾斜量达到0.606°，偏心量达到0.279 mm，增加了装调的难度。
光学系统的质量和光学元件的装调精度息息相关，国内外调研结果表明，透射式系统装调方法多为双光路定心法，定心仪测试透镜表面球心的跳动量给出球心位置，球心连线确定光轴，装调时最小化不同透镜光轴的偏心和倾斜，达到系统设计指标[1-6]。双光路定心法只适用于同轴透射式系统，对于离轴透射式系统的装调方法未见相关报道。光谱仪成像系统最大倾斜量超出了定心仪的装调范围，无法应用双光路定心法。针对这个难题提出了一种多基准轴的定心装调方法（MAA），通过结构预置偏心和倾斜的方法构建基准轴，利用光学平板实现光轴引出，将复杂的多光轴系统装调分解成单光轴系统装调，实现了离轴透射式光学系统的高精度装调。实测结果验证了该方法的有效性，为离轴透射式系统装调开拓了新的思路。
1 装调原理
1.1 成像透镜系统参数
光谱仪采用光栅和棱镜作为色散元件，成像组件采用离轴透射式系统，补偿由光栅和棱镜引起的畸变，可实现系统的畸变最小化。成像组件包含3个光轴，光轴之间存在偏心和倾斜，如图1所示。透镜1和透镜2组成光轴1，透镜3和透镜4组成光轴2，透镜5为光轴3。光轴1和光轴2倾角为0.262°，偏心量为0.279 mm，光轴3相对于光轴1倾角为0.606°，参数如表1所示。
表 1 透镜的偏心和倾斜参数Table 1. Parameters of lens eccentricity and tilt透镜 偏心X 偏心Y/mm 倾斜X 倾斜Y/（°） 透镜1 0 0 0 0 透镜2 0 0 0 0 透镜3 0 −0.279 0 −0.262 透镜4 0 −0.279 0 −0.262 透镜5 0 0 0 −0.606 1.2 装调分析
畸变是实际像高和理想像高的偏差，根据δ初级像差理论，像差分为5种初级像差，以5个塞得和数表示。畸变是主光线的垂轴像差，表达式为
$$\delta Y' = - \dfrac{1}{{2n'u'}} \displaystyle\sum \limits_{i = 1}^k {S_{\rm{V}}}$$ (1) 根据塞得和数表达式：
$$ \displaystyle\sum \limits_{i = 1}^k {S_{\rm{I}}} = \displaystyle\sum \limits_{i = 1}^k luni\left( {i - i'} \right)\left( {i' - u} \right)$$ (2) $$ \displaystyle\sum \limits_{i = 1}^k {S_{{\rm{III}}}} = \displaystyle\sum \limits_{i = 1}^k {S_{{\rm{II}}}}\dfrac{{{i_{\textit{z}}}}}{i} = {S_{\rm{I}}}\dfrac{{{i_{\textit{z}}}^2}}{{{i^2}}}$$ (3) $$ \displaystyle\sum \limits_{i = 1}^k {S_{{\rm{IV}}}} = \displaystyle\sum \limits_{i = 1}^k {J^2}\dfrac{{n' - n}}{{n'nr}}$$ (4) $$ \displaystyle\sum \limits_{i=1}^{k}{S}_{\rm{V}}= \displaystyle\sum\limits _{i=1}^{k}({S}_{\rm{III}}+{S}_{\rm{IV}})\dfrac{{i}_{{\textit{z}}}}{i} $$ (5) 推导得出光学系统的畸变表达式：
$$ \begin{split} \delta {Y^\prime } = & - \dfrac{1}{{2n'u'}}\displaystyle\sum\limits_{i = 1}^k {\Bigg(luni\left( {i - {i^\prime }} \right)\left( {{i^\prime } - u} \right)\dfrac{{{i_{\textit{z}}}^2}}{{{i^2}}} + } \\ & nu{y^2}\dfrac{{{n^\prime } - n}}{{n'nr}}\Bigg)\dfrac{{{i_{\textit{z}}}}}{i} \end{split}$$ (6) 简化后的畸变表达式为
$$ \begin{split} \delta {Y^\prime } = & - \dfrac{1}{{2n'u'}} \displaystyle\sum \limits_{i = 1}^k \Bigg(\dfrac{{n\left( {{n^\prime } - n} \right)\left( {n - nr - {n^\prime }r} \right)lu}}{{{n^\prime }^2(l - r)}}{i_{\textit{z}}}^3+\\ & + \dfrac{{({n^\prime } - n{\rm{)}}u{y^2}}}{{l - r}}{i_{\textit{z}}}\Bigg) \end{split}$$ (7) 式中：n和n′为折射率；k为光学元件的数量；r为曲率半径；u为数值孔径；y为像高；
${i_{\textit{z}} }$ 为主光线和光轴的夹角。由公式（7）可知畸变与主光线和光轴的夹角以及像高正相关，本系统中数值孔径和像高无法改变，为了纠正畸变，透镜系统设计为离轴透射式系统，改变主光线和光轴的夹角。装调过程中精确控制离轴透镜组的倾角可有效控制畸变。将实测的透镜曲率半径和中心厚度输入到光学系统优化设计软件中[7-8]，重新对系统镜间距和偏心进行优化，以光轴1为基准，根据畸变计算光轴2和光轴3的失调量敏感度，如图2所示。结果表明，当畸变小于3 μm时，透镜偏心公差需小于0.05 mm，倾斜公差小于60″，镜间距公差小于0.05 mm，根据以往研究结果，直接装配无法满足系统要求[9-10]。
1.3 MAA装调方法
系统装调过程为最小化偏心以及倾斜量误差最小的过程，透镜组光轴偏差达到0.606°，超出了定心仪的测试范围，需要在系统中引入新的装调基准。考虑到装调公差相对宽松，提出了在透镜筒结构上一体化加工基准轴，预置光轴的倾斜量和偏差量，光学平板引出结构基准轴，应用OptiCentric双光路定心仪在新的基准轴上装调，将多光轴系统的装调分解成单光轴子系统装调，系统的装调流程如图3所示。
2 装调过程
2.1 机床定心
机床定心可去除光学件加工过程中产生的偏心和倾斜[11]，将透镜框的安装端面和透镜框的外圆加工成和光轴一致，如图4所示。固定透镜组件到机床上，千分表测量透镜框外圈的圆跳动，调整使其和机床同轴，通过光学定心方法调整透镜的偏心和倾斜，使其光轴和机床转轴重合，加工透镜框的外圆和端面与透镜光轴重合。
2.2 同轴系统装调
系统包含2个同轴透镜组，单透镜机床定心完成后，选用OptiCentric双光路定心仪进行定心装调[12]。调整透镜1的光轴与定心仪的旋转轴一致，安装透镜2，通过垫片和侧面顶丝调整透镜2的光轴与定心仪的旋转轴一致，如图5和图6所示。同理装调透镜3和透镜4组件。
2.3 光轴引出
系统包含3个光轴，最大偏差0.606°，镜筒一体化加工预置偏心和倾斜，构建3个基准轴，建立装调基准。如图7所示，基准轴1和镜筒外径同轴，基准轴2相对于基准轴1的夹角为0.262°，偏心量为0.279 mm，基准轴3相对于基准轴1的夹角为0.606°。
基准轴的加工精度直接影响系统的装调精度，基准轴偏差计算公式为
$$ {\rm{\alpha }} = \dfrac{{{h_1} - {h_2}}}{D} $$ (8) 式中：
$ \alpha$ 为基准轴倾角；$ {h_1} $ 和$ {h_2} $ 为基准轴两侧的高度；D为基准内径。对公式（8）求导可得：$$ {\rm{\delta \alpha }} = \dfrac{1}{D}(\delta {h_1} - \delta {h_2}) - \dfrac{{{h_1} - {h_2}}}{{{D^2}}}\delta D $$ (9) 其中内径D为100 mm，对应0.606°的倾角，基准轴两侧的高度差为1.06 mm，公式（9）第2项相对于第1项可以忽略。提高基准轴精度的关键是控制
$ {h_1}$ 和$ {h_2}$ 的加工精度，加工过程中重点保证基准面的高度差，加工完成后，高精度三坐标测量机测试表明，偏心公差优于0.02 mm，倾斜公差优于30″，能够满足基准轴引出精度要求。2.4 系统装调
装调过程如图8和图9所示。将镜筒固定在双光路定心仪的转台上，采用光学平板将光轴1引出，结合杠杆千分表调整光轴和定心仪同轴，倾斜量小于10″，圆跳动量小于0.015 mm，作为光轴1的基准轴。安装透镜1和透镜2组件，调整到倾斜偏差小于10″，偏心误差小于0.015 mm。将镜筒翻转180°，通过光学平板和杠杆千分表引出光轴2，作为透镜3和透镜4组件的安装基准。安装透镜3和透镜4组件时，调整倾斜偏差小于10″，偏心小于0.015 mm。光学平板引出光轴3，安装透镜5组件，调整到倾斜偏差小于10″，偏心误差小于0.015 mm，完成系统偏心和倾斜的装调。
装调误差分布如图10所示。从图10可知，基准轴的引出误差为主要因素，通过进一步提高镜筒的加工精度可提高系统的装调精度。
1） 镜筒同轴度误差。镜筒的同轴度为15 μm，千分尺的测量误差为1 μm，引起的镜头偏心误差为8 μm。
2） 镜筒基准轴偏心、倾斜误差。三坐标测量结果表明，镜筒偏心误差优于20 μm，倾斜误差优于30″。
3） 机床主轴回转误差。机床主轴引起的倾斜误差为2″，偏心误差为3 μm。
4） 定心仪测量误差。双光路定心仪倾斜测量误差为1″，偏心测量误差为1 μm。
5） 同轴透镜调整误差。透镜1和透镜2组件，透镜3和透镜4组件为同轴系统，调整误差较小，偏心可控制在10 μm，倾斜控制在10″。
6） 离轴透镜调整误差。透镜倾斜调整误差为10″，偏心调整误差为15 μm。
7） 光学平板接触误差。光学平板平面度优于10 nm，光学平板和结构面接触误差优于10 μm，镜筒的内径为100 mm，引起镜头倾斜偏差为20.6″。
综合以上误差，可计算得出透镜组的倾斜误差优于38.8″，偏心误差优于28.3 μm，满足系统的指标要求。
2.5 镜间距控制
同轴透射式系统的镜间距通过测试球面顶点得到[13]，球面顶点同时也是透镜的最高点或者最低点，对于离轴系统，透镜表面的最高点和最低点不再位于球面顶点，偏差量和倾斜偏心量相关，如图11所示。球面顶点A和表面最高点A′之间距离X如下式所示：
$$X = R - R \times {\rm{cos}}a$$ (10) 式中：a为透镜的倾角；R为球面曲率半径。
根据公式（10）计算得出透镜最高点距离和透镜间距的差值如表2所示。
表 2 镜间距和测试距离对应表Table 2. Corresponding lens distance and test distanceItem Lens1 & Lens2 Lens2 & Lens3 Lens3 & Lens4 Lens4 & Lens5 Distance between vertex of lens/mm 1.800 50.765 25.248 6.928 Distance between lens/mm 1.800 50.763 25.248 6.923 Difference/mm 0 0.002 0 0.005 在多基准轴装调过程中，通过接触式方法测量镜间距[14-15]，如图12所示。首先测量透镜1和透镜2之间的镜间距以及透镜3和透镜4之间的镜间距，将透镜2的光轴调整到和双光路定心仪的转台同轴，通过探针分别测量透镜1和透镜2上表面的顶点高度d1和d2。透镜1和透镜2的镜间距计算方法如下式：
$${D_{12}} = {d_2} - {d_1} - {D_2}$$ (11) 式中：D2为透镜2的中心厚度；D3为透镜3的中心厚度。同理测量透镜3和透镜4镜间距D34。
透镜1和透镜2组件安装到镜筒中，测量透镜2的上表面最高点H2，安装透镜3和透镜4组件，测量透镜4上表面最高点H4，透镜2和透镜3的镜间距D23如下式：
$${D_{23}} = {H_4} - {H_2} - {D_{34}} - {D_3} - {D_4}$$ (12) 同理测量透镜4和透镜5镜间距D45。系统装调后实物图如图13所示。
镜间距测量误差如表3所示。探针2次测量得出镜间距，系统装调倾斜误差优于38.8″，偏心误差优于28.3 μm，引起的镜间距测量误差≤1 μm，综合两种测量误差，镜间距测量误差≤14.2 μm，满足系统指标要求。
表 3 镜间距测量误差Table 3. Measurement error of lens distance误差源 探针单点测量
误差/μm偏心倾斜引起的
误差/μm镜间距
误差/μm数值 10 1 14.2 3 装调结果
系统装调完成后，分别测试光轴1，光轴2和光轴3，因为光轴1和光轴3的夹角为0.606°，超出了定心仪的测量范围，采用分段测试的方式，分别测试光轴1和光轴2的偏差，光轴2和光轴3的偏差，测试结果如表4和表5所示，与设计结果的偏差如表6所示。最大偏心误差为25.4 μm，最大倾斜误差为17.7″，实际畸变和理论畸变对比如图14所示。从图14可看出，最大畸变为2.77 μm，平均偏差0.32 μm，系统装调结果满足指标要求。
表 4 透镜1和透镜2组件以及透镜3和透镜4组件的偏心倾斜Table 4. Eccentricity and tilt of lens 1, 2 and lens 3, 4Parameters Decenter X/μm Decenter Y/μm Tilt X/（″） Tilt Y/（″） Axis1 0 0 0 0 Axis2 −10.4 −297.5 −3.3 −960.9 表 5 透镜3和透镜4组件以及透镜5组件的偏心倾斜Table 5. Eccentricity and tilt of lens 3, 4 and lens 5Decenter X/μm Decenter Y/μm Tilt X/（″） Tilt Y/（″） Axis2 0 0 0 0 Axis3 −9.1 −253.5 −17.6 −1232.1 表 6 透镜组的偏心倾斜偏差Table 6. Eccentricity and tilt deviation of lensDifference Decenter X/μm Decenter Y/μm Tilt X/（″） Tilt Y/（″） Axis1 0 0 0 0 Axis2 −10.4 −18.5 −3.3 −17.7 Axis3 1.3 25.4 −14.3 6.3 4 结论
本文提出了一种新的离轴透射式系统的装调方法——多基准轴定心装调方法（MAA），克服传统的同轴透射系统定心装调缺陷，通过结构预置偏心和倾斜的方法构建基准轴，并辅助光学平板将复杂的多光轴系统装调分解成单光轴系统装调，利用此方法装调了光谱仪多光轴成像系统。装调结果表明，透镜偏心误差小于25.4 μm，倾斜误差小于17.7″，实现了系统的高精度装调。本装调方法不受定心仪测量范围的限制，可针对任意光轴的透射系统进行装调，突破了传统透射式装调方法的边界，为离轴透射式系统装调开拓了新的思路。
-
![]()
图 2 一氧化碳气体室与测量的特征吸收谱线
Figure 2. Carbon monoxide gas chamber and measured characteristic absorption spectrum line
![]()
图 3 自制的法布里-珀罗标准具与测量的干涉光谱
Figure 3. Self-fabricated Fabry-Perot etalon and measured interference spectrum
![]()
图 4 太赫兹光谱仪校准溯源传递框图
Figure 4. Block diagram of calibration tracing transmission for terahertz spectrometer
![]()
图 5 测量的太赫兹反射光谱比和研制的太赫兹辐射计
Figure 5. Measured reflectance spectrum ratio and self-fabricated terahertz radiometer
![]()
图 6 太赫兹功率计校准溯源传递框图
Figure 6. Block diagram of calibration tracing transmission for terahertz power meter
![]()
图 7 国际首次太赫兹功率测量比对结果
Figure 7. Comparison results of the first international terahertz power measurement
![]()
图 8 基于频率梳测量太赫兹频率的装置示意图
Figure 8. Schematic diagram of terahertz frequency measurement with frequency comb
![]()
图 10 干涉法测量太赫兹频率结果
Figure 10. Terahertz frequency measurement result with terahertz interferometry
![]()
图 11 太赫兹波长和频率校准溯源传递框图
Figure 11. Block diagram of calibration tracing transmission for terahertz wavelength and frequency
![]()
图 12 电光采样测量太赫兹空间辐射强度装置示意图
Figure 12. Schematic diagram of device for terahertz space radiation intensity measurement
![]()
图 13 不同距离下100 GHz辐射源的空间辐射功率密度测量结果
Figure 13. Measurement results of space radiation power density for 100 GHz radiation source at different distances
![]()
图 14 太赫兹波长和频率校准溯源传递框图
Figure 14. Block diagram of calibration tracing transmission for terahertz wavelength and frequency
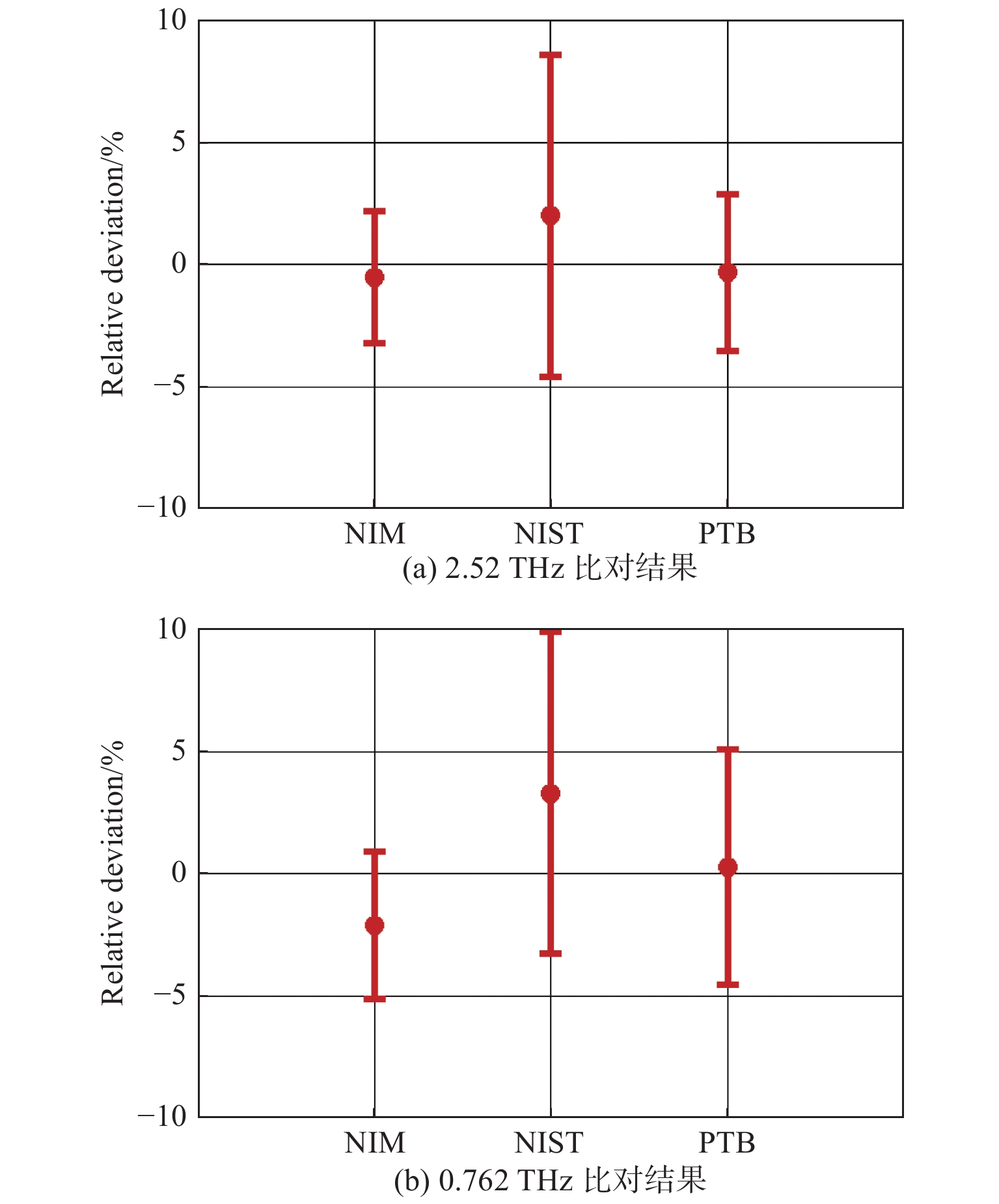
表 1 国际首次太赫兹功率比对的测量不确定度
Table 1 Measurement uncertainty of the first international terahertz power comparison
% 比对频率 /THz 中国 美国 德国 2.52 Urel=2.8（k=2） Urel=6.6（k=2） Urel=3.2（k=2） 0.762 Urel=3.2（k=2） Urel=6.6（k=2） Urel=4.8（k=2） 
下载: 导出CSV
-
[1] SIRTORI C. Bridge for the terahertz gap[J]. Nature,2002,417(6855):132-133.
[2] TONOUCHI M. Cutting-edge terahertz technology[J]. Nature Photonics,2007,1(2):97-105. doi: 10.1038/nphoton.2007.3
[3] SIEGEL P H. Terahertz technology[J]. IEEE Transactions on Microwave Theory and Techniques,2002,50(3):910-928. doi: 10.1109/22.989974
[4] KLEINE-OSTMANN T, SCHRADER T, BIELER M, et al. THz metrology[J]. Frequenz,2008,62(5/6):137-148.
[5] SMITH P R, AUSTON D H, NUSS M C. Subpicosecond photoconducting dipole antennas[J]. IEEE Journal of Quantum Electronics,1988,24(2):255-260. doi: 10.1109/3.121
[6] XIE X, DAI J M, ZHANG X C. Coherent control of THz wave generation in ambient air[J]. Physical Review Letters,2006,96(7):075005. doi: 10.1103/PhysRevLett.96.075005
[7] DAI J M, XIE X, ZHANG X C. Detection of broadband terahertz waves with a laser-induced plasma in gases[J]. Physical Review Letters,2006,97(10):103903. doi: 10.1103/PhysRevLett.97.103903
[8] DENG Y Q, SUN Q, YU J. On-line calibration for linear time-base error correction of terahertz spectrometers with echo pulses[J]. Metrologia,2014,51(1):18-24. doi: 10.1088/0026-1394/51/1/18
[9] DENG Y Q, LI J, SUN Q. Traceable measurements of CW and pulse terahertz power with terahertz radiometer[J]. IEEE Journal of Selected Topics in Quantum Electronics,2017,23(4):1-6.
[10] ZHANG J, XIA T, CHEN Q, et al. Measurement of absorption coefficient of paraformaldehyde and metaldehyde with terahertz spectroscopy[J]. Journal of Applied Spectroscopy,2018,85(1):84-89. doi: 10.1007/s10812-018-0616-6
[11] DENG Y Q, SUN Q, YU J, et al. Broadband high-absorbance coating for terahertz radiometry[J]. Optics Express,2013,21(5):5737-5742. doi: 10.1364/OE.21.005737
[12] ZHANG Y, ZHANG L, SUN P, et al. Extracting complex refractive index from polycrystalline glucose with self-referenced method for terahertz time-domain reflection spectroscopy[J]. Applied Spectroscopy,2016,70(7):1102-1108. doi: 10.1177/0003702816652318
[13] WANG J, DENG Y, WU Y, et al. A single-resonant-structure and optically transparent broadband THz metamaterial absorber[J]. Journal of Infrared, Millimeter, and Terahertz Waves,2019,40(6):648-656. doi: 10.1007/s10762-019-00598-w
[14] WU Y H, DENG Y Q, WANG J J, et al. THz broadband absorber fabricated by EHD printing technology with high error tolerance[J]. IEEE Transactions on Terahertz Science and Technology,2019,9(6):637-642. doi: 10.1109/TTHZ.2019.2943564
[15] 邓玉强, 邢岐荣, 郎利影, 等. THz波的小波变换频谱分析[J]. 物理学报,2005,54(11):5224-5227. doi: 10.3321/j.issn:1000-3290.2005.11.042 DENG Yuqiang, XING Qirong, LANG Liying, et al. Wavelet-transform in terahertz time-domain spectroscopy[J]. Acta Physica Sinica,2005,54(11):5224-5227. doi: 10.3321/j.issn:1000-3290.2005.11.042
[16] 邓玉强, 郎利影, 邢岐荣, 等. Gabor小波分析太赫兹波时间-频率特性的研究[J]. 物理学报,2008,57(12):7747-7752. doi: 10.3321/j.issn:1000-3290.2008.12.054 DENG Yuqiang, LANG Liying, XING Qirong, et al. Terahertz time-frequency analysis with Gabor wavelet-transform[J]. Acta Physica Sinica,2008,57(12):7747-7752. doi: 10.3321/j.issn:1000-3290.2008.12.054
[17] NAFTALY M, DUDLEY R A, FLETCHER J R, et al. Frequency calibration of terahertz time-domain spectrometers[J]. Journal of the Optical Society of America B,2009,26(7):1357-1392. doi: 10.1364/JOSAB.26.001357
[18] 中华人民共和国国家质量监督检验检疫总局. JJF 1603-2016 (0.1～2.5)THz太赫兹光谱仪校准规范[S]. 北京: 中国标准出版社, 2016. General Administration of Quality Supervision, Inspection and Quarantine of the People's Republic of China. JJF 1603-2016 Calibration specification for (0.1～2.5) THz terahertz spectrometers[S]. Beijing: China Standards Press, 2016.
[19] NAFTALY M, DUDLEY R. Linearity calibration of amplitude and power measurements in terahertz systems and detectors[J]. Optics Letters,2009,34(5):674-676. doi: 10.1364/OL.34.000674
[20] 尹演子, 李在清. 光谱规则透射比标准装置的建立[J]. 计量学报,1994,15(4):249-254. YIN Yanzi, LI Zaiqing. Establishment of a standard apparatus for spectral regular transmittance[J]. Acta Metrologica Sinica,1994,15(4):249-254.
[21] LIU J Q, CHEN J Y, WANG T, et al. High efficiency and high power continuous-wave semiconductor terahertz lasers at ~3.1 THz[J]. Solid-State Electronics,2013,81:68-71. doi: 10.1016/j.sse.2013.01.014
[22] ZHU H, WANG F F, YAN Q, et al. Terahertz master-oscillator power-amplifier quantum cascade lasers[J]. Applied Physics Letters,2016,109:231105. doi: 10.1063/1.4969067
[23] GUO X G, ZHANG R, CAO J C, et al. Surface plasmon-enhanced absorption in metal grating coupled terahertz quantum well photodetectors[J]. IEEE Journal of Quantum Electronics,2012,48(9):1113-1119. doi: 10.1109/JQE.2012.2202637
[24] WERNER L, HÜBERS H W, MEINDL P, et al. Towards traceable radiometry in the terahertz region[J]. Metrologia,2009,46(4):S160-S164. doi: 10.1088/0026-1394/46/4/S05
[25] LEHMAN J H, LEE B, GROSSMAN E N. Far infrared thermal detectors for laser radiometry using a carbon nanotube array[J]. Applied Optics,2011,50(21):4099-4104. doi: 10.1364/AO.50.004099
[26] 中华人民共和国国家质量监督检验检疫总局. JJF 1600-2016 辐射型太赫兹功率计校准规范[S]. 北京: 中国标准出版社, 2016. General Administration of Quality Supervision, Inspection and Quarantine of the People's Republic of China. JJF 1600-2016 Calibration specification for terahertz radiation power meters[S]. Beijing: China Standards Press, 2016.
[27] STEIGER A, MULLER R, OLIVA A R, et al. Terahertz laser power measurement comparison[J]. IEEE Transactions on Terahertz Science and Technology,2016,6(5):664-669.
[28] 邓玉强, 孙青, 于靖, 等. 太赫兹辐射功率计量研究进展与国际比对[J]. 中国激光,2017,44(3):270-278. DENG Yuqiang, SUN Qing, YU Jing, et al. Progress of terahertz radiometry and international comparison[J]. Chinese Journal of Lasers,2017,44(3):270-278.
[29] YOKOYAMA S, NAKAMURA R, NOSE M, et al. Terahertz spectrum analyzer based on a terahertz frequency comb[J]. Optics Express,2008,16(17):13052-13061. doi: 10.1364/OE.16.013052
[30] FÜSER H, JUDASCHKE R, BIELER M. High-precision frequency measurements in the THz spectral region using an unstabilized femtosecond laser[J]. Applied Physics Letters,2011,99(12):121111. doi: 10.1063/1.3640234
[31] FÜSER H, BIELER M. Terahertz frequency combs[J]. Journal of Infrared, Millimeter, and Terahertz Waves,2014,35(8):585-609. doi: 10.1007/s10762-013-0038-8
[32] 孟莹, 邓玉强, 郭树恒, 等. 基于频率梳的太赫兹辐射功率密度测量[J]. 红外与毫米波学报,2019,38(2):254-261. doi: 10.11972/j.issn.1001-9014.2019.02.020 MENG Ying, DENG Yuqiang, GUO Shuheng, et al. Terahertz spatial radiant power density measurements based on terahertz frequency comb[J]. Journal of Infrared and Millimeter Waves,2019,38(2):254-261. doi: 10.11972/j.issn.1001-9014.2019.02.020
[33] GUO S H, DENG Y Q, MENG Y, et al. Measurement and traceablility of terahertz wavelength and frequency[C]//2019 Cross Strait Quad-Regional Radio Science and Wireless Technology Conference (CSQRWC), July 18-21, 2019. Taiyuan, China. USA: IEEE, 2019.
[34] 孙青, 杨奕, 孟飞, 等. 基于频率梳的太赫兹频率精密测量方法研究[J]. 光学学报,2016,36(4):133-138. SUN Q, YANG Y, MENG F, et al. High-precision measurement of terahertz frequency based on frequency comb[J]. Acta Optica Sinica,2016,36(4):133-138.
[35] DENG Y Q, FÜSER H, BIELER M. Absolute intensity measurements of CW GHz and THz radiation using electro-optic sampling[J]. IEEE Transactions on Instrumentation and Measurement,2015,64(6):1734-1740. doi: 10.1109/TIM.2014.2375692
-
期刊类型引用(2)
1. 杜国军，张玉贵，崔博伦，江澄，欧宗耀. 碳卫星超光谱探测仪地面光谱定标. 光谱学与光谱分析. 2023(05): 1556-1562 . 
百度学术
2. 严冬，国成立，刘泉，黎发志，余晨阳，马洋，张宇龙，闫力松. 大数值孔径宽光谱折反式物镜设计(特邀). 红外与激光工程. 2023(09): 66-72 . 百度学术
其他类型引用(3)
计量
- 文章访问数: 3254
- HTML全文浏览量: 1583
- PDF下载量: 77
- 被引次数: 5
陕公网安备 61011302001501号 