Research on diffraction effect of primary mirror in segmented telescope
-
摘要: 拼接式主镜的设计使超大口径望远镜的构想成为现实。为了研究拼接式望远镜的成像性能,精确分析和量化了拼接式主镜构型、平移误差、倾斜误差对衍射效应的影响,从拼接式光学系统的成像原理出发,基于齐次坐标变换建立了拼接式主镜的光瞳模型,并仿真分析了拼接主镜构型对衍射效应的影响。分析结果表明:对于不同构型的拼接主镜,其衍射效应受拼接主镜的填充因子和孔径间隔共同影响,填充因子越高,孔径间隔越小,系统成像质量越好。以典型的拼接主镜构型为例,分别仿真分析了单个子镜平移误差、倾斜误差和拼接主镜整体平移误差、倾斜误差对衍射效应的影响。分析结果表明:对于单个子镜,平移误差对远场衍射的影响具有周期性;对于拼接主镜整体,当子镜piston误差的均方根值小于0.039λ时,斯特列尔比大于0.95;当子镜tip-tilt误差的均方根值小于0.036λ时,斯特列尔比大于0.95。分析结果为拼接式望远镜的成像性能分析、主镜的构型设计、平移误差和倾斜误差的检测与调整等提供了依据。
-
关键词:
- 拼接式望远镜 /
- 衍射效应 /
- 主镜构型 /
- piston误差 /
- tip-tilt误差
Abstract: The design of the segmented primary mirror makes an idea of the extremely large aperture telescope a reality. In order to study the imaging performance of the segmented telescope, the effect of the segmented primary mirror configuration, translation error, and tilt error on diffraction effect was precisely analyzed and quantified. Based on the imaging principle of the segmented optical system, the pupil models of the segmented primary mirror were established based on the homogeneous coordinate transformation. And the effect of the segmented primary mirror configuration on the diffraction effect was simulated and analyzed. The analysis results show that for the segmented primary mirrors with different configurations, the diffraction effect is affected by the fill factor and the aperture interval of the segmented primary mirror, the higher the fill factor, the smaller the aperture interval, and the better the system imaging quality. Taking the typical segmented primary mirror configuration as an example, the effect of the translation error and tilt error of the single sub-mirror and the overall translation error and tilt error of the segmented primary mirror on diffraction effect was simulated and analyzed, respectively. The analysis results show that for the single sub-mirror, the effect of the translation error on far-field diffraction is periodic. And for the overall segmented primary mirror, the Strehl ratio is higher than 0.95, when the root mean square of the sub-mirror piston error is less than 0.039λ, or the root mean square of the sub-mirror tip-tilt error is less than 0.036λ. The analysis results provide the basis for the imaging performance analysis of the segmented telescope, the configuration design of the primary mirror, and the detection and adjustment of the translation error and tilt error. -
引言
随着科学技术的发展,天文学家迫切需求更高分辨率的天文望远镜来探索宇宙的奥秘。增大望远镜的主镜口径是提高观测分辨率和集光能力的有效手段[1-2]。然而随着主镜口径的不断增大,给望远镜的制造、加工、检测、集成装调等技术都带来了巨大的挑战[3]。为了解决这一技术难题,科学家提出采用拼接式主镜代替单块主镜的方案[4-5]。其中,地基望远镜KECK I/II、GTC、GMT[6]、TMT[7]、E-ELT[8]、天基望远镜JWST[9]等皆采用拼接式主镜的设计方案。虽然拼接式主镜的设计具有很多优势,但如何选择合适的构型以及如何检测和调整拼接失调所引起的平移误差、倾斜误差成为必须要解决的难题。
为了使拼接式望远镜的分辨率接近等效口径的衍射极限,首先分析拼接式主镜构型、平移误差、倾斜误差对衍射效应的影响。目前,国外Chanan G[10]、Yaitskova N[11]等人通过点扩散函数和斯特列尔比(Strehl ratio)[12]简单分析了平移误差、倾斜误差对衍射效应的影响,但都没有研究拼接式主镜模型建立的方式和分析拼接式主镜构型对衍射效应的影响。在国内,针对拼接式望远镜的研究主要集中在平移误差、倾斜误差的检测技术上[13-14],对拼接式主镜的衍射效应研究较少,其中,李毅超[15]等人分析了单个子镜在不同平移误差、倾斜误差下拼接主镜的衍射效应,得到的结论为分析拼接镜系统的成像质量提供了依据。
本文从标量衍射的原理入手,分析了拼接式光学系统的成像原理。基于齐次坐标变换建立了拼接式主镜的光瞳模型,并利用拼接主镜的构型参数、远场衍射的点扩散函数及其归一化相对强度分析了拼接式主镜构型对衍射效应的影响。然后以典型的拼接主镜构型为例,分别仿真分析了单个子镜在不同平移误差、倾斜误差下拼接主镜远场衍射的点扩散函数及其归一化相对强度,并利用斯特列尔比仿真分析了拼接主镜整体平移误差、倾斜误差对衍射效应的影响。分析结果为发展拼接式望远镜提供了技术支持。
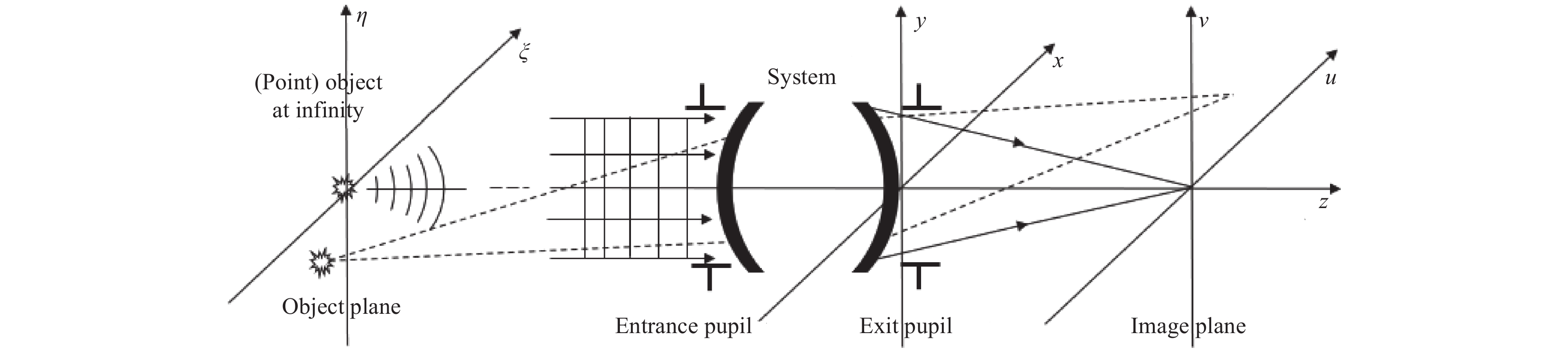
1 拼接式光学系统的成像原理
如图1所示,对于任意一个光学成像系统模型,均可以简化为由物面、系统的入瞳和出瞳、像面3部分组成。其中,(出射)光瞳函数
$p(x,y)$ 决定了光学成像系统的主要性质。对于拼接式望远镜系统,拼接主镜的光瞳函数
$p(x,y)$ 可以描述为[16-17]$$p(x,y) = \sum\limits_{n = 1}^N {{p_n}(x,y)} \cdot {\delta _{{u_n}}}$$ (1) 式中:
${p_n}(x,y)$ 表示每个孔径的光瞳函数,对应的中心坐标为${u_n}$ ;${\delta _{{u_n}}}$ 表示基于齐次坐标变换对光瞳的平移和旋转。每个孔径光瞳函数的模$\Pi (u)$ 为$$\Pi (u) = \left\{ {\begin{array}{*{20}{l}} {1\;\;\;{\rm{inside}}\;\;{\rm{the}}\;\;{\rm{aperture}}}\\ {0\;\;\;\;\;\;\;\;{\rm{elsewhere}}} \end{array}} \right.$$ (2) 基于泽尼克多项式[18],每个孔径的光瞳函数
${p_n}(u)$ 可表示为$${p_n}(u) = \Pi (u)\exp \left[ {{\rm{j}}\sum\limits_{k = 1}^{{k_{\max }}} {{\alpha _{kn}}{Z_k}(u)} } \right]$$ (3) 式中:
${j^2} = - 1$ ;${\alpha _{kn}}$ 是孔径$n$ 的像差系数;${Z_k}(u)$ 是泽尼克多项式的项。对于拼接式望远镜,特有的波前误差为${\rm{pistons}}(k = 1)$ 和${\rm{tips/tilts}}(k = \left\{ {2,3} \right\})$ ,因此将对${k_{\max }} = 3$ 的情况展开深入研究。对于非相干、准单色光照明,非相干成像系统对于光强这一物理量具有线性空间不变性。像强度
${I_i}$ 是强度脉冲响应${h_I}$ 与理想的像强度${I_g}$ 的卷积:$${I_i}(x,y) = {I_g}(\bar \xi ,\bar \eta ) * {h_I}(x,y)$$ (4) 式中:
${h_I}$ 定义为点扩散函数(point spread function,PSF),是通过光瞳函数$p(x,y)$ 作傅里叶变换并取模的平方得到。2 拼接式主镜光瞳模型建立
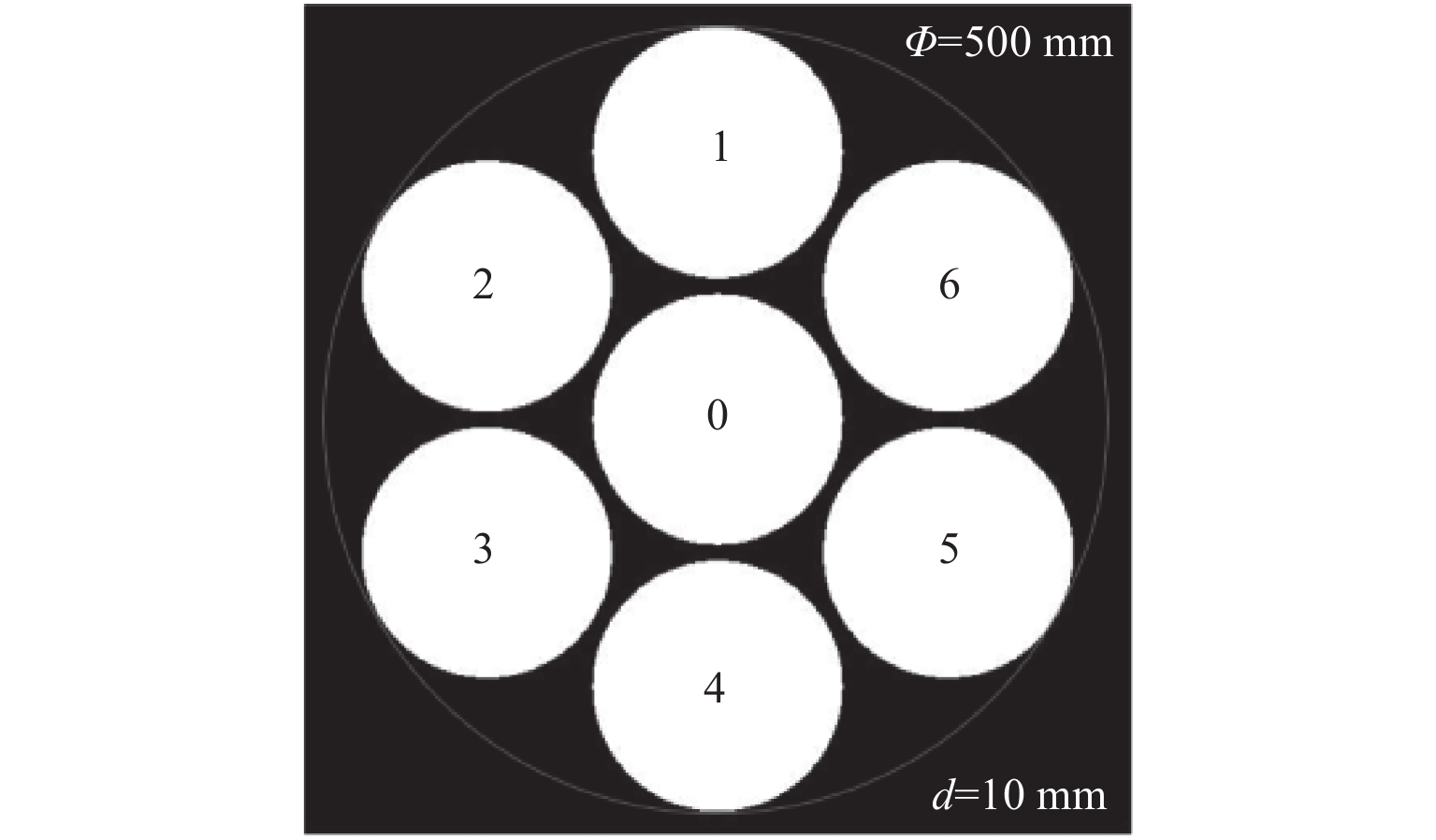
参考KECK、GMT、JWST等拼接式望远镜的主镜结构,设计同心圆环形结构(由一个中心镜和
$M$ 环相同子镜拼接组成)的主镜模型,如图2所示。令环数$M = 1$ ,忽略孔径遮拦问题,通过齐次坐标变换对拼接主镜的光瞳进行建模。假设拼接主镜等效口径为${\mathit{\Phi}} ({\mathit{\Phi}} = 500\;\,{\rm{mm)}}$ ,主镜孔径间隔为$d(d = $ $10\,\,{\rm{mm}})$ ,每个孔径$n(n = 7)$ 的半径为$R(R = 160\,\,{\rm{mm}})$ 。因此,每个孔径光瞳函数${p_n}$ 的模$\Pi $ 可以描述为$$\Pi (u) = \left\{ {\begin{array}{*{20}{l}} {1\;\;\;{\rm{for}}\;\;0 \leqslant \left| u \right| \leqslant 1}\\ {0\;\;\;\;\;\;{\rm{elsewhere}}} \end{array}} \right. $$ (5) 式中:
${u^2} = {x^2} + {y^2}$ ($x,y$ 都是归一化的)。对中心镜0进行建模,然后对原始坐标系$oxy$ 进行平移变换,计算公式如下:$$\left[ {x'\;\;y'\;\;1} \right] = \left[ {x\;\;y\;\;1} \right]\left[ {\begin{array}{*{20}{l}} {1\;\;\;0\;\;\;0}\\ {0\;\;\;1\;\;\;0}\\ {{{{\varDelta}} _x}\;{{{\varDelta}} _y}\;1} \end{array}} \right] = \left[ {x + {{{\varDelta}} _x}\;\;y + {{{\varDelta}} _y}\;\;1} \right]$$ (6) 式中:
${{{\varDelta}} _x}$ 、${{{\varDelta}} _y}$ 是平移矢量,在新坐标系${o'}{x'}{y'}$ 下,可以对子镜1进行建模。对原始坐标系$oxy$ 进行旋转变换,旋转角为$\theta (\theta = {60^ \circ })$ ,得到坐标系${o''}{x''}{y''}$ ,计算公式为$$ \begin{aligned} \left[ {x''\;\;y''\;\;1} \right] = & \left[ {x\;\;y\;\;1} \right]\left[ {\begin{array}{*{20}{c}} {\cos \theta }&{\sin \theta }& 0 \\ { - \sin \theta }&{\cos \theta }& 0 \\ 0&0&1 \end{array}} \right] =\\ &\left[ {x\cos \theta - y\sin \theta \;\;x\sin \theta + y\cos \theta\;\; 1} \right] \end{aligned} $$ (7) 然后再对坐标系
${o''}{x''}{y''}$ 进行平移变换得到坐标系${o^{'''}}{x^{'''}}{y^{'''}}$ ,在坐标系${o^{'''}}{x^{'''}}{y^{'''}}$ 下可以对子镜2进行建模。同理,依次完成子镜3、4、5、6的光瞳建模。3 拼接式主镜构型对衍射效应的影响
根据典型拼接主镜光瞳模型的建立过程,基于齐次坐标变换构造不同孔径形状、孔径间隔的拼接主镜模型,然后分别对其衍射效应进行仿真分析。
3.1 孔径形状对衍射效应的影响
假设拼接主镜的等效口径
${\mathit{\Phi}} = 500\,\,{\rm{mm}}$ ,孔径间隔$d = 10\,\,{\rm{mm}}$ ,焦距$f = 700\,\,{\rm{mm}}$ 。如图3(aI)所示,对等效口径的光瞳进行建模,记为Circle型,其远场衍射强度分布I0如图3(aII)所示,将其用于归一化处理。然后建立如图3(bI)、3(cI)、3(dI)所示的拼接主镜光瞳模型,分别记为Hexagon Sector型、Hexagon型和Circle 7型,其远场衍射对应的强度分布I(I1、I2、I3)分别如图3(bII)、3(cII)、3(dII)所示。由于拼接式主镜的光瞳形状接近圆形,对应的点扩散函数的中心亮斑也接近于圆形。但是由于孔径形状不同,对应的点扩散函数的旁瓣能量分布不同,其衍射效应也不同。为了进一步分析孔径形状对衍射效应的影响,一方面,数值仿真了如图4所示的拼接主镜(不同孔径形状)远场衍射的点扩散函数归一化相对强度(
$I/{I_0}$ )曲线;另一方面,利用几何关系求得不同孔径形状的构型参数(其中,填充因子是有效孔径和等效孔径的比值:$F = S/{S_0}$ ;斯特列尔比是指所求点扩散函数的峰值强度与完美的衍射极限点扩散函数的峰值强度之比)如表1所示。从表1可知,拼接主镜的填充因子越高,斯特列尔比的值越大,系统成像性能越好。表 1 拼接主镜(不同孔径形状)构型参数及衍射效应Table 1. Configuration parameters and diffraction effects of segmented primary mirror (different aperture shapes)主镜构型 中心镜外接圆
直径/mm填充因子 斯特列尔比 Circle 500 1 1 Hexagon Sector
(d=10 mm)238 0.958 0.842 3 Hexagon
(d=10 mm)183 0.775 0.608 2 Circle 7
(d=10 mm)160 0.716 0.516 7 3.2 孔径间隔对衍射效应的影响
以Circle 7型拼接主镜为例,假设等效口径为
${\mathit{\Phi}} = 500\,\,{\rm{mm}}$ ,焦距$f = 700\,\,{\rm{mm}}$ 。如图5(aI)、5(bI)、5(cI)、5(dI)所示,建立孔径间隔d=0、d=10 mm、d=50 mm、d=100 mm的拼接主镜光瞳模型,其远场衍射的强度分布(I1、I2、I3、I4)如图5(aII)、5(bII)、5(cII)、5(dII)所示。以孔径间隔d=0时远场衍射的强度分布I1为基准作归一化处理,得到如图5(e)所示的点扩散函数的归一化相对强度曲线。从图5可知,随着主镜孔径间隔的增大,对应的点扩散函数的旁瓣能量增大,能量集中度下降,系统成像质量下降。为了进一步分析孔径间隔对衍射效应的影响,利用几何关系求得如表2所示的不同孔径间隔的构型参数。综合分析可知:在等效口径固定的前提下,主镜孔径间隔增大,一方面使旁瓣能量增加,能量集中度下降;另一方面使每个孔径的直径减小,填充因子变小。二者共同作用使成像质量下降。当孔径间隔小于3 mm时,斯特列尔比大于0.95;当孔径间隔小于6 mm时,斯特列尔比大于0.9;当孔径间隔小于12 mm时,斯特列尔比大于0.8。
表 2 拼接主镜(不同孔径间隔)构型参数及其衍射效应Table 2. Configuration parameters and diffraction effects of segmented primary mirror (different aperture intervals)主镜间隔d/mm 孔径直径D/mm 填充因子 斯特列尔比 0 166 0.771 1 5 163 0.743 0.913 10 160 0.716 0.855 20 153 0.655 0.739 50 133 0.495 0.447 100 100 0.280 0.128 4 平移误差对衍射效应的影响
${\rm{Piston}}$ 误差是指子镜沿光轴方向(${\textit{z}}$ 轴)发生平移所引起的波前误差(其为子镜平移误差的2倍)。以Circle 7型拼接主镜为例,假设等效口径${\mathit{\Phi}} = 500\,\,{\rm{mm}}$ ,孔径间隔$d = 10\,\,{\rm{mm}}$ ,焦距$f = 700\,\,{\rm{mm}}$ 。首先通过仿真子镜1(见图2)在不同${\rm{piston}}$ 误差下拼接主镜的点扩散函数及其归一化相对强度来分析平移误差对衍射效应的影响。如图6所示,当${\rm{piston}} = 0$ 时,此时光强最集中、光最强(将其用于计算归一化相对强度)。随着平移误差的增大,能量分布逐渐下移,导致中心光斑能量逐渐降低。当piston = 0.5λ时,光斑上下呈对称分布,能量相等,但都小于${\rm{piston}} = 0$ 时的光强。随着平移误差进一步增大,能量分布继续下移,直到piston = 1λ时,形成一个与${\rm{piston}} = 0$ 时能量分布一样的光斑。平移误差超过一个波长后,衍射光斑呈周期性变化。为了进一步分析平移误差对衍射效应的影响,进行拼接主镜整体平移误差的研究。以中心镜为基准,子镜的
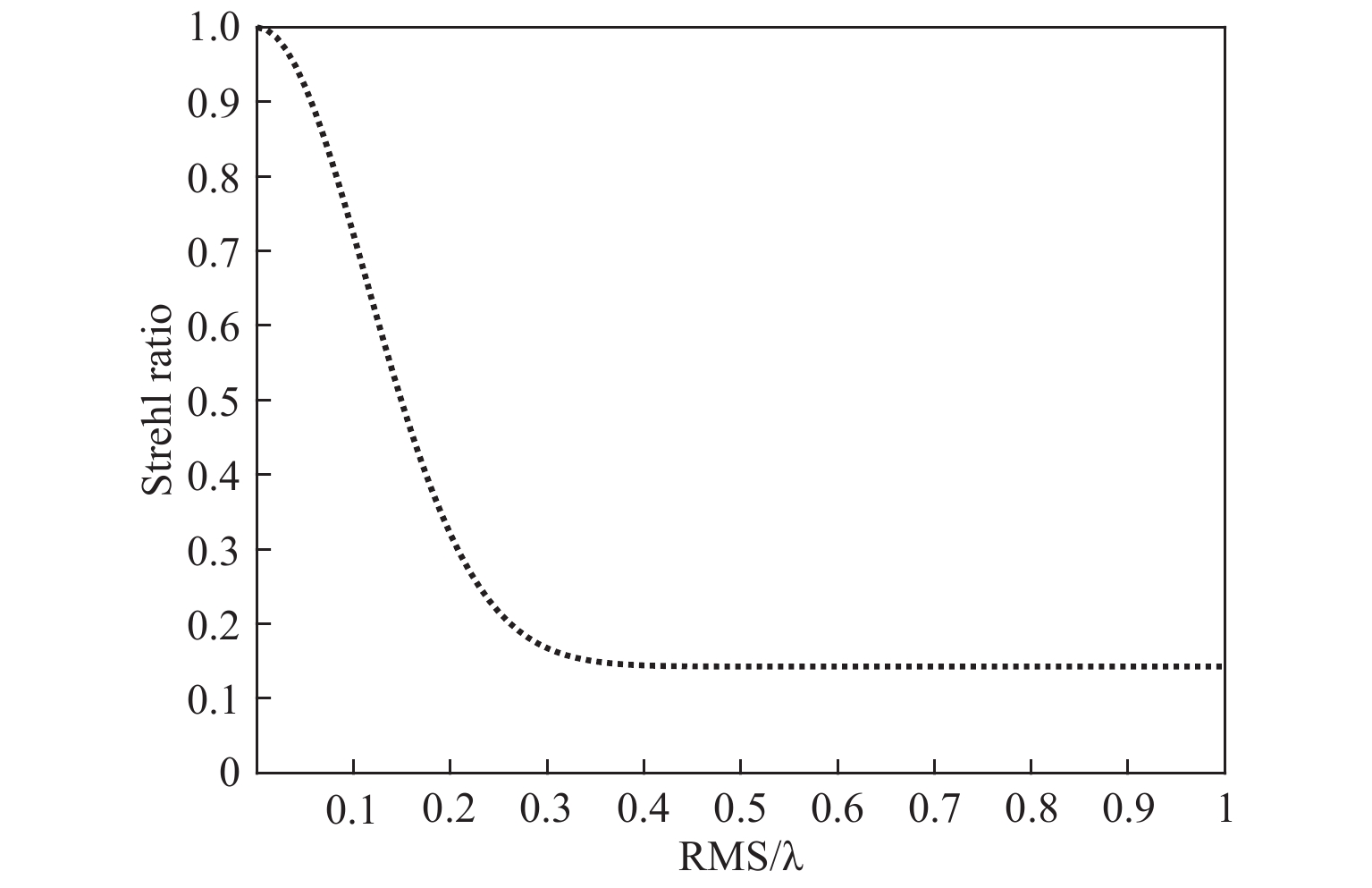
${\rm{piston}}$ 误差的均方根值(root mean square,RMS)为$\sigma $ ,假设各子镜的${\rm{piston}}$ 误差服从$(0,{\sigma ^2})$ 高斯分布,拼接主镜斯特列尔比$S$ 与子镜${\rm{piston}}$ 误差均方根值$\sigma $ 的关系表达式为[10]$$S = \frac{1}{N}\left[ {1 + (N - 1)\exp ( - {\sigma ^2})} \right]$$ (8) 根据表达式进行数值仿真
$(N = 7)$ ,仿真结果如图7所示。由图7可见,随着${\rm{piston}}$ 误差均方根值的不断增大,斯特列尔比呈下降趋势,最终稳定在$1/7 \approx 0.142$ 。当${\rm{piston}}$ 误差的均方根值小于0.039λ时,斯特列尔比大于0.95;当${\rm{piston}}$ 误差的均方根值小于0.056λ时,斯特列尔比大于0.9;当${\rm{piston}}$ 误差的均方根值小于0.082λ时,斯特列尔比大于0.8。5 倾斜误差对衍射效应的影响
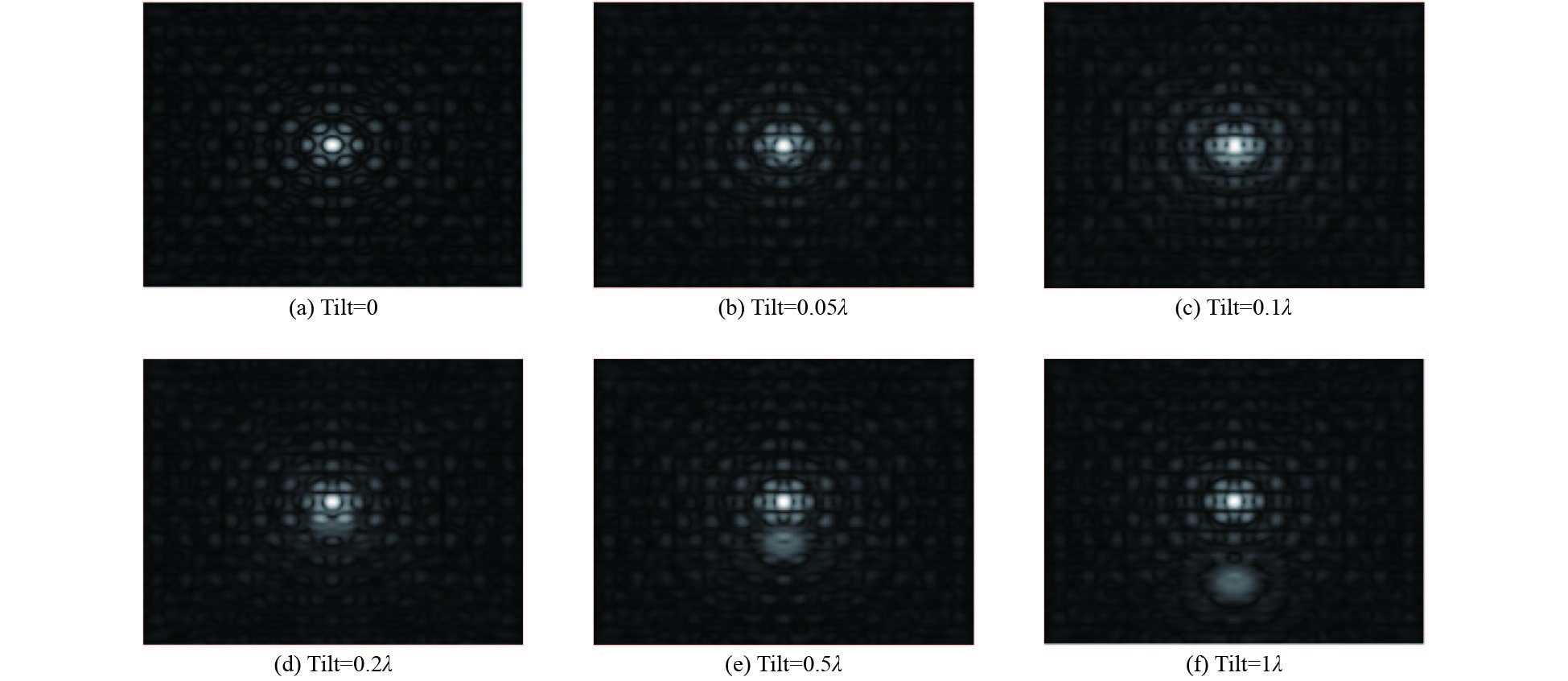
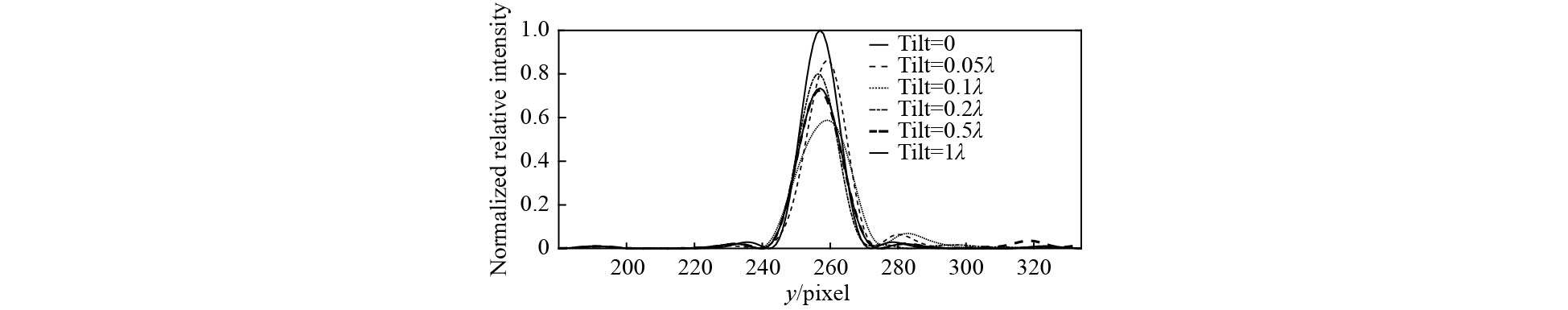
${\rm{Tip}}/{\rm{tilt}}$ 误差是指子镜绕$x,y$ 轴旋转所引起的波前误差。以Circle 7型拼接主镜为例,假设等效口径为${\mathit{\Phi}} = 500\,\;{\rm{mm}}$ ,孔径间隔为$d = 10\;\,{\rm{mm}}$ ,焦距为$f = 700\,\,{\rm{mm}}$ 。以${\rm{tilt}}$ 方向的波前误差为例,通过仿真子镜1(见图2)在不同${\rm{tilt}}$ 误差下拼接主镜的点扩散函数及其归一化相对强度来分析倾斜误差对衍射效应的影响,如图8所示。当${\rm{tilt}} = 0$ 时,此时光强最集中、光最强,将其用于计算归一化相对强度。随着倾斜误差逐渐增大,由于干涉效应,中心光斑能量逐渐减小。当倾斜误差进一步增大,中心光斑能量先是增加(小于${\rm{tilt}} = 0$ 时的能量),然后减小,同时下方产生新的光斑并且逐渐远离中心光斑。为了进一步分析倾斜误差对衍射效应的影响,下面进行拼接主镜整体倾斜误差的研究。子镜的
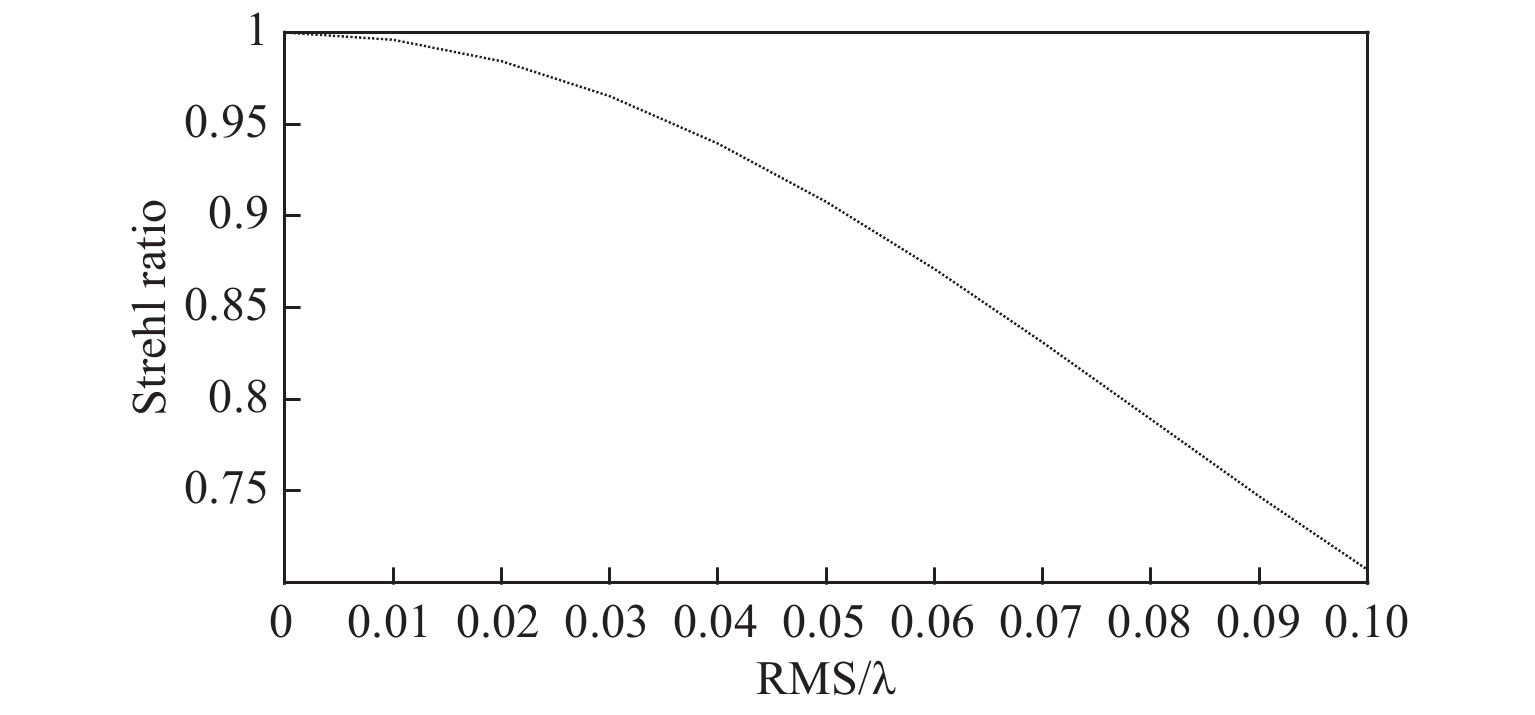
${\rm{tip{\text{-}}tilt}}$ 误差的均方根值为$\sigma $ ,假设各子镜的${\rm{tip{\text{-}}tilt}}$ 误差服从$(0,{\sigma ^2})$ 高斯分布,当$\sigma $ 很小时,拼接主镜斯特列尔比$S$ 与子镜${\rm{tip{\text{-}}tilt}}$ 误差均方根值$\sigma $ 的关系表达式为[11]$$S \approx 1 - {\sigma ^2} + \frac{{{\sigma ^4}}}{4}(1 + {\gamma '} + \frac{2}{N})$$ (9) 式中
${\gamma '}$ 为形状因子,决定于孔径的几何形状。对于圆形孔径,${\gamma '}$ 为1.33。根据表达式进行数值仿真$(N = 7)$ ,结果如图9所示。由图9可以看出,随着${\rm{tip{\text{-}}tilt}}$ 误差均方根值的不断增大,斯特列尔比呈下降趋势。当${\rm{tip{\text{-}}tilt}}$ 误差的均方根值小于0.036λ时,斯特列尔比大于0.95;当${\rm{tip{\text{-}}tilt}}$ 误差的均方根值小于0.052λ时,斯特列尔比大于0.9;当${\rm{tip{\text{-}}tilt}}$ 误差的均方根值小于0.077λ时,斯特列尔比大于0.8。6 结论
本文基于标量衍射研究了拼接式光学系统的成像原理,采用齐次坐标变换建立了等效口径
${\mathit{\Phi}} = 500\,\,{\rm{mm}}$ 、不同孔径形状、不同孔径间隔的光瞳模型。利用点扩散函数及其归一化相对强度、斯特列尔比等远场特性详细分析了拼接主镜的构型、平移误差、倾斜误差对衍射效应的影响。分析结果显示:对于不同构型的拼接主镜,其衍射效应受拼接主镜的填充因子和孔径间隔共同影响,填充因子越高,孔径间隔越小,系统成像质量越好;对于单个子镜,平移误差对远场衍射的影响具有周期性;对于拼接主镜整体,当子镜${\rm{piston}}$ 误差的均方根值小于0.039λ时,斯特列尔比大于0.95;当子镜${\rm{tip{\text{-}}tilt}}$ 误差的均方根值小于0.036λ时,斯特列尔比大于0.95。分析结果对未来拼接式望远镜成像性能分析、主镜的构型设计、平移误差和倾斜误差的检测与调整等具有一定的指导意义。 -
表 1 拼接主镜(不同孔径形状)构型参数及衍射效应
Table 1 Configuration parameters and diffraction effects of segmented primary mirror (different aperture shapes)
主镜构型 中心镜外接圆
直径/mm填充因子 斯特列尔比 Circle 500 1 1 Hexagon Sector
(d=10 mm)238 0.958 0.842 3 Hexagon
(d=10 mm)183 0.775 0.608 2 Circle 7
(d=10 mm)160 0.716 0.516 7 表 2 拼接主镜(不同孔径间隔)构型参数及其衍射效应
Table 2 Configuration parameters and diffraction effects of segmented primary mirror (different aperture intervals)
主镜间隔d/mm 孔径直径D/mm 填充因子 斯特列尔比 0 166 0.771 1 5 163 0.743 0.913 10 160 0.716 0.855 20 153 0.655 0.739 50 133 0.495 0.447 100 100 0.280 0.128 -
[1] 张学军, 樊延超, 鲍赫, 等. 超大口径空间光学遥感器的应用与发展[J]. 光学精密工程,2016,24(11):2613-2626. doi: 10.3788/OPE.20162411.2613 ZHANG Xuejun, FAN Yanchao, BAO He, et al. Applications and development of ultra large aperture space optical remote sensors[J]. Optics and Precision Engineering,2016,24(11):2613-2626. doi: 10.3788/OPE.20162411.2613
[2] 徐萌萌, 薛栋林, 曾雪锋. 大口径超长焦距紧凑型光学系统设计[J]. 应用光学,2018,39(5):52-57. doi: 10.5768/JAO201839.0501010 XU Mengmeng, XUE Donglin, ZENG Xuefeng. Design of compact optical system with large aperture and ultra long focal length[J]. Journal of Applied Optics,2018,39(5):52-57. doi: 10.5768/JAO201839.0501010
[3] 李全超, 李蕾, 谭淞年, 等. 大口径铝合金主反射镜设计与分析[J]. 应用光学,2016,37(3):337-341. doi: 10.5768/JAO201637.0301003 LI Quanchao, LI Lei, TAN Songnian, et al. Design and analysis for large aperture primary aluminum mirrors[J]. Journal of Applied Optics,2016,37(3):337-341. doi: 10.5768/JAO201637.0301003
[4] 周程灏, 王治乐, 朱峰. 大口径光学合成孔径成像技术发展现状[J]. 中国光学,2017(1):25-38. ZHOU Chenghao, WANG Zhile, ZHU Feng. Review on optical synthetic aperture imaging technique[J]. Chinese Optics,2017(1):25-38.
[5] NELSON J. Segmented mirror telescopes[M]//Optics in Astrophysics. Dordrecht:Springer, 2006: 61-72.
[6] FANSON J, McCARTHY P J, BERNSTEIN R, et al. Overview and status of the Giant Magellan telescope project[C]//Ground-based and airborne telescopes VⅡ. USA: International Society for Optics and Photonics, 2018: 1070012.
[7] NELSON J, SANDERS G H. The status of the thirty meter telescope project[C]//Ground-based and airborne telescopes Ⅱ. USA: International Society for Optics and Photonics, 2008: 70121A.
[8] TAMAI R, CIRASUOLO M, GONZÁLEZ J C, et al. The E-ELT program status[C]//Ground-based and airborne telescopes VI. USA: International Society for Optics and Photonics, 2016: 99060W.
[9] GREENHOUSE M. The James webb space telescope: mission overview and status[C]//2016 IEEE Aerospace Conference.USA: IEEE, 2016: 1-11.
[10] CHANAN G, TROY M. Strehl ratio and modulation transfer function for segmented mirror telescopes as functions of segment phase error[J]. Applied Optics,1999,38(31):6642-6647. doi: 10.1364/AO.38.006642
[11] YAITSKOVA N, DOHLEN K. Tip-tilt error for extremely large segmented telescopes: detailed theoretical point-spread-function analysis and numerical simulation results[J]. JOSA A,2002,19(7):1274-1285. doi: 10.1364/JOSAA.19.001274
[12] ROBERTS Jr L C, PERRIN M D, MARCHIS F, et al. Is that really your Strehl ratio?[C]//Advancements in Adaptive Optics. USA: International Society for Optics and Photonics, 2004: 504-515.
[13] JIANG F, JU G, QI X, et al. Cross-iteration deconvolution strategy for differential optical transfer function (dOTF) wavefront sensing[J]. Optics Letters,2019,44(17):4283-4286. doi: 10.1364/OL.44.004283
[14] YUE D, XU S, NIE H. Co-phasing of the segmented mirror and image retrieval based on phase diversity using a modified algorithm[J]. Applied Optics,2015,54(26):7917-7924. doi: 10.1364/AO.54.007917
[15] 李毅超, 康福增, 王昊. 拼接镜成像性能分析[J]. 红外与激光工程,2019,48(1):118006-6. doi: 10.3788/IRLA201948.0118006 LI Yichao, KANG Fuzeng, WANG Hao. Imaging property analysis of segmented mirror[J]. Infrared and Laser Engineering,2019,48(1):118006-6. doi: 10.3788/IRLA201948.0118006
[16] GOODMAN J W. Introduction to Fourier optics[M].Colorado: Roberts and Company Publishers, 2005.
[17] BORN M, WOLF E. Principles of optics: electromagnetic theory of propagation, interference and diffraction of light[M]. Amsterdam: Elsevier, 2013.
[18] Malacara D. Optical shop testing[M].NYSE: John Wiley & Sons, 2007.
-
期刊类型引用(1)
1. 张雅琼,郭建都,徐阳,郝小健,陈光辉,周婧,朱晶晶. 快速周扫探测系统扫描平台的高精度稳定控制. 应用光学. 2022(03): 375-385 .  本站查看
本站查看
其他类型引用(5)



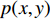
 下载:
下载:
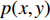
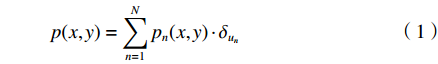
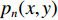
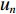
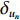
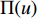

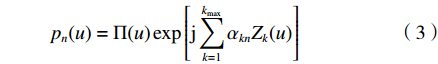
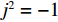
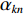


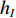
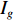
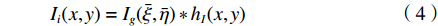
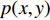
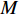

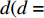
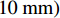
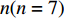
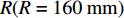
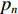


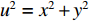
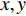
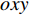
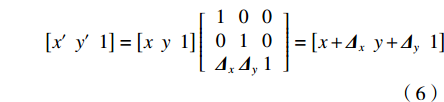
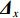


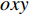
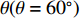
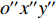
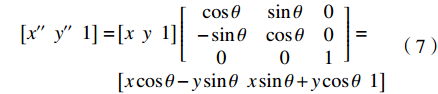
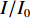
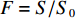


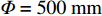
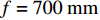



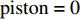
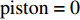
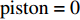

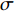

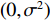

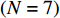

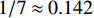




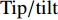

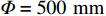
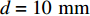
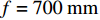
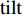
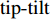
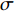
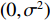
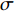
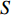
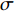





 陕公网安备 61011302001501号
陕公网安备 61011302001501号 