Focal length measurement for thin convex lens based on parallel tube method
-
摘要: 薄凸透镜是光学仪器中最重要、最基本的元件，在天文、军事、医学等众多领域发挥着重要作用。焦距是薄透镜、反射镜等光学系统最重要的特性参量，因而准确测量薄透镜的焦距则显得尤为重要。实验室测量薄凸透镜焦距的方法有物距像距法、自准直法、光电法、平行光管法等。由于采用前3种方法测量透镜焦距的精度偏低，针对该问题，提出利用平行光管法测量薄凸透镜的焦距，并对实验误差作简单分析。实验结果表明，该方法可以高精度地测量薄凸透镜焦距，相对误差仅为0.138%。因此，采用平行光管法的薄凸透镜焦距测量方法是有效可行的。Abstract: Thin convex lenses are the most important and basic components of optical instruments. It plays an important role in many fields such as astronomy, military and medicine. The focal length is the most important characteristic parameter of optical systems such as thin lens and mirrors. Hence, it is particularly important to accurately measure the focal length of the thin lens. The methods for measuring focal length of thin convex lens are the object/image distance method, self-collimation method, optical-electrical method and parallel tube method in laboratory. Aiming at the problem of low accuracy of measuring the lens focal length by the first three methods, the parallel tube method was adopted to measure the focal length of thin convex lens and the experimental error was analyzed simply. Experimental result shows that, the method can measure the focal length of thin convex lens with high precision, the relative error is only 0.138 %. Therefore, the method for measuring focal length of thin convex lens by parallel tube method is effective and feasible.
-
Keywords:
- thin convex lenses /
- focal length /
- parallel tube method
-
引言
凸透镜焦距的测量是大学《应用光学》课程中必做的实验之一，测量方法有：物距像距法、自准直法、光电法、平行光管法[1-6]。采用前3种方法测量薄凸透镜焦距时，光路比较简单，易于操作，学生容易掌握和理解。但这些方法中都需要用透镜的位置、成清晰像的位置、物的位置等参量，经运算得到透镜的焦距。此外，物距像距法和自准直法所得结果的准确度还受到人眼主观观察判断能力及像差的限制；光电法虽然能解决由像距的景深所引入的系统误差，但其测量精度还与透镜光心是否与支杆中心处于同一垂直于导轨平面有关，因此测量精度都偏低[7-10]。为了提高凸透镜焦距的测量精度，丰富透镜焦距的测量手段，可采用平行光管法测量透镜的焦距[11]。
1 基于平行光管法的薄凸透镜焦距测量原理
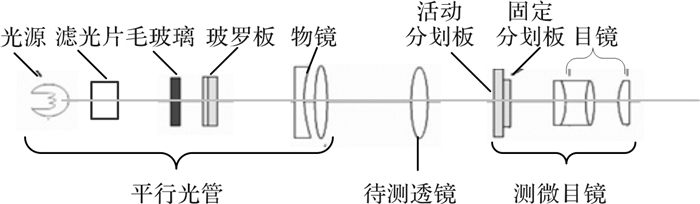
平行光管是一种能发射平行光束的精密的光学仪器，它有一个质量优良的准直物镜L0，其焦距是经过精确测定的[12-14]。本实验中所用的平行光管，其物镜焦距为143 mm(数值由厂家提供)。其焦距仪光学系统主要结构如图 1所示。
由图 1可以看出，测量透镜焦距时，平行光管以白炽灯作为光源，用滤光片来收窄光源参与成像光谱，用毛玻璃将不均匀的面光源转换成均匀的面光源照射到玻罗板上。玻罗板置于物镜的物方焦平面上，其上刻有5对平行线，如图 2所示。每对平行线中心的线距分别是20、10、4、2、1(单位：mm)。因此，从物镜发出的光为平行光束。平行光束经待测透镜后成清晰像于目镜的分划板上，只需测出玻罗板线对的像高，即可算出待测透镜的焦距。
![]()
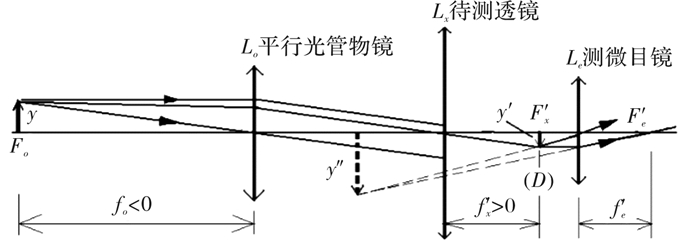
图 2 玻罗板的平行线及线距标称值Figure 2. Porro board's parallel lines and nominal values of its interval本实验利用物像之间的比例关系测量透镜的焦距。用平行光管法测量凸透镜焦距的光路图如图 3所示。由物点(物高为y)发出的光经平行光管物镜Lo后成为平行光，它与光轴夹角的正切为y/fo，该光束经待测透镜Lx后成像在其焦平面上，像高为y′。从图中的几何关系可以看出待测透镜的焦距f′x为
![]()
图 3 平行光管法测量凸透镜焦距的光路图Figure 3. Convex lens' focal length measurement optical path based on parallel tube method$$ f^{\prime}_{x}=-\frac{y^{\prime}}{y} \cdot f^{\prime}_o $$ (1) 式中：f′o为平行光管物镜的焦距，其数值已标在平行光管(标称值为143 mm)；y为玻罗板上某一线对的间距；y′为用测微目镜测得的同一线对像的间距，y′ < 0；f′x为待测凸透镜的焦距。
2 实验步骤
本实验中各元件的等高共轴调节极为重要，若共轴调节不准，在测微目镜中就可能观察不到玻罗板中某一线对的像。因此，等高共轴是整个实验的关键之处。等高共轴的调节要点如下：
1) 粗调。分别使透镜光轴、“物”的中心、像屏中心及测微目镜光轴基本在平行光管光轴上；
2) 共轴调节的“大像追小像”法。在光具座上依次放置“物”、透镜和像屏，使“物”到像屏的距离大于6倍焦距估值[15]。透镜沿光具座平移时像屏上会出现一大一小两次清晰的像。先记住屏上小像中心位置，再在屏上出现大像时，上下左右细调“物”或者对透镜做转动等调节，使大像的中心趋近原先的小像中心位置，称为“大像追小像”。再观察小像位置，再次使大像追小像。经过几轮调节使大小像中心重合，说明“物”的中心已经与透镜共轴。
先旋转调节目镜，看清叉丝，然后仔细调节透镜与测微目镜间距，使玻罗板线对的像与叉丝基本消视差，再用小力偶矩轻轻地单向旋转测微目镜的鼓轮，使叉丝依次对准玻罗板线对的两条线中心，分别记下测微目镜的读数值y′1和y′2，y′=-|y′1-y′2 |，计算出焦距f′x=-f′o y′/y。
3 凸透镜焦距测量实验及精度分析
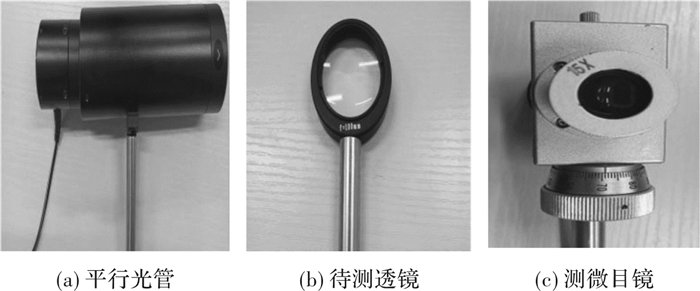
为验证本文方法测量凸透镜焦距的可行性，在WZG型多功能积木式组合光谱仪光学实验平台上，使用搭建的焦距仪光学系统(图 4)来测量待测透镜焦距。其中，图 5(a)为平行光管；图 5(b)为待测透镜，其焦距标称值为100 mm；图 5(c)为测微目镜，其倍率为15倍。
用平行光管法测量待测凸透镜的焦距，测量次数为6次，实际测量结果如表 1所示，其中选取玻罗板线对间距y=4 mm，f′o=143 mm，y′1、y′2分别为刻线对两条刻线在测微目镜中对应的读数。对6次待测透镜焦距值取平均值，求得待测透镜焦距的平均值f′x=99.862 mm。实验结果可以看出，采用平行光管法可以测量薄凸透镜的焦距。
表 1 平行光管法测量的数据记录mm Table 1. Data records of parallel tube method measurements次数 1 2 3 4 5 6 y′1 2.280 2.220 2.240 2.210 2.280 2.230 y′2 5.050 5.020 5.040 5.020 5.050 5.040 y′=-|y′1-y′2| -2.770 -2.800 -2.800 -2.810 -2.770 -2.810 $f_{x}^{\prime}=-\frac{y^{\prime}}{y} \cdot f_{o}^{\prime} $ 99.028 100.100 100.100 100.458 99.028 100.458 为验证平行光管法测量凸透镜焦距的精确性，将其与物距像距法、自准直法、光电法相比，以同一待测透镜为研究对象，其中，a)物距像距法：f′x=98.650 mm；b)自准直法：f′x=101.452 mm；c)光电法：100.190 mm；d)平行光管法：f′x=99.862 mm。通过数据可以看出，采用平行光管法测得焦距的相对误差最小，仅为0.138%。结果表明，平行光管法可以高精度地测量薄凸透镜的焦距。
采用平行光管法测量凸透镜焦距时，测量结果的不确定度分量有：1)测y′的测微目镜的仪器误差限；2)平行光管物镜焦距的不确定度；3)玻罗板线对间距的误差影响，可忽略不计。
4 结论
针对薄凸透镜焦距的测量，结合平行光管法的有关理论，提出了用平行光管法测量薄凸透镜焦距。该方法在一定程度上提高了薄凸透镜焦距测量的测量精度，并通过实验验证了该方法的可行性、有效性、精确性，对透镜焦距测量的研究及应用具有一定的实际意义。
-
![]()
图 2 玻罗板的平行线及线距标称值
Figure 2. Porro board's parallel lines and nominal values of its interval
![]()
图 3 平行光管法测量凸透镜焦距的光路图
Figure 3. Convex lens' focal length measurement optical path based on parallel tube method
表 1 平行光管法测量的数据记录
mm Table 1 Data records of parallel tube method measurements
次数 1 2 3 4 5 6 y′1 2.280 2.220 2.240 2.210 2.280 2.230 y′2 5.050 5.020 5.040 5.020 5.050 5.040 y′=-|y′1-y′2| -2.770 -2.800 -2.800 -2.810 -2.770 -2.810 $f_{x}^{\prime}=-\frac{y^{\prime}}{y} \cdot f_{o}^{\prime} $ 99.028 100.100 100.100 100.458 99.028 100.458 
下载: 导出CSV
-
[1] 张伟, 张晓辉, 刘振江.光具座测量透镜焦距的自动调焦方法研究[J].应用光学, 2002, 23(6): 25-28. doi: 10.3969/j.issn.1002-2082.2002.06.007 ZHANG Wei, ZHANG Xiaohui, LIU Zhenjiang. Study on method of automatic focalization to measure focal length of lens on light-utensil seat[J]. Journal of Applied Optics, 2002, 23(6): 25-28. doi: 10.3969/j.issn.1002-2082.2002.06.007
[2] 陈舟, 倪敏.平凸透镜焦距的测量与研究[J].物理通报, 2018, 37(4): 71-78. doi: 10.3969/j.issn.0509-4038.2018.04.018 CHEN Zhou, NI Min. Measurement and research on focal length of planoconvex lens[J]. Physics Bulletin, 2018, 37(4) : 71-78. doi: 10.3969/j.issn.0509-4038.2018.04.018
[3] YANG Guoqing, MIAO Liang, ZHANG Xin, et al. High-accuracy measurement of the focal length and distortion of optical systems based on interferometry.[J]. Applied Optics, 2018, 57(18): 5217-5223. doi: 10.1364/AO.57.005217
[4] 黄阳, 王春雨, 牛锦川, 等.长焦红外光学系统焦距的高精度测量技术[J].应用光学, 2017, 38(6): 995-998. http://www.yygx.net/CN/abstract/abstract11049.shtml HUANG Yang, WANG Chunxue, NIU Jinchuan, et al. High-precision measurement of focal length for long-focus infrared optical system[J]. Journal of Applied Optics, 2017, 38(6): 995-998. http://www.yygx.net/CN/abstract/abstract11049.shtml
[5] LU Zhangji, CAI Lilong. Paraxial focal length measurement method with a simple apparatus[J]. Optics express, 2019, 27(3): 2044-2055. doi: 10.1364/OE.27.002044
[6] 李林, 黄一帆.应用光学[M].北京:北京理工大学出版社, 2017. LI Lin, HUANG Yifan. Applied Optics [M]. Beijing: Beijing Institute of Technology Press, 2017.
[7] PASINETTI S, BODINI I, LANCINI M, et al. Automatic selection of focal lengths in a depth from defocus measurement system based on liquid lenses[J]. Optics and Lasers in Engineering, 2017, 96: 68-74. doi: 10.1016/j.optlaseng.2017.04.011
[8] PRAKASH, O M. Comparison of uncertainties in the measurement of focal length of convex lens using nodal point and magnification methods[C].[S.l]: SPIE, 2002: 47-56.
[9] 蒋正东, 朱荣刚, 陈磊, 等.基于双朗奇光栅的焦距测量技术研究[J].应用光学, 2018, 39(5): 687-690. http://www.yygx.net/CN/abstract/abstract11169.shtml JIANG Zhengdong, ZHU Ronggang, CHEN Lei, et al. Research on focal length measurement based on double ronchi gratings[J]. Journal of Applied Optics, 2018, 39(5): 687-690. http://www.yygx.net/CN/abstract/abstract11169.shtml
[10] CHANG C W, SU D C. An improved technique of measuring the focal length of a lens[J]. Opt. Commun., 1989, 73(4): 257-262. doi: 10.1016/0030-4018(89)90213-7
[11] LEE Juyoung, HONG Byunghee, KIM Wooyoun, et al. Near-field focusing and magnification through self-assembled nanoscale spherical lenses[J]. Nature, 2009, 460(7254): 456-456. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0211304608/
[12] CHEN Lingfeng, MENG Xiaojien, XIAO He, et al. Experimental ray-tracing with point diffraction interferometry and its application in focal length measurement[J]. Applied Optics, 2018, 57(29): 8648-8653. doi: 10.1364/AO.57.008648
[13] CAO Binhxuan, HOANG Phuongle, AHN Sanghoon, et al. Measurement of focal length based on laser-beam-spot tracking system using diffractive beam sampler[J]. Measurement, 2018, 122: 135-140. doi: 10.1016/j.measurement.2018.03.011
[14] DONG Shengsheng, HE Bingwei, LIN Chang, et al. Calibration method for a structured light measurement system with two different focal length cameras[J]. Measurement, 2015, 73: 462-472. doi: 10.1016/j.measurement.2015.05.033
[15] JIN Xiaorong, ZHANG Jinchun, BAI Jian, et al. Calibration method for high-accuracy measurement of long focal length with Talbot interferometry[J]. Applied Optics, 2012, 51(13): 2407-2413. doi: 10.1364/AO.51.002407
-
期刊类型引用(4)
1. 刘坤，李克武，王爽，王志斌，张易琨. 弹光调制器动态参数测量与高效驱动匹配研究. 应用光学. 2024(02): 415-421 . 
本站查看
2. 杨军营，韩培高，魏莹莹. 无频响影响的光弹调制器定标新方法. 中国激光. 2024(08): 127-133 . 百度学术
3. 刘坤，李克武，李坤钰，王爽，王志斌. 弹光调制器相频特性分析与稳定控制技术. 激光杂志. 2024(07): 36-41 . 百度学术
4. 臧晓阳，李克武，王志斌，李坤钰，梁振坤，刘坤. 快轴可调弹光调制器闭环稳定控制研究. 激光与光电子学进展. 2023(07): 329-336 . 百度学术
其他类型引用(4)
计量
- 文章访问数: 2304
- HTML全文浏览量: 809
- PDF下载量: 131
- 被引次数: 8
陕公网安备 61011302001501号 