Weighted on-orbit calibration method of principal point and focal length for star sensor
-
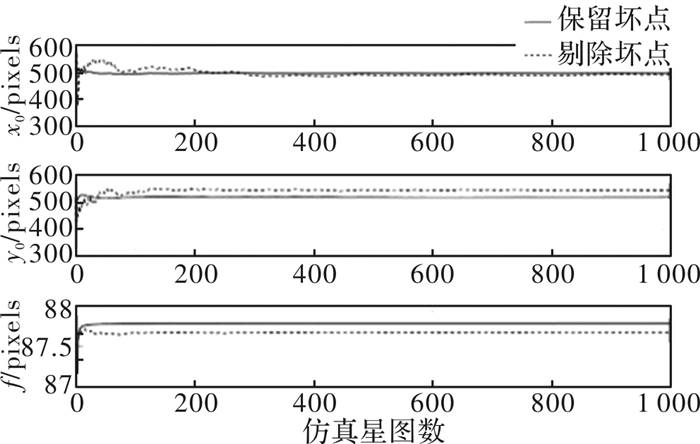
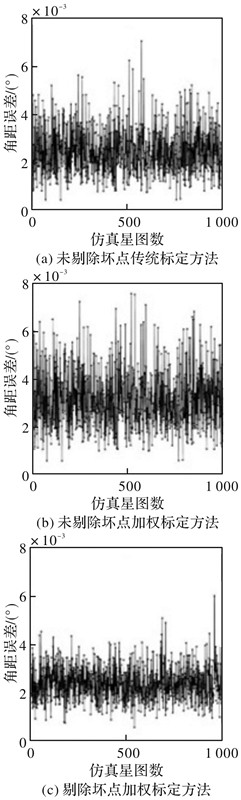
摘要: 星敏感器作为卫星姿态测量装置，其在轨服役过程中，主点和焦距的标定精度是影响其姿态输出精度的主要因素。针对标定过程中含有随机测量噪声偏大的星像点，导致星敏感器主点和焦距的标定结果产生较大偏差的问题，提出了一种星敏感器主点和焦距的加权在轨标定方法。该方法首先建立了星敏感器在轨标定模型，然后引入合理的标定权值，加入到最小二乘估计主点和焦距的过程中，寻找并剔除随机测量噪声偏大的星点，最后将加权估计出的结果作为测量，采用扩展卡尔曼滤波对星图进行处理。仿真结果表明，在星点位置存在较大误差的情况下，该方法能剔除随机测量噪声偏大的坏点。星内角距统计偏差约为传统方法的1/10，与真值相比标定参数精度分别为0.219 9像素、0.148 7像素、3.38 μm。Abstract: As a satellite attitude measurement device, the calibration accuracy of the star sensor's principal point and focal length serves as the main factor affecting the accuracy of its attitude output during the on-orbit service procession. If the calibration process contains the star image point with large random measurement noise, this will lead to large deviation of the calibration result of the star sensor's main point and focal length. Aiming at this problem, a weighted on-orbit calibration method of principal point and focal length for star sensor was proposed. Firstly, the method established the on-orbit calibration model of the star sensor.Then we added a reasonable calibration weight to the least squares estimation. In this way, we found and removed the points with large random measurement noise. Finally, the weighted least-squares estimation result was served as the measurement, and the extended Kalman filter was used to process the star image. The simulation results show that, if there is a large error in the position of the star point, this method can remove the bad points where the random measurement noise is too large. The statistical deviation of the angular distance is about 1/10 of the traditional method. Compared with the true value, the accuracy of the calibration parameters is 0.219 9 pixel, 0.148 7 pixel and 3.38 μm, respectively.
-
Keywords:
- star sensor /
- on-orbit calibration /
- weighted method /
- least squares estimation /
- Kalman filter
-
引言
星敏感器是一种以恒星为参照系，以星空为工作对象的高精度空间姿态敏感器, 被广泛应用于卫星控制中，其主点和焦距的精确校准是实现其姿态测量的重要步骤[1-3]。在实际的飞行任务中，由于发射时的震动和冲击、以及在恶劣太空环境下和长期工作后的磨损[4-5]，其主点和焦距相对地面标定时发生很大的变化，会导致星敏感器导航精度下降。因此为了保证星敏感器的观测精度和可靠性，必须对其主点和焦距进行在轨标定。
传统的在轨标定方法[6-9]均是在星像点随机测量噪声较小的条件下进行的，这些方法在随机测量噪声偏大时，并不能很好地选择标定星点，从而导致最终标定结果偏差较大。
针对传统方法的不足，本文提出了一种星敏感器主点和焦距的加权标定方法。新的方法在迭代计算过程中引入合理的权值，通过计算权重的大小来达到剔除测量噪声较大的星点。在剔除坏点后，重新加权计算得到主点和焦距的最终结果。
1 星敏感器在轨测量模型
星敏感器的理想成像模型为针孔模型[10]，恒星产生的星光经过星敏感器光学系统后所成的像在CCD面阵上会形成一个光斑，如图 1所示。
若星敏感器的焦距为f，恒星在星敏感器成像平面坐标系下投影点中心坐标为(xi, yi)，在考虑主点位置误差的情况下，恒星i在星敏感器成像平面坐标系Oc-XcYcZc下的单位方向矢量可表示为
$$ {w_i} = \frac{1}{{\sqrt {{{\left( {{x_i} - {x_0}} \right)}^2} + {{\left( {{y_i} - {y_0}} \right)}^2} + {f^2}} }}\left[ {\begin{array}{*{20}{c}} { - \left( {{x_i} - {x_0}} \right)}\\ { - \left( {{y_i} - {y_0}} \right)}\\ f \end{array}} \right] $$ (1) 式中：x0与y0表示主点的待标定量；xi与yi表示第i颗恒星在星敏感器图像坐标系下的位置坐标。
在地心赤道惯性坐标系中星光矢量可以表示为
$$ {v_i} = \left[ {\begin{array}{*{20}{c}} {\cos {\alpha _i}\cos {\delta _i}}\\ {\sin {\alpha _i}\sin {\delta _i}}\\ {\sin {\delta _i}} \end{array}} \right] $$ (2) 式中：αi与δi分别表示第i颗星的赤经和赤纬。
从(1)式中可以看出，相机的主点和焦距的估计精度与星点在星敏感器图像坐标系下的坐标有着密不可分的关系，因此对星点位置的准确估计就显得尤为重要。
2 星敏感器在轨标定模型
星敏感器成像平面坐标系中星光矢量夹角θij如图 2所示。
取星光矢量夹角θij的方向余弦，在地心赤道惯性坐标系与成像平面坐标系中，有如下关系
$$ \cos {\theta _{ij}} = w_i^T{w_j} = v_i^T{v_j} $$ (3) 2.1 传统星敏感器标定算法
将(1)式代入(5)式可得
$$ v_i^T{v_j} = \frac{{\left( {{x_i} - {x_0}} \right)\left( {{x_j} - {x_0}} \right) + \left( {{y_i} - {y_0}} \right)\left( {{y_j} - {y_0}} \right) + {f^2}}}{{\sqrt {{{\left( {{x_i} - {x_0}} \right)}^2} + {{\left( {{y_i} - {y_0}} \right)}^2} + {f^2}} \sqrt {{{\left( {{x_j} - {x_0}} \right)}^2} + {{\left( {{y_j} - {y_0}} \right)}^2} + {f^2}} }} = {F_{ij}}\left( {{x_0}, {y_0}, f} \right) $$ (4) 设S=[x0, y0, f]T的估值为$\widehat S = {\left[ {{{\widehat x}_0}, {{\widehat y}_0}, \widehat f} \right]^T} $估计值Ŝ与标称值S之间的误差为ΔS=[Δx0, Δy0, Δf]T。
将(6)式线性化并令
$$ \begin{array}{l} \;\;\;\;\;{R_{ij}}\;\;\;\;\;\;\; = \;\;\;\;\;\;v_i^T{v_j}\;\;\; - \;\;\;\;{F_{ij}}\;\;({\widehat x_0}, {\widehat y_0}, \widehat f) = \\ \left[ {\begin{array}{*{20}{c}} {\frac{{\partial {F_{ij}}}}{{\partial {x_0}}}}&{\frac{{\partial {F_{ij}}}}{{\partial {y_0}}}}&{\frac{{\partial {F_{ij}}}}{{\partial f}}} \end{array}} \right]\Delta S \end{array} $$ (5) 式中：i=1, …, n-1;j=i+1, …, n，其中i, j分别表示第i和第j颗星。则
$$ R = A\Delta S $$ (6) 式中：
$$ A = \left[ {\begin{array}{*{20}{c}} {\frac{{\partial {F_{12}}}}{{\partial {x_0}}}\;}&{\frac{{\partial {F_{12}}}}{{\partial {y_0}}}}&{\frac{{\partial {F_{12}}}}{{\partial {f_0}}}}\\ {\frac{{\partial {F_{13}}}}{{\partial {x_0}}}}&{\frac{{\partial {F_{13}}}}{{\partial {y_0}}}}&{\frac{{\partial {F_{13}}}}{{\partial {f_0}}}}\\ \vdots & \vdots & \vdots \\ {\frac{{\partial {F_{\left( {n - 1} \right)n}}}}{{\partial {x_0}}}}&{\frac{{\partial {F_{\left( {n - 1} \right)n}}}}{{\partial {y_0}}}}&{\frac{{\partial {F_{\left( {n - 1} \right)n}}}}{{\partial {f_0}}}} \end{array}} \right];R = \left[ {\begin{array}{*{20}{c}} {{R_{12}}}\\ {{R_{13}}}\\ \vdots \\ {{R_{\left( {n - 1} \right)n}}} \end{array}} \right] $$ (7) 根据最小二乘最优估计理论，公式(6)可转换为
$$ \Delta {S_k} = {\left[ {A_k^T{A_k}} \right]^{ - 1}}A_k^T{R_k} $$ (8) 式中：k为迭代次数。经k次迭代后，可得
$$ {\widehat S_{k + 1}} = {\widehat S_k} + \Delta {S_k} $$ (9) 2.2 星敏感器主点和焦距加权标定算法
由于待标定参数有3个，因此仅需要3个及以上的星点即可确定主点和焦距的值，而星敏感器视场中的恒星往往远超3个星点。可是如果在计算的过程中所使用的星光矢量对中含有位置偏差较大的星点，不仅不能减小误差，而且会使标定结果误差变大。仅使用最小二乘法对主点和焦距进行标定时，并不能找出不适合作为标定的坏点，因此就需要设计一种算法来减弱甚至消除这些误差较大的坏点的影响，从而提高标定精度。
为此我们将地心赤道惯性坐标系下的星光矢量夹角与每一步Fij估计值的偏差作为权值计算的条件，在计算R的过程中对(9)式进行加权处理，可得到带有权重的R的值
$$ 令\left\{ \begin{array}{l} \sigma _i^2 = \sum\limits_{j \ne i}^n {{{\left\| {v_i^T{v_j} - {F_{ij}}({{\widehat x}_0}, {{\widehat y}_0}, \widehat f)} \right\|}^2}} \\ w{o_i} = 1/\sigma _i^2\\ {w_i} = \frac{{\left( {n - 1} \right)w{o_i}}}{{\sum\limits_{i = 1}^n {w{o_i}} }} \end{array} \right. $$ (10) 即
$$ {w_i} = \frac{{n - 1}}{{\sum\limits_{j \ne i}^n {{{\left\| {v_i^T{v_j} - {F_{ij}}({{\widehat x}_0}, {{\widehat y}_0}, \widehat f)} \right\|}^2}} \cdot \sum\limits_{i = 1}^n {\frac{1}{{\sum\limits_{j \ne i}^n {{{\left\| {v_i^T{v_j} - {F_{ij}}({{\widehat x}_0}, {{\widehat y}_0}, \widehat f)} \right\|}^2}} }}} }} $$ (11) 式中：wi是每个星像点的权值；n表示视场内标定所使用的星点个数。
在使用最小二乘法加权计算ΔS的结果收敛后，引入一个评价变量Th作为剔除随机测量噪声偏大星点的条件，其计算公式如下所示
$$ \left\{ \begin{array}{l} Th = \frac{1}{{\sqrt m }}\sqrt {\sum\limits_{i = 1}^{n - 1} {\sum\limits_{j = i + 1}^n {{{\left\| {\arccos \left( {v_i^T{v_j}} \right) - \arccos \left( {{F_{ij}}({{\widehat x}_0}, {{\widehat y}_0}, \widehat f)} \right)} \right\|}^2}} } } \\ m = \frac{{n\left( {n - 1} \right)}}{2} \end{array} \right. $$ (12) 式中：m表示星光矢量夹角对数；n表示视场内标定所使用的星点个数。
我们可以将初始阈值设置为第一次计算所得Th值的1/10，当计算出Th的结果大于我们设置的阈值时，我们就将权重最小的星点剔除，利用剩余的星点继续计算，直到计算出Th的结果小于阈值时，我们可以认为剩余的星点的误差已在同一数量级，则停止剔除星点。
为滤除星点随机测量噪声的影响，提高标定精度，需要以最小二乘法加权计算出的结果作为输入，使用扩展卡尔曼滤波器(EKF)对其进行滤波，其计算步骤如下
$$ \left\{ \begin{array}{l} {K_{k + 1}} = {P_k}A_k^T{\left[ {{A_k}{P_k}{A_k} + {R_e}} \right]^{ - 1}}\\ {X_{k + 1}} = {X_k} + {K_{k + 1}}\left[ {{R_{k + 1}} - {A_{k + 1}}{X_k}} \right]\\ {R_{k + 1}} = {P_k} - {K_{k + 1}}{A_{k + 1}}{P_k} \end{array} \right. $$ (13) 式中：X为Δx0、Δy0、Δf经扩展卡尔曼滤波后的在轨标定的值；K为增益矩阵；Re为估计测量噪声方差矩阵；P为估计误差的协方差矩阵。
标定的结果的精度可使用星内角距的统计偏差作为评价指标[11]，其表达式如下
$$ \begin{array}{l} \;\;\;\Delta \alpha = \frac{1}{{\sqrt m }}\\ \sqrt {\sum\limits_{i = 1}^{n - 1} {\sum\limits_{j = i + 1}^n {\mathit{k}{{(\arccos \left( {{v_i}{v_j}} \right) - \arccos \left( {{w_i}{w_j}} \right))}^2}} } } \end{array} $$ (14) 3 仿真结果及分析
为模拟真实的在轨星图，使用SAO星表进行星图模拟仿真，星敏感器视场为10°×10°，CCD面阵为1 024像素×1 024像素，像元尺寸为15 μm，敏感星等为5 Mv，光学主点真实位置在(500, 520)像素处，焦距的真实值为87.782 8 mm。为模拟在轨星图，对星图中标定所使用的恒星位置上随机对其中的2个星点位置添加均值为0，方差为3的高斯噪声，而对其余星点位置添加均值为0，方差为0.1的高斯噪声。
![]()
图 3 未剔除坏点时非加权与加权算法标定结果对比Figure 3. Comparison of unweighted and eighted algorithms calibration results when no bad points are removed随机生成星敏感器光轴中心指向，仿真模拟1 000张星图，在不剔除随机测量噪声偏大星点的情况下，不使用加权方法和使用加权方法的标定结果对比如图 4所示。其中(x0, y0)和f估计的初始值分别是(512, 512)像素和87 mm。根据结果可以看出在不剔除随机测量噪声偏大的星点时所提出的加权标定方法的(x0, y0)和f的估计结果似乎比不加权的方法估计效果略好一些，但是它们的标定结果与真值之间还有相对较大的偏差，所以还需要对随机测量噪声偏大的星点剔除后重新进行标定。
![]()
图 4 使用加权算法时未剔除与剔除坏点标定结果对比Figure 4. Comparison of bad points removed and not when using weighted algorithm calibration同样使用加权方法，对比未剔除和剔除随机测量噪声偏大的星点后的标定结果如图 5所示。根据结果可以看出剔除随机测量噪声偏大的星点后(x0, y0)和f的估计结果明显比未剔除的效果好得多，而且标定结果收敛得更快，在大约输出200幅星图后标定参数即可达到稳定状态。
使用星内角距统计偏差来作为标定精度的评价指标，几种方法的结果如图 6所示。从图中可以看出，提出的加权剔除标定算法的误差明显小于其他2种方法。所提出的加权剔除算法星内角距统计偏差约为传统算法的1/10，极大地提高了标定精度。与真值比较，使用加权剔除标定算法时(x0, y0)和f的估计结果的精度分别可达到0.219 9像素、0.148 7像素、3.38 μm。
4 结论
针对标定过程中含有随机测量噪声偏大的星像点时，本文提出了一种星敏感器主点和焦距的加权的标定方法。该方法首先建立了星敏感器在轨标定模型，然后引入合理的标定权值，加入到最小二乘最优估计计算主点和焦距的过程中，寻找并剔除随机测量噪声较大的星点，最后将加权估计出的结果进行测量，采用扩展卡尔曼滤波对星图进行处理。仿真结果表明：输出大约200幅星图即可达到稳定状态，其对星敏感器主点和焦距的标定精度可分别达到0.219 9像素、0.148 7像素、3.38 μm。在使用星内角距统计偏差作为标定精度的评价指标时，本文提出方法的偏差仅为传统标定方法的1/10，在剔除随机测量噪声偏大星点的同时，极大提高了主点和焦距的标定精度。
-
![]()
图 3 未剔除坏点时非加权与加权算法标定结果对比
Figure 3. Comparison of unweighted and eighted algorithms calibration results when no bad points are removed
![]()
图 4 使用加权算法时未剔除与剔除坏点标定结果对比
Figure 4. Comparison of bad points removed and not when using weighted algorithm calibration
-
[1] 乔培玉, 何昕, 魏仲慧, 等. 高精度星敏感器的标定[J]. 红外与激光工程, 2012, 41(10):2779-2784. doi: 10.3969/j.issn.1007-2276.2012.10.042 QIAO Peiyu, HE Xin, WEI Zhonghui, et al. Calibration of high-accuracy star sensor[J]. Infrared Laser Engineering, 2012, 41(10):2779-2784. doi: 10.3969/j.issn.1007-2276.2012.10.042
[2] 张磊, 周宇, 林荣峰, 等. 一种快速三角形星图识别算法[J]. 应用光学, 2018, 39(1):71-75. http://d.old.wanfangdata.com.cn/Periodical/yygx201801012 ZHANG Lei, ZHOU Yu, LIN Rongfeng, et al. Fast triangle star pattern recognition algorithm[J]. Journal of Applied Optics, 2018, 39(1):71-75. http://d.old.wanfangdata.com.cn/Periodical/yygx201801012
[3] 郝雪涛, 张广军, 江洁. 星敏感器模型参数分析与校准方法研究[J].光电工程, 2005, 32(3):5-8. doi: 10.3969/j.issn.1003-501X.2005.03.002 HAO Xuetao, ZHANG Guangjun, JIANG Jie. Star sensor model parameter analysis and calibration method[J]. Opto-electronic Engineering, 2005, 32(3):5-8. doi: 10.3969/j.issn.1003-501X.2005.03.002
[4] 贺鹏举, 梁斌, 张涛, 等. 大视场星敏感器标定技术研究[J]. 光学学报, 2011, 31(10):192-198. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=gxxb201110035 HE Pengju, LIANG Bin, ZHANG Tao, et al. Calibration method for wide field of view star sensor[J]. Acta Optica Sinica, 2011, 31(10):192-198. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=gxxb201110035
[5] Xie J, Tang H, Dou X, et al. On -orbit calibration of domestic APS star tracker[C]//Changsha, China: Earth Observation and Remote Sensing Applications, 2014 3rd International Workshop on, 2014: 239-242.
[6] SAMAAN M A, GRIFFITH T, SINGLA P, et al. Autonomous on-orbit calibration of star trackers[C].Colorado Springs:Core Technologies for Space Systems Conference (Communication and Navigation Session), 2001.
[7] 钟红军, 杨孟飞, 卢欣. 星敏感器标定方法研究[J]. 光学学报, 2010, 30(5):1343-1348. http://d.old.wanfangdata.com.cn/Periodical/yqyb201709008 ZHONG Hongjun, YANG Mengfei, LU Xin. Calibration method of star sensor[J]. Acta Optica Sinica, 2010, 30(5):1343-1348. http://d.old.wanfangdata.com.cn/Periodical/yqyb201709008
[8] LIU H, JIA H. Autonomous on-orbit calibration of a star tracker camera[J]. Optical Engineering, 2011, 50(2):68-68. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=3028dfb73872e093abcd25fc1cec53e2
[9] MEDAGLIA E. Autonomous on-orbit calibration of a star tracker[C]// Florence, Italy:Metrology for Aerospace. IEEE, 2016. https://ieeexplore.ieee.org/document/7573258
[10] YAN K, LIU E, TIAN H, et al. Calibration of camera intrinsic parameters using a single image[C].Shanghai, China:International Conference, 2017:39-45.
[11] 张辉, 田宏, 袁家虎, 等. 星敏感器参数标定及误差补偿[J]. 光电工程, 2005, 32(9):1-4. doi: 10.3969/j.issn.1003-501X.2005.09.001 ZHANG Hui, TIAN Hong, YUAN Jiahu, et al. Parameter calibration and error compensation of star sensor[J]. Opto-electronic Engineering, 2005, 32(9):1-4. doi: 10.3969/j.issn.1003-501X.2005.09.001
-
期刊类型引用(3)
1. 闫浩东，支帅，陈旭睿，李照雄，丁国鹏，张洋洋，张永合，朱振才. 基于模型和样条的星敏感器在轨自标定方法. 光学学报. 2024(12): 262-270 . 
百度学术
2. 张宇轩，王宏力，何贻洋，肖永强，张鹏飞，冯磊. 光学畸变耦合下的星敏感器焦距误差建模与分析. 电光与控制. 2022(02): 63-66 . 百度学术
3. 张新盈. 星敏感器在海上遥感测绘中的计量方法. 舰船科学技术. 2019(20): 175-177 . 百度学术
其他类型引用(4)
下载:
计量
- 文章访问数: 680
- HTML全文浏览量: 251
- PDF下载量: 75
- 被引次数: 7
陕公网安备 61011302001501号 