Design and tolerance analysis of infrared off-axis three-mirror optical system with large aperture
-
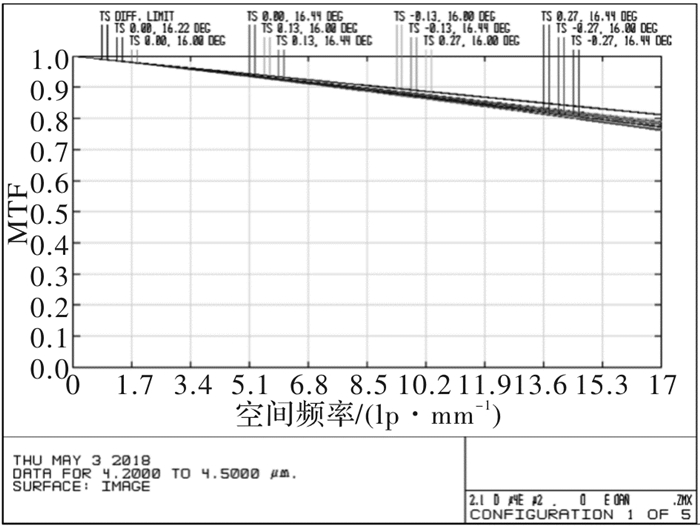
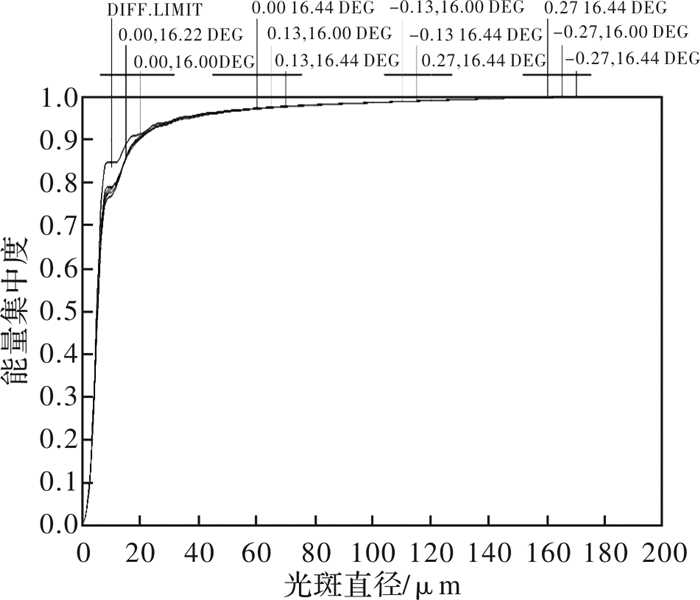
摘要: 在空间光学领域中,光学系统的发展趋势为长焦距、大视场、轻量化、大相对孔径、高成像质量等。为适应该发展趋势,对大口径反射式光学系统进行研究,在共轴三反系统的成像理论基础上,为避免中心遮拦,提高成像质量,采用视场离轴方式,设计了一款大口径离轴三反式光学系统。该光学系统在奈奎斯特空间频率17 lp/mm处,光学传递函数MTF大于0.75,成像质量接近衍射极限。此外,光学系统公差的合理分配是影响相机总体性能的主要因素,运用公差灵敏度分析和反转灵敏度分析,计算各公差对光学系统成像质量的影响,给出了合适的公差分配,经过模拟分析,按照给定的公差加工装调,系统光学传递函数大于0.55。Abstract: In the field of space optics, the development trend of optical systems is long focal length, large field of view, light weight, large relative aperture, high imaging quality, and so on. In order to adapt to this development trend, the large-caliber reflective optical system is studied.An off-axis three-mirror optical system with large aperture was designed based on the imaging theory of coaxial three-mirror optical system and the way of field off-axis was used to avoid central shielding problem and improve the image quality. The modulation transform function (MTF) of the optical system is more than 0.75 at the Nyquist frequency of 17 lp/mm and the image quality is close to the diffraction limits. In addition, since the tolerance reasonable distribution of optical system is the main factor that affects the performance of camera, the sensitivity analysis and the inverse sensitivity analysis were used to compute the effect of each tolerance parameter on the optical system image quality and the proper tolerance distribution was determined. Through simulation analysis, the optical system can has high image quality with fabricated and assembled on the basis of tolerance given, and the MTF can be more than 0.55.
-
引言
某型号星载光谱仪聚焦于陆地生态系统植被和森林蓄积量探测,利用670 nm~780 nm谱段的光谱获取空间连续分布的植被荧光信号,得到太阳诱导植被荧光遥感数据,从而准确绘制植被荧光时空分布规律,满足全球植被定量监测、森林植被生产力评估的需求。光谱仪采用光栅作为色散元件,由于分光系统的存在,系统会残余较大畸变,导致数据处理困难,星载光谱仪为了校正系统的畸变,成像透镜设计为离轴透射式光学系统,系统存在3个光轴,光轴之间倾斜量达到0.606°,偏心量达到0.279 mm,增加了装调的难度。
光学系统的质量和光学元件的装调精度息息相关,国内外调研结果表明,透射式系统装调方法多为双光路定心法,定心仪测试透镜表面球心的跳动量给出球心位置,球心连线确定光轴,装调时最小化不同透镜光轴的偏心和倾斜,达到系统设计指标[1-6]。双光路定心法只适用于同轴透射式系统,对于离轴透射式系统的装调方法未见相关报道。光谱仪成像系统最大倾斜量超出了定心仪的装调范围,无法应用双光路定心法。针对这个难题提出了一种多基准轴的定心装调方法(MAA),通过结构预置偏心和倾斜的方法构建基准轴,利用光学平板实现光轴引出,将复杂的多光轴系统装调分解成单光轴系统装调,实现了离轴透射式光学系统的高精度装调。实测结果验证了该方法的有效性,为离轴透射式系统装调开拓了新的思路。
1 装调原理
1.1 成像透镜系统参数
光谱仪采用光栅和棱镜作为色散元件,成像组件采用离轴透射式系统,补偿由光栅和棱镜引起的畸变,可实现系统的畸变最小化。成像组件包含3个光轴,光轴之间存在偏心和倾斜,如图1所示。透镜1和透镜2组成光轴1,透镜3和透镜4组成光轴2,透镜5为光轴3。光轴1和光轴2倾角为0.262°,偏心量为0.279 mm,光轴3相对于光轴1倾角为0.606°,参数如表1所示。
表 1 透镜的偏心和倾斜参数Table 1. Parameters of lens eccentricity and tilt透镜 偏心X 偏心Y/mm 倾斜X 倾斜Y/(°) 透镜1 0 0 0 0 透镜2 0 0 0 0 透镜3 0 −0.279 0 −0.262 透镜4 0 −0.279 0 −0.262 透镜5 0 0 0 −0.606 1.2 装调分析
畸变是实际像高和理想像高的偏差,根据δ初级像差理论,像差分为5种初级像差,以5个塞得和数表示。畸变是主光线的垂轴像差,表达式为
$$\delta Y' = - \dfrac{1}{{2n'u'}} \displaystyle\sum \limits_{i = 1}^k {S_{\rm{V}}}$$ (1) 根据塞得和数表达式:
$$ \displaystyle\sum \limits_{i = 1}^k {S_{\rm{I}}} = \displaystyle\sum \limits_{i = 1}^k luni\left( {i - i'} \right)\left( {i' - u} \right)$$ (2) $$ \displaystyle\sum \limits_{i = 1}^k {S_{{\rm{III}}}} = \displaystyle\sum \limits_{i = 1}^k {S_{{\rm{II}}}}\dfrac{{{i_{\textit{z}}}}}{i} = {S_{\rm{I}}}\dfrac{{{i_{\textit{z}}}^2}}{{{i^2}}}$$ (3) $$ \displaystyle\sum \limits_{i = 1}^k {S_{{\rm{IV}}}} = \displaystyle\sum \limits_{i = 1}^k {J^2}\dfrac{{n' - n}}{{n'nr}}$$ (4) $$ \displaystyle\sum \limits_{i=1}^{k}{S}_{\rm{V}}= \displaystyle\sum\limits _{i=1}^{k}({S}_{\rm{III}}+{S}_{\rm{IV}})\dfrac{{i}_{{\textit{z}}}}{i} $$ (5) 推导得出光学系统的畸变表达式:
$$ \begin{split} \delta {Y^\prime } = & - \dfrac{1}{{2n'u'}}\displaystyle\sum\limits_{i = 1}^k {\Bigg(luni\left( {i - {i^\prime }} \right)\left( {{i^\prime } - u} \right)\dfrac{{{i_{\textit{z}}}^2}}{{{i^2}}} + } \\ & nu{y^2}\dfrac{{{n^\prime } - n}}{{n'nr}}\Bigg)\dfrac{{{i_{\textit{z}}}}}{i} \end{split}$$ (6) 简化后的畸变表达式为
$$ \begin{split} \delta {Y^\prime } = & - \dfrac{1}{{2n'u'}} \displaystyle\sum \limits_{i = 1}^k \Bigg(\dfrac{{n\left( {{n^\prime } - n} \right)\left( {n - nr - {n^\prime }r} \right)lu}}{{{n^\prime }^2(l - r)}}{i_{\textit{z}}}^3+\\ & + \dfrac{{({n^\prime } - n{\rm{)}}u{y^2}}}{{l - r}}{i_{\textit{z}}}\Bigg) \end{split}$$ (7) 式中:n和n′为折射率;k为光学元件的数量;r为曲率半径;u为数值孔径;y为像高;
${i_{\textit{z}} }$ 为主光线和光轴的夹角。由公式(7)可知畸变与主光线和光轴的夹角以及像高正相关,本系统中数值孔径和像高无法改变,为了纠正畸变,透镜系统设计为离轴透射式系统,改变主光线和光轴的夹角。装调过程中精确控制离轴透镜组的倾角可有效控制畸变。将实测的透镜曲率半径和中心厚度输入到光学系统优化设计软件中[7-8],重新对系统镜间距和偏心进行优化,以光轴1为基准,根据畸变计算光轴2和光轴3的失调量敏感度,如图2所示。结果表明,当畸变小于3 μm时,透镜偏心公差需小于0.05 mm,倾斜公差小于60″,镜间距公差小于0.05 mm,根据以往研究结果,直接装配无法满足系统要求[9-10]。
1.3 MAA装调方法
系统装调过程为最小化偏心以及倾斜量误差最小的过程,透镜组光轴偏差达到0.606°,超出了定心仪的测试范围,需要在系统中引入新的装调基准。考虑到装调公差相对宽松,提出了在透镜筒结构上一体化加工基准轴,预置光轴的倾斜量和偏差量,光学平板引出结构基准轴,应用OptiCentric双光路定心仪在新的基准轴上装调,将多光轴系统的装调分解成单光轴子系统装调,系统的装调流程如图3所示。
2 装调过程
2.1 机床定心
机床定心可去除光学件加工过程中产生的偏心和倾斜[11],将透镜框的安装端面和透镜框的外圆加工成和光轴一致,如图4所示。固定透镜组件到机床上,千分表测量透镜框外圈的圆跳动,调整使其和机床同轴,通过光学定心方法调整透镜的偏心和倾斜,使其光轴和机床转轴重合,加工透镜框的外圆和端面与透镜光轴重合。
2.2 同轴系统装调
系统包含2个同轴透镜组,单透镜机床定心完成后,选用OptiCentric双光路定心仪进行定心装调[12]。调整透镜1的光轴与定心仪的旋转轴一致,安装透镜2,通过垫片和侧面顶丝调整透镜2的光轴与定心仪的旋转轴一致,如图5和图6所示。同理装调透镜3和透镜4组件。
2.3 光轴引出
系统包含3个光轴,最大偏差0.606°,镜筒一体化加工预置偏心和倾斜,构建3个基准轴,建立装调基准。如图7所示,基准轴1和镜筒外径同轴,基准轴2相对于基准轴1的夹角为0.262°,偏心量为0.279 mm,基准轴3相对于基准轴1的夹角为0.606°。
基准轴的加工精度直接影响系统的装调精度,基准轴偏差计算公式为
$$ {\rm{\alpha }} = \dfrac{{{h_1} - {h_2}}}{D} $$ (8) 式中:
$ \alpha$ 为基准轴倾角;$ {h_1} $ 和$ {h_2} $ 为基准轴两侧的高度;D为基准内径。对公式(8)求导可得:$$ {\rm{\delta \alpha }} = \dfrac{1}{D}(\delta {h_1} - \delta {h_2}) - \dfrac{{{h_1} - {h_2}}}{{{D^2}}}\delta D $$ (9) 其中内径D为100 mm,对应0.606°的倾角,基准轴两侧的高度差为1.06 mm,公式(9)第2项相对于第1项可以忽略。提高基准轴精度的关键是控制
$ {h_1}$ 和$ {h_2}$ 的加工精度,加工过程中重点保证基准面的高度差,加工完成后,高精度三坐标测量机测试表明,偏心公差优于0.02 mm,倾斜公差优于30″,能够满足基准轴引出精度要求。2.4 系统装调
装调过程如图8和图9所示。将镜筒固定在双光路定心仪的转台上,采用光学平板将光轴1引出,结合杠杆千分表调整光轴和定心仪同轴,倾斜量小于10″,圆跳动量小于0.015 mm,作为光轴1的基准轴。安装透镜1和透镜2组件,调整到倾斜偏差小于10″,偏心误差小于0.015 mm。将镜筒翻转180°,通过光学平板和杠杆千分表引出光轴2,作为透镜3和透镜4组件的安装基准。安装透镜3和透镜4组件时,调整倾斜偏差小于10″,偏心小于0.015 mm。光学平板引出光轴3,安装透镜5组件,调整到倾斜偏差小于10″,偏心误差小于0.015 mm,完成系统偏心和倾斜的装调。
装调误差分布如图10所示。从图10可知,基准轴的引出误差为主要因素,通过进一步提高镜筒的加工精度可提高系统的装调精度。
1) 镜筒同轴度误差。镜筒的同轴度为15 μm,千分尺的测量误差为1 μm,引起的镜头偏心误差为8 μm。
2) 镜筒基准轴偏心、倾斜误差。三坐标测量结果表明,镜筒偏心误差优于20 μm,倾斜误差优于30″。
3) 机床主轴回转误差。机床主轴引起的倾斜误差为2″,偏心误差为3 μm。
4) 定心仪测量误差。双光路定心仪倾斜测量误差为1″,偏心测量误差为1 μm。
5) 同轴透镜调整误差。透镜1和透镜2组件,透镜3和透镜4组件为同轴系统,调整误差较小,偏心可控制在10 μm,倾斜控制在10″。
6) 离轴透镜调整误差。透镜倾斜调整误差为10″,偏心调整误差为15 μm。
7) 光学平板接触误差。光学平板平面度优于10 nm,光学平板和结构面接触误差优于10 μm,镜筒的内径为100 mm,引起镜头倾斜偏差为20.6″。
综合以上误差,可计算得出透镜组的倾斜误差优于38.8″,偏心误差优于28.3 μm,满足系统的指标要求。
2.5 镜间距控制
同轴透射式系统的镜间距通过测试球面顶点得到[13],球面顶点同时也是透镜的最高点或者最低点,对于离轴系统,透镜表面的最高点和最低点不再位于球面顶点,偏差量和倾斜偏心量相关,如图11所示。球面顶点A和表面最高点A′之间距离X如下式所示:
$$X = R - R \times {\rm{cos}}a$$ (10) 式中:a为透镜的倾角;R为球面曲率半径。
根据公式(10)计算得出透镜最高点距离和透镜间距的差值如表2所示。
表 2 镜间距和测试距离对应表Table 2. Corresponding lens distance and test distanceItem Lens1 & Lens2 Lens2 & Lens3 Lens3 & Lens4 Lens4 & Lens5 Distance between vertex of lens/mm 1.800 50.765 25.248 6.928 Distance between lens/mm 1.800 50.763 25.248 6.923 Difference/mm 0 0.002 0 0.005 在多基准轴装调过程中,通过接触式方法测量镜间距[14-15],如图12所示。首先测量透镜1和透镜2之间的镜间距以及透镜3和透镜4之间的镜间距,将透镜2的光轴调整到和双光路定心仪的转台同轴,通过探针分别测量透镜1和透镜2上表面的顶点高度d1和d2。透镜1和透镜2的镜间距计算方法如下式:
$${D_{12}} = {d_2} - {d_1} - {D_2}$$ (11) 式中:D2为透镜2的中心厚度;D3为透镜3的中心厚度。同理测量透镜3和透镜4镜间距D34。
透镜1和透镜2组件安装到镜筒中,测量透镜2的上表面最高点H2,安装透镜3和透镜4组件,测量透镜4上表面最高点H4,透镜2和透镜3的镜间距D23如下式:
$${D_{23}} = {H_4} - {H_2} - {D_{34}} - {D_3} - {D_4}$$ (12) 同理测量透镜4和透镜5镜间距D45。系统装调后实物图如图13所示。
镜间距测量误差如表3所示。探针2次测量得出镜间距,系统装调倾斜误差优于38.8″,偏心误差优于28.3 μm,引起的镜间距测量误差≤1 μm,综合两种测量误差,镜间距测量误差≤14.2 μm,满足系统指标要求。
表 3 镜间距测量误差Table 3. Measurement error of lens distance误差源 探针单点测量
误差/μm偏心倾斜引起的
误差/μm镜间距
误差/μm数值 10 1 14.2 3 装调结果
系统装调完成后,分别测试光轴1,光轴2和光轴3,因为光轴1和光轴3的夹角为0.606°,超出了定心仪的测量范围,采用分段测试的方式,分别测试光轴1和光轴2的偏差,光轴2和光轴3的偏差,测试结果如表4和表5所示,与设计结果的偏差如表6所示。最大偏心误差为25.4 μm,最大倾斜误差为17.7″,实际畸变和理论畸变对比如图14所示。从图14可看出,最大畸变为2.77 μm,平均偏差0.32 μm,系统装调结果满足指标要求。
表 4 透镜1和透镜2组件以及透镜3和透镜4组件的偏心倾斜Table 4. Eccentricity and tilt of lens 1, 2 and lens 3, 4Parameters Decenter X/μm Decenter Y/μm Tilt X/(″) Tilt Y/(″) Axis1 0 0 0 0 Axis2 −10.4 −297.5 −3.3 −960.9 表 5 透镜3和透镜4组件以及透镜5组件的偏心倾斜Table 5. Eccentricity and tilt of lens 3, 4 and lens 5Decenter X/μm Decenter Y/μm Tilt X/(″) Tilt Y/(″) Axis2 0 0 0 0 Axis3 −9.1 −253.5 −17.6 −1232.1 表 6 透镜组的偏心倾斜偏差Table 6. Eccentricity and tilt deviation of lensDifference Decenter X/μm Decenter Y/μm Tilt X/(″) Tilt Y/(″) Axis1 0 0 0 0 Axis2 −10.4 −18.5 −3.3 −17.7 Axis3 1.3 25.4 −14.3 6.3 4 结论
本文提出了一种新的离轴透射式系统的装调方法——多基准轴定心装调方法(MAA),克服传统的同轴透射系统定心装调缺陷,通过结构预置偏心和倾斜的方法构建基准轴,并辅助光学平板将复杂的多光轴系统装调分解成单光轴系统装调,利用此方法装调了光谱仪多光轴成像系统。装调结果表明,透镜偏心误差小于25.4 μm,倾斜误差小于17.7″,实现了系统的高精度装调。本装调方法不受定心仪测量范围的限制,可针对任意光轴的透射系统进行装调,突破了传统透射式装调方法的边界,为离轴透射式系统装调开拓了新的思路。
-
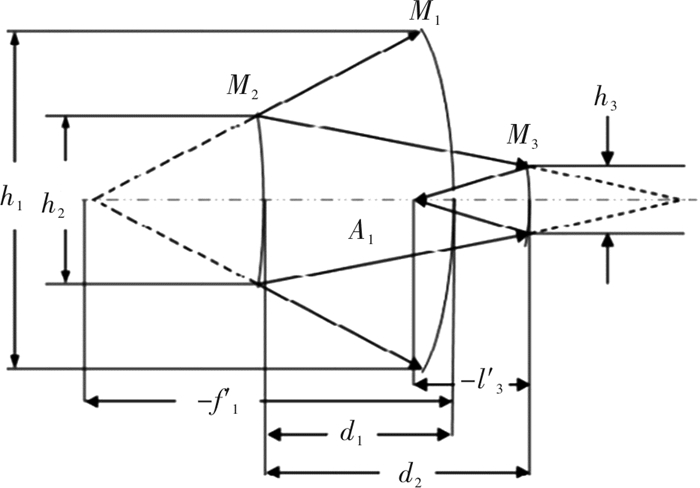
表 1 系统离轴优化后结构参数
Table 1 Structure parameters after off-axial optimization of system
名称 半径/mm 厚度/mm 非球面系数 2次项 4次项 PM 1918.400 1035.400 -0.644 -1.866E-004 -9.072E-012 SM 1799.100 -1045.100 4 TM 1676.000 1198.100 0.388 1.252E-005 表 2 几种材料的性能比较(25℃)
Table 2 Performance comparisons of several commonly used materials(25℃)
材料 弹性模量/GPa 泊松比/v 热膨胀系数/10-6·K-1 密度/g·m-3 热传导率/W·m-1·K-1 比热/J·kg-1·K-1 最优值 大 小 小 小 大 大 铝 68~75 0.34 22~44 2.7~2.8 165~220 0.9~1.0 铍 280~305 0.027 11~11.5 1.85 190~220 1.8~2.0 SiC 445~470 0.16 2.2~2.7 2.33~3.21 100~330 0.64~0.73 石英 72 0.17 0.51 2.2 1.38 0.772 ULE 66 0.17 0.03±0.06 2.2 1.31 0.770 ZERODUR 91 0.24 -0.03±0.05 2.53 1.64 0.821 表 3 光学系统公差分配
Table 3 Tolerance distribution of optical system
面形/λ(λ=632.8 nm) 曲率半径/mm CONIC TILT X/(″) TILT Y/(″) DEC X/mm DEC Y/mm 间距/mm PM 1/20 ±0.2 ±0.001 30 30 ±0.1 ±0.1 ±0.1 SM 1/20 ±0.2 ±0.005 30 30 ±0.1 ±0.1 ±0.1 TM 1/20 ±0.1 ±0.000 5 30 30 ±0.1 ±0.1 —— 表 4 蒙特卡罗分析结果
Table 4 Monte Carlo analysis results
百分比/% MTF值 90 >0.574 80 >0.627 50 >0.680 20 >0.713 10 >0.725 -
[1] 郭永祥, 李永强, 廖志波, 等.新型离轴三反射光学系统设计[J].红外与激光工程, 2014, 43(2):547-550. http://d.old.wanfangdata.com.cn/Periodical/hwyjggc201402039 GUO Yongxiang, LI Yongqiang, LIAO Zhibo, et al. Novel design of off-axis three reflective optical system[J]. Infrared and Laser Engineering, 2014, 43(2):547-550. http://d.old.wanfangdata.com.cn/Periodical/hwyjggc201402039
[2] 张文颖, 张国玉, 张雷.大口径离轴反射式星模拟器光学系统设计[J].应用光学, 2014, 35(6):950-954. http://d.old.wanfangdata.com.cn/Periodical/yygx201406008 ZHANG Wenying, ZHANG Guoyu, ZHANG Lei. Optical system design of large-diameter off-axis reflection-type star simulation[J]. Journal of Applied Optics, 2014, 35(6):950-954. http://d.old.wanfangdata.com.cn/Periodical/yygx201406008
[3] 赵文才.改进的离轴三反光学系统的设计[J].光学·精密工程, 2011, 19(12):2837-2843. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201112004 ZHAO Wencai. Design of improved off-axial TMA optical systems[J].Optical Precision Engineering, 2011, 19(12):2837-2843. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201112004
[4] 潘君骅.光学非球面的设计、加工与检验[M].苏州:苏州大学出版社, 2004: 130-138. PAN Junhua.The design, manufacture and test of the aspherical optical surfaces[M]. Suzhou: Suzhou University Press, 2004:130-138.
[5] 李岩, 张葆, 洪永丰.灵巧型离轴三反光学系统设计[J].应用光学, 2014, 35(3): 391-394. http://d.old.wanfangdata.com.cn/Periodical/yygx201403008 LI Yan, ZHANG Bao, HONG Yongfeng. Compact and multispectral off-axial there-mirror reflective optical system[J]. Journal of Applied Optics, 2014, 35(3): 391-394. http://d.old.wanfangdata.com.cn/Periodical/yygx201403008
[6] 孟庆宇, 汪洪源, 王严, 等.大线视场自由曲面离轴三反光学系统设计[J].红外与激光工程, 2016, 45(10):1018002-1-1018002-8. http://d.old.wanfangdata.com.cn/Periodical/hwyjggc201610024 MENG Qingyu, WANG Hongyuan, WANG Yan, et al. Off-axis three-mirror freeform optical system with large linear field of view[J]. Infrared and Laser Engineering, 2016, 45(10):1018002-1-1018002-8. http://d.old.wanfangdata.com.cn/Periodical/hwyjggc201610024
[7] 孟庆宇,王维,纪振华,等. 主三镜一体化离轴三反光学系统设计[J]. 红外与激光工程,2015, 44(2):578-582. doi: 10.3969/j.issn.1007-2276.2015.02.033 MENG Qingyu, WANG Wei, JI Zhenhua, et al. Design of off-axis three-mirror system based on integration of primary and tertiary mirrors[J]. Infrared and Laser Engineering, 2015, 44(2):578-582. doi: 10.3969/j.issn.1007-2276.2015.02.033
[8] 杨乐, 孙强, 郭邦辉.离轴三反红外双波段景象模拟器光学系统设计[J].应用光学, 2011, 32(6): 1212-1216. http://d.old.wanfangdata.com.cn/Periodical/yygx201106031 YANG Le, SUN Qiang, GUO Banghui. Optical design of off-axis there-mirror system for infrared dual-band scene simulation[J]. Journal of Applied Optics, 2011, 32(6): 1212-1216. http://d.old.wanfangdata.com.cn/Periodical/yygx201106031
[9] 宋岩峰, 邵晓鹏, 徐军.离轴三反射镜光学系统研究[J].红外与激光工程, 2008, 37(4): 706-709. doi: 10.3969/j.issn.1007-2276.2008.04.034 SONG Yanfeng, SHAO Xiaopeng, XU Jun. Off-axis three-mirror reflective optical system[J]. Infrared and Laser Engineering, 2008, 37(4): 706-709. doi: 10.3969/j.issn.1007-2276.2008.04.034
[10] 梁宝雯, 吴晗平, 王华泽.空间相机离轴三反红外光学系统设计[J].红外技术, 2013, 35(4): 217-222. http://d.old.wanfangdata.com.cn/Periodical/hwjs201304008 LIANG Baowen, WU Hanping, WANG Huaze. Design of off-axial TMA infrared optical system for space camera[J]. Infrared Technology, 2013, 35(4):217-222. http://d.old.wanfangdata.com.cn/Periodical/hwjs201304008
[11] 王红, 田铁印.三线阵测绘相机光学系统的设计和公差分析[J].光学·精密工程, 2011, 19(7):1444-1449. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201107002 WANG Hong, TIAN Tieyin. Design of three line array mapping camera and its tolerance analysis[J]. Optics and Precision Engineering, 2011, 19(7):1444-1449. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201107002
[12] 栗孟娟, 廖志波, 王春雨.小口径高精度折射式光学系统装调公差的分析与控制[J].应用光学, 2015, 36(2):277-281. http://d.old.wanfangdata.com.cn/Periodical/yygx201502019 LI Mengjuan, LIAO Zhibo, WANG Chunyu. Analysis and control on assemblage tolerance in small-aperture high-precision refractive optical system[J]. Journal of Applied Optics, 2015, 36(2):277-281. http://d.old.wanfangdata.com.cn/Periodical/yygx201502019
-
期刊类型引用(2)
1. 杜国军,张玉贵,崔博伦,江澄,欧宗耀. 碳卫星超光谱探测仪地面光谱定标. 光谱学与光谱分析. 2023(05): 1556-1562 .  百度学术
百度学术
2. 严冬,国成立,刘泉,黎发志,余晨阳,马洋,张宇龙,闫力松. 大数值孔径宽光谱折反式物镜设计(特邀). 红外与激光工程. 2023(09): 66-72 .  百度学术
百度学术
其他类型引用(3)




 下载:
下载:


































 陕公网安备 61011302001501号
陕公网安备 61011302001501号 