Centering method for non-diffracting spot images based on correlation-coefficient
-
摘要: 为实现无衍射光斑作为直线基准在复杂噪声背景下精确定中, 提出了一种基于相关因子的无衍射光斑图像定中算法。该算法先根据光强重心理论计算光斑中心的大致位置, 再将光斑图像转换成极坐标系下的灰度图, 并生成角频率与光斑图像空间频率相同的离散周期正弦信号, 求解其相位角并对各径向上的相位信息作均方差评价, 计算出极坐标系下理想光斑中心与实际光斑中心的相关因子, 从而达到定中无衍射光斑中心的目的。在模拟噪声环境下, 对比该算法与其他常规算法, 其结果表明:该算法抗背景噪声干扰能力强、计算耗时短, 具有稳定的亚像素定中精度。Abstract: In order to realize fast and precise centering with non-diffracting spot as straight-line datum under complex background noises, a centering method for non-diffracting images based on correlation-coefficient was proposed. In this algorithm, firstly the approximate position of the beam spot center is calculated based on the theory of light intensity gravity; then the image is converted to the grayscale image in polar coordinates, which generates the discrete-cycle sinusoidal signal with the same angular frequency as the spatial frequency of the spot image, and the phase angle is calculated and the average variance is evaluated for the phase information of each diameter; finally the correlation-coefficient is established in polar coordinate system for ideal beam center and actual beam center, which realizes centering the non-diffracting spot image. In the simulated noise environment, result shows that the proposed algorithm has strong ability of anti-noise interference, less time-consuming in comparison with other common algorithms, which realizes sub-pixel level accuracy.
-
Keywords:
- spot centering /
- ring filtering /
- non-diffracting spot /
- sub-pixel /
- image processing
-
引言
光束在工程中有许多不同的方式作为直线基准(如高斯光束)。基于非相干光束的常规仪表, 如经纬仪、激光水平仪等都是利用在图像空间中分划板的轨迹作为线性基准, 但聚焦误差难以消除。一般的激光自准直仪采用准直和扩展激光能量中心的轨迹作为基准, 其精度受光束漂移、激光器热变形引起的模式转变、非线性传播轨迹、光电测量误差等影响。目前, 广泛使用的双频激光干涉仪是通过两束互成角度且相互干扰的激光的空间位置作为基准。该方法灵敏度高、精密度好, 但仅用于测量一维, 它在测量过程中不能中断, 且激光器[1-2]需要较高的稳定性。而衍射法(如区域和位相板)可以避免非对称分布, 但仍存在许多缺陷, 如衍射十字的成像位置不连续、聚焦过程中会发生聚焦误差、波前误差引起衍射十字变形和模糊、空气扰动等[3-4]缺陷, 因此定位精度受此影响。
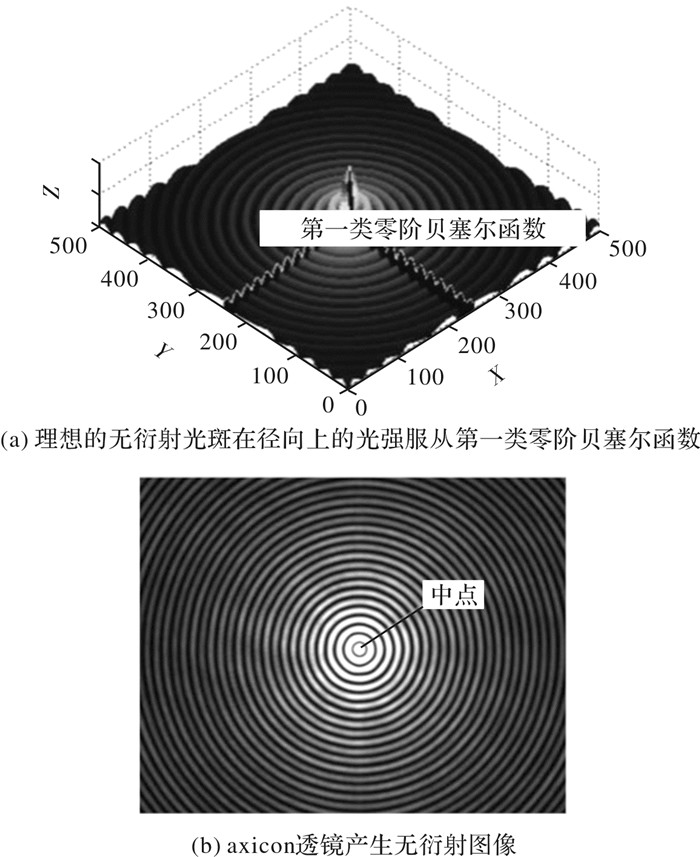
Durnin于1987年首次提出无衍射光束, 它是自由空间标量波动方程的一组特殊解, 其场分布具有第一类零阶贝塞尔函数J0的形式。近似的无衍射光束[5-6]可以通过Axicion透镜生成, 当它在自由空间中传播, 其强度和光斑尺寸保持不变。在有限范围内, 由于激光入射角近似地等于无衍射光的传播角度[7-8], 所以入射激光平行于无衍射光束轨迹中心。由于无衍射光没有聚焦和像差特征, 且在传播过程中中心光斑尺寸保持不变, 因此在直线度和姿态角[9-10]的测量中可以把无衍射光斑作为新的参考方法。数据的精度取决于无衍射光斑中心的拟合精度, 因此测量精度的关键在于精确定位其圆形条纹的中心。
目前, 常用的几种定心方法有如下几种:第1种是重心法, 它在无衍射光斑的最强强度区域(图 2(a)零阶区)使用重心法, 理论上光斑在此区域强度分布最强, 由于存在波面[11-12]像差, 衍射光斑的中心位置将会发生改变, 在较大波面像差下其形状难以估计, 因此最强强度区域的位置范围是不确定的; 另一方面, 中心位置的最高精度取决于检测单元的尺度(目前, 图像检测器的检测单元的大小大约是5 μm, 还达不到精密测量的要求)。第2种是Hough变换或圆拟合法[13-14]。传统Hough变换法有较高的鲁棒性, 但是其算法存在计算量大、计算成本高、消耗存储空间和数据可能丢失等问题。相反圆拟合法有较快的计算速度, 但只能用于检测单个圆, 且不稳定。这两种方法都是在图像二值化后使用, 因此其精度、可靠性和鲁棒性都取决于提取的图像边缘质量。第3种方法是数字莫尔条纹扫描法[15-16], 该方法是环形数字光栅叠加在无衍射图像上, 通过改变数字光栅的相位, 使用莫尔条纹扫描计算出无衍射光斑的中心。该方法实现了对白噪声的亚像素定心精度, 有较高的鲁棒性, 但是由于入射角度的倾斜、光源到轴棱锥[17-18]入射表面的距离及相位锥的计算量大等原因, 它不具备识别圆形和非圆形条纹的能力。
在本研究中, 提出了一种基于相关因子的无衍射图像的定中算法, 它沿径向方向计算同心灰度条纹图像的中心位置, 类似于相干匹配。由于其中心取决于所有的圆形条纹的共同中心, 因此可以达到较高的亚像素分辨率, 并能显著提高入射角测量的精度。
1 定中原理
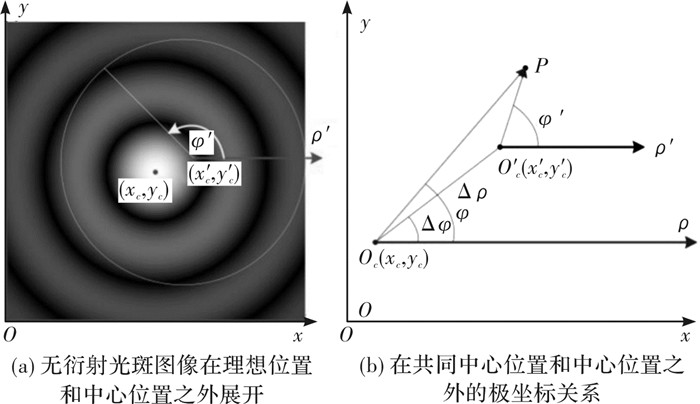
理想的无衍射光束在沿光轴传播方向上其光强分布服从第一类零阶贝塞尔函数, 其截面光斑为多级同心圆环分布, 如图 1所示。如果无衍射光斑的理论中心点为[xc, yc], 则图像f(ρ, φ)捕获的无衍射光束可以表示为
$$ f(\rho ,\varphi ) = {\rm{A}} \cdot {J_0}(\alpha \rho ) $$ (1) 其中:A和α是常量, 由无衍射光斑的生成方法决定; ρ是极半径到无衍射灰度中心点坐标[xc, yc]的距离; φ是极角, 如图 2(a)所示。
根据无衍射光斑的圆环中心进行滤波, 得到:
$$ \bar f(\rho ,\varphi ) = \frac{{\int_0^{2\pi } {f(\rho ,\varphi )} \rho {\rm{d}}\varphi }}{{2\pi \rho }} $$ (2) 若无衍射光斑的理论中心点坐标为[xc, yc], 则无衍射光斑的图像极坐标形式f (ρ, φf)表达为
$$ \bar f(\rho ,\varphi ) = f(\rho ,\varphi ) $$ (3) 从f(ρ, φf)和f(ρ, φf)相关运算中, 我们得到相关因子Q(xc, yc):
$$ \begin{array}{*{20}{l}} {Q({x_c},{y_c}) = }\\ {\qquad \frac{{\int_0^{2\pi } {\int_{{r_1}}^{{r_2}} {f(\rho ,\varphi )} } \cdot \bar f(\rho ,\varphi ){\rm{d}}\rho {\rm{d}}\varphi }}{{{{[\int_0^{2\pi } {\int_{{r_1}}^{{r_2}} {{f^2}} } (\rho ,\varphi ){\rm{d}}\rho {\rm{d}}\varphi \cdot \int_0^{2\pi } {\int_{{r_1}}^{{r_2}} {{{\bar f}^2}} } (\rho ,\varphi ){\rm{d}}\rho {\rm{d}}\varphi ]}^{\frac{1}{2}}}}} = 1} \end{array} $$ (4) 当图像极坐标变换原点从[xc, yc]偏移至[x′c, y′c]后, 如图 2(b)所示, 通过极坐标变换得到:
$$ \left\{ {\begin{array}{*{20}{l}} {{\rho ^2} = {\rho ^{\prime 2}} + \Delta {\rho ^2} + 2\Delta \rho {\rho ^\prime } {\rm{cos}} ({\varphi ^\prime } - \Delta \varphi )}\\ { {\rm{tan}} {\varphi _f} = \frac{{{\rho ^\prime } {\rm{sin}} (\varphi _f^\prime ) + \Delta \rho {\rm{sin}} (\Delta \varphi )}}{{{\rho ^\prime } {\rm{cos}} (\varphi _f^\prime ) + \Delta \rho {\rm{cos}} (\Delta \varphi )}}} \end{array}} \right. $$ (5) 其中(Δρ, Δφ)是原点O′c在极坐标Ocp中的极坐标位置, 建立极轴Ocp与O′cp′平行, $\Delta \varphi = {\tan ^{ - 1}}\left( {\frac{{y_c^\prime - {y_c}}}{{x_c^\prime - {x_c}}}} \right), {\Delta _\rho } = {\left[ {{{\left( {x_c^\prime - {x_c}} \right)}^2} + {{\left( {y_c^\prime - {y_c}} \right)}^2}} \right]^{\frac{1}{2}}}$。
近似方程式(5)根据麦克劳林展开, 当Δρ≪ρ时可以表示为
$$ \rho \approx {\rho ^\prime } + \Delta \rho {\rm{cos}}{ ^\prime }({\varphi ^\prime } - \Delta \varphi ) $$ (6) 则无衍射灰度的分布可以表示为
$$ {f^\prime }({\rho ^\prime },{\varphi ^\prime }) \approx {\rm{A}} \cdot {J_0}\{ \alpha [{\rho ^\prime } + \Delta \rho {\rm{cos}} ({\varphi ^\prime } - \Delta \varphi )]\} $$ (7) 将(7)式替换为(2)式, 得到:
$$ \begin{array}{*{20}{l}} {\bar f({\rho ^\prime },{\varphi ^\prime }) = }\\ {\frac{{\int_0^{2\pi } {\rm{A}} \cdot {J_0}\{ \alpha [{\rho ^\prime } + \Delta \rho {\rm{cos}} ({\varphi ^\prime } - \Delta \varphi )]\} {\rm{d}}{\varphi ^\prime }}}{{2\pi }}} \end{array} $$ (8) 从f′(ρ′, φ′)和f′(ρ′, φ′)的相关计算中, 得到相关因子Q(x′c, y′c), 让χ(φ′)=Δρ cos(φ′-Δφ)
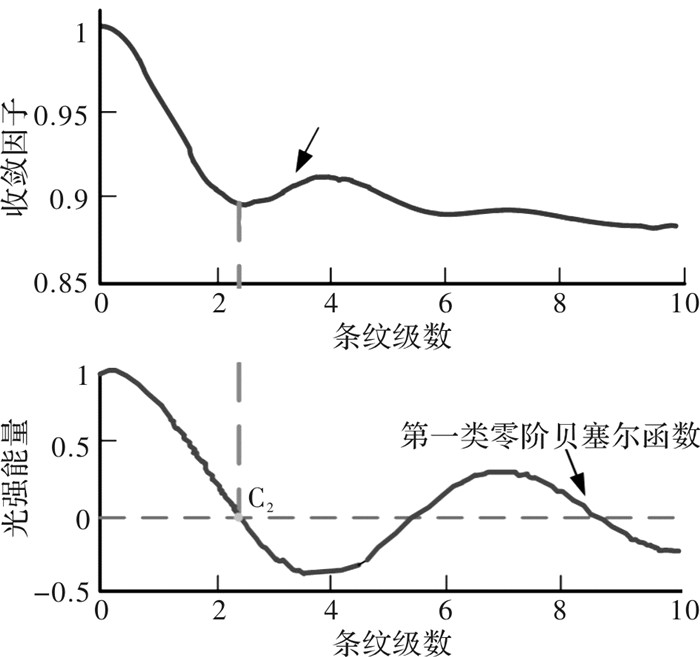
$$ \begin{array}{l} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} Q({x^\prime }_c,{y^\prime }_c) = \\ \frac{{\int_0^{2\pi } {\int_{{r_1}}^{{r_2}} {{J_0}} } [\alpha {\rho ^\prime } + \chi ({\varphi ^\prime })]{\rm{d}}\rho {\rm{d}}\varphi }}{{{{[\int_0^{2\pi } {\int_{{r_1}}^{{r_2}} {J_0^2} } [\alpha {\rho ^\prime } + \chi ({\varphi ^\prime })]{\rm{d}}\rho {\rm{d}}\varphi \cdot \int_0^{2\pi } {\int_{{r_1}}^{{r_2}} {{{\overline {{f^\prime }} }^2}} } ({\rho ^\prime },{\varphi ^\prime }){\rm{d}}\rho {\rm{d}}\varphi ]}^{\frac{1}{2}}}}} \end{array} $$ (9) 根据Cauchy-Schwarz不等式, (9)式相关因子Q的最大值接近或等于1, 只有当中心重合时, 才是圆环滤波的中心, 或者Oc与无衍射图像Oc′的共同中心。分析方程(9)和图 3表明, 当圆环滤波的中心坐标[x′c, y′c]重合于已定义的中心坐标[xc, yc]时, 相关因子Q(x′c, y′c)有峰值(近似或等于1)。此外Q是一个单调函数, 在间隔[0, 2.405]内仅能水平偏移圆心Δρ。由于贝塞尔函数是一个不可积的方程, 采用数字积分法求解单调区间, 因此在贝塞尔函数的零阶区域, 找到峰值的坐标位置Q(x′c, y′c), 则求出无衍射光斑的中心。
2 算法实现
根据上述原则, 迭代算法的设计如下:
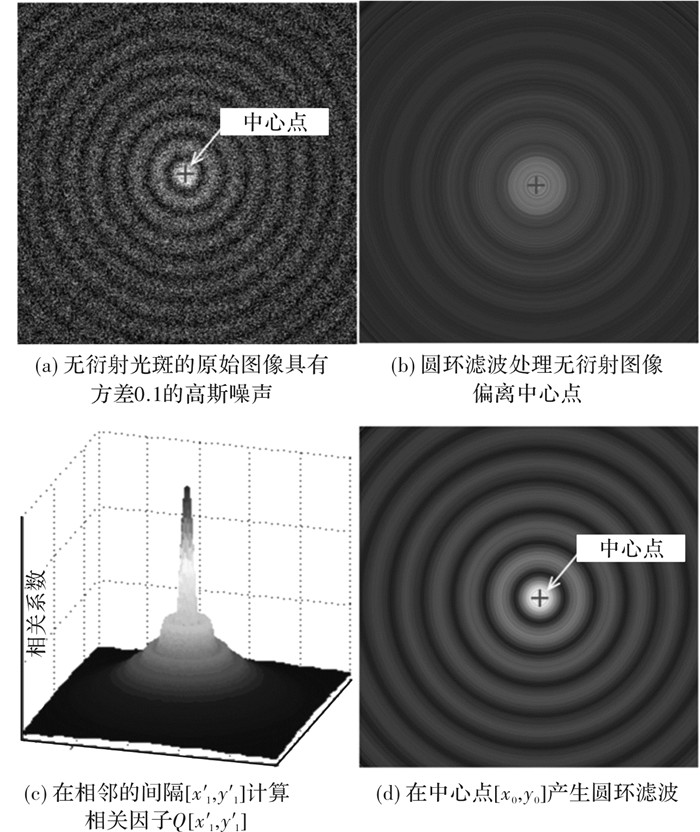
1) 对于给定的无衍射光斑f(ρ, φ)有假定的初始中心, 利用图像灰度重心法计算初始中心位置, 则初始中心坐标[x′1, y′1]为
$$ \left\{ {\begin{array}{*{20}{l}} {{x^\prime }_1 = \frac{{\int_0^{2\pi } {f({\rho _1},\varphi )} \rho {\rm{cos}} \varphi {\rm{d}}\varphi }}{{\int_0^{2\pi } {f(\rho ,\varphi )} {\rm{d}}\varphi }}}\\ {{y^\prime }_1 = \frac{{\int_0^{2\pi } {f(\rho ,\varphi )} \rho {\rm{sin}} \varphi {\rm{d}}\varphi }}{{\int_0^{2\pi } {f(\rho ,\varphi )} {\rm{d}}\varphi }}} \end{array}} \right. $$ (10) 2) 以坐标为中心, 对无衍射图像进行圆环滤波处理:
$$ \bar f(\rho ,\varphi ) = \frac{{\int_0^{2\pi } {{f_1}} (\rho ,\varphi )\rho {\rm{d}}\varphi }}{{2\pi \rho }} $$ (11) $\rho = \sqrt {{{\left( {x - x_1^\prime } \right)}^2} + {{\left( {y + y_1^\prime } \right)}^2}} $是极半径并且包含了无衍射图像。
3) 计算f(ρ, φ)和f(ρ, φ)的相关性, 相关因子Q(x′1, y′1)在[x′1, y′1]位置得到。
4) 步骤2)~3)重复不同的极半径间隔, 获得一系列数组Qi(x′1, y′1), 并且找到峰值Qi以及所对应的坐标[x′11, y′11], [x′12, y′12], …, [x′1N, y′1N]。
5) 计算无衍射图像的平均中心坐标[x0, y0]:
$$ {x_0} = \sum\limits_{i = 1}^N {{x^\prime }_{2i}} /N,{y_0} = \sum\limits_{i = 1}^N {{y^\prime }_{2i}} /N $$ (12) 根据上述步骤, 每个阶段的相应结果如图 4所示。
3 噪声灵敏度数值分析的算法
在实践中, 无衍射光受3种基本因素的影响:一是由图像传感器的激光散斑和电子噪声产生的随机光强分布; 二是Axicon锥面形貌的加工误差, 引起同心环形条纹波动起伏或断裂; 三是背景光环境噪声影响, 如胡椒盐噪声等。
为了验证算法的有效性, 用统计方法分析了噪声的影响, 并与其他算法进行了比较。首先生成一个无衍射的图像, 将其中心位置作为理想中心。然后利用计算机语言中的随机噪声函数(如Matlab中的Imnoise函数), 生成了一系列具有方差的噪声图像, 并将其添加到测量图像中。最后对无衍射图像的中心点进行迭代计算。表 1列出了不同算法的噪声效应的模拟结果。
表 1 不同算法中的噪声影响的数值分析Table 1. Numerical analysis of noise effects in different algorithms零均值高斯噪声σn 数字圆环滤波、圆环匹配的中心偏差σc 莫尔条纹的中心偏差[15]σm 霍夫变换的中心偏差[13]σh δ2=[N(x, y)] 偏移量/mm 时耗/s 偏移量/mm 时耗/s 偏移量/mm 时耗/s 0.010 0 0.059 8 10.257 0 0.452 0 30.452 0 1.206 0 76.035 0 0.005 0 0.038 8 10.304 0 0.275 0 30.720 0 0.993 0 80.084 0 0.002 5 0.020 5 10.427 0 0.206 0 30.672 0 0.468 0 79.862 0 0.001 0 0.015 1 10.579 0 0.187 0 30.585 0 0.245 0 79.633 0 σc、σm和σh分别为数字圆环滤波、莫尔条纹扫描定中算法和Hough变换定中位置与理论中心的偏离量与时耗。注意, 无衍射光的径向强度分布与中心位置的半径呈反比关系, 这说明零阶区的强度远大于外边缘的强度。因此当中心强度趋于1时, 方差为0.1的高斯噪声已经影响到了无衍射光斑灰度图像。而数字仿真实验表明, 利用本文所提出的算法, 计算出的中心位置误差小于0.1, 表明该算法误差远远小于图像传感器的一个传感器元件尺寸。基于相关因子的定中算法能实现亚像素定中精度, 相对于数字莫尔条纹扫描法、Hough变换、重心法等算法有较高的鲁棒性。
4 结论
基于大尺度空间中测量空间入射角需对无衍射光斑图像精确定中的前提, 本文提出了基于相关因子的无衍射光斑图像定中算法, 该算法在复杂噪声背景环境下能够快速精确定中, 并能实现非圆条纹和同心圆的识别。为无衍光束作为空间直线基准在实际应用中提供了新的有效定中方法。
-
表 1 不同算法中的噪声影响的数值分析
Table 1 Numerical analysis of noise effects in different algorithms
零均值高斯噪声σn 数字圆环滤波、圆环匹配的中心偏差σc 莫尔条纹的中心偏差[15]σm 霍夫变换的中心偏差[13]σh δ2=[N(x, y)] 偏移量/mm 时耗/s 偏移量/mm 时耗/s 偏移量/mm 时耗/s 0.010 0 0.059 8 10.257 0 0.452 0 30.452 0 1.206 0 76.035 0 0.005 0 0.038 8 10.304 0 0.275 0 30.720 0 0.993 0 80.084 0 0.002 5 0.020 5 10.427 0 0.206 0 30.672 0 0.468 0 79.862 0 0.001 0 0.015 1 10.579 0 0.187 0 30.585 0 0.245 0 79.633 0 -
[1] FAN K C, ZHAO Y.Laser straightness measurement system using optical fiber and modulation techniques[J]. International Journal of Machine Tools & Manufacture, 2000, 40:2073-2081. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ026184739/
[2] GILOAN M, GUTT R, SAPLACAN G.Optical chiral metamaterial based on meta-atoms with three-fold rotational symmetry arranged in hexagonal lattice[J]. Journal of Optics, 2015, 17(8):085102. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=7b719b3d014963625c443a1747a8cdf1
[3] LIN S T.A laser interferometer for measuring straightness[J].Optics and Laser Technology, 2001, 33:195-199. http://d.old.wanfangdata.com.cn/OAPaper/oai_pubmedcentral.nih.gov_3848380
[4] CHEN B Y, XU B, YAN L P, et al.Laser straightness interferometer system with rotational error compensation and simultaneous measurement of six degrees of freedom error parameters[J].Optics Express, 2015, 23:9052-9073. http://cn.bing.com/academic/profile?id=3e589e376251222e543c0d9326832e7a&encoded=0&v=paper_preview&mkt=zh-cn
[5] DURNIN J.Diffraction-free beams[J].Physical Review Letters, 1987, 58:1499-1501. http://d.old.wanfangdata.com.cn/Periodical/qjgylzs200911015
[6] DURNIN J.Exact solutions for non-diffracting beams I. The scalar theory[J].Journal of the Optical Society of America A-Optics Image Science and Vision, 1987, 4:651-654. doi: 10.1097-MOP.0b013e328331e873/
[7] WALDE M, JOST A, WICKER K, et al.Engineering an achromatic Bessel beam using a phase-only spatial light modulator and an iterative Fourier transformation algorithm[J].Optics Communications, 2017, 383:64-68. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=6123193e1ba727a78d487f8cd612c2ed
[8] 钟金钢, 梁智强, 李仕萍.激光自混合干涉角度测量参数优化及旋转方向判别[J].光学精密工程, 2016, 24(5):1001-1008 http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201605006 ZHONG Jingang, LIANG Zhiqiang, LI Shiping.Parameter optimization and direction recognition in angle measurement by laser self-mixing interference[J].Optics and Precision Engineering, 2016, 24(5):1001-1008. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201605006
[9] 景敏.基于影像仪测量直线度误差的优化方法研究[J].应用光学, 2016, 37(3):419-424. doi: 10.5768/JAO201637.0303004 JING Min.Optimization method of straightness error based on video measuring machine[J].Journal of Applied Optics, 2016, 37(3):419-424. doi: 10.5768/JAO201637.0303004
[10] 张新宝, 赵斌, 李柱.无衍射光莫尔条纹空间直线度误差的测量方法[J].华中理工大学学报, 2004, 6:121-126. http://d.old.wanfangdata.com.cn/Periodical/hzlgdxxb200007015 ZHANG Xinbao, ZHAO Bin, LI Zhu.Measurement method of spatial straightness error using non-diffracting technology[J].Journal of Huazhong University of Science and Technology, 2004, 6:121-126. http://d.old.wanfangdata.com.cn/Periodical/hzlgdxxb200007015
[11] 薛乐堂, 陈涛, 徐涛, 等.快速反射镜姿态角的高精度解算[J].光学精密工程, 2016, 24(8):2000-2009. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201608023 XUE Letang, CHEN Tao, XU Tao, et al.High-precision calculation for attitude angles of fast steering mirror[J]. Optics and Precision Engineering, 2016, 24(8):2000-2009. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201608023
[12] 马国鹭, 曾国英, 赵斌.基于无衍射姿态探针和全站仪组合测量空间隐藏坐标[J].光学精密工程, 2015, 16:1-6. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201502007 MA Guolu, ZENG Guoying, ZHAO Bin.Arago-Poisson diffraction spot observed in the shadow area of an axicon lens[J].Optics and Precision Engineering, 2015, 16:1-6. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201502007
[13] LIU Y, ZHOU S.Detecting point pattern of multiple line segments using Hough transformation[J]. Semiconductor Manufacturing, 2015, 28(1):13-24. http://cn.bing.com/academic/profile?id=29b90ed849c774edcbcef3b1e6493217&encoded=0&v=paper_preview&mkt=zh-cn
[14] WU J P, CHEN K, GAO X H.Fast and accurate circle detection using gradient-direction-based segmentation[J].Journal of the Optical Society of America A-Optics Image Science and Vision, 2013, 30:1184-1192. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0230013798/
[15] 赵斌.环栅图像的数字莫尔条纹扫描定中方法[J].光学精密工程, 2004, 43:2833-2839. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc200201004 ZHAO Bin. Digital Moiré fringe-scanning method for centering a circular fringe image[J].Optics and Precision Engineering, 2004, 43:2833-2839. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc200201004
[16] 左洋, 龙科慧, 刘金国, 等.非均匀采样莫尔条纹信号的分析与处理[J].光学精密工程, 2015, 23(04):1146-1152. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201504030 ZUO Yang, LONG Kehui, LIU Jinguo, et al.Analysis and processing of Moiré fringe signals based on non-uniform sampling[J].Optics and Precision Engineering, 2015, 23(04):1146-1152. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc201504030
[17] JUAREZ-REYES S A, ORTEGA-VIDALS P, SILVAORTIGOZA G.Wavefronts, light rays and caustic associated with the refraction of a spherical Gave by two interfaces: The axicon and the plano-convex parabolic lenses[J].Journal of Optics, 2015, 17 (6):1-23
[18] STUTI J, YADAV B K, KHAN M S, et al.Effect of coherence and polarization on the polychromatic partially coherent dark hollow beam generated from axicon-lens system[J].Journal of Optics, 2014, 16 (7):2433-2439. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=f05b0a68081a2e779f8a1b73b0efa703
-
期刊类型引用(2)
1. 姚林海,陆培国,杨永安,龙井宇,杨修林,卜英华. 光束指向稳定性高精度检测方法研究. 应用光学. 2022(02): 339-344 .  本站查看
本站查看
2. 丁善婷,程壮,翟中生,李劲松,黄娇洁,吕清花,王选择. 无衍射光莫尔条纹的中心定位方法. 应用光学. 2019(04): 652-657 .  本站查看
本站查看
其他类型引用(2)



 下载:
下载:

 陕公网安备 61011302001501号
陕公网安备 61011302001501号 