Directional error analysis of 2D galvanometer scanning system
-
摘要: 对比分析现有光束扫描系统的优劣，提出采用二维振镜扫描系统作为多光谱集成靶标的光轴调向装置，光轴调向装置的调向精度影响多光谱集成靶标的校准精度。通过对二维振镜扫描系统调向误差进行分析，建立二维振镜扫描系统调向模型，并进行了二维振镜扫描系统调向误差测量实验。结果表明:二维振镜扫描系统的方位角误差和俯仰角误差小于8″，调向角误差小于9″，调向误差小于10″，能够满足多光谱集成靶标光轴调向精度要求。Abstract: The advantages and disadvantages of existing beam scanning system were compared and analyzed.A 2D galvanometer scanning system was used as the optical axis alignment device of the multispectral integrated target.The alignment precision of the optical axis alignment device can affect the calibration accuracy of the multispectral integrated target. The directional error of 2D galvanometer scanning system was analyzed, the directional model of 2D galvanometer scanning system was established, and the measurement experiment of alignment error of 2D galvanometer scanning system was carried out. The results show that the azimuth error and pitch angle error of the 2D galvanometer scanning system are less than 8″, the alignment angle error is less than 9″, and the alignment error is less than 10″, which demonstrates that the optical axis alignment accuracy of multispectral integrated target can be satisfied.
-
引言
随着自由曲面技术和精密制造技术的快速发展，出现了多种复杂屈光度分布的多焦点镜片。渐进多焦点镜片作为一种特殊的多焦点镜片，具有非旋转对称性和渐变的屈光度分布，能够弥补传统的球面和非球面镜片无法同时视远及视近的缺陷[1-2]。然而，由于其复杂的曲面面形设计及加工工艺，现有的检测标准和检测设备均不适用于评价渐进多焦点镜片的成像质量[3]。
波前像差的测量在渐进多焦点镜片的加工设计及成像质量评价中具有重要作用。由于被测镜片的直径通常大于波前传感器接收孔径，目前主要是利用子孔径拼接的方法测量镜片的波前像差信息。该方法主要是将大口径镜片划分为多个测量子区域，利用小口径传感器分别实现子区域波前像差的检测。通过最小二乘法得到各自子波面的拼接参数，再利用波前重构复原出整片镜片的波前像差，这种测量方法对于仪器精度要求较高，测量过程及数据处理较为繁琐。本文提出一种渐进多焦点镜片波前像差的扩束测量方法，该方法操作便捷，测量效率高，能高效地实现对渐进多焦点镜片波前像差分布信息的测量。
1 镜片波前像差的测量方法
1.1 哈特曼-夏克波前传感器测量原理
Hartmann-Shack(H-S)传感器的核心部件是微透镜阵列和CCD探测器[4]。当有光波入射到H-S波前传感器时，微透镜阵列将对波前信息进行采集，每个微透镜在CCD探测器表面上会产生一个光斑，光斑质心位置取决于微透镜区域前的波前倾斜。如果入射光波是平面波，产生的光斑呈规则状排列，这时的光斑称为参考光斑，如图 1所示。如果入射光波含有像差，产生的光斑就会产生偏离，这时的光斑称为实际光斑。
![]()
图 1 畸变波前入射H-S传感器示意图Figure 1. Schematic diagram of distortion wavefront entering H-S sensorH-S传感器工作原理示意图如图 2所示，当实际入射波与参考平面波之间在y轴上存在夹角αy时，实际光斑与参考光斑在y轴上产生的偏移为Δy，用波前相位表示，则测量得到的实际波前与参考波前之间光斑的偏移量与局部波前斜率的关系可以表达为
$$ ~\frac{\partial }{\partial y}W\left( x, y \right)=~\frac{\Delta y}{{{f}_{L}}}~\text{tan}{{\alpha }_{y}}~ $$ (1) 同上述原理，在x轴方向上，光斑的偏移量与局部波前斜率的关系可以表达为
$$ \frac{\partial }{\partial x}W\left( x, y \right)=~\frac{\Delta x}{{{f}_{L}}}=\text{tan}{{\alpha }_{x}} $$ (2) 式中fL为微透镜与CCD探测器之间的距离，通常是微透镜的焦距。
由于Zernike多项式在单位圆内具有正交和归一化性质，使得其在波前重构中具有广泛的应用，测得的波前斜率可以复原成Zernike多项式的各阶系数[5-6]，用模式法重构得到的镜片波前像差表示为
$$ W\left( x, y \right)=\sum\limits_{k=1}^{n}{{{c}_{k}}{{z}_{k}}(x, y)}\text{ }~ $$ (3) 1.2 波前像差的扩束-缩束测量方法
由于激光传感器发射的激光光斑直径和H-S波前传感器接收口径均较小，分别选用扩束系统和缩束系统搭建测量光路，使得激光传感器发射的激光光束经过扩束系统后出射的光束直径与待测渐进多焦点镜片直径相配合，出射缩束系统后的光束直径与哈特曼-夏克波前传感器接收口径相配合。
设计的渐进多焦点镜片波前像差测量原理如图 3所示，其中L0是准直镜，L1、L2和L3组成可变焦扩束系统，L4是待测渐进多焦点镜片，L5是缩束镜。激光传感器发射的波面经过准直、扩束后覆盖待测渐进多焦点镜片的中心区域，波面透射待测渐进多焦点镜片后再经过缩束镜入射哈特曼波前传感器。
Taylor多项式在表示光学系统函数上有着重要的应用，波前像差可以用Taylor多项式展开，代表离焦项的Taylor多项式系数可以转化为传统屈光度参数中的球镜度数[7-8]，即有关系式：
$$ S={{W}_{4}}+{{W}_{6}}-\frac{2\text{ }\sqrt{\left[ {{\left( {{W}_{6}}-{{W}_{4}} \right)}^{2}}+W_{5}^{2} \right]}}{2}\text{ }~ $$ (4) 式中：S表示传统屈光度参数中的球镜度数，单位为m-1；W4、W5和W6为Taylor多项式的系数。
由于Zernike多项式的正交归一化及系数便于与初级像差转换等一系列优点，现今主要用Zernike多项式表示波前像差，Taylor多项式系数可与Zernike多项式系数之间相互转换[9]。
屈光度参数中的球镜度数S与Zernike多项式系数的关系为
$$ S=-\frac{2}{{{R}^{2}}}(2{{C}_{4}}-6{{C}_{12}}-\sqrt{C_{3}^{2}+C_{5}^{2}}~) $$ (5) 式中：C4、C5、C6和C12为Zernike多项式系数；R为瞳孔半径。
2 镜片的波前像差测量实验及结果分析
按照设计的渐进多焦点镜片波前像差测量系统光路搭建的实验装置如图 4所示。测量实验选取直径70 mm、屈光度参数为BASE-1.00D+ADD1.50D的外渐进多焦点镜片，调节位于精密导轨上的H-S波前传感器与缩束镜间的距离为157 mm。开始测量后，波长为655 nm的单模光纤耦合输出激光传感器发射光斑直径为2.2 mm的光束，光束经过准直后入射扩束比为10:1的扩束系统。经过扩束系统后的平行光束照射该片渐进多焦点镜片的中央直径为22 mm的圆形区域，接着出射光束经过焦距为175 mm的缩束镜后被入瞳直径为3.5 mm的H-S波前传感器接收检测。
![]()
图 4 镜片的波前像差测量实验装置图Figure 4. Experiment device for lens wavefront aberration measurement实验测得的渐进多焦点镜片的波前像差如图 5所示，测得的镜片波前像差的Zernike多项式表述中前四阶系数如表 1所示。从图 5和表 1中可以发现，镜片的波前像差主要由平移项、X及Y向倾斜项、0°及45°向像散项和离焦项组成，其余项对镜片像差影响较小。将各阶系数代入(4)式，即能得到渐进多焦点镜片波前像差的Zernike多项式表述。
表 1 Zernike多项式前四阶系数Table 1. First four order coefficients of Zernike polynomial序列号 阶 频 系数 类型 1 0 0 7.795 波面平移 2 1 -1 -1-- Y向倾斜 2.7 3 1 1 -1.365 X向倾斜 4 2 -2 -0.161 45°向像散 5 2 0 -0.239 离焦 6 2 2 -0.036 0°向像散 7 3 -3 -0.011 Y向三叶草 8 3 -1 -0.004 X向彗差 9 3 1 0.01 Y向彗差 10 3 3 -0.005 X向三叶草 11 4 -4 -0.001 Y向四频像差 12 4 -2 -0.001 Y向次级像散 13 4 0 0.01 三阶球差 14 4 2 -0.007 X向次级像散 15 4 4 0.001 X向四频像差 为了验证实验测量结果的准确性，设计对比试验，将镜片波前像差Zernike表述中的离焦项转换为球镜度后与传统方法测量得到的屈光度参数中的球镜度进行对比。H-S波前传感器测量得到镜片的波前像差Zernike表述中离焦项如图 6所示。将离焦项系数代入公式(9)得到镜片每个区域的球镜度，然后利用Matlab进行插值拟合，得到渐进多焦点镜片中间区域的球镜度分布如图 7所示。在渐进多焦点镜片的视远区中心点，镜片的球镜度约为-1.0 D，在视近区中心点，镜片的球镜度约为0.50 D。利用条纹偏折法测量该片渐进多焦点镜片[10-11]，得到整片渐进多焦点镜片的球镜度分布图如图 8所示，在视远区中心点，镜片的球镜度为-1.1 D，在视近区中心点，镜片的球镜度为0.49 D。
![]()
图 6 镜片波前像差Zernike表述中离焦项Figure 6. Defocus term in Zernike statement of lens wavefront aberration![]()
图 7 H-S波前传感器测量的球镜度分布图Figure 7. Distribution of sphere degree measured by H-S wavefront sensor![]()
图 8 条纹偏折法测量的球镜度分布图Figure 8. Distribution of sphere degree measured by ray deflection method采用两种不同的测量方法测量同一块渐进多焦点镜片，从图 7和图 8可以看出，两种检测结果得到渐进多焦点镜片渐变区直径为22 mm的圆形区域内的球镜度均是从约0.50 D递减到约为-1.0 D，整体渐变趋势基本保持一致。在视远区与视近区的中心点，两种方法测量得到的球镜度数接近，说明渐进多焦点镜片波前像差的扩束测量方法得到的测量结果是可行的。
3 结论
本文根据哈德曼-夏克波前传感器的测量原理，提出了一种采用扩束-缩束系统实现渐进多焦点镜片波前像差的测量方法，进行了球镜度测量计算公式的理论推导，通过实验检测得到了渐进多焦点镜片中央直径为22 mm的圆形区域内的波前像差，并将波前像差Zernike多项式表述中的离焦项转换为球镜度后与条纹偏折法测量的结果进行对比。实验结果表明, 渐进多焦点镜片波前像差的扩束测量方法可以高效测量渐进多焦点镜片的波前像差和球镜度，原理简单且实验简便快捷。
-
![]()
图 2 二维振镜扫描系统调向角分布图
Figure 2. Alignment angle distribution of 2D galvanometer scanning system
![]()
图 8 二维振镜扫描系统调向误差测量装置
Figure 8. Directional measuring device of 2D galvanometer scanning system
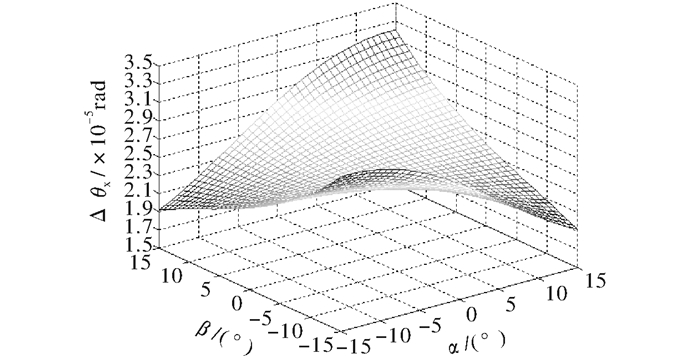
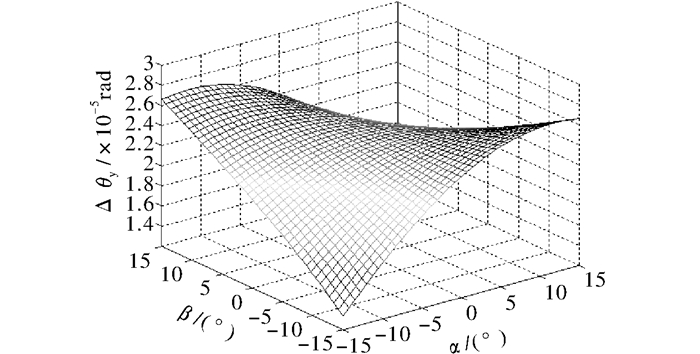
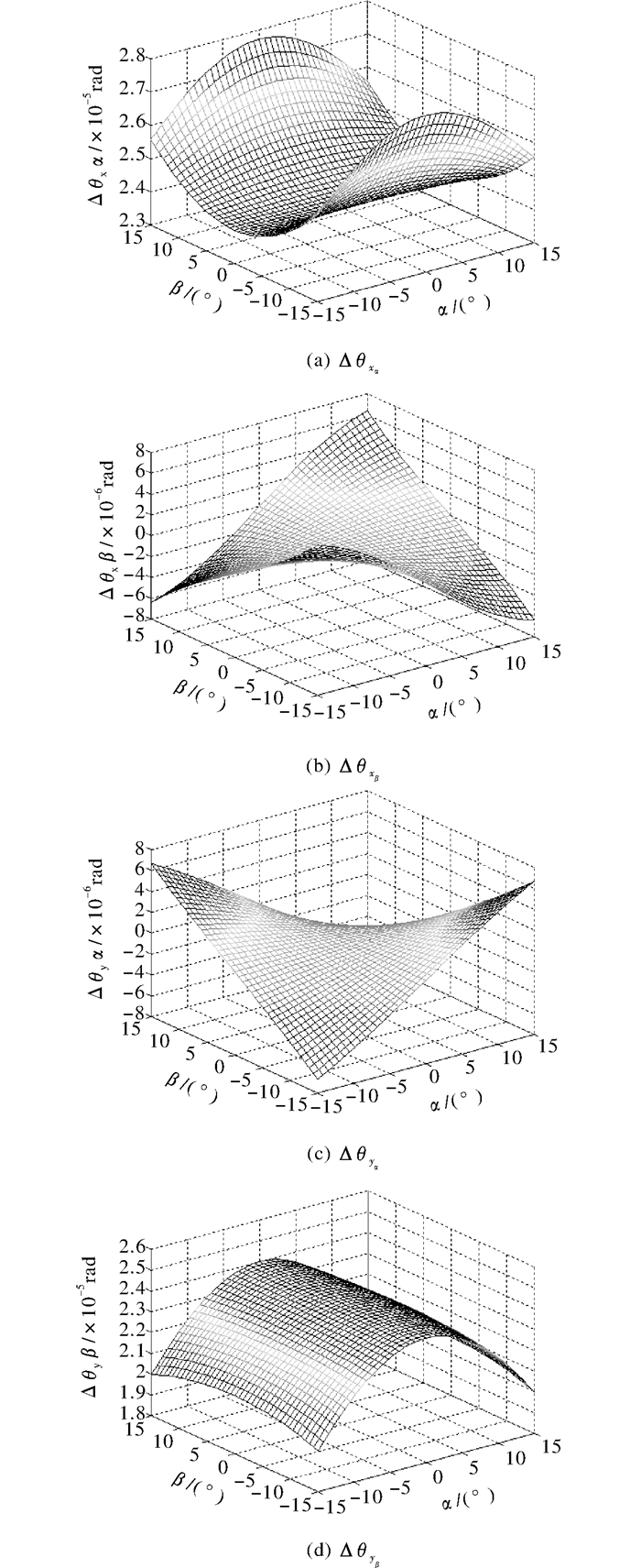
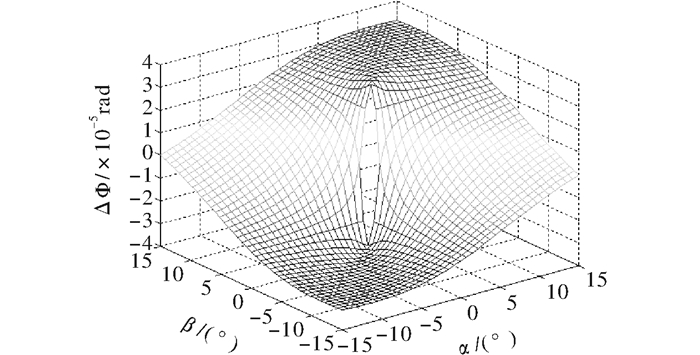
表 1 光轴调向测量数据(已减去基准值)
Table 1 Optical axis alignment measurement data (Reference value has been eliminated)
序号 α β θx θy φ θx′ θy′ Φ′ Δθx Δθy Δφ 1 -0°59′58″ -1°0′6″ -2°0′0″ -2°0′8″ 2°49′47″ -1°59′52″ -2°0′4″ 2°49′38″ 8″ 4″ -9″ 2 -1°0′4″ -0°0′2″ -2°0′8″ -0°0′4″ 2°0′8″ -2°0′15″ 0°0′1″ 2°0′15″ -7″ 5″ 7″ 3 -1°0′6″ 1°0′5″ -2°0′16″ 2°0′6″ 2°49′57″ -2°0′21″ 1°59′58″ 2°49′54″ -5″ -8″ -3″ 4 -0°0′2″ 0°59′55″ -0°0′4″ 1°59′50″ 1°59′50 -0°0′8″ 1°59′57″ 1°59′57″ -4″ 7″ 7″ 5 0°0′4″ 0°0′2″ 0°0′8″ 0°0′4″ 0°0′9″ 0°0′4″ -0°0′3″ 0°0′4″ -4″ -7″ -4″ 6 0°0′3″ -1°0′7″ 0°0′6″ -2°0′14″ 2°0′14″ 0°0′11″ -2°0′8″ 2°0′8″ 5″ 6″ -6″ 7 1°0′3″ -1°0′9″ 2°0′10″ -2°0′14″ 2°49′58″ 2°0′6″ -2°0′21″ 2°50′0″ -4″ -7″ -2″ 8 0°59′54″ 0°0′5″ 1°59′48″ 0°0′10″ 1°59′48″ 1°59′54″ 0°0′15″ 1°59′54″ 6″ 5″ 6″ 9 1°0′5″ 1°0′2″ 2°0′14″ 2°0′0″ 2°49′51″ 2°0′7″ 1°59′56″ 2°49′43″ -7″ -4″ -8″ 
下载: 导出CSV
-
[1] AYLWARD R P. Advanced galvanometer-based optical scanner design[J]. Sensor Review, 2003, 23(3):216-222. doi: 10.1108/02602280310481968
[2] DUMA V F. Optimal scanning function of a galvanometer scanner for an increased duty cycle[J]. Optical Engineering, 2010, 49(10):730-738. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=7c15ae7aac91ba1f306c4baa1665e20b
[3] BOSCO A, BOOGERT S T, BOORMAN G E, et al. A large aperture electro-optic deflector[J]. Applied Physics Letters, 2009, 94(21):1675-1678. http://cn.bing.com/academic/profile?id=f65cfc33bcb82380be1df2b5dee0aaaa&encoded=0&v=paper_preview&mkt=zh-cn
[4] 万玲玉, 卢智勇, 王戎承.一种8行任意扫描的电光扫瞄器[J].应用光学, 2011, 32(2):323-328. doi: 10.3969/j.issn.1002-2082.2011.02.027 WAN Lingyu, LU Zhiyong, WANG Rongcheng. Electro-optic laser scanner for arbitrary scanning in 8 rows[J].Journal of Applied optics, 2011, 32(2):323-328. doi: 10.3969/j.issn.1002-2082.2011.02.027
[5] 马辰昊, 付跃刚, 宫平, 等.一种激光雷达复合式扫描方法及试验[J].红外与激光工程, 2015, 44(11):3270-3275. doi: 10.3969/j.issn.1007-2276.2015.11.018 MA Chenhao, FU Yuegang, GONG Ping, et al. A composite scanning method and experiment of laser radar[J].Infrared and Laser Engineering, 2015, 44(11):3270-3275. doi: 10.3969/j.issn.1007-2276.2015.11.018
[6] 罗远, 贺岩, 胡善江, 等.基于声光扫描的三维视频激光雷达技术[J].中国激光, 2014, 41(08):27-33. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zgjg201408005 LUO Yuan, HE Yan, HU Shanjiang, et al. Three-dimensional video imaging lidar system based on acousto-optic laser scanning[J]. Chinese Journal of Lasers, 2014, 41(08):27-33. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=zgjg201408005
[7] 孙国斌, 弥谦.光学振镜转角与扫描角度变化关系的研究[J].西安工业大学学报, 2010, 30(05):421-424. doi: 10.3969/j.issn.1673-9965.2010.05.003 SUN Guobin, MI Qian.Research on the relationship between angle of optical galvanometer and scanning angle[J].Journal of Xi'an Technological University, 2010, 30(05):421-424. doi: 10.3969/j.issn.1673-9965.2010.05.003
[8] 郭亚晶, 唐顺兴, 姜秀青, 等.基于振镜扫描方式的光学元件表面损伤检测[J].光学学报, 2017, 37(06):125-131. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=gxxb201706015 GUO Yajing, TANG Shunxing, JIANG Xiuqing, et al. Damage inspection of optical surface based on galvanometer scanning[J]. Acta Optica Sinica, 2017, 37(06):125-131. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=gxxb201706015
[9] MNERIE C, DUMA V F. Mathematical model of a galvanometer-based scanner: simulations and experiments[J].SPIE, 2013, 8789:8789-1-15. http://cn.bing.com/academic/profile?id=6b26b84009c4826e3355a015db3a74c0&encoded=0&v=paper_preview&mkt=zh-cn
[10] 高明, 侯宏录.外场多光轴瞄准偏差测试的基准光轴建立方法[J].光子学报, 2008, 37(05):1029-1033. http://d.old.wanfangdata.com.cn/Periodical/gzxb200805040 GAO Ming, HOU Honglu. Method for establishing reference optical axis of outfield multi axis collimation deviation test[J]. Acta Photonica Sinica, 2008, 37(05):1029-1033. http://d.old.wanfangdata.com.cn/Periodical/gzxb200805040
[11] 叶露, 赵建川.多光谱多光轴设备光轴平行性测量装置研制[J].光机电信息, 2011, 28(12):32-36. http://d.old.wanfangdata.com.cn/Periodical/gjdxx201112007 YE Lu, ZHAO Jianchuan.Development of measuring optical-axis parallelism instrument for multispectraland multi axial facility[J]. OME Information, 2011, 28(12):32-36. http://d.old.wanfangdata.com.cn/Periodical/gjdxx201112007
[12] 金伟其, 王霞, 张其扬, 等.多光轴一致性检测技术进展及其分析[J].红外与激光工程, 2010, 39(03):526-531. doi: 10.3969/j.issn.1007-2276.2010.03.031 JIN Weiqi, WANG Xia, ZHANG Qiyang, et al. Technical progress and its analysis in detecting of multi axesparallelismsystem[J]. Infrared and Laser Engineering, 2010, 39(03):526-531. doi: 10.3969/j.issn.1007-2276.2010.03.031
[13] 陈志斌, 范磊, 秦梦泽, 等.基于二维振镜的多光谱集成靶标设计及应用[J].应用光学, 2017, 38(2):187-192. http://d.old.wanfangdata.com.cn/Periodical/yygx201702006 CHEN Zhibin, FAN Lei, QIN Mengze, et al. Multi-spectral target design and application based on two-dimensional galvanometer[J]. Journal of Applied Optics, 2017, 38(2):187-192. http://d.old.wanfangdata.com.cn/Periodical/yygx201702006
[14] 李雅灿, 邱丽荣, 张鹏嵩, 等.便携式多光轴平行性检校系统的研制[J].中国激光, 2012, 39(10):149-153. http://d.old.wanfangdata.com.cn/Conference/8561565 LI Yacan, QIU Lirong, ZHANG Pengsong, et al.Development of portable multi-optical axes parallelism calibration system[J].Chinese Journal of Lasers, 2012, 39(10):149-153. http://d.old.wanfangdata.com.cn/Conference/8561565
[15] 韩万鹏, 蒙文, 李云霞, 等.双振镜激光扫描的误差分析及校正方法[J].光电技术应用, 2011, 26(04):14-18. doi: 10.3969/j.issn.1673-1255.2011.04.005 HAN Wanpeng, MENG Wen, LI Yunxia, et al.Error analysis and correction methods of dual galvanometer scanning[J].Electro-optic Technology Application, 2011, 26(04):14-18. doi: 10.3969/j.issn.1673-1255.2011.04.005
[16] 贾和平, 史玉升, 谢军.振镜式激光扫描误差分析及几何校正算法[J].光电工程, 2007, 34(08):37-40. doi: 10.3969/j.issn.1003-501X.2007.08.009 JIA Heping, SHI Yusheng, XIE Jun. Error analysis of laser galvanometer scan system and its geometrical correction arithmetic[J]. Opto-Electronic Engineering, 2007, 34(08):37-40. doi: 10.3969/j.issn.1003-501X.2007.08.009
[17] 杨志卿, 吴登喜, 郑永超.二维光学扫描中扫描角度非线性研究[J].激光技术, 2004, 28(03):262-265. doi: 10.3969/j.issn.1001-3806.2004.03.009 YANG Zhiqing, WU Dengxi, ZHEN Yongchao.Study of angular non-linearity in 2-D optical scanning[J].Laser Technology, 2004, 28(03):262-265. doi: 10.3969/j.issn.1001-3806.2004.03.009
-
期刊类型引用(1)
1. 禹静，蒋威炜，沈小燕. 自由曲面镜片波前像差扩束-缩束拼接技术. 光子学报. 2019(08): 160-169 . 
百度学术
其他类型引用(1)
计量
- 文章访问数: 1049
- HTML全文浏览量: 576
- PDF下载量: 186
- 被引次数: 2
陕公网安备 61011302001501号 